Dual-channel supply chain decisions under asymmetric information with a risk-averse retailer

Abstract

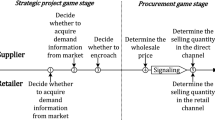
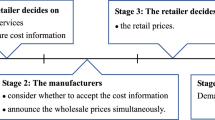
Many upstream brand manufacturers have established their direct channels to compete with the retailers; this is encroachment behavior by the manufacturers. In this paper, we introduce the retailer’s risk-averse behavior into the manufacturer’s encroachment problem under asymmetric information, focusing specifically on how the risk-averse behavior of the retailer and the per-unit selling cost of the manufacturer influence the optimal decisions. To address this problem, we assume that the market demand may be high or low, and each kind of demand follows a truncated normal distribution. The retailer has more information regarding the market size than the manufacturer. Under the mean–variance decision framework, we develop a dual-channel supply chain model and obtain three feasible regions of the optimal equilibrium results. We find that whether the profit of the manufacturer and the utility of the retailer are better off or worse off depends on the manufacturer’s per-unit selling cost and the degree of risk aversion of the retailer. Numerical experiments provide the comparisons of the expected profits and the utilities of both members in the supply chain under asymmetric information and symmetric information.

Appendix

Proof of Lemma 2 and Proposition 1

When the information is symmetric, the retailer has not mimicking incentives, the optimal order quantity follows $q_R (w;i)=\frac{\mu _i -2w+c-k\sigma }{2}$ (it is positive because of$w<\frac{\mu _i +c-k\sigma }{2})$. $\forall i,j\in \{H,L\}$, $i\ne j$, the retailer’s utility function is

$$\begin{aligned} V_{ij} (q_R )=\frac{1}{2}\left[ {2\mu _i -\mu _j -q_R (w;j)+c-2w-\sqrt{5}k\sigma } \right] q_R (w;j). \end{aligned}$$

(23)

Similarly, we have $V_{ii} (q_R )=\frac{1}{2}\left[ {\mu _i -q_R (w;i)+c-2w-k\sigma } \right] q_R (w;i)$, $\forall i\in \{H,L\}$. $\square$

Obviously, we have

$$\begin{aligned} V_{HH} \left( {\frac{\mu _H -2w+c-k\sigma }{2}} \right)= & {} \frac{1}{8}\left( {\mu _H -2w+c-k\sigma } \right) ^{2},\end{aligned}$$

(24)

$$\begin{aligned} V_{HL} \left( {\frac{\mu _L -2w+c-k\sigma }{2}} \right)= & {} \frac{1}{8}\left( {4\mu _H -3\mu _L -2w+c+k\sigma -2\sqrt{5}k\sigma } \right) \nonumber \\&\left( {\mu _L -2w+c-k\sigma } \right) , \end{aligned}$$

(25)

Then, we can obtain $V_{HL} \left( \frac{\mu _L -2w+c-k\sigma }{2}\right) \le \frac{1}{8}\left( {\mu _H -2w+c-k\sigma } \right) ^{2}$ and

$$\begin{aligned} \overline{{w}}=\frac{1}{4}\left( {3\mu _L -\mu _H +2c-2k\sigma } \right) . \end{aligned}$$

It is obvious that, if $V_{HL} \left( {\frac{\mu _L -2w+c-k\sigma }{2}} \right) \le V_{HH} \left( {\frac{\mu _H -2w+c-k\sigma }{2}} \right)$, then the retailer that observes a high market size has no incentive to mimic the ordering decision under a low market size. (Note that the retailer will never mimic the decision under a high market size, when the true market size is low). The condition can be written as $w\ge \overline{{w}}=\frac{3\mu _L -\mu _H +2c-2k\sigma }{4}$. Then, the next condition is satisfied, $V_{HL} \left( {\frac{\mu _L -2w+c-k\sigma }{2}} \right) >V_{HH} \left( {\frac{\mu _H -2w+c-k\sigma }{2}} \right)$, or identically, $w<\overline{{w}}$; then the retailer who observes a high market size may attempt to mimic the ordering decision under a low market size. Consequently, for a separating equilibrium to hold, the retailer, when observing a low market size, must downward distort order quantity to a level such that he would have no incentive to mimic when observing a high market size, i.e.,

$$\begin{aligned} V_{HL} (q_R (w;L))\le V_{HH} \left( {\frac{\mu _H -2w+c-k\sigma }{2}} \right) . \end{aligned}$$

By expanding the condition

$$\begin{aligned} \frac{1}{2}\left[ {2\mu _H -\mu _L -2w+c-q_R (w;L)-\sqrt{5}k\sigma } \right] q_R (w;L)\le \frac{1}{8}\left( {\mu _H -2w+c-k\sigma } \right) ^{2}, \end{aligned}$$

we obtain the threshold order quantity is

$$\begin{aligned} \overline{{q}}_R (w)=\frac{1}{2}(2\mu _H -\mu _L -2w+c-k\sigma )-\frac{1}{2}\sqrt{B}, \end{aligned}$$

(26)

where $B=\left( {\mu _H -\mu _L } \right) \left( {3\mu _H -\mu _L -4w+2c-2k\sigma } \right)$.

We can verify that

(27)

where and the manufacturer’s quantity $q_M (w;i)=\frac{\mu _i -q_R (w;i)-c}{2}$. In conclusion, a separating equilibrium is constituted.

Proof of Proposition 2

When $w\ge \overline{{w}}$, the retailer will not deceive the manufacturer and thus $q_R (w;i)=\frac{\mu _i -2w+c-k\sigma }{2}$. The manufacturer’s optimal expected profit in the natural separating equilibrium is (NS denotes natural separating, i.e., without distortion):

$$\begin{aligned} E\left[ {\prod \nolimits _M^{NS} (w)} \right]= & {} w\left[ {\lambda \left( {\frac{\mu _H -2w+c-k\sigma }{2}} \right) +(1-\lambda )\left( {\frac{\mu _L -2w+c-k\sigma }{2}} \right) } \right] \nonumber \\&+\,\lambda \left( {\frac{\mu _H +2w-3c+k\sigma }{4}} \right) ^{2}+(1-\lambda )\left( {\frac{\mu _L +2w-3c+k\sigma }{4}} \right) ^{2}.\nonumber \\ \end{aligned}$$

(28)

When $w\le \overline{{w}}$, the retailer distorts his order quantity when the market size is low and thus $q_R (w;L)=\overline{{q}}_R (w)$. The optimal expected profit of the manufacturer is (SD denotes separating with distortion):

$$\begin{aligned} E\left[ {\prod \nolimits _M^{SD} (w)} \right]= & {} w\left[ {\lambda \left( {\frac{\mu _H -2w+c-k\sigma }{2}} \right) +(1-\lambda )\overline{{q}}_R (w)} \right] \nonumber \\&+\,\lambda \left( {\frac{\mu _H +2w-3c+k\sigma }{4}} \right) ^{2}+(1-\lambda )\left( {\frac{\mu _L -\overline{{q}}_R (w)-c}{2}} \right) ^{2}. \end{aligned}$$

(29)

$\square$

The manufacturer sets the best wholesale price according to the above two types of separating equilibrium; that is to say, the manufacturer can choose the better results by solving the next two programs with constraint.

$$\begin{aligned} \begin{array}{l} \max \quad E\left[ \displaystyle \prod \nolimits _M^{NS} (w)\right] \\ \hbox {s.t. }w\ge \overline{{w}} \\ \end{array}\quad \hbox {and}\quad \begin{array}{l} \max \quad E\left[ \displaystyle \prod \nolimits _M^{SD} (w)\right] \\ \hbox {s.t. }w<\overline{{w}} \\ \end{array} \end{aligned}$$

The first order condition of $E\left[ {\prod \nolimits _M^{NS} (w)} \right]$ reduces to the unconstrained optimal solution

$$\begin{aligned} w^{AI}=w^{NS*}=\frac{3\mu -c-k\sigma }{6}. \end{aligned}$$

The first derivative of $E[ {\prod \nolimits _M^{SD} (w)} ]$ follows

$$\begin{aligned} \frac{dE\left[ {\prod \nolimits _M^{SD} (w)} \right] }{dw}= & {} \frac{\lambda }{4}\left( {3\mu _H -6w-c-k\sigma } \right) +\frac{1}{4}(1-\lambda )\nonumber \\&\times \left( {\mu _H +2\mu _L -6w-c-k\sigma } \right) \left( {1-\frac{M}{N}} \right) , \end{aligned}$$

(30)

where $\frac{M}{N}=\frac{\sqrt{\mu _H -\mu _L }}{\sqrt{3\mu _H -\mu _L +2c-4w-2k\sigma }}$, which yield the first order condition

$$\begin{aligned} \lambda \left( {3\mu _H -6w-c-k\sigma } \right) +(1-\lambda )\left( {\mu _H +2\mu _L -6w-c-k\sigma } \right) \left( {1-\frac{M}{N}} \right) =0. \end{aligned}$$

(31)

The first-order condition of $\overline{{q}}_R (w)$ is

$$\begin{aligned} \frac{d\overline{{q}}_R (w)}{dw}=-1+\frac{1}{\sqrt{C}}\left( {\mu _H -\mu _L } \right) >-1, \end{aligned}$$

where $C=\left( {\mu _H -\mu _L } \right) \left( {\mu _H -\mu _L +2(\mu _H -2w+c)-2k\sigma } \right) =M^{2}N^{2}$.

According to the condition $\frac{\sqrt{\mu _H -\mu _L }}{\sqrt{3\mu _H -\mu _L +2c-4w-2k\sigma }}=\frac{M}{N}=\frac{M}{\sqrt{G+M^{2}}}$, where $G=2(\mu _H -2w+c-k\sigma )$, when $w<\overline{{w}},\overline{{w}}=\frac{1}{4}\left( {3\mu _L -\mu _H +2c-2k\sigma } \right) ,G>3\left( {\mu _H -\mu _L } \right) >0$, then $0<\frac{M}{N}<1$.

The solution of (23) is $w_f =\frac{\mu _H +2\mu _L -c-k\sigma }{6}$ when $\lambda =0$, and $w_f =\frac{1}{6}\left( {3\mu _H -c-k\sigma } \right)$ when $\lambda =1$, where $\frac{M}{N}=\frac{\sqrt{\mu _H -\mu _L }}{\sqrt{3\mu _H -\mu _L +2c-4w-2k\sigma }}$.

Notice that when $w<\overline{{w}}$, which implies $0<1-\frac{M}{N}<1$. One can be shown by the implicit function theorem that $w_f$ increases in $\lambda$. Thus, for $\frac{dE[ {\prod \nolimits _M^{NS} (w)} ]}{dw}=0$ to hold for $w_f <\overline{{w}}$, we must have $3\mu _H -c-k\sigma -6w_f >0$ and $\mu _H +2\mu _L -c-k\sigma -6w_f <0$ (given $3\mu _H -c-k\sigma >\mu _H +2\mu _L -c-k\sigma$).

Then,

$$\begin{aligned}&\frac{dE\left[ {\prod \nolimits _M^{SD} (w_f )} \right] }{dw}\ge \frac{1}{4}\left[ {\lambda \left( {3\mu _H \!-\!6w_f \!-\!c\!-\!k\sigma } \right) \!+\!(1\!-\!\lambda )\left( {\mu _H \!+\!2\mu _L \!-\!6w_f \!-\!c\!-\!k\sigma } \right) } \right] \\&\quad >\frac{1}{4}\left[ {3\mu -c-6w_f -k\sigma } \right] . \end{aligned}$$

Due to $\frac{dE[ {\prod \nolimits _M^{SD} (w)} ]}{dw}<0$, that is to say,

$$\begin{aligned}&\frac{dE\left[ {\prod \nolimits _M^{SD} (w)} \right] }{dw}=\frac{\lambda }{4}\left( {3\mu _H -6w-c-k\sigma } \right) +\frac{1}{4}(1-\lambda )\left( {\mu _H +2\mu _L -6w-c-k\sigma } \right) \\&\quad \times \,\left( {1-\frac{M}{N}} \right) <0,\hbox { and }\frac{1}{4}\left[ {3\mu -c-6w_f -k\sigma } \right] <0. \end{aligned}$$

Then we obtain that if $\frac{dE[ {\prod \nolimits _S^{NS} (w)} ]}{dw}=0$ has a solution $w_f \in (0,\overline{{w}})$, which asserts that the unconstrained maximizer, $w_f$, of $E[ {\prod \nolimits _M^{SD} (w)} ]$ must be larger than $\frac{3\mu -c-k\sigma }{6}$ i.e., the unconstrained maximizer of $E[ {\prod \nolimits _M^{NS} (w)} ]$. Note that we can derive the second and third derivatives of $E[ {\prod \nolimits _M^{SD} (w)} ]$. In particular, the third derivative $\frac{d^{3}E[ {\prod \nolimits _M^{SD} (w)} ]}{dw^{3}}$ is always positive when $w\le \overline{{w}}$ and thus the second derivative $\frac{d^{2}E[ {\prod \nolimits _M^{SD} (w)} ]}{dw^{2}}$ is increasing when $w\le \overline{{w}}$. Therefore, there at most exists one solution of $\frac{dE[ {\prod \nolimits _M^{SD} (w)} ]}{dw}=0$ in $(0,\overline{{w}})$.

$$\begin{aligned} \overline{{w}}=\frac{3\mu _L -\mu _H +2c-2k\sigma }{4}\le \frac{3\mu -c-k\sigma }{6},\hbox { then }c<\frac{3\left( {1+2\lambda } \right) (\mu _H -\mu _L )+4k\sigma }{8}. \end{aligned}$$

Note that $E[ {\prod \nolimits _M^{NS} (w)} ]$ and $E[ {\prod \nolimits _M^{SD} (w)} ]$ coincide at $w=\overline{{w}}$ because the retailer’s order quantity in the equilibrium without distortion coincides with that with distortion at $w=\overline{{w}}$. Therefore, if $\overline{{w}}\le w^{NS*}=\frac{3\mu -c-k\sigma }{6}$, or identically, $c\le \frac{3( {1+2\lambda } )(\mu _H -\mu _L )+4k\sigma }{8}$, then $w^{NS*}$ induces a natural separating equilibrium; moreover, $E[ {\prod \nolimits _S^{SD} (w)} ]$ must be increasing at $w=\overline{{w}}$ given that $E[ {\prod \nolimits _M^{SD} (w)} ]$ is increasing at $w=0$ and any solution of the first order condition of $E[ {\prod \nolimits _M^{SD} (w)} ]$ is larger than $w^{NS*}$ or $\overline{{w}}$. Hence, if $c\le \frac{3( {1+2\lambda } )(\mu _H -\mu _L )+4k\sigma }{8}$, $\overline{{w}}<w^{NS*}$, $w^{AI}=w^{NS*}=\frac{3\mu -c-k\sigma }{6}$ is the manufacturer’s optimal wholesale price. In contrast, if $\overline{{w}}>w^{NS*}$, or identically, $c>\frac{3( {1+2\lambda } )(\mu _H -\mu _L )+4k\sigma }{8}$, then $w^{NS*}$ does not induce a natural separating equilibrium and the corner solution $\overline{{w}}$ would be the manufacturer’s best choice for achieving a natural separating equilibrium. Note that if $\frac{dE[ {\prod \nolimits _M^{SD} (w)} ]}{dw}=0$ has a solution $w_f \in (0,\overline{{w}})$, then $w_f$ induces the distorted separating equilibrium which is the optimal solution. If $\frac{dE[ {\prod \nolimits _M^{SD} (w)} ]}{dw}=0$ does not have a solution in $(0,\overline{{w}})$, then $E[ {\prod \nolimits _M^{SD} (w)} ]$ must be increasing in $(0,\overline{{w}})$ and the corner solution $\overline{{w}}$ will be the manufacturer’s optimal wholesale price. Given the optimal wholesale price, we can directly obtain the retailer’s order quantity and then the manufacturer’s direct selling quantity.

Notice that given the optimal wholesale price $w^{AI}=\frac{3\mu -k\sigma -c}{6}$ in the separating equilibrium without distortion, the manufacturer’s optimal expected profit can be derived as:

$$\begin{aligned} \prod \nolimits _M^{^{AI}} =\frac{1}{16}\left[ {\frac{4}{3}(k^{2}\sigma ^{2}+3\mu ^{2})+\left( {9+\frac{1}{3}} \right) c^{2}-\frac{8c}{3}(2k\sigma +3\mu )+\sigma ^{2}} \right] . \end{aligned}$$

(32)

where $D=\lambda (\mu _H -\mu )^{2}+(1-\lambda )(\mu _L -\mu )^{2}=\lambda \mu _H ^{2}+(1-\lambda )\mu _L ^{2}-\mu ^{2}$. The manufacturer’s expected profit in the separating equilibrium with distortion cannot be explicitly expressed.

The last step is to characterize the boundary conditions such that the manufacturer’s and the retailer’s selling quantities are strictly positive. If the manufacturer’s selling cost is low, the retailer’s selling quantity under a low market size will first go to zero. This always happens in a natural separating equilibrium, i.e., when $c\le \frac{3\left( {1+2\lambda } \right) (\mu _H -\mu _L )+4k\sigma }{8}$ (when the retailer’s order quantity goes to zero under a low market size, the retailer would never mimic such an ordering decision when the market size is high; thus, it must be a separating equilibrium without distortion). It can be easily shown that when $c>\frac{3\left( {1+2\lambda } \right) (\mu _H -\mu _L )+4k\sigma }{8}$, the retailer’s order quantity is positive. On the contrary, as the manufacturer’s selling cost increases, the manufacturer’s direct selling quantity may go to zero. Given $\mu _H$ and $\mu _L$, we define the smallest threshold $\overline{{c}}(\lambda )$ that can be implicitly determined at which the manufacturer’s direct selling quantity goes to zero under at least one market size.

Proof of Part (i) The manufacturer can also choose a wholesale price such that the retailer does not place a low market size order. The manufacturer’s optimal expected profit in this case is

$$\begin{aligned} \prod \nolimits _M^{AI}= & {} \lambda \left[ {\left( {\frac{\mu _H -2w^{AI}+c-k\sigma }{2}} \right) w^{AI}+\left( {\frac{\mu _H +2w^{AI}-3c+k\sigma }{4}} \right) ^{2}} \right] \nonumber \\&+\,(1-\lambda )\left( {\frac{\mu _L -c}{2}} \right) ^{2}, \end{aligned}$$

(33)

The retailer’s expected utility is

$$\begin{aligned} E\left[ {U_R } \right] =\frac{1}{8}\left( {\mu _H +c-2w-k\sigma } \right) \left[ {\lambda \left( {\mu _H +c-2w+k\sigma } \right) -2\sqrt{\lambda }k\sigma } \right] . \end{aligned}$$

(34)

There exists a unique maximizer $w^{AI}=\frac{3\mu _H -k\sigma -c}{6}$ and the subgame equilibrium follows directly: $q_R^{AI} (H)=\frac{4c}{3},q_R^{AI} (L)=0$.

When $c\in \left( {\max \left\{ {\frac{-3\sqrt{\lambda }(\mu _H -\mu _L )+2k\sigma }{4},0} \right\} ,\frac{3\sqrt{\lambda }(\mu _H -\mu _L )+2k\sigma }{4}} \right)$, the optimal expected utility of the retailer is $U_R^{AI} =\frac{2c}{9}\lambda k\sigma -\frac{4c}{3}k\sigma \sqrt{\lambda }$ and the optimal expected profit of the manufacturer is

$$\begin{aligned} \prod \nolimits _M^{AI} =\frac{c^{2}(4\lambda +3)-c\left( {6\mu +4k\lambda \sigma } \right) +\lambda k^{2}\sigma ^{2}+3\left( {\mu ^{2}+D} \right) }{12}. \end{aligned}$$

(35)

In Region $R_1$, the optimal expected utility of the retailer is

$$\begin{aligned} U_R ^{AI}=\frac{2c}{9}\lambda k\sigma -\frac{4c}{3}k\sigma \sqrt{\lambda }. \end{aligned}$$

(36)

In Region $R_2$, the retailer’s optimal expected utility is

$$\begin{aligned} U_R ^{AI}= & {} \frac{1}{72}\lambda (3\mu _H -3\mu +4c+5k\sigma )(3\mu _H -3\mu +4c-3k\sigma ) \nonumber \\&+\,\frac{1}{72}(1-\lambda )(3\mu _L -3\mu +4c+5k\sigma )(3\mu _L -3\mu +4c-3k\sigma ) \nonumber \\&-\,\frac{1}{6}k\sigma \sqrt{\lambda \left( {3\mu _H -3\mu +4c-3k\sigma } \right) ^{2}+(1-\lambda )\left( {3\mu _L -3\mu +4c-3k\sigma } \right) ^{2}}.\quad \quad \quad \end{aligned}$$

(37)

Proof of Proposition 3

We first show that in Region $R_1$, whether the manufacturer will benefit or hurt from encroachment depends on additional conditions c and k. In this region, with encroachment, the natural separating equilibrium arises and we can obtain the manufacturer’s expected profit. From Sect. 2.1, we know that without encroachment, the manufacturer’s optimal expected profit is $\prod \nolimits _M^N =\frac{\left( {\mu -k\sigma } \right) ^{2}}{8}$ with the presumption that $0<k<\frac{2\mu _L -\mu }{\sigma }\,\psi$ (the retailer is induced to order a positive quantity in both market scenarios).

Thus, we derive:

$$\begin{aligned} \prod \nolimits _M^{AI} -\prod \nolimits _M^N =\frac{1}{16}\left[ {\left( 1+\frac{1}{3}\right) c^{2}+2(\mu -2c)^{2}+\sigma ^{2}+\frac{2}{3}k\sigma (6\mu -k\sigma -8c)} \right] . \end{aligned}$$

(38)

Let $h_1 =\frac{3}{56}\left( {8\mu +\frac{16}{3}k\sigma } \right) -E$, where

$$\begin{aligned} E=\frac{3}{14}\sqrt{4\left( {\mu +\frac{2}{3}k\sigma } \right) ^{2}-\frac{7}{3}\left( {\lambda \mu _H ^{2}+(1-\lambda )\mu _L ^{2}+\mu ^{2}+\frac{2}{3}k\sigma (6\mu -k\sigma )} \right) }. \end{aligned}$$

Second, when we investigate Region $R_2$, we obtain the following results. By solving $\prod \nolimits _M^{AI} -\prod \nolimits _M^N >0$, the feasible range of c is $c>\frac{3}{56}\left( {8\mu +\frac{16}{3}k\sigma } \right) +E$ or $0\le c\le \max \{h_1 ,0\}$; that is, in this range, the manufacturer will benefit from encroachment under this situation. Correspondingly, if $\max \{h_1 ,0\}<c<h_2$, where $h_2 =\frac{3}{56}\left( {8\mu +\frac{16}{3}k\sigma } \right) +E$, we obtain $\prod \nolimits _M^{AI} -\prod \nolimits _M^N <0$; in other words, the manufacturer will hurt from encroachment under this situation. $\square$

In the next section, we investigate Region $R_1$, we derive that

$$\begin{aligned} \prod \nolimits _M^{AI} -\prod \nolimits _M^N= & {} \frac{c^{2}(4\lambda +3)-c\left( {6\mu +4k\lambda \sigma } \right) +\lambda k^{2}\sigma ^{2}+3\left( {\mu ^{2}+D} \right) }{12}-\frac{\left( {\mu -k\sigma } \right) ^{2}}{8}\nonumber \\= & {} \frac{1}{24}\left( {(8\lambda +6)c^{2}-(12\mu +8\lambda k\sigma )c} \right) +\frac{1}{24} \nonumber \\&\times \,\left( {6\mu k\sigma +(2\lambda -3)k^{2}\sigma ^{2}+3\mu ^{2}+6\left( {\lambda \mu _H ^{2}+(1-\lambda )\mu _L ^{2}-\mu ^{2}} \right) } \right) .\qquad \quad \end{aligned}$$

(39)

By solving $\prod \nolimits _M^{AI} -\prod \nolimits _M^N =0$, we obtain $c=g_1$ or $c=g_2$, where

$$\begin{aligned} g_1= & {} \frac{6\mu +4\lambda k\sigma -F}{2(4\lambda +3)},\quad g_2 =\frac{6\mu +4\lambda k\sigma +F}{2(4\lambda +3)},\\ F= & {} \sqrt{4(3\mu \!+\!2\lambda k\sigma )^{2}\!-\!2(4\lambda \!+\!3)\left( {6\mu k\sigma \!+\!(2\lambda \!-\!3)k^{2}\sigma ^{2}\!-\!3\mu ^{2}\!+\!6\left( {\lambda \mu _H ^{2}+(1-\lambda )\mu _L ^{2}} \right) } \right) }. \end{aligned}$$

In Region $R_1$, we obtain the following result. If $c<g_1$ or $c>g_2$, $\prod \nolimits _M^{AI} -\prod \nolimits _M^N >0$. Otherwise, $g_1 <c<g_2$, then we obtain $\prod \nolimits _M^{AI} -\prod \nolimits _M^N <0$.

Proof of Proposition 4

Notice that with manufacturer encroachment, in Region $R_1$, the retailer’s optimal expected utility is

$$\begin{aligned} U_R^{AI} =\frac{2c}{9}\lambda k\sigma -\frac{4c}{3}k\sigma \sqrt{\lambda }. \end{aligned}$$

(40)

In Region $R_2$, the optimal expected utility of the retailer $U_R^{AI}$ is

$$\begin{aligned} U_R^{AI}= & {} \frac{1}{72}\lambda (3(1-\lambda )(\mu _H -\mu _L )+4c+5k\sigma )(3(1-\lambda )(\mu _H -\mu _L )+4c-3k\sigma ) \nonumber \\&+\,\frac{1}{72}(1-\lambda )(-3\lambda (\mu _H -\mu _L )+4c+5k\sigma )(-3\lambda (\mu _H -\mu _L )+4c-3k\sigma ) \nonumber \\&-\,\frac{1}{6}k\sigma _H \left( {3(1-\lambda )(\mu _H -\mu _L )+4c-3k\sigma } \right) . \end{aligned}$$

(41)

In feasible Region $R_2$, when all the parameters are fixed except $c, \frac{\partial U_R^{AI} }{\partial c}=\frac{1}{9}(4c-5k\sigma )$. For any given k, when $c>\frac{5}{4}k\sigma , U_R^{AI}$ increases in c. When $c<\frac{5}{4}k\sigma , U_R^{AI}$ decreases in c. When $c=\frac{5}{4}k\sigma , U_R^{AI}$ achieve the maximum value. $\square$

The retailer’s optimal expected utility without manufacturer encroachment $U_R^N$ is

$$\begin{aligned} U_R^N= & {} \frac{D+\mu k\sigma }{4}+\frac{(\mu -3k\sigma )(\mu +k\sigma )}{16}\nonumber \\&-\,\frac{1}{2}k\sigma \sqrt{4(D+\mu ^{2})+(\mu +k\sigma )^{2}-4\mu }, \end{aligned}$$

(42)

which is independent of c.

Comparing (40) and (42), we obtain

$$\begin{aligned} \frac{2c}{9}\lambda k\sigma -\frac{4c}{3}k\sigma \sqrt{\lambda }= & {} \frac{(\lambda \mu _H ^{2}+(1-\lambda )\mu _L ^{2})-\mu (\mu -k\sigma )}{4}+\frac{(\mu -3k\sigma )(\mu +k\sigma )}{16}\\&-\frac{1}{2}k\sigma \sqrt{4(\lambda \mu _H ^{2}+(1-\lambda )\mu _L ^{2})+(\mu +k\sigma )^{2}-4\mu }, \end{aligned}$$

which yields a threshold

$$\begin{aligned} c_R^l (\lambda )= & {} \frac{9}{2\sqrt{\lambda }k\sigma (\sqrt{\lambda }-6)}\left( {\frac{(A+\mu ^{2})-\mu (\mu -k\sigma )}{4}+\frac{(\mu -3k\sigma )(\mu +k\sigma )}{16}} \right) \nonumber \\&-\,\frac{9}{4\sqrt{\lambda }(\sqrt{\lambda }-6)}\sqrt{4(A+\mu ^{2})+(\mu +k\sigma )^{2}-4\mu }, \end{aligned}$$

(43)

where the two utilities of the retailer are equal; in the same manner, comparing (41) with (42) generates another threshold $c_R^h (\lambda )$, which is an implicit function of $\lambda$. If $\max \left\{ {\frac{-3\sqrt{\lambda }(\mu _H -\mu _L )+2k\sigma }{4},\frac{k\sigma }{2}} \right\} <c_R^l (\lambda )\le \min \left\{ {\frac{3\sqrt{\lambda }(\mu _H -\mu _L )+2k\sigma }{4},3\mu _H -k\sigma ,\frac{3\mu _H +k\sigma }{5}} \right\}$, the retailer is worse off in $c\in \left( {\max \left\{ {\frac{-3\sqrt{\lambda }(\mu _H -\mu _L )+2k\sigma }{4},\frac{k\sigma }{2}} \right\} ,c_R^l (\lambda )} \right]$ and better off in $c\in \left( {c_R^l (\lambda ),\;\min \left\{ {\frac{3\sqrt{\lambda }(\mu _H -\mu _L )+2k\sigma }{4},3\mu _H -k\sigma ,\frac{3\mu _H +k\sigma }{5}} \right\} } \right]$; otherwise, if $c_R^h (\lambda )<b$, then the retailer is worse off when the manufacturer’s per-unit selling cost $c\in \left( {\left. {\max \left\{ {\frac{2k\sigma +3\lambda (\mu _H -\mu _L )}{4},\frac{3\sqrt{\lambda }(\mu _H -\mu _L )+2k\sigma }{4}} \right\} ,c_R^h (\lambda )} \right] } \right.$ and better off in $c\in \left( {\left. {c_R^h (\lambda ),b} \right] } \right.$. In conclusion, whether the retailer is better off or worse off by manufacturer encroachment depends on the limitative range of c and k. Based on the above results, we obtain a threshold $c_R (\lambda )$ that is either $c_R^l (\lambda )$, $c_R^h (\lambda )$ or b. The threshold $c_R (\lambda )$ exists such that the retailer is worse off in the expectation utility with manufacturer encroachment when c satisfy the condition: $c\in \left( {\max \left\{ {\frac{2k\sigma +3\lambda (\mu _H -\mu _L )}{4},\frac{3\sqrt{\lambda }(\mu _H -\mu _L )+2k\sigma }{4}} \right\} ,c_R (\lambda )} \right)$ and the retailer is better off when $c\in \left( {c_R (\lambda ),b} \right)$.

Proof of Remark 1

In Region $R_3$, the manufacturer may be better off or worse off when the retailer distorts the order quantity downward. Because there is not closed-form solution for the manufacturer’s optimal wholesale price in a low-sized market, it is technically challenging to derive the necessary and sufficient condition under which the manufacturer is better off or worse off. Thus, we analyze the simple limiting case with $\lambda \rightarrow 0$, then obtain the following results.

The manufacturer’s profit is $\prod \nolimits _M^N =\frac{\left( {\mu _L -k\sigma } \right) ^{2}}{8}$ without encroachment, and the retailer’s utility $U_R^N$ is

$$\begin{aligned} U_R^N =\frac{(\mu _L -k\sigma )(\mu _L +3k\sigma )}{16}-\frac{1}{2}k\sigma _H \sqrt{5\mu _L ^{2}+2\mu _L k\sigma +k\sigma -4\mu _L }. \end{aligned}$$

The manufacturer’s optimal wholesale price is

$$\begin{aligned} w^{N}=\left\{ {\begin{array}{l} \frac{\mu _H +2\mu _L -c-k\sigma }{6},\quad \hbox {if }\frac{5(\mu _H -\mu _L )+4k\sigma }{8}\le c<\overline{{c}}(\lambda ) \\ \overline{w} ,\quad \hbox {if }\frac{3(\mu _H -\mu _L )+4k\sigma }{8}<c<\frac{5(\mu _H -\mu _L )+4k\sigma }{8} \\ \end{array}} \right. , \end{aligned}$$

(44)

where $\overline{w} =\frac{1}{4}\left( {3\mu _L -\mu _H +2c-2k\sigma } \right) .$ We obtain the following results:

$$\begin{aligned}&E\left[ {\prod \nolimits _M^{SD} (w)} \right] -\prod \nolimits _M^N \nonumber \\&\quad =\frac{1}{2}\lambda w^{AI}\left( {\mu _H -2w^{AI}+c-k\sigma } \right) +\frac{1}{16}\lambda \left( {\mu _H +2w^{AI}-3c+k\sigma } \right) ^{2}-\frac{\left( {\mu _L -k\sigma } \right) ^{2}}{8}\nonumber \\&\quad \quad +\frac{1}{2}(1-\lambda )w^{AI}\left[ 2\mu _H -\mu _L -2w^{AI}+c-k\sigma \right. \nonumber \\&\quad \quad \left. -\,\sqrt{\left( {\mu _H -\mu _L } \right) \left( {3\mu _H -\mu _L -4w^{AI}+2c-2k\sigma } \right) } \right] \nonumber \\&\quad \quad +\,\frac{1}{16}(1-\lambda )\left[ 3\mu _L -3c-2\mu _H +2w^{AI}+k\sigma \right. \nonumber \\&\quad \quad \left. +\,\sqrt{\left( {\mu _H -\mu _L } \right) \left( {3\mu _H -\mu _L -4w^{AI}+2c-2k\sigma } \right) } \right] ^{2} ,\end{aligned}$$

(45)

$$\begin{aligned}&E\left[ {U_R (w)} \right] -U_R^N \nonumber \\&\quad =\frac{1}{8}\lambda (\mu _H -2w^{AI}+c+k\sigma )\left( {\mu _H -2w^{AI}+c-k\sigma } \right) \nonumber \\&\quad \quad +\,\frac{1}{8}(1\!-\!\lambda )\left( {5\mu _L \!-\!4\mu _H \!+\!c\!-\!2w^{AI}\!+\!k\sigma \!+\!MN} \right) \left( {2\mu _H \!-\!\mu _L \!-\!2w^{AI}\!+\!c\!-\!k\sigma \!-\!MN} \right) \nonumber \\&\quad \quad -\,\frac{1}{2}k\sigma \sqrt{\lambda \left( {\mu _H \!-\!2w^{AI}\!+\!c\!-\!k\sigma } \right) ^{2}\!+\!(1\!-\!\lambda )\left( {2\mu _H \!-\!\mu _L \!-\!2w^{AI}\!+\!c\!-\!k\sigma \!-\!MN} \right) ^{2}} \nonumber \\&\quad \quad -\,\frac{(\mu _L -k\sigma )(\mu _L +3k\sigma )}{16}+\frac{1}{2}k\sigma \sqrt{5\mu _L ^{2}+2\mu _L k\sigma +k\sigma -4\mu _L }. \end{aligned}$$

(46)

When k takes different values, the difference between $E[ {\prod \nolimits _M^{SD} (w)} ]$ with $\prod \nolimits _M^N$, i.e., $\prod \nolimits _M^{AI} -\prod \nolimits _M^N =E[ {\prod \nolimits _M^{SD} (w^{AI})} ]-\prod \nolimits _M^N >0$ or $E[ {\prod \nolimits _M^{SD} (w^{AI})} ]-\prod \nolimits _M^N <0$. Given that the two parties’ profit functions are continuous in $\lambda$, because of $\mu _H$, $\mu _L$, $\lambda >0$, in Region $R_3$, we obtain that whether both parties are better off or worse off depends on the range of c and the different values k takes for a manufacturer’s encroachment. $\square$