Abstract
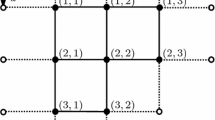
We describe a fast and robust gradient integration method that computes scene depths (or heights) from surface gradient (or surface normal) data such as would be obtained by photometric stereo or interferometry. Our method allows for uncertain or missing samples, which are often present in experimentally measured gradient maps; for sharp discontinuities in the scene’s depth, e.g. along object silhouette edges; and for irregularly spaced sampling points. To accommodate these features of the problem, we use an original and flexible representation of slope data, the weight-delta mesh. Like other state of the art solutions, our algorithm reduces the problem to a system of linear equations that is solved by Gauss-Seidel iteration with multi-scale acceleration. Its novel key step is a mesh decimation procedure that preserves the connectivity of the initial mesh. Tests with various synthetic and measured gradient data show that our algorithm is as accurate and efficient as the best available integrators for uniformly sampled data. Moreover our algorithm remains accurate and efficient even for large sets of weakly-connected instances of the problem, which cannot be efficiently handled by any existing algorithm.
Chapter PDF
Similar content being viewed by others
Keywords
These keywords were added by machine and not by the authors. This process is experimental and the keywords may be updated as the learning algorithm improves.
References
Agrawal, A.: Matlab/Octave code for [3] (2006), http://www.umiacs.umd.edu/~aagrawal/software.html
Agrawal, A., Chellappa, R., Raskar, R.: An algebraic approach to surface reconstruction from gradient fields. In: Proc. 7th ICCV, pp. 174–181 (2005)
Agrawal, A., Raskar, R., Chellappa, R.: What is the Range of Surface Reconstructions From a Gradient Field? In: Leonardis, A., Bischof, H., Pinz, A. (eds.) ECCV 2006. LNCS, vol. 3951, pp. 578–591. Springer, Heidelberg (2006)
Cormen, T.H., Leiserson, C.E., Rivest, R.L.: Introduction to Algorithms. McGraw-Hill (1990)
Fraile, R., Hancock, E.R.: Combinatorial surface integration. In: Proc. 18th ICPR 2006, vol. 1, pp. 59–62 (2006)
Frankot, R.T., Chellappa, R.: A method for enforcing integrability in shape from shading algorithms. IEEE TPAMI 10(4), 439–451 (1988)
Georghiades, Belhumeur, Kriegman: Illumination cone models for face recognition under variable lighting and pose. IEEE TPAMI 23, 643–660 (2001)
Horn, B.K.P.: Height and gradient from shading. IJCV 5(1), 37–75 (1990)
Horn, B.K.P., Brooks, M.J.: Shape from Shading. MIT Press (1989)
Horn, B.K.P., Woodham, R.J., Silver, W.M.: Determining shape and reflectance using multiple images. Technical Report AI Memo 490. MIT (1978)
Kampel, M., Sablatnig, R.: 3D puzzling of archeological fragments. In: Skocaj, D. (ed.) Proc. of 9th Computer Vision Winter Workshop, pp. 31–40 (2004)
Kirkpatrick, D.G.: Optimal search in planar subdivisions. SIAM J. on Computing 12, 28–35 (1983)
Smith, L.N., Hansen, M.F., Atkinson, G.A., Smith, M.L.: 3D face reconstructions from photometric stereo using near infrared and visible light. Computer Vision and Image Understanding 114, 942–951 (2010)
Ng, Wu, Tang: Surface-from-gradients without discrete integrability enforcement: A Gaussian kernel approach. IEEE TPAMI 32 (November 2010)
Robles-Kelly, A., Hancock, E.R.: Surface height recovery from surface normals using manifold embedding. In: Proc. ICIP (October 2004)
Saracchini, Stolfi, Leitao, Atkinson, Smith: Multi-scale depth from slope with weights. In: Proceedings of the BMVC, pp. 40.1–40.12. BMVA Press (2010)
Smith, G.D.J., Bors, A.G.: Height estimation from vector fields of surface normals. In: Proc. IEEE DSP, pp. 1031–1034 (2002)
Smith, M.L., Smith, L.N.: Polished Stone Surface Inspection using Machine Vision, page 33. OSNET (2004)
Terzopoulos, D.: The computation of visible-surface representations. IEEE TPAMI 10(4), 417–438 (1988)
Terzopoulos, D.: Image analysis using multigrid relaxation methods. IEEE TPAMI PAMI 8(2), 129–139 (1986)
Wei, T., Klette, R.: Height from gradient using surface curvature and area constraints. In: Proc. 3rd ICVGIP (2002)
Woodham, R.J.: Photometric method for determining suface orientation from multiple images. Optical Engineering 19(1), 139–144 (1980)
Author information
Authors and Affiliations
Editor information
Editors and Affiliations
Rights and permissions
Copyright information
© 2011 Springer-Verlag Berlin Heidelberg
About this paper
Cite this paper
Saracchini, R.F.V., Stolfi, J., Leitão, H.C.G., Atkinson, G., Smith, M.L. (2011). Multi-scale Integration of Slope Data on an Irregular Mesh. In: Ho, YS. (eds) Advances in Image and Video Technology. PSIVT 2011. Lecture Notes in Computer Science, vol 7087. Springer, Berlin, Heidelberg. https://doi.org/10.1007/978-3-642-25367-6_10
Download citation
DOI: https://doi.org/10.1007/978-3-642-25367-6_10
Publisher Name: Springer, Berlin, Heidelberg
Print ISBN: 978-3-642-25366-9
Online ISBN: 978-3-642-25367-6
eBook Packages: Computer ScienceComputer Science (R0)