Abstract
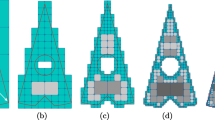
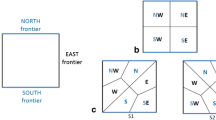
This article presents special quadrilateral quadratic refinement elements, which provide geometry and field continuity across T-junctions where two elements are connected to one side of a larger quadrilateral. The main idea in element refinement is to place one or more nodes outside the element area and to modify element shape functions in order to maintain continuity at refinement edges. Special refinement elements allow one to adaptively refine a mesh in such a way that it fits a quadtree data structure. An algorithm of surface modeling starts with a coarse mesh of quadratic quadrilateral elements. Adaptive mesh refinement is done in an iterative manner. At each iteration, the finite element equation system is solved to provide nodal locations with minimization of global approximation error. Elements with excessive local errors are split into four new elements. The mesh refinement iteration process is terminated when no element splits occur. The created mesh of quadratic quadrilaterals can be used directly in finite element analysis.
Chapter PDF
Similar content being viewed by others
Keywords
These keywords were added by machine and not by the authors. This process is experimental and the keywords may be updated as the learning algorithm improves.
References
Duchineau, M., et al.: ROAMing terrain: real-time optimally adapting meshes. In: Procs of the IEEE Visualization, vol. 97, pp. 81–88 (1997)
Pajarola, R.: Large scale terrain visualization using the restricted quadtree triangulation. In: Procs of the IEEE Visualization, vol. 98, pp. 19–24 (1998)
Grosso, R., Lurig, C., Ertl, T.: The multilevel finite element method for adaptive mesh optimization and vizualization of volume data. In: Procs of the IEEE Visualization, vol. 97, pp. 387–395 (1997)
Bathe, K.-J.: Finite Element Procedures. Prentice-Hall, Englewood Cliffs (1996)
Frey, P.J., George, P.-L.: Mesh Generation. Application to Finite Elements. Hermes (2000)
Zorin, D., Schröder, P.: Subdivision for modeling and animation. In: SIGGRAPH 2000 Course Notes (2000)
Kobbelt, L.P.: A subdivision scheme for smooth interpolation of quad-mesh data. In: Procs of EUROGRAPHICS 1998, Tutorial (1998)
Fortin, M., Tanguy, P.: A non-standard mesh refinement procedure through node labelling. Int. J. Numer. Meth. Eng. 20, 1361–1365 (1984)
Sederberg, T.W., Zheng, J., Bakenov, A., Nasri, A.: T-splines and T-NURCCs. ACM Trans. on Graphics 22, 477–484 (2003)
de Berg, M., van Kreveld, M., Overmars, M., Schwarzkopf, O.: Computational Geometry. Algorithms and Applications. Springer, Heidelberg (2000)
Author information
Authors and Affiliations
Editor information
Editors and Affiliations
Rights and permissions
Copyright information
© 2005 Springer-Verlag Berlin Heidelberg
About this paper
Cite this paper
Nikishkov, G.P. (2005). Adaptive Surface Modeling Using a Quadtree of Quadratic Finite Elements. In: Sunderam, V.S., van Albada, G.D., Sloot, P.M.A., Dongarra, J.J. (eds) Computational Science – ICCS 2005. ICCS 2005. Lecture Notes in Computer Science, vol 3515. Springer, Berlin, Heidelberg. https://doi.org/10.1007/11428848_38
Download citation
DOI: https://doi.org/10.1007/11428848_38
Publisher Name: Springer, Berlin, Heidelberg
Print ISBN: 978-3-540-26043-1
Online ISBN: 978-3-540-32114-9
eBook Packages: Computer ScienceComputer Science (R0)