Dynamical structure factor of magnetic Bloch oscillations at finite temperatures

Abstract

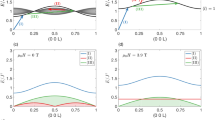
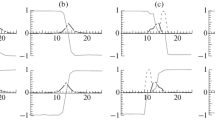
Domain-walls in one-dimensional Ising ferromagnets can undergo Bloch oscillations when subjected to a skew magnetic field. Such oscillations imply finite temperature non-dispersive low-frequency peaks in the dynamical structure factor which can be probed in neutron scattering. We study in detail the spectral weight of these peaks. Using an analytical approach based on an approximate treatment of a gas of spin-cluster excitations we give an explicit expression for the momentum- and temperature-dependence of the spectral weights. Generally the spectral weights increase with temperature T and approaches the same order of magnitude as the spin-wave spectral weights at high temperatures. We compare the analytical expression to numerical exact diagonalizations and find that it can, without any adjustable parameters, account for the T and momentum-transfer dependence of the numerically obtained spectral weights in the parameter regime where the ratio of magnetic fields h ^x/h ^z ≪ 1 and the temperature is h ^x < T < ∼ J ^z/2. We also carry out numerical calculations pertinent to the material CoNb₂O₆, and find qualitatively similar results.