Skip to main content

Advertisement

SpringerLink
Log in

Energy demand elasticities and weather worldwide

  • Published:
Economia Politica Aims and scope Submit manuscript

Abstract

The purpose of this paper is to empirically estimate a model of aggregate residential and commercial energy demand elasticities, taking into account capital stock and climatic effects. We model a theoretically founded non-linear energy demand system, the generalized almost ideal, for the most important 117 countries in the world, which represent around 95% of the world population and 97% of the primary residential energy consumption, for the period 1978–2012. To this end, we assume a multi-stage utility maximization process, which models energy demand within a comprehensive theoretical framework. This paper offers three new contributions to research. First, we model energy aggregate demand response with a flexible and theoretically plausible simultaneous system. Second, we empirically measure the complete structure of price and expenditure elasticities of energy demand worldwide. Third, we explicitly estimate the impact of climate conditions on energy demand, with a newly constructed measure of weather impact based on geo-located heating and cooling degree-days. Econometric estimation reveals quantitative evidence of different income and price elasticities across countries and highlights the weather and capital stock impact on energy demand, inducing energy efficiency. Electricity tends to be a luxury good in advanced economies. Our results have welfare-improving policy implications, because appropriate policy strategies can help public decision-makers promote production efficiency and consumer welfare.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4

Similar content being viewed by others

Notes

  1. Sato and Dechezleprêtre (2015) use exogenous industrial prices to explain international trade patterns for a panel of 42 countries.

  2. This method was justified by Miljkovic et al. (2016), in their estimate of major fossil fuel demand, to method to tackle the problem of cross-country correlation among quantities and prices.

  3. In particular, Kaufmann et al. (2013) estimate the energy-weather relationship, arguing in favor of using hourly weather measures and computing heating degree-days using hourly values, without fully considering prices and capital stock effects.

  4. The time series relating to the CDD and HDD degree-days were extracted from the CMCC-KAPSARC database (Atalla et al. 2015), which provides annual population weighted degree days values for 147 countries covering from 1948 to 2013. The dataset was developed by actual values and reanalysis of several location appropriate climate parameters—air temperature, relative humidity, solar radiation—on a daily, 6-h frequency for the whole period. The weather data was later population-weighted using extrapolations from both the UNEP/GRID-Sioux Falls and the Columbia University’s Gridded Population of the World datasets for the period considered. Gridded local values were later summed up within national boundaries to obtain country values. Atalla et al. (2015) calculated various thermal comfort indices at varying reference temperatures. A degree-day is defined as the variation between a reference temperature (T ref ) and the average of the period’s temperature (T mean ). If T ref is higher than T mean the absolute value of the difference is counted as HDD while the opposite is accounted for as CDD.

  5. Multi-stage cost functions allow parsimonious parameterization without sacrificing empirical flexibility. Note that we are interested in only one sub-group at the second level: the energy group. Thus, we are not interested in the issue of price dependence and preference homotheticity (Blundell 1988; Edgerton 1997).

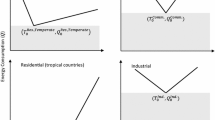
  6. Further algebraic development of Eqs. (7)–(12) deriving Allen elasticity of substitution shows that elementary goods could be complements within the same group but substitutes in the overall allocation (Caves and Christensen 1980).

  7. Our choice of aggregating residential and commercial energy demand depends on the price regime and the economic conditions. Commercial demand tends to react in parallel with residential (with minimal lag) as most of the demand for energy is used for heating and cooling or appliances. This is in contrast with the manufacturing sector where energy demand is used for machinery work that is seldom affected by temperature variation.

  8. In this way, the capital stock variable represents a proxy for the relative endowment of energy consuming capital goods of the households worldwide.

  9. Moreover, Chang et al. (2001) report that using their non-linear estimator does not gain efficiency of the coefficients of the stationary variables in the regression; it is not affected by the inclusion of trend regressors; allows for Chi square tests to be performed. This is so because their non-linear estimator is more for non-linear regressions arising from solutions of rational expectations and dynamic stochastic general equilibrium model.

  10. In the test we specified the null hypothesis always with and without a constant, but without a trend. This is in line with the literature which analyses energy quantities of consumption, because the inclusion of a trend leads to mixed results, based on arbitrary choice of broken trends, as highlighted by Smyth (2013). The results with and without a constant are similar, so we report only the test without a constant. In summary, in Table 2, the null for ADF in unit root; the null for EG is no cointegration, the null for KAP and KAPS is unit root.

  11. This is a well-known result due to the integrability conditions, which have to be respected to recover the underlying utility function from the demand functions, demonstrated by Houthakker (1960) and Gorman (1981).

  12. There are only few examples of electricity demand estimation explicitly based on multi-stage utility or separability theory, e.g. Hausman et al. (1979), Dean and Lawson (1992), Petersen (2002). Multistage demand estimation is more popular in agricultural economics, e.g. Hassan (2011).

  13. Precisely, in each stage, excluding the country dummies, there are 16 structural parameters and 12 free parameters, taking into account the theoretical restrictions. There are six committed quantity parameters: f 1o f 1h f 1c f 2o f 2h f 2c ; one a i and one γ i and one ζ i , given adding up ∑h α h  = 1 and ∑h γ h  = ∑h ζ k  = 0; three β ij given symmetry β ij  = β ji , for ∀ j.

  14. As already explained above, detailed data to breakdown energy demand into its main components is available only for a subset of countries. In the following, we have also estimated all the different model specifications at the first stage, only for the subset of countries. We find that all the restrictions remain valid and the model estimation of the preferred model GAI is robust. Detailed results are available upon request.

  15. We estimated the demand systems with both specifications of the P* functions, namely Eq. (13b) at the first stage and (16b) at the second stage, obtaining very similar results. We report estimation values based on the Stone index specifications.

  16. Parameters are almost all significant at 1% and are available upon request. The standard errors are derived from the estimated covariance matrix computed in the non-linear SUR estimation by summing the outer product of the gradient vector for all structural parameters and error covariance parameters over all observations, inverting this matrix and taking the submatrix corresponding to the structural parameters.

    This is a consistent estimate of the information matrix, with good small sample properties.

  17. In other words, we reject the possibility of estimating electricity demand with a single equation approach, because this test implies that electricity is not separable from other energy sources (contrary to Petersen 2002).

  18. We have computed the elasticities, estimating both the exact formulas and the Stone approximations (see Alston et al. 1994) for Eqs. (13b) and (16b), without finding appreciable differences. We report the empirical results for (uncompensated) elasticities using the Stone approximations. We report unconditional elasticities at the second stage. Compensated elasticities are available upon request.

  19. For the impact of capital formation on energy demand, see also Steinbuks and Neuhoff (2014 ).

  20. We are grateful to a referee for this suggestion.

References

  • Adeyemi, O. I., & Hunt, L. C. (2007). Modelling OECD industrial energy demand: Asymmetric price responses and energy-saving technical change. Energy Economics, 29(2007), 693–709.

    Article  Google Scholar 

  • Alston, J. M., Foster, K. A., & Green, R. D. (1994). Estimating elasticities with the linear approximate almost ideal demand system: Some Monte Carlo results. The Review of Economics and Statistics, 76(2), 351–356.

    Article  Google Scholar 

  • Atalla, T. N., Gualdi, S., & Lanza, A. (2015). A global degree days database for energy-related applications. KAPSARC working paper KS-1514-DP08A. Available at SSRN 2586960.

  • Auffhammer, M., & Mansur, E. T. (2014). Measuring climatic impacts on energy consumption: A review of the empirical literature. Energy Economics, 46, 522–530.

    Article  Google Scholar 

  • Barten, A. P. (1969). Maximum Likelihood estimation of a complete system of demand equation. In R. Bewley & T. Van Hoa (Eds.), Contribution to consumer demand and econometrics. London: Macmillian.

    Google Scholar 

  • Bašta, M., & Helman, K. (2013). Scale-specific importance of weather variables for explanation of variations of electricity consumption: The case of Prague, Czech Republic. Energy Economics, 4, 503–514. doi:10.1016/j.eneco.2013.07.023. (ISSN:0140-9883).

    Google Scholar 

  • Beaver, R. D., & Huntington, H. G. (1992). A comparison of aggregate energy demand models for global warming policy analyses. Energy Policy, 20(6), 568–574.

    Article  Google Scholar 

  • Bernstein, M. A., & Griffin, J. (2006). Regional differences in the price-elasticity of demand for energy. Subcontract report, NREL/SR-620-39512, RAND Corporation Santa Monica, California, http://www.nrel.gov/docs/fy06osti/39512.pdf. Accessed 15 Oct 2011.

  • Bigerna, S., & Bollino, C. A. (2014). Electricity demand in wholesale italian market. The Energy Journal, 35(3), 25–46.

    Article  Google Scholar 

  • Blundell, R. (1988). Consumer behaviour: Theory and empirical evidence. Economic Journal, 98(389), 16–65.

    Article  Google Scholar 

  • Blundell, R., & Walker, I. (1982). Modelling the joint determination of household labour supplies and commodity demands. Economic Journal, 92(366), 351–364.

    Article  Google Scholar 

  • Bollino, C. A. (1987). Gaids: a generalized version of the almost ideal demand system. Economic Letters, 23(2), 199–202.

    Article  Google Scholar 

  • Bollino, C. A., Perali, F., & Rossi, N. (2000). Linear household technologies. Journal of Applied Econometrics, 15(3), 257–288.

    Article  Google Scholar 

  • Breuer, J. B., McNown, R., & Wallace, M. (2002). Series-specific unit root tests with panel data. Oxford Bulletin of Economics and Statistics, 64(5), 527–546.

    Article  Google Scholar 

  • Browning, M., & Meghir, C. (1991). The effects of male and female labor supply on commodity demands. Econometrica, 59, 925–951.

    Article  Google Scholar 

  • Cao J., Ho, M. S., & Liang, H. (2014). Household energy demand in Urban China: Accounting for regional prices and rapid income change, working paper, Tsinghua University.

  • Caves, D. W., & Christensen, L. R. (1980). Residential electricity pricing experiments. Journal of Econometrics, 14, 287–306.

    Article  Google Scholar 

  • Chang, Y., Park, J. Y., & Phillips, P. C. (2001). Nonlinear econometric models with cointegrated and deterministically trending regressors. The Econometrics Journal, 4(1), 1–36.

    Article  Google Scholar 

  • Coers, R., & Sanders, M. (2013). The energy–GDP nexus; addressing an old question with new methods. Energy Economics, 36, 708–715.

    Article  Google Scholar 

  • Curtis, J., & Pentecost, A. (2015). Household fuel expenditure and residential building energy efficiency ratings in Ireland. Energy Policy, 76(January), 57–65.

    Article  Google Scholar 

  • Dean, C., & Lawson, M. E. (1992). A disaggregated non-homothetic modeling of responsiveness to residential time-of-use electricity rates. International Economic Review, 33, 181–207.

    Article  Google Scholar 

  • Deaton, A. S., & Muellbauer, J. (1980). An almost ideal demand system. American Economic Review, 70, 312–326.

    Google Scholar 

  • Deaton, A. S., & Muellbauer, J. (1981). Functional forms for labour supply and commodity demands with and without quantity restrictions. Econometrica, 49, 1521–1533.

    Article  Google Scholar 

  • Duarte, R., Maina, A., & Sánchez-Chóliza, J. (2012). Social groups and CO2 emissions in Spanish households. Energy Policy, 44, 441–450.

    Article  Google Scholar 

  • Edgerton, D. L. (1997). Weak separability and the estimation of elasticities in multistage demand systems. American Journal of Agricultural Economics, 79, 62–79.

    Article  Google Scholar 

  • Enerdata. (2015). Enerdata’s global energy and CO2 database, retrieved on February 12th, 2015.

  • Fallahi, F., Karimib, M., & Voia, M. C. (2016). Persistence in world energy consumption: Evidence from subsampling confidence intervals. Energy Economics, 57(2016), 175–183.

    Article  Google Scholar 

  • Faruqui, A., & Sergici, S. (2010). Household response to dynamic pricing of electricity—A survey of 15 experiments. Journal of Regulatory Economics, 38(2), 82–109.

    Article  Google Scholar 

  • Fezzi, C., & Bunn, D. (2010). Structural analysis of electricity demand and supply interactions. Oxford Bulletin of Economics and Statistics, 72(6), 827–856.

    Article  Google Scholar 

  • Filippini, M., & Hunt, L. C. (2011). Energy demand and energy efficiency in the OECD countries: A stochastic demand frontier approach. Energy Journal, 32(2), 59–80.

    Article  Google Scholar 

  • Filippini, M., & Pachauri, S. (2004). Elasticities of electricity demand in urban Indian households. Energy Policy, 32(3), 429–436.

    Article  Google Scholar 

  • Flaig, G. (1990). Household production and the short- and long-run demand for electricity. Energy Economics, 12(2), 116–121.

    Article  Google Scholar 

  • Gately, D., & Huntington, H. G. (2002). The asymmetric effects of changes in price and income on energy and oil demand. The Energy Journal, 23(1), 19–55.

    Article  Google Scholar 

  • Gorman, W. (1981). Some engel curves. In A. Deaton (Ed.), Essays in the theory and measurement of consumer behaviour. Cambridge: Cambridge Univ Press.

    Google Scholar 

  • Hasanov, M., & Telatar, E. (2011). A re-examination of stationarity of energy consumption: Evidence from new unit root tests. Energy Policy, 39, 7726–7738.

    Article  Google Scholar 

  • Hassan, A. R. (2011). A multi-stage almost ideal demand system: The case of beef demand in Colombia. A technical report from Department of Economics, EAFIT University. Medellín (Colombia).

  • Hausman, J. A., Kinnucan, M., & McFadden, D. (1979). A two-level electricity demand model: Evaluation of the Connecticut time-of-day pricing test. Journal of Econometrics, 10(3), 263–289.

    Article  Google Scholar 

  • Hodge, T., & Dahl, C. A. (2012). Power marketer pricing behavior in the California Power Exchange. Energy Economics, 34(2), 568–575.

    Article  Google Scholar 

  • Horowitz, M. J. (2007). Changes in electricity demand in the United States from the 1970s to 2003. The Energy Journal, 28(3), 93–119.

    Article  Google Scholar 

  • Houthakker, H. S. (1960). Additive preferences. Econometrica, 28, 244–257.

    Article  Google Scholar 

  • Hsu, Y. C., Lee, C. C., & Lee, C. C. (2008). Revisited: Are shocks to energy consumption permanent or temporary? New evidence from a panel SURADF approach. Energy Economics, 30, 2314–2330.

    Article  Google Scholar 

  • Huang, K. S., & Huang, S. W. (2012). Consumer welfare effects of increased food and energy prices. Applied Economics, 44(19), 2527–2536.

    Article  Google Scholar 

  • Huang, B. N., Hwang, M. J., & Yang, C. W. (2008). Causal relationship between energy consumption and GDP growth revisited: A dynamic panel data approach. Ecological Economics, 67, 41–54.

    Article  Google Scholar 

  • Hunt, L. C., & Ryan, D. L. (2015). Economic modelling of energy services: Rectifying misspecified energy demand functions. Energy Economics, 50, 273–285.

    Article  Google Scholar 

  • International Energy Agency (IEA). (2014). World energy balances, IEA World Energy Statistics and Balances database). doi:10.1787/data-00512-en.

  • Kapetanios, G., Shin, Y., & Snell, A. (2003). Testing for a unit root in the nonlinear STAR framework. Journal of Econometrics, 112, 359–379.

    Article  Google Scholar 

  • Karimu, A., & Brännlund, R. (2013). Functional form and aggregate energy demand elasticities: A nonparametric panel approach for 17 OECD countries. Energy Economics, 36, 19–27.

    Article  Google Scholar 

  • Kaufmann, R. K., Gopal, S., Tang, X., Raciti, S. M., Lyons, P. E., Geron, N., et al. (2013). Revisiting the weather effect on energy consumption: Implications for the impact of climate change. Energy Policy, 62, 1377–1384.

    Article  Google Scholar 

  • Krishnamurthy, C. K. B., & Kriström, B. (2015). A cross-country analysis of residential electricity demand in 11 OECD-countries. Resource and Energy Economics, 39, 68–88.

    Article  Google Scholar 

  • Labandeira, X., Labeaga, J. M., & López-Otero, X. (2012). Estimation of elasticity price of electricity with incomplete information. Energy Economics, 34(3), 627–633.

    Article  Google Scholar 

  • Lee, C. C., & Chien, M. S. (2010). Dynamic modelling of energy consumption, capital stock, and real income in G-7 countries. Energy Economics, 32, 564–581.

    Article  Google Scholar 

  • Lee, C. C., & Chiu, Y. B. (2011). Electricity demand elasticities and temperature: Evidence from panel smooth transition regression with instrumental variable approach. Energy Economics, 33(5), 896–902.

    Article  Google Scholar 

  • Mark, N. C., Ogaki, M., & Sul, D. (2005). Dynamic seemingly unrelated cointegrating regressions. The Review of Economic Studies, 72(3), 797–820.

    Article  Google Scholar 

  • Miljkovic, D., Dalbec, N., & Zhang, Lei. (2016). Estimating dynamics of US demand for major fossil fuels. Energy Economics, 55, 284–291.

    Article  Google Scholar 

  • Neary, J. P., & Roberts, K. W. S. (1980). The theory of household behaviour under rationing. American Economic Review, 13, 25–42.

    Google Scholar 

  • Petersen, S. L. (2002). Micro econometric modeling of household energy use: testing for dependence between demand for electricity and natural gas. The Energy Journal, 23(4), 57–84.

    Google Scholar 

  • Pollak, R. A. (1971). Conditional demand functions and the implication of separable utility. Southern Economic Journal, 4(78), 60–78.

    Google Scholar 

  • Pollak, R. A. (1991). Welfare comparisons and situation comparisons. Journal of Econometrics, 50(1–2), 31–48.

    Article  Google Scholar 

  • Rowland, C. S., Mjelde, J. W., & Dharmasena, S. (2017). Policy implications of considering pre-commitments in US aggregate energy demand system. Energy Policy, 102, 406–413.

    Article  Google Scholar 

  • Salamaliki, P. K., & Venetis, I. A. (2013). Energy consumption and real GDP in G-7: Multi-horizon causality testing in the presence of capital stock. Energy Economics, 39(2013), 108–121.

    Article  Google Scholar 

  • Sato, M., & Dechezleprêtre, A. (2015). Asymmetric industrial energy prices and international trade. Energy Economics, 52, S130–S141.

    Article  Google Scholar 

  • Schaffrin, A., & Reiblin, N. (2015). Household energy and climate mitigation policies: Investigating energy practices in the housing sector. Energy Policy, 77, 1–10.

    Article  Google Scholar 

  • Smyth, R. (2013). Are fluctuations in energy variables permanent or transitory? A survey of the literature on the integration properties of energy consumption and production. Applied Energy, 104, 371–378.

    Article  Google Scholar 

  • Smyth, R., & Narayan, P. K. (2015). Applied econometrics and implications for energy economics research. Energy Economics, 50, 351–358.

    Article  Google Scholar 

  • Steinbuks, J., & Neuhoff, K. (2014). Assessing energy price induced improvements in efficiency of capital in OECD manufacturing industries. Journal of Environmental Economics and Management, 68, 340–356.

    Article  Google Scholar 

  • Willett, K., & Naghshpour, S. (1987). Residential demand for energy commodities: A household production function approach. Energy Economics, 9(4), 251–256.

    Article  Google Scholar 

  • Wolak, F. A. (2011). Do residential customers respond to hourly prices? Evidence from a dynamic pricing experiment. American Economic Review: Papers & Proceedings, 101(3), 83–87.

    Article  Google Scholar 

  • Wu, J. L., & Lee, H. Y. (2009). A revisit to the non-linear mean reversion of real exchange rates: Evidence from a series-specific non-linear panel unit-root test. Journal of Macroeconomics, 31, 591–601.

    Article  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. KAPSARC, Riyadh, Saudi Arabia

    Tarek Atalla & Carlo Andrea Bollino

  2. University of Perugia, Perugia, Italy

    Simona Bigerna & Carlo Andrea Bollino

Authors
  1. Tarek Atalla
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Simona Bigerna
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Carlo Andrea Bollino
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Tarek Atalla.

Appendix

Appendix

1.1 Detailed estimated elasticities

See Tables 6 and 7.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Atalla, T., Bigerna, S. & Bollino, C.A. Energy demand elasticities and weather worldwide. Econ Polit 35, 207–237 (2018). https://doi.org/10.1007/s40888-017-0074-2

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s40888-017-0074-2

Keywords

JEL classification

Search

Navigation