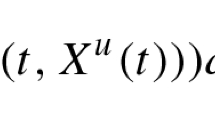Scaling and Saturation in Infinite-Dimensional Control Problems with Applications to Stochastic Partial Differential Equations

Abstract

We establish the dual notions of scaling and saturation from geometric control theory in an infinite-dimensional setting. This generalization is applied to the low-mode control problem in a number of concrete nonlinear partial differential equations. We also develop applications concerning associated classes of stochastic partial differential equations (SPDEs). In particular, we study the support properties of probability laws corresponding to these SPDEs as well as provide applications concerning the ergodic and mixing properties of invariant measures for these stochastic systems.

Appendices

Appendix A: Supplemental PDE Bounds

A.1. A Priori Estimates

Here we present a collection of a priori estimates which assure that the solution maps in each equation have the necessary cocycle and semigroup structures. We begin with the a priori estimates for the reaction–diffusion equations (5.1).

A.1.1. Reaction–Diffusion

Recall that for $V\in \Omega$, we define solutions $u=u(t, u_0, V)$ with $u(0)=u_0$ of (5.1) by $u(t, u_0, V) = v(t, u_0, \sigma \cdot V) + \sigma \cdot V$ where v satisfies the shifted equation (5.6). In order to make the estimates more legible, for $k\ge 0, T>0$ we introduce the sup norms

$$\begin{aligned} |V|_{k,T}= \sup _{t \in [0, T]}\Vert V(\,\cdot \,,t)\Vert _{W^{k, \infty }([0, 2\pi ])}. \end{aligned}$$

Proposition 5.1 follows immediately once we establish:

Proposition A.1

We have the following estimates.

• (a) Let $u_0 \in L^2$, $T>0$, $V\in \Omega$ and $v(\,\cdot \,)=v(\,\cdot \,, u_0, \sigma \cdot V)$. Then there exists a constant $C_1>0$ depending only on $T, \Vert u_0\Vert , | \sigma \cdot V|_{2, T}$ such that for any $t\in [0, T]$

  $$\begin{aligned} \Vert v(t)\Vert ^2 + 2\kappa \int _0^t \Vert \partial _x v(s)\Vert ^2 ds + \nu \int _0^t \Vert v(s)\Vert ^{2n} \, ds \le C_1 . \end{aligned}$$

  (A.1)

• (b) Let $u_1, u_2\in L^2$, $T>0$, $V_1, V_2 \in \Omega$ and set $w(t)= v(t, u_1,\sigma \cdot V_1) - v(t, u_2, \sigma \cdot V_2)$, $w_0=u_1-v_1$ and ${\bar{V}}= V_1-V_2$. Then there exists a constant $C_2>0$ depending only on $T, \Vert u_i\Vert , | \sigma \cdot V_i|_{2, T}$ such that for any $t\in [0, T]$

  $$\begin{aligned}&\Vert w(t) \Vert ^2 + 2 \kappa \int _0^t \Vert \partial _x w (s)\Vert ^2 ds \le C_2( \Vert w_0 \Vert ^2 + |\partial _{xx} (\sigma \cdot {\bar{V}})|_{T}^2 + |\sigma \cdot {\bar{V}}|_{T} ). \end{aligned}$$

  (A.2)

Proof of Proposition A.1

To obtain the first estimate (A.1), observe that there exists a constant $K=K(|\sigma \cdot V |_{T})>0$ such that

$$\begin{aligned} \frac{1}{2}\frac{d}{dt} \Vert v(t) \Vert ^2&\le - \kappa \Vert \partial _x v(t)\Vert ^2 + \kappa \Vert v(t) \Vert \Vert \partial _{xx} (\sigma \cdot V)\Vert + \langle v, f(v+\sigma \cdot V)\rangle \nonumber \\&\le - \kappa \Vert \partial _x v(t)\Vert ^2 + 2\pi \kappa \Vert v(t)\Vert | \partial _{xx} (\sigma \cdot V)|_{T} + K - \frac{\nu }{2} \Vert v(t) \Vert ^{2n}. \end{aligned}$$

(A.3)

Hence we have that

$$\begin{aligned} \frac{1}{2}\frac{d}{dt} \Vert v(t) \Vert ^2&\le K_1 \Vert v(t)\Vert ^2 + K_2 \end{aligned}$$

for some constants $K_1>0$ and $K_2= K_2(|\sigma \cdot V|_{2,T} )>0.$ Gronwall’s inequality then implies the existence of a constant $K_3$ depending only on T, $\Vert u_0\Vert$, $| \sigma \cdot V|_{2,T}$ such that for all $t\in [0, T]$

$$\begin{aligned} \Vert v(t) \Vert ^2 \le K_3. \end{aligned}$$

(A.4)

Integrating (A.3) with respect to time and using the estimate (A.4) we arrive at the bound (A.1).

Turning our attention to the second estimate (A.2), note that for $t\in [0, T]$

$$\begin{aligned} \frac{1}{2}\frac{d}{dt} \Vert w(t) \Vert ^2&\le - \kappa \Vert \partial _x w(t) \Vert ^2 + 2\pi \kappa \Vert w(t)\Vert | \partial _{xx}( \sigma \cdot {\bar{V}}) |_{T} \nonumber \\&\qquad + \kappa \langle w, f(v_1 + \sigma \cdot V_1) - f(v_2 + \sigma \cdot V_2) \rangle . \end{aligned}$$

(A.5)

To estimate the last term above, recall the explicit form form of the Mean Value Theorem applied to the polynomial f: For $a, b \in {\mathbf {R}}$ we have

$$\begin{aligned} f(b)-f(a)=(b-a) f'(\xi )= (b-a) \int _0^1 f'(a + \beta (b-a) ) \, d\beta \end{aligned}$$

(A.6)

for some $\xi =\xi (a,b)$ lying between a, b. Hence since $f' \le K$ for some constant $K>0$ only depending on f we find that

$$\begin{aligned} \langle w , f(v_1 + V_1) - f(v_2+V_2) \rangle&= \langle w, f'(\xi ) w \rangle + \langle w, f'(\xi ) (\sigma \cdot {\bar{V}}) \rangle \nonumber \\&\le K \Vert w\Vert ^2 + | \sigma \cdot {\bar{V}}|_{0,T} \int _0^{2\pi } |w| |f'(\xi )| \, dx. \end{aligned}$$

(A.7)

Integrating (A.5) with respect to time using the bound (A.7) and applying Young’s inequality we obtain the estimate

$$\begin{aligned}&\frac{1}{2}\Vert w(t)\Vert ^2 + \kappa \int _0^t \Vert \partial _x w(s)\Vert ^2 \, ds \\&\le \Vert w_0 \Vert ^2 + K_1 \int _0^t \Vert w(s)\Vert ^2 \, ds + K_2 | \sigma \cdot {\bar{V}}|_{2,T}^2 \\&\quad + |\sigma \cdot {\bar{V}}|_{0,T} \Vert w\Vert _{L^n([0, 2\pi ] \times [0, t])} \Vert f'(\xi ) \Vert _{L^{p}([0, 2\pi ]\times [0, t])} \end{aligned}$$

where $p=2n/(2n-2)$, for some constants $K_1, K_2>0$. Applying the estimate (A.1) to the last term above using the explicit form for $f'(\xi )=f'(\xi (v_1 + \sigma \cdot V_1, v_2 + \sigma \cdot V_2))$, we determine the existence of a constant $K_3$ depending only on $T, \Vert u_i\Vert , | \sigma \cdot V_i|_{2,T}$ such that

$$\begin{aligned}&\frac{1}{2}\Vert w(t)\Vert ^2 + \kappa \int _0^t \Vert \partial _x w(s)\Vert ^2 \, ds \le \Vert w_0 \Vert ^2 \nonumber \\&\quad + K_1 \int _0^t \Vert w(s)\Vert ^2 \, ds + K_2 | \sigma \cdot {\bar{V}}|_{2,T}^2 + K_3 |\sigma \cdot {\bar{V}}|_{0,T}. \end{aligned}$$

(A.8)

From this, using Gronwall’s inquality we arrive at the claimed estimate (A.2) when combined with (A.8). $\square$

A.2. Boussinesq Equations

We now provide the needed a priori estimates for the Boussinesq equations (5.32)–(5.33). We begin by establishing the $L^2$ estimates below in Proposition A.2 for the shifted equation (5.35)–(5.36) so that the $\phi$ defined in the statement of Proposition 5.17 is a continuous adapted cocycle. For $k\ge 0$ and $T>0$, we again use compact notation for sup norms, which in this context will read

$$\begin{aligned} |V|_{k, T} = \sup _{t\in [0,T]} \{ \Vert V(\cdot , t) \Vert _{W^{k, \infty }({\mathbf {T}}^2)}\}. \end{aligned}$$

Proposition A.2

We have the following:

• (1) Fix $T>0$, ${\tilde{\theta }}(0), {\tilde{\xi }}(0) \in L^2$ and $V\in \Omega$. Then for all $t\in [0, T]$ we have that

  $$\begin{aligned} \Vert {\tilde{\theta }}(t)\Vert ^2 + \Vert {\tilde{\xi }}(t) \Vert ^2+ \int _0^t \nu \Vert \nabla {\tilde{\xi }}(s) \Vert ^2 + \kappa \Vert \nabla {\tilde{\theta }}(s) \Vert \, ds \le C \end{aligned}$$

  (A.9)

  where $C>0$ is a constant depending only on $\Vert {\tilde{\xi }}(0)\Vert , \Vert {\tilde{\theta }}(0) \Vert , T, \kappa , g, | \sigma \cdot V|_{2, T}, \Vert h^0 \Vert$.

• (2) Let $({\tilde{\xi }}_1, {\tilde{\theta }}_1, V_1)$ and $({\tilde{\xi }}_2, {\tilde{\theta }}_2, V_2)$ solve (5.35)–(5.36) with ${\tilde{\xi }}_i(0), {\tilde{\theta }}_i(0)\in L^2$ and $V_i \in \Omega$. Then if ${\bar{\xi }}= {\tilde{\xi }}_1-{\tilde{\xi }}_2$, ${\bar{\theta }}= {\tilde{\theta }}_1-{\tilde{\theta }}_2$, $T>0$, ${\bar{V}}=V_1-V_2$, we have for $t\in [0, T]$

  $$\begin{aligned} \Vert {\bar{\xi }}(t)\Vert ^2 + \Vert {\bar{\theta }}(t) \Vert ^2 \le C \big ( \Vert {\bar{\xi }}(0)\Vert ^2 + \Vert {\bar{\theta }}(0) \Vert ^2 + |\sigma \cdot {\bar{V}}|_{1,T}\big ) \end{aligned}$$

  (A.10)

  for some constant $C>0$ where C depends only on $\Vert {\tilde{\xi }}_i(0)\Vert , \Vert {\tilde{\theta }}_i(0) \Vert , T, \kappa , g, |\sigma \cdot V_i|_{2,T}, \Vert h^0 \Vert$.

Proof

We begin by establishing the bound (A.9). Let $T>0$ and $t\in [0, T]$. First observe that

$$\begin{aligned}&\frac{1}{2}\frac{d}{dt} \Vert {\tilde{\xi }}\Vert ^2 + \nu \Vert \nabla {\tilde{\xi }}\Vert ^2 \le g \Vert {\tilde{\xi }}\Vert \Vert \partial _x \theta \Vert + 4\pi ^2 g \Vert {\tilde{\xi }}\Vert | \sigma \cdot V|_{1,T}\\&\quad \le C_1 \Vert {\tilde{\xi }}\Vert ^2 + \frac{\kappa }{2}\Vert \partial _x {\tilde{\theta }}\Vert ^2 + \frac{\kappa }{2}| \sigma \cdot V |^2_{1,T} \end{aligned}$$

for some constant $C_1>0$ depending only on $g, \kappa$. Also note that

$$\begin{aligned} \frac{1}{2}\frac{d}{dt}\Vert {\tilde{\theta }}\Vert ^2 + \kappa \Vert \nabla {\tilde{\theta }}\Vert ^2&\le C_2\Vert {\tilde{\theta }}\Vert \Vert {\tilde{u}} \Vert |\sigma \cdot V|_{1,T} + \kappa \Vert {\tilde{\theta }}\Vert \Vert \sigma \cdot V\Vert _{H^2} + \Vert {\tilde{\theta }}\Vert \Vert h^0\Vert \\&\le C_3( \Vert {\tilde{\theta }}\Vert ^2 + \Vert {\tilde{\xi }}\Vert ^2 | \sigma \cdot V|^2_{1,T} + \Vert h^0 \Vert ^2 + | \sigma \cdot V|_{2, T}) \end{aligned}$$

for some constant $C_3=C_3(\kappa )>0$. Summing the previous two inequalities we obtain

$$\begin{aligned} \frac{1}{2} \frac{d}{dt}( \Vert {\tilde{\xi }}\Vert ^2 + \Vert {\tilde{\theta }}\Vert ^2) + \frac{\nu }{2} \Vert \nabla {\tilde{\xi }}\Vert ^2 + \frac{\kappa }{2} \Vert \nabla {\tilde{\theta }}\Vert ^2&\le C( \Vert {\tilde{\theta }}\Vert ^2 + \Vert {\tilde{\xi }}\Vert ^2) + D \end{aligned}$$

(A.11)

for some constants $C=C(\kappa , g, |\sigma \cdot V|_{1,T})>0$ and $D=D(\kappa , \Vert h^0 \Vert , |\sigma \cdot V|_{2,T})>0$. Applying Gronwall’s inequality we obtain

$$\begin{aligned} \Vert {\tilde{\xi }}(t) \Vert ^2 + \Vert {\tilde{\theta }}(t) \Vert ^2 \le C \end{aligned}$$

for all $t\in [0, T]$ where $C>0$ is a constant depending only on $\Vert {\tilde{\xi }}(0)\Vert , \Vert {\tilde{\theta }}(0) \Vert , T, \kappa , g, \Vert h^0 \Vert , | \sigma \cdot V|_{2,T}$. Plugging this back into the righthand side of (A.11) gives the desired bound (A.9).

Moving onto the second estimate (A.10), first note that since $\langle {\bar{\xi }}, {\tilde{u}}_1 \cdot \nabla {\bar{\xi }} \rangle =0$

$$\begin{aligned}&\frac{1}{2}\frac{d}{dt} \Vert {\bar{\xi }}\Vert ^2 + \nu \Vert \nabla {\bar{\xi }}\Vert ^2 \le g \Vert {\bar{\xi }}\Vert \Vert \partial _x {\bar{\theta }}\Vert + g \Vert {\bar{\xi }}\Vert \Vert \partial _x (\sigma \cdot {\bar{V}}) \Vert - \langle {\bar{\xi }}, ({\tilde{u}}_1 - {\tilde{u}}_2) \cdot \nabla {\tilde{\xi }}_2 \rangle ,\\&\frac{1}{2}\frac{d}{dt} \Vert {\bar{\theta }}\Vert ^2 + \kappa \Vert \nabla {\bar{\theta }}\Vert ^2 \le \langle {\bar{\theta }}, {\tilde{u}}_2 \cdot \nabla ({\tilde{\theta }}_2+ \sigma \cdot V_2) - {\tilde{u}}_1 \cdot \nabla ({\tilde{\theta }}_1 + \sigma \cdot V_1)\rangle \\&\quad + \kappa \Vert {\bar{\theta }}\Vert \Vert \sigma \cdot {\bar{V}}\Vert _{H^2} \end{aligned}$$

To combine the estimates as we did above, we now bound the terms remaining in inner product form as follows:

$$\begin{aligned}&- \langle {\bar{\xi }}, ({\tilde{u}}_1 - {\tilde{u}}_2) \cdot \nabla {\tilde{\xi }}_2 \rangle = \langle ({\tilde{u}}_1 - {\tilde{u}}_2) \cdot \nabla {\bar{\xi }}, {\tilde{\xi }}_2 \rangle \\&\le C_1\Vert \nabla {\bar{\xi }}\Vert \Vert {\tilde{u}}_1 - {\tilde{u}}_2\Vert _{L^4} \Vert {\tilde{\xi }}_2 \Vert _{L^4}\\&\le C_2 \Vert \nabla {\bar{\xi }} \Vert \Vert {\bar{\xi }} \Vert \Vert \nabla {\tilde{\xi }}_2 \Vert ^{1/2} \Vert {\tilde{\xi }}_2 \Vert ^{1/2}\\&\le \frac{\nu }{2} \Vert \nabla {\bar{\xi }}\Vert ^2 + C'\Vert {\bar{\xi }}\Vert ^2 \Vert \nabla {\tilde{\xi }}_2\Vert \Vert {\tilde{\xi }}_2 \Vert , \end{aligned}$$

for some constant $C'>0$ depending on $\nu$, and in a similar fashion

$$\begin{aligned}&\langle {\bar{\theta }}, {\tilde{u}}_2 \cdot \nabla ({\tilde{\theta }}_2+ \sigma \cdot V_2) - {\tilde{u}}_1 \cdot \nabla ({\tilde{\theta }}_1 + \sigma \cdot V_1)\rangle \\&=- \langle ({\tilde{u}}_2- {\tilde{u}}_1) \cdot \nabla {\bar{\theta }}, {\tilde{\theta }}_2 + \sigma \cdot V_2 \rangle + \langle {\bar{\theta }}, {\tilde{u}}_1 \cdot \nabla (\sigma \cdot {\bar{V}})\rangle \\&\le \frac{\kappa }{4}\Vert \nabla {\bar{\theta }}\Vert ^2 + C'' \Vert {\bar{\xi }}\Vert ^2 \Vert \nabla ({\tilde{\theta }}_2 + \sigma \cdot V_2) \Vert \Vert {\tilde{\theta }}_2 + \sigma \cdot V_2 \Vert \\&\qquad + C''' \Vert {\bar{\theta }}\Vert \Vert {\tilde{\xi }}_1 \Vert |\nabla (\sigma \cdot {\bar{V}}) |_T \end{aligned}$$

for some constant $C''>0$ depending only on $\kappa$ and some constant $C'''>0$. Thus by summing the first two inequalities, applying the inequalities above and weighting appropriately using Young’s inequality we obtain

$$\begin{aligned}&\frac{1}{2} \frac{d}{dt}( \Vert {\bar{\xi }}\Vert ^2 + \Vert {\bar{\theta }}\Vert ^2) + \frac{\nu }{2}\Vert \nabla {\bar{\xi }}\Vert ^2 + \frac{\kappa }{2}\Vert \nabla {\bar{\theta }}\Vert ^2 \le C f [\Vert {\bar{\xi }}\Vert ^2 + \Vert {\bar{\theta }}\Vert ^2 ]+ D \Vert {\tilde{\xi }}_1 \Vert ^2 |\sigma \cdot {\bar{V}} |_{1,T}^2 \end{aligned}$$

for some constants $C, D>0$ depending only on $\kappa , \nu , g$ and

$$\begin{aligned} f = 1+ \Vert \nabla {\tilde{\xi }}_2 \Vert ^2 + \Vert {\tilde{\xi }}_2 \Vert ^2 + \Vert \nabla ({\tilde{\theta }}_2+ \sigma \cdot V_2)\Vert ^2+ \Vert {\tilde{\theta }}_2 + \sigma \cdot V_2 \Vert ^2. \end{aligned}$$

Gronwall’s inequality then implies that for $t\in [0, T]$

$$\begin{aligned}&\Vert {\bar{\xi }}(t)\Vert ^2 + \Vert {\bar{\theta }}(t) \Vert ^2 \le \bigg ( \Vert {\bar{\xi }}(0)\Vert ^2 + \Vert {\bar{\theta }}(0) \Vert ^2 \\&\quad + D |\sigma \cdot {\bar{V}}|_{1,T} \int _0^t \Vert {\tilde{\xi }}_1(s)\Vert ^2 \,ds\bigg )\exp \bigg (\int _0^t C f(s) \, ds \bigg ). \end{aligned}$$

Applying the first inequality (A.9) to estimate $\int _0^t \Vert {\tilde{\xi }}_1(s)\Vert ^2 \, ds$ and $\int _0^t f(s) \, ds$, we obtain the desired inequality. $\square$

We next turn our attention to the a priori estimates needed to validate Assumption 4.13. Here it will be convenient to express the system (5.32)–(5.33) using the abstract evolution equation notation for the solution $U=(\xi (t), \theta (t))$:

$$\begin{aligned} \frac{dU}{dt}+ AU + GU+ B(U,U) = \iota _\theta h^0 + \iota _\theta (\sigma \cdot \partial _t V), \end{aligned}$$

(A.12)

which we recall was introduced above equation (5.44). Following Remark 4.16, our principal interest will be in establishing estimates for the linear equation

$$\begin{aligned} \partial _t \rho + A\rho + G \rho + B(U, \rho ) + B(\rho , U) = 0, \,\, \rho (s)=\rho _0 \end{aligned}$$

(A.13)

where $\rho _0 \in H$ and U solves (A.12). To do so, we will make use of the following inequalities for $u,v, w\in H$

$$\begin{aligned} |\langle u, B(v, w)\rangle |&\le C \Vert \nabla u \Vert ^{1/2} \Vert u\Vert ^{1/2} \Vert \nabla (K * v) \Vert ^{1/2} \Vert K*v \Vert ^{1/2} \Vert \nabla w\Vert \end{aligned}$$

(A.14)

$$\begin{aligned}&\le C_1 \Vert \nabla u \Vert \Vert u\Vert + C_2 \Vert v\Vert ^2 \Vert \nabla w\Vert ^2 \end{aligned}$$

(A.15)

where $C, C_1, C_2>0$ are constants. The first inequality (A.14) is $L^4$-$L^4$-$L^2$ bound followed by an application of the Gagliardo-Nirenberg interpolation inequality. The second (A.15) is simply Young’s inequality applied to the righthand side of  (A.14).

Proposition A.3

We have the following:

• (1) Fix $T>0$, $\rho _0 \in H$ and let $\rho =(\rho _1, \rho _2)$ solve (A.13) with $\rho (0)=\rho _0$ and corresponding U with $U(0)=U_0\in H$. Then there exists a constant $C>0$ depending only on $\Vert U_0 \Vert , T, \kappa , \nu , g, |\sigma \cdot V|_{2,T}, \Vert h^0\Vert$ such that for all $0\le s\le t \le T$

  $$\begin{aligned} \Vert \rho (t) \Vert ^2 + \int _s^t \nu \Vert \nabla \rho _1 (v) \Vert ^2 + \kappa \Vert \nabla \rho _2(v) \Vert ^2 \, dv \le C. \end{aligned}$$

  (A.16)

• (2) Fix $T>0$ and let $U_1, U_2$ solve (A.12) with corresponding initial data $U_1(0), U_2(0) \in H$ and corresponding $V_1, V_2 \in \Omega$. Here we assume that $U_1$ and $U_2$ solve (A.12) with the same $h^0\in L^2$. Let $\rho _1, \rho _2$ solve (A.13) with corresponding data $\rho _1(s), \rho _2(s) \in H$ and corresponding $U_1, U_2$. Set ${\bar{\rho }}_0= \rho _1(s) - \rho _2(s)$, and ${\bar{U}}=U_1 - U_2$. Then there exists a constant $C>0$ depending only on $T, \Vert U_i(0)\Vert , \kappa , \nu , g, |\sigma \cdot V_i|_{C^2, T}, \Vert h^0\Vert$ such that for all $0\le s\le t\le T$

  $$\begin{aligned} \Vert {\bar{\rho }}(t)\Vert ^2 \le C( \Vert {\bar{\rho }}_0\Vert ^2 + \Vert {\bar{U}}(0)\Vert ^2 + | \sigma \cdot {\bar{V}}|_{C^1, T}). \end{aligned}$$

  (A.17)

Proof

We begin by establishing (1). Observe that

$$\begin{aligned} \frac{1}{2}\frac{d}{dt}\Vert \rho (t) \Vert ^2 + \nu \Vert \nabla \rho _1(t) \Vert ^2 + \kappa \Vert \nabla \rho _2(t) \Vert ^2 + \langle \rho , G \rho \rangle + \langle \rho , B(\rho , U)\rangle =0 \end{aligned}$$

and

$$\begin{aligned} |\langle \rho , G \rho \rangle | = g |\langle \rho _1, \partial _x \rho _2\rangle | \le \frac{\kappa }{2}\Vert \nabla \rho _2 \Vert ^2 + C\Vert \rho \Vert ^2 \end{aligned}$$

for some constant $C>0$ depending only on $g, \kappa$. Applying the inequality (A.15), we also find that

$$\begin{aligned} | \langle \rho , B(\rho , U)\rangle |&\le C_1 \Vert \nabla \rho \Vert \Vert \rho \Vert + C_2 \Vert \rho \Vert ^2 \Vert \nabla U \Vert ^2 \\&\quad \le \frac{\nu \wedge \kappa }{4} \Vert \nabla \rho \Vert ^2 + C (1+ \Vert \nabla U \Vert ^2) \Vert \rho \Vert ^2 \end{aligned}$$

for some constant $C>0$. Putting these estimates together produces the bound

$$\begin{aligned} \frac{1}{2}\frac{d}{dt}\Vert \rho (t) \Vert ^2 + \frac{\nu }{4} \Vert \nabla \rho _1(t) \Vert ^2 +\frac{\kappa }{4} \Vert \nabla \rho _2(t) \Vert ^2 \le C( 1+ \Vert \nabla U\Vert ^2) \Vert \rho \Vert ^2. \end{aligned}$$

(A.18)

Since we also have that

$$\begin{aligned} \frac{1}{2}\frac{d}{dt}\Vert \rho (t) \Vert ^2 \le C( 1+ \Vert \nabla U\Vert ^2) \Vert \rho \Vert ^2, \end{aligned}$$

applying Gronwall’s inequality and then Proposition A.2 implies

$$\begin{aligned} \Vert \rho (t)\Vert ^2 \le \Vert \rho _0\Vert ^2 \exp \bigg ( \int _s^t 2 C ( 1+ \Vert \nabla U(v)\Vert ^2 \, dv\bigg )\le C \end{aligned}$$

for all $0\le s\le t \le T$ where $C>0$ is a constant depending only on $\Vert U_0 \Vert , T, \kappa , \nu , g, |\sigma \cdot V|_{2,T}, \Vert h^0\Vert .$ Using the information on the righthand side of equation (A.18), integrating with respect to time, and then applying Proposition A.2 again we arrive at the estimate in (1).

To see (2), note that

$$\begin{aligned} 0=&\frac{1}{2}\frac{d}{dt}\Vert {\bar{\rho }}(t) \Vert ^2 + \nu \Vert \nabla {\bar{\rho }}_1 \Vert ^2 + \kappa \Vert \nabla {\bar{\rho }}_2\Vert ^2 + \langle {\bar{\rho }}, G {\bar{\rho }}\rangle \\&\qquad + \langle {\bar{\rho }}, B(\rho _1, U_1)- B(\rho _2, U_2)\rangle + \langle {\bar{\rho }}, B(U_1, \rho _1)- B(U_2, \rho _2)\rangle . \end{aligned}$$

We can again bound $\langle {\bar{\rho }}, G {\bar{\rho }}\rangle$ as follows:

$$\begin{aligned} |\langle {\bar{\rho }}, G {\bar{\rho }}\rangle | \le \frac{\kappa }{2}\Vert \nabla {\bar{\rho }}_2 ||^2 + C \Vert {\bar{\rho }}\Vert ^2 \end{aligned}$$

for some constant $C>0$ depending only on $g, \kappa$. Using bilinearity and (A.15), also observe that

$$\begin{aligned} |\langle {\bar{\rho }}, B(\rho _1, U_1)- B(\rho _2, U_2)\rangle |&\le | \langle {\bar{\rho }}, B({\bar{\rho }}, U_1)\rangle | + |\langle {\bar{\rho }}, B(\rho _2, {\bar{U}})\rangle |\\&\le C_1 \Vert \nabla {\bar{\rho }} \Vert \Vert {\bar{\rho }} \Vert + C_2 \Vert {\bar{\rho }}\Vert ^2 \Vert \nabla U_1 \Vert ^2 + C_3 \Vert \rho _2 \Vert ^2 \Vert \nabla {\bar{U}}\Vert ^2 \end{aligned}$$

and

$$\begin{aligned} | \langle {\bar{\rho }}, B(U_1, \rho _1)- B(U_2, \rho _2)\rangle |&= | \langle {\bar{\rho }}, B({\bar{U}}, \rho _2)\rangle |\\&\le C_4 \Vert \nabla {\bar{\rho }} \Vert \Vert {\bar{\rho }} \Vert + C_5 \Vert {\bar{U}} \Vert ^2 \Vert \nabla \rho _2\Vert ^2 \end{aligned}$$

for some constants $C_i>0$. Combining these estimates and applying Young’s inequality to the terms $C_1 \Vert \nabla {\bar{\rho }} \Vert \Vert {\bar{\rho }}\Vert$ and $C_4 \Vert \nabla {\bar{\rho }} \Vert \Vert {\bar{\rho }}\Vert$ we find that

$$\begin{aligned}&\frac{1}{2}\frac{d}{dt}\Vert {\bar{\rho }}(t) \Vert ^2 + \frac{\nu }{4}\Vert \nabla {\bar{\rho }}_1 \Vert ^2 + \frac{\kappa }{4}\Vert \nabla {\bar{\rho }}_2 \Vert ^2 \nonumber \\&\le C(1+ \Vert \nabla U_1\Vert ^2) \Vert {\bar{\rho }}\Vert ^2 + C_3 \Vert \rho _2\Vert ^2 \Vert \nabla {\bar{U}}\Vert ^2 + C_5 \Vert {\bar{U}}\Vert ^2 \Vert \nabla \rho _2 \Vert ^2. \end{aligned}$$

(A.19)

By Proposition A.2, we note that

$$\begin{aligned} \Vert {\bar{U}}\Vert ^2 \le C( \Vert {\bar{U}}(0)\Vert ^2 + | \sigma \cdot {\bar{V}}|^2_{1,T}) \end{aligned}$$

(A.20)

where $C>0$ is a constant depending only on $T, \Vert U_i(0) \Vert , \kappa , g, | \sigma \cdot V_i |_{C^2, T}, \Vert h^0\Vert$. By the first part of this proposition, we also have that

$$\begin{aligned} \Vert \rho _2 \Vert ^2 \le C' \end{aligned}$$

(A.21)

where $C'>0$ is a constant depending only on $\Vert U_2(0)\Vert , T, \kappa , \nu , \kappa , g, |\sigma \cdot V_2|_{C^2, T}, \Vert h^0\Vert$. Applying the inequalities (A.20)–(A.21) to the righthand side of (A.19) and then applying Gronwall’s inequality produces the estimate

$$\begin{aligned} \Vert {\bar{\rho }}(t)\Vert ^2&\le \bigg ( \Vert {\bar{\rho }}_0 \Vert ^2 + C \int _s^t \Vert \nabla {\bar{U}}(v)\Vert ^2 \bigg )\exp \bigg (C\int _s^t 1+ \Vert \nabla U_1(v)\Vert ^2 \, dv \bigg )\nonumber \\&\quad + C(\Vert {\bar{U}}(0)\Vert ^2 + |\sigma \cdot {\bar{V}}|_{1, T}) \int _s^t \Vert U_1(v)\Vert ^2 \, dv \bigg ) \exp \bigg (C\int _s^t 1+ \Vert \nabla U_1(v)\Vert ^2 \, dv \bigg ) \end{aligned}$$

(A.22)

where $C>0$ is a constant depending only on $T, \Vert U_i(0)\Vert , \kappa , \nu , g, |\sigma \cdot V_i|_{C^2, T}, \Vert h^0\Vert$. Applying Proposition A.2 again, we arrive at the claimed bound in (2). $\square$

All parts of Assumption 4.13 follow from the above proposition except (v) which concerns the non-degeneracy of the $L^2$-adjoint of the Jacobi flow. This, however, can be established by following a nearly identical process to the one used in the case of the two-dimensional Navier–Stokes equations as in Proposition 2.2 of [34]. There, non-degeneracy follows by uniqueness of the associated backwards PDE satisfied by the adjoint.

Finally, we establish the higher-order Sobolev a priori estimates for the Boussinesq equations (5.32)–(5.33) when forced by a smoother V; that is, we now consider the equations

$$\begin{aligned} \partial _t \xi + u \cdot \nabla \xi - \nu \Delta \xi&= g \partial _x \theta , \,\, \, \xi (0)= \xi _0 \end{aligned}$$

(A.23)

$$\begin{aligned} \partial _t \theta + u \cdot \nabla \theta - \kappa \Delta \theta&= f, \,\,\, \theta (0)=\theta _0 \end{aligned}$$

(A.24)

where f is a generic constant element in the relevant Sobolev space. Note that the only difference between equations (5.32)–(5.33) and the equations above is that the forcing term $h^0+ \sigma \cdot \partial _t V$ has replaced by f.

Proposition A.4

We have the following:

• (i) Suppose that $\xi _0, \theta _0, f \in L^2$ and let $(\xi , \theta )$ be the corresponding solution of (A.23)–(A.24). Then

  $$\begin{aligned} \sup _{r \in [0,t]} (\Vert \xi (r) \Vert + \Vert \theta (r) \Vert ) \le C (\Vert \xi _0\Vert + \Vert \theta _0 \Vert + t\Vert f\Vert ) \end{aligned}$$

  (A.25)

  and

  $$\begin{aligned} \int _0^t (\Vert \nabla \xi \Vert ^2 + \Vert \nabla \theta \Vert ^2) dr \le C (\Vert \xi _0\Vert ^2 + \Vert \theta _0 \Vert ^2 + t^2\Vert f\Vert ^2) \end{aligned}$$

  (A.26)

  where the constant C depends only $\kappa , \nu , g$ and universal quantities.

• (ii) Suppose that $\xi _0, \theta _0, f \in H^m$ for any $m \ge 1$. Then

  $$\begin{aligned}&\sup _{r \in [0,t]} (\Vert \xi (r) \Vert _{H^m} + \Vert \theta (r) \Vert _{H^m}) + \int _0^t (\Vert \xi \Vert _{H^{m+1}} + \Vert \theta \Vert _{H^{m+1}}) dr \nonumber \\&\le C\exp \left( C (\Vert \xi _0\Vert ^2 + \Vert \theta _0 \Vert ^2 + t^2\Vert h\Vert ^2 + t)\right) (1+ \Vert \xi _0\Vert _{H^m} + \Vert \theta _0 \Vert _{H^m} + t\Vert f\Vert _{H^m}). \end{aligned}$$

  (A.27)

• (iii) Fix any $m \ge 0$ and suppose $U_0=(\xi _0, \theta _0), {\tilde{U}}_0=({\tilde{\xi }}_0, {\tilde{\theta }}_0)\in H^m({\mathbf {T}}^2)^2$ and $f, {\tilde{f}} \in H^m({\mathbf {T}}^2)$. Let $(\xi , \theta )$, $({\tilde{\xi }}, {\tilde{\theta }})$ be the solutions of (A.23)–(A.24) the corresponding to this data. Then

  $$\begin{aligned}&\sup _{r \in [0,t]} (\Vert \xi (r) - {\tilde{\xi }}(r) \Vert _{H^m} + \Vert \theta (r) - {\tilde{\theta }}(r) \Vert _{H^m}) \nonumber \\&\qquad \le C \left( \Vert \xi _0 - {\tilde{\xi }}_0 \Vert _{H^m} + \Vert \theta _0 - {\tilde{\theta }}_0 \Vert _{H^m} + t \Vert f - {\tilde{f}} \Vert _{H^m}\right) . \end{aligned}$$

  (A.28)

  where C is a constant depending only on $\kappa , \nu , g, \Vert U_0\Vert _{H^m} , \Vert {\tilde{U}}_0\Vert _{H^m}, \Vert f\Vert _{H^m}, \Vert {\tilde{f}}\Vert _{H^m}, t$ and universal quantities.

Proof

We begin with the basic $L^2$ estimates for (A.23)–(A.24). Multiplying the first equation by $\xi$, the second equation by $\theta$ and integrating over the domain yields

$$\begin{aligned}&\frac{1}{2} \frac{d}{dt} \Vert \xi \Vert ^2 + \nu \Vert \nabla \xi \Vert ^2 = \langle g \partial _x \theta , \xi \rangle \le \frac{g^2}{2\nu } \Vert \theta \Vert ^2 + \frac{\nu }{2} \Vert \nabla \xi \Vert ^2, \end{aligned}$$

and

$$\begin{aligned}&\frac{1}{2} \frac{d}{dt} \Vert \theta \Vert ^2 + \kappa \Vert \nabla \theta \Vert ^2 = \langle f, \theta \rangle \le \Vert f\Vert \Vert \theta \Vert . \end{aligned}$$

The fact that the velocity u is divergence free justifies dropping the non-linear contributions in the above. Suitably weighting and then adding these two inequalities we find

$$\begin{aligned} \frac{d}{dt} \left( \Vert \xi \Vert ^2 + \frac{g^2}{2\nu \kappa } \Vert \theta \Vert ^2 \right) + \nu \Vert \nabla \xi \Vert ^2 + \frac{g^2}{\nu } \Vert \nabla \theta \Vert ^2 \le \frac{g^2}{\nu \kappa }\Vert f\Vert \Vert \theta \Vert . \end{aligned}$$

(A.29)

The first item, (A.25), follows immediately. Moreover

$$\begin{aligned} \int _0^t (\Vert \nabla \xi \Vert ^2 + \Vert \nabla \theta \Vert ^2) dr \le&C\left( \Vert \xi _0\Vert ^2 + \Vert \theta _0\Vert ^2 + \sup _{r \in [0,t]} \Vert \theta (r)\Vert \cdot t \Vert f \Vert \right) \\ \le&C \left( \Vert \xi _0\Vert ^2 + \Vert \theta _0\Vert ^2 + (\Vert \xi _0\Vert + \Vert \theta _0 \Vert + t\Vert f\Vert ) t\Vert f\Vert \right) , \end{aligned}$$

implying (A.26).

Given any multi-index $\alpha$ and taking the associated spatial derivatives of (5.32)–(5.33) we obtain

$$\begin{aligned} \partial _t \partial ^\alpha \xi + \partial ^\alpha (u \cdot \nabla \xi ) - \nu \Delta \partial ^\alpha \xi = g \partial _x \partial ^\alpha \theta , \quad \partial _t \partial ^\alpha \theta + \partial ^\alpha (u \cdot \nabla \theta ) - \kappa \Delta \partial ^\alpha \theta = \partial ^\alpha f. \end{aligned}$$

Multiplying, integrating and summing over $|\alpha | \le m$ yields

$$\begin{aligned}&\frac{1}{2} \frac{d}{dt}\Vert \xi \Vert ^2_{H^m} + \nu \Vert \xi \Vert ^2_{H^{m+1}} =\sum _{|\alpha | \le m} \langle g \partial _x \partial ^\alpha \theta -\partial ^\alpha (u \cdot \nabla \xi ), \partial ^\alpha \xi \rangle \end{aligned}$$

(A.30)

$$\begin{aligned}&\frac{1}{2} \frac{d}{dt} \Vert \theta \Vert ^2_{H^m} + \kappa \Vert \theta \Vert ^2_{H^{m+1}} = \sum _{|\alpha | \le m} \langle \partial ^\alpha f -\partial ^\alpha (u \cdot \nabla \theta ), \partial ^\alpha \theta \rangle \end{aligned}$$

(A.31)

Taking advantage of the fact that u is divergence free and applying standard interpolation/commutator estimates produces for any $m \ge 1$

$$\begin{aligned}&\sum _{|\alpha | \le m} |\langle \partial ^\alpha (u \cdot \nabla \xi ) , \partial ^\alpha \xi \rangle | \nonumber \\&\quad = \sum _{|\alpha | \le m} |\langle \partial ^\alpha (u \cdot \nabla \xi ) - u \cdot \nabla \partial ^\alpha \xi , \partial ^\alpha \xi \rangle | \nonumber \\&\quad \le C \sum _{|\alpha | \le m} (\Vert \partial ^\alpha u \Vert _{L^\infty } \Vert \nabla \xi \Vert + \Vert \nabla {\mathbf {u}}\Vert _{L^4} \Vert \partial ^\alpha \xi \Vert _{L^4} )\Vert \xi \Vert _{H^m} \nonumber \\&\quad \le C \Vert \nabla \xi \Vert \Vert \xi \Vert _{H^m}^{3/2} \Vert \xi \Vert _{H^{m+1}}^{1/2} \le \frac{\nu }{6} \Vert \xi \Vert _{H^{m+1}}^{2} + C\Vert \nabla \xi \Vert ^{4/3} \Vert \xi \Vert _{H^m}^{2}. \end{aligned}$$

(A.32)

Similarly

$$\begin{aligned}&\sum _{|\alpha | \le m} | \langle \partial ^\alpha (u \cdot \nabla \theta ) , \partial ^\alpha \theta \rangle |\nonumber \\&\quad = \sum _{|\alpha | \le m} |\langle \partial ^\alpha (u \cdot \nabla \theta ) - u \cdot \nabla \partial ^\alpha \theta , \partial ^\alpha \theta \rangle | \nonumber \\&\quad \le C \sum _{|\alpha | \le m} (\Vert \partial ^\alpha u \Vert _{L^\infty } \Vert \nabla \theta \Vert + \Vert \nabla {\mathbf {u}}\Vert _{L^4} \Vert \partial ^\alpha \theta \Vert _{L^4} )\Vert \theta \Vert _{H^m} \nonumber \\&\quad \le C( \Vert \xi \Vert _{H^m}^{1/2} \Vert \xi \Vert _{H^{m+1}}^{1/2}\Vert \nabla \theta \Vert \Vert \theta \Vert _{H^m} + \Vert \xi \Vert ^{1/2} \Vert \nabla \xi \Vert ^{1/2} \Vert \theta \Vert ^{3/2}_{H^m} \Vert \theta \Vert ^{1/2}_{H^{m+1}}) \nonumber \\&\quad \le \frac{\nu }{6} \Vert \xi \Vert _{H^{m+1}}^2 + \frac{\kappa }{4} \Vert \theta \Vert _{H^{m+1}}^2 + C(\Vert \xi \Vert _{H^m}^2 + (\Vert \nabla \theta \Vert ^2 + \Vert \nabla \xi \Vert ^{4/3}) \Vert \theta \Vert _{H^m}^2). \end{aligned}$$

(A.33)

Finally

$$\begin{aligned} \sum _{|\alpha | \le m} |\langle g \partial _x \partial ^\alpha \theta , \partial ^\alpha \xi \rangle | \le \frac{\sqrt{3} g^2}{2\nu } \Vert \theta \Vert ^2_{H^m} + \frac{\nu }{6} \Vert \xi \Vert ^2_{H^{m+1}}. \end{aligned}$$

(A.34)

Combining (A.30), (A.31) with the estimates (A.32)–(A.34) we now obtain

$$\begin{aligned}&\frac{d}{dt} \left( 1+ \Vert \xi \Vert ^2_{H^m} + \frac{\sqrt{3}g^2}{2\nu \kappa } \Vert \theta \Vert ^2_{H^m} \right) + \nu \Vert \xi \Vert ^2_{H^{m+1}} + \frac{\sqrt{3}g^2}{\nu } \Vert \theta \Vert ^2_{H^{m+1}} \nonumber \\&\quad \le C \Vert h\Vert _{H^m}\Vert \theta \Vert _{H^m} + C(1+ \Vert \nabla \theta \Vert ^2 + \Vert \nabla \xi \Vert ^{4/3})( \Vert \theta \Vert _{H^m}^2 + \Vert \xi \Vert _{H^m}^2 ). \end{aligned}$$

(A.35)

Thus, taking $X := (1+ \Vert \xi \Vert ^2_{H^m} + \frac{\sqrt{3}g^2}{2\nu \kappa } \Vert \theta \Vert ^2_{H^m})^{1/2}$, $Y := (\nu \Vert \xi \Vert ^2_{H^{m+1}} + \frac{\sqrt{3}g^2}{\nu } \Vert \theta \Vert ^2_{H^{m+1}})^{1/2}$ we have,

$$\begin{aligned} \frac{d}{dt} X + C Y \le C \Vert h\Vert _{H^m} + C(1+ \Vert \nabla \theta \Vert ^2 + \Vert \nabla \xi \Vert ^{2}) X. \end{aligned}$$

With this bound and (A.26) we now infer infer (A.27).

We turn next to establish the continuous dependence estimates Let $\xi = \xi - {\tilde{\xi }}$, $\zeta = \theta - {\tilde{\theta }}$, $\phi = f - {\tilde{f}}$. Then $(\xi , \zeta )$ satisfy

$$\begin{aligned}&\partial _t \xi + {\tilde{u}} \cdot \nabla \xi + (K *\xi ) \cdot \nabla \xi - \nu \Delta \xi = g \partial _x \zeta , \nonumber \\&\partial _t \zeta + {\tilde{u}} \cdot \nabla \zeta + (K *\xi ) \cdot \nabla \theta - \kappa \Delta \zeta = \phi . \end{aligned}$$

(A.36)

Start with the $L^2$ based estimates

$$\begin{aligned} \frac{d}{dt} \Vert \xi \Vert ^2 + \nu \Vert \nabla \xi \Vert ^2 =&\langle g \partial _x \zeta - (K *\xi ) \cdot \nabla \xi , \xi \rangle \nonumber \\ \le&\frac{g^2}{\nu } \Vert \zeta \Vert ^2 + \frac{\nu }{4} \Vert \nabla \xi \Vert ^2 + \Vert \nabla \xi \Vert \Vert K *\xi \Vert _{L^\infty } \Vert \xi \Vert \nonumber \\ \le&\frac{g^2}{\nu } \Vert \zeta \Vert ^2 + \frac{\nu }{4} \Vert \nabla \xi \Vert ^2 + C \Vert \nabla \xi \Vert \Vert \nabla \xi \Vert ^{1/2} \Vert \xi \Vert ^{3/2} \nonumber \\ \le&\frac{g^2}{\nu } \Vert \zeta \Vert ^2 + \frac{\nu }{2} \Vert \nabla \xi \Vert ^2 + C \Vert \nabla \xi \Vert ^{4/3} \Vert \xi \Vert ^{2} \end{aligned}$$

(A.37)

where we used Agmond’s inequality for the penultimate estimate. Similarly

$$\begin{aligned} \frac{d}{dt} \Vert \zeta \Vert ^2 + \kappa \Vert \nabla \zeta \Vert ^2&= \langle \phi - (K *\xi ) \cdot \nabla \theta , \zeta \rangle \nonumber \\&\le C \Vert \phi \Vert \Vert \zeta \Vert + \Vert \nabla \theta \Vert \Vert \xi \Vert ^{1/2} \Vert \nabla \xi \Vert ^{1/2} \Vert \zeta \Vert \nonumber \\&\le C \Vert \phi \Vert \Vert \zeta \Vert + \frac{\nu }{2}\Vert \nabla \xi \Vert ^2 + C\Vert \nabla \theta \Vert ^2 \Vert \zeta \Vert ^2. \end{aligned}$$

(A.38)

Combining the estimates (A.37), (A.38) we obtain the bound

$$\begin{aligned} \frac{d}{dt} (\Vert \xi \Vert ^2 + \Vert \zeta \Vert ^2) \le C \Vert \phi \Vert ^2 + C(1 +\Vert \nabla \theta \Vert ^2 + \Vert \nabla \xi \Vert ^2 )\Vert \zeta \Vert ^2. \end{aligned}$$

(A.39)

We turn to make the continuous dependence estimates in higher Sobolev norms. Applying $\partial ^\alpha$ for any multi-index $\alpha$ and summing over all $|\alpha | \le m$ for any $m \ge 1$, we find that

$$\begin{aligned}&\frac{d}{dt} \Vert \xi \Vert ^2_{H^m} + \nu \Vert \xi \Vert ^2_{H^{m+1}} = \sum _{|\alpha | \le m} \langle \partial ^\alpha ( g \partial _x \zeta - {\tilde{u}} \cdot \nabla \xi - (K *\xi ) \cdot \nabla \xi ), \partial ^\alpha \xi \rangle := I_1, \end{aligned}$$

(A.40)

$$\begin{aligned}&\frac{d}{dt} \Vert \zeta \Vert ^2_{H^m} + \kappa \Vert \zeta \Vert ^2_{H^{m+1}} = \sum _{|\alpha | \le m} \langle \partial ^\alpha (\phi - {\tilde{u}} \cdot \nabla \zeta - (K *\xi ) \cdot \nabla \theta ) , \partial ^\alpha \zeta \rangle := I_2. \end{aligned}$$

(A.41)

Regarding $I_1$ we have

$$\begin{aligned} |I_1|&\le C \Vert \zeta \Vert _{H^m}^2 + \frac{\nu }{2} \Vert \xi \Vert ^2_{H^{m+1}} + C \Vert \xi \Vert ^2_{H^m} \! \sum _{|\alpha |\le m} \Vert \partial ^\alpha {\tilde{u}} \Vert _{L^\infty } \nonumber \\&\quad + C \Vert \xi \Vert _{H^m} ( \Vert \nabla \xi \Vert _{L^4}\! \sum _{|\alpha |\le m} \Vert \partial ^\alpha (K *\xi ) \Vert _{L^4} +\Vert \xi \Vert _{H^{m+1}} \Vert K *\xi \Vert _{L^\infty } ) \nonumber \\&\le C \Vert \zeta \Vert _{H^m}^2 + \frac{\nu }{2} \Vert \xi \Vert ^2_{H^{m+1}} + C(\Vert {\tilde{\xi }} \Vert _{H^{m+1}} +\Vert \xi \Vert _{H^{m+1}}) \Vert \xi \Vert ^2_{H^m} \end{aligned}$$

(A.42)

For $I_2$

$$\begin{aligned} |I_2|&\le \Vert \phi \Vert _{H^m} \Vert \zeta \Vert _{H^m} + C \Vert \zeta \Vert ^2_{H^m} \! \sum _{|\alpha |\le m} \Vert \partial ^\alpha {\tilde{u}} \Vert _{L^\infty } \nonumber \\&\quad + C \Vert \zeta \Vert _{H^m} ( \Vert \nabla \theta \Vert _{L^4}\! \sum _{|\alpha |\le m} \Vert \partial ^\alpha (K *\xi ) \Vert _{L^4} +\Vert \theta \Vert _{H^{m+1}} \Vert K *\xi \Vert _{L^\infty } ) \nonumber \\&\le \Vert \phi \Vert _{H^m} \Vert \zeta \Vert _{H^m} + C\Vert {\tilde{\xi }}\Vert _{H^{m+1}} \Vert \zeta \Vert ^2_{H^m} + C \Vert \theta \Vert _{H^{m+1}} \Vert \zeta \Vert _{H^m} \Vert \xi \Vert _{H^m}. \end{aligned}$$

(A.43)

Combining these estimates we conclude that

$$\begin{aligned}&\frac{d}{dt} (\Vert \xi \Vert ^2_{H^m} + \Vert \zeta \Vert ^2_{H^m})\\&\le \Vert \phi \Vert _{H^m} \Vert \zeta \Vert _{H^m}+ C(1 + \Vert {\tilde{\xi }} \Vert _{H^{m+1}} +\Vert \xi \Vert _{H^{m+1}} + \Vert \theta \Vert _{H^{m+1}})(\Vert \xi \Vert ^2_{H^m} + \Vert \zeta \Vert ^2_{H^m}). \end{aligned}$$

$\square$

A.3. Euler Equations

Proposition 5.28 follows immediately once we establish the following result.

Proposition A.5

Fix any ${\mathbf {g}}\in {\mathcal {X}}$ and any finite-dimensional subspace $X_0 \subset {\mathcal {X}}$.

• (i) For any ${\mathbf {u}}_0 \in {\mathcal {X}}$ and any ${\mathbf {h}}\in X_0$, there exists a unique $0<T_{{\mathbf {u}}_0, {\mathbf {h}}} \le \infty$ and ${\mathbf {u}}(\cdot )={\mathbf {u}}(\cdot , {\mathbf {u}}_0, {\mathbf {h}}) \in C([0, T_{{\mathbf {u}}_0, {\mathbf {h}}}); {\mathcal {X}})$ solving (5.70) such that if $T_{{\mathbf {u}}_0, {\mathbf {h}}}< \infty$ then

  $$\begin{aligned} \limsup _{t\uparrow T_{{\mathbf {u}}_0, {\mathbf {h}}}} \Vert \nabla {\mathbf {u}}(t) \Vert _{L^\infty } = \infty . \end{aligned}$$

• (ii) For any ${\mathbf {u}}_0 \in {\mathcal {X}}$ and any ${\mathbf {h}}\in X_0$, let

  $$\begin{aligned} \tau _{{\mathbf {u}}_0,{\mathbf {h}}}^n= \inf \{ t>0 \, : \, \Vert {\mathbf {u}}(t)\Vert _{H^3} \ge n\} \,\,\, \text { and } \,\,\, \tau _{{\mathbf {u}}_0, {\mathbf {h}}}= \sup _{n\in {\mathbf {N}}} \tau _{{\mathbf {u}}_0, {\mathbf {h}}}^n. \end{aligned}$$

  Then $\tau _{{\mathbf {u}}_0, {\mathbf {h}}}>0$ and $\tau _{{\mathbf {u}}_0, {\mathbf {h}}}\le T_{{\mathbf {u}}_0, {\mathbf {h}}}$. Moreover for all $m\ge 3$, $t < \tau _{{\mathbf {u}}_0, {\mathbf {h}}}^n$ and $n\in {\mathbf {N}}$ we have the estimate

  $$\begin{aligned} \Vert {\mathbf {u}}(t) \Vert _{H^m}^2 \le \Vert {\mathbf {u}}_0 \Vert _{H^m} e^{C (n+1) t} + \int _0^t C e^{C (n+1)(t-s) } \Vert {\mathbf {g}}+ {\mathbf {h}}\Vert _{H^m}\, ds \end{aligned}$$

  for some constant C depending only on m.

• (iii) Let ${\mathbf {u}}_1(0), {\mathbf {u}}_2(0)\in {\mathcal {X}}$, ${\mathbf {h}}_1, {\mathbf {h}}_2 \in X_0$ and ${\mathbf {u}}_1(t, {\mathbf {u}}_1(0), {\mathbf {h}}_1)$ and ${\mathbf {u}}_2(t)= {\mathbf {u}}(t, {\mathbf {u}}_2(0), {\mathbf {h}}_2)$. Let $n, T>0$. Then for all $t<\tau _{{\mathbf {u}}_1(0), {\mathbf {h}}_1}^n \wedge \tau ^n_{{\mathbf {u}}_2(0), {\mathbf {h}}_2}$ there exists a constant C depending only on m and a constant $D>0$ depending only on $m, T, \Vert {\mathbf {u}}_2(0)\Vert _{H^m}, \Vert {\mathbf {u}}_1(0)\Vert _{H^{m+1}}, \Vert {\mathbf {g}}+ {\mathbf {h}}_2\Vert _{H^m} , \Vert {\mathbf {g}}+ {\mathbf {h}}_1 \Vert _{H^{m+1}}$ such that

  $$\begin{aligned} \Vert {\mathbf {u}}_1(t)-{\mathbf {u}}_2(t) \Vert _{H^m}^2 \le \Vert {\mathbf {u}}_1(0)-{\mathbf {u}}_2(0)\Vert _{H^m}^2 e^{D t} + C_m \int _0^t e^{D(t-s)} \Vert {\mathbf {h}}_1 - {\mathbf {h}}_2 \Vert _{H^m}^2 \, ds. \end{aligned}$$

Proof of Proposition A.5

For the proof of (i), see [33, 35]. To see (ii), first note that for ${\mathbf {h}}\in X_0$ and ${\mathbf {u}}_0 \in {\mathcal {X}}$, the fact that $\tau _{{\mathbf {u}}_0, {\mathbf {h}}}>0$ and $\tau _{{\mathbf {u}}_0, {\mathbf {h}}} \le T_{{\mathbf {u}}_0, {\mathbf {h}}}$ follow from (i) and the Gagliardo-Nirenberg inequality. To obtain the claimed estimate, let ${\mathbf {f}}= {\mathbf {g}}+ {\mathbf {h}}$ and observe that for all multi-indices $\beta$ with $|\beta |\le m$, $m\ge 3$, we have the estimate

$$\begin{aligned} \frac{1}{2}\frac{d}{dt}\Vert \partial ^\beta {\mathbf {u}}(t)\Vert ^2&= \langle \partial ^\beta {\mathbf {u}}(t), \partial ^\beta {\mathbf {f}}\rangle - \langle \partial ^\beta {\mathbf {u}}(t), \partial ^\beta B({\mathbf {u}}(t), {\mathbf {u}}(t))\rangle \\&\le \Vert {\mathbf {u}}(t) \Vert _{H^m} \Vert {\mathbf {f}}\Vert _{H^m} - \langle \partial ^\beta {\mathbf {u}}(t), \partial ^\beta B({\mathbf {u}}(t), {\mathbf {u}}(t)) - B({\mathbf {u}}(t), \partial ^\beta {\mathbf {u}}(t))\rangle \end{aligned}$$

where in the inequality we used the fact that $\langle \partial ^\beta {\mathbf {u}}(t), B({\mathbf {u}}(t), \partial ^\beta {\mathbf {u}}(t))\rangle =0$ as ${\mathbf {u}}(t)$ is divergence-free. To estimate the contribution from the nonlinear term, we first observe that by interpolation and Agmon’s inequality

$$\begin{aligned} \Vert \partial ^\beta B({\mathbf {u}}(t), {\mathbf {u}}(t)) - B({\mathbf {u}}(t), \partial ^\beta {\mathbf {u}}(t)) \Vert&\le c_{m} \Vert {\mathbf {u}}(t)\Vert _{W^{1, \infty }} \Vert {\mathbf {u}}(t) \Vert _{H^m}\\&\le c_{m}' \Vert {\mathbf {u}}(t) \Vert _{H^3} \Vert {\mathbf {u}}(t)\Vert _{H^m} \end{aligned}$$

as $m\ge 3$, where $c_{m}, c'_{m}$ are constants depending only on m. Putting these estimates together, we find that

$$\begin{aligned} \frac{1}{2}\frac{d}{dt}\Vert \partial ^\beta {\mathbf {u}}(t)\Vert ^2&\le \Vert {\mathbf {u}}(t) \Vert _{H^m} \Vert {\mathbf {f}}\Vert _{H^m} + c'_{m} \Vert {\mathbf {u}}(t)\Vert _{H^3} \Vert {\mathbf {u}}(t)\Vert _{H^m}^2. \end{aligned}$$

Summing over all multi-indices $\beta$ with $|\beta | \le m$ and using Young’s inequality produces

$$\begin{aligned} \frac{1}{C_{m}} \frac{d}{dt}\Vert {\mathbf {u}}(t) \Vert _{H^m}^2 \le \Vert {\mathbf {f}}\Vert _{H^m}^2 + (1 + \Vert {\mathbf {u}}(t)\Vert _{H^3}) \Vert {\mathbf {u}}(t) \Vert _{H^m}^2 \end{aligned}$$

for some constant $C_{m}$ depending only on m. Supposing that $t< \tau ^n_{{\mathbf {u}}_0, {\mathbf {h}}}$, Gronwall’s inequality then implies the claimed estimate in (ii).

To prove (ii), let ${\mathbf {w}}(t) = {\mathbf {u}}_1(t)- {\mathbf {u}}_2(t)$. Then for $m\ge 3$ and any multi-index $\beta$ with $|\beta | \le m$ we have the estimate

$$\begin{aligned} \frac{1}{2}\frac{d}{dt}\Vert \partial ^\beta {\mathbf {w}}(t) \Vert ^2&= \langle \partial ^\beta {\mathbf {w}}(t), \partial ^\beta ( {\mathbf {h}}_1- {\mathbf {h}}_2)\rangle \\&\quad + \langle \partial ^\beta {\mathbf {w}}(t), \partial ^\beta B({\mathbf {u}}_2(t), {\mathbf {u}}_2(t)) - \partial ^\beta B({\mathbf {u}}_1(t), {\mathbf {u}}_1(t))\rangle \\&\le \Vert {\mathbf {w}}(t) \Vert _{H^m} \Vert {\mathbf {h}}_1-{\mathbf {h}}_2 \Vert _{H^m} + \langle \partial ^\beta {\mathbf {w}}(t), \\&\quad \partial ^\beta B({\mathbf {u}}_2(t), {\mathbf {u}}_2(t)) - \partial ^\beta B({\mathbf {u}}_1(t), {\mathbf {u}}_1(t))\rangle \\&= \Vert {\mathbf {w}}(t) \Vert _{H^m} \Vert {\mathbf {h}}_1-{\mathbf {h}}_2 \Vert _{H^m} \\&\qquad - \langle \partial ^\beta {\mathbf {w}}(t), \partial ^\beta B({\mathbf {w}}(t), {\mathbf {u}}_1(t)) + \partial ^\beta B({\mathbf {u}}_2(t), {\mathbf {w}}(t))\\&\qquad - B({\mathbf {u}}_2(t), \partial ^\beta {\mathbf {w}}(t))\rangle \end{aligned}$$

where again we used the fact that ${\mathbf {u}}_2$ is divergence-free as $\langle \partial ^\beta {\mathbf {w}}(t), B({\mathbf {u}}_2(t), \partial ^\beta {\mathbf {w}}(t))\rangle =0.$ Note by interpolation

$$\begin{aligned}&| \langle \partial ^\beta {\mathbf {w}}(t), \partial ^\beta B({\mathbf {w}}(t), {\mathbf {u}}_1(t)) + \partial ^\beta B({\mathbf {u}}_2(t), {\mathbf {w}}(t))- B({\mathbf {u}}_2(t), \partial ^\beta {\mathbf {w}}(t))\rangle |\\&\quad \le c_{m} (\Vert {\mathbf {w}}(t)\Vert _{H^m}^2 \Vert {\mathbf {u}}_1(t) \Vert _{H^{m+1}} + \Vert {\mathbf {w}}(t)\Vert _{H^m}^2 \Vert {\mathbf {u}}_2(t)\Vert _{H^m}). \end{aligned}$$

for some constant $c_{m}$ depending only on m. Thus combining this inequality with the previous, summing over all multi-indices $\beta$ with $|\beta | \le m$ and applying Young’s inequality produces the following bound

$$\begin{aligned} \frac{1}{C}\frac{d}{dt}\Vert {\mathbf {w}}(t) \Vert _{H^m}^2 \le \Vert {\mathbf {h}}_1 - {\mathbf {h}}_2\Vert _{H^m}^2 + \Vert {\mathbf {w}}(t)\Vert _{H^m}^2 ( \Vert {\mathbf {u}}_1(t)\Vert _{H^{m+1}} + \Vert {\mathbf {u}}_2(t) \Vert _{H^m}+1) \end{aligned}$$

for some constant $C>0$ depending only on m. Now for any $T>0$ if $t< \tau _{{\mathbf {u}}_1(0), {\mathbf {h}}_1}^n\wedge \tau _{{\mathbf {u}}_2(0), {\mathbf {h}}_2}^n\wedge T$, by the estimate in (ii) and Gronwall’s inequality there exists a constant $D>0$ depending only on $m, T, \Vert {\mathbf {u}}_2(0)\Vert _{H^m}, \Vert {\mathbf {u}}_1(0)\Vert _{H^{m+1}}, \Vert {\mathbf {g}}+ {\mathbf {h}}_2\Vert _{H^m} , \Vert {\mathbf {g}}+ {\mathbf {h}}_1 \Vert _{H^{m+1}}$ such that

$$\begin{aligned} \Vert {\mathbf {w}}(t) \Vert _{H^m}^2 \le \Vert {\mathbf {w}}(0)\Vert _{H^m}^2 e^{D t} + C \int _0^t e^{D(t-s)} \Vert {\mathbf {h}}_1 - {\mathbf {h}}_2 \Vert _{H^m}^2 \, ds. \end{aligned}$$

This finishes the proof of the estimate in (iii). $\square$

Appendix B: Comparison Theorem

For the estimates in Section 5, we make repeated use of the following comparison principal.

Proposition B.1

Let $f:{\mathbf {R}}\rightarrow {\mathbf {R}}$ be locally Lipschitz continuous. Fix $0 < T \le \infty$ and suppose that $\phi :[0, T) \rightarrow [0, \infty )$ is continuous and satisfies

$$\begin{aligned} \phi (t) = \phi (s) + \int _s^t f(\phi (u)) \, du \end{aligned}$$

for all $0\le s\le t < T$. On the other hand suppose that for some $0 < S \le \infty$, $\psi :[0, S)\rightarrow [0, \infty )$ is continuous with $\psi (0) = \phi (0)$,

$$\begin{aligned} \limsup _{t \rightarrow S} \psi (t) = \infty \end{aligned}$$

and

$$\begin{aligned} \psi (t) \le \psi (s) + \int _s^t f(\psi (u)) \, du \end{aligned}$$

for all $0 \le s\le t < T \wedge S$. Then $S \ge T$ and $\psi (t) \le \phi (t)$ for all $0\le t \le T$.

In particular, we will leverage this proposition for the estimates above in the form of the following corollary.

Corollary B.2

Let $T>0$. Suppose that for every $\lambda > 0$, there exists a $T_\lambda \in (0,\infty ]$ and a $C^1$-function $x_\lambda : [0,T_\lambda ) \rightarrow [0,\infty )$ satisfying

$$\begin{aligned} \frac{d x_\lambda }{dt} \le \frac{c_0}{\lambda }(x_\lambda ^p + \kappa _0) \text { on } [0, T\wedge T_\lambda ) \qquad \,\text { and } \, \qquad \limsup _{t \rightarrow T_\lambda } x_\lambda (t) = \infty , \end{aligned}$$

(B.1)

where $c_0, \kappa _0> 0$ and $p > 1$ are constants independent of $\lambda >0$. For $\gamma , \lambda >0$ and $t\ge 0$, define

$$\begin{aligned} T^*_{\lambda }(\gamma ) = \frac{\lambda }{2 c_0 (p-1)\gamma ^{p-1}} \quad \text { and } \quad R_\lambda (t, \gamma ) = \left( 1 - \frac{2 c_0 (p-1) \gamma ^{p-1} }{\lambda } \, t \right) ^{-\frac{1}{p-1}}. \end{aligned}$$

(B.2)

Then for all $0 \le t \le T_\lambda ^*(x_\lambda (0) + \kappa _0) \wedge T$ we have

$$\begin{aligned} x_\lambda (t) \le x_\lambda (0) R_\lambda (t, x_\lambda (0) + \kappa _0) + \kappa _0( R_\lambda (t,x_\lambda (0) + \kappa _0 ) -1) \end{aligned}$$

(B.3)

Remark B.3

Observe that if $x_\lambda (0)=x_0 \ge 0$ is independent of $\lambda >0$, then the comparison (B.3) holds for all $t\in [0, T]$ and all $\lambda \ge 2 c_0 T(p-1) (x_0 + \kappa _0)^{p-1}$.

Let us first prove Corollary B.2 using Proposition B.1 and then establish the Proposition thereafter.

Proof of Corollary B.2

Under the given conditions on $x_\lambda$ notice that

$$\begin{aligned} \frac{d (x_\lambda + \kappa _0)}{dt} \le \frac{2c_0}{\lambda }(x_\lambda + \kappa _0)^p \end{aligned}$$

Now consider y solving

$$\begin{aligned} \frac{d y}{dt} = \frac{2c_0}{\lambda }y^p \quad y(0) = y_0. \end{aligned}$$

When $y_0 \ge 0$, this equation has the unique solution

$$\begin{aligned} y(t, y_0) := y_0 \left( 1 - t \, \frac{2c_0 (p-1)}{\lambda } \, y_0^{p-1} \right) ^{-\frac{1}{p-1}}. \end{aligned}$$

defined on the interval $[0,\frac{\lambda }{2c_0 (p-1) y_0^{p-1}})$. Thus, by comparing $y(\cdot , x_\lambda (0) + \kappa _0)$ to $x_\lambda + \kappa _0$, we obtain the desired result by invoking Proposition B.1. $\square$

Proof of Proposition B.1

We first show that $\psi$ remains below $\phi$ on their common interval of definition. Let $R < T \wedge S$ and define

$$\begin{aligned} T_0 := \inf _{ t \in [0, R)} \{ \psi (t) > \phi (t) \} \wedge R. \end{aligned}$$

(B.4)

Let us show that $T_0 = R$. If not, then there exist times $T_0 \le T_1< T_2 < R$ such that

$$\begin{aligned} \psi (T_1) = \phi (T_1) \text { and } \psi (t) > \phi (t) \text { for every } T_1 < t \le T_2. \end{aligned}$$

Take

$$\begin{aligned} K= \{\phi (t) \, : \, t \in [T_1, T_2]\}\cup \{ \psi (t) \, : \, t \in [T_1, T_2] \}. \end{aligned}$$

By the continuity of $\phi$ and $\psi$, K is compact and since f is locally Lipshitz, there exists a constant $C_K>0$ such that

$$\begin{aligned} |f(u) - f(v) | \le C_K|u-v| \,\,\text { for all } \,\, u,v \in K. \end{aligned}$$

Now, for $T_1 < t \le T_2$,

$$\begin{aligned} 0 < \psi (t)-\phi (t) \le \int _{T_1}^t f(\psi (r)) - f(\phi (r)) \, dr \le C_K \int _{T_1}^t \psi (r) - \phi (r) \, du. \end{aligned}$$

Invoking Grönwall’s inequality, we have that $\psi (t) = \phi (t) = 0$ for $t \in [T_1, T_2]$, a contridiction.

To show that $T \ge S$ we again argue by contridiction and suppose on the contrary that $S < T$. Take

$$\begin{aligned} S_n = \inf _{t \in [0,S)} \{ \psi (t) > n \}. \end{aligned}$$

Then, by what we have already established, $\phi (S_n) \ge \psi (S_n) = n$. This in turn would imply that $\sup _{t \in [0,S]} \phi (t) = \infty$, violating the continuity of $\phi$ and yielding the desired contridiction. The proof is complete. $\square$