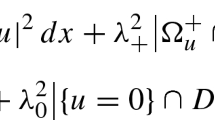Two-phase Free Boundary Problems in Convex Domains

Abstract

We study the regularity of minimizers of a two-phase free boundary problem. For a class of n-dimensional convex domains, we establish the Lipschitz continuity of the minimizer up to the fixed boundary under Neumann boundary conditions. Our proof uses an almost monotonicity formula for the Alt–Caffarelli–Friedman functional restricted to the convex domain. This requires a variant of the classical Friedland–Hayman inequality for geodesically convex subsets of the sphere with Neumann boundary conditions. To apply this inequality, in addition to convexity, we require a Dini condition governing the rate at which the fixed boundary converges to its limit cone at each boundary point.

Appendix

In the Appendix we record the proofs of some of the technical propositions and lemmas needed to prove Theorems 2.3 and 2.4.

Proof of Theorem 4.1

We first prove Theorem 4.1 without Assumption 4.2 via an approximation argument. From Sect. 4, the result of Theorem 4.1 holds when W is smooth and strictly geodesically convex, and $\gamma ^{\pm }$ are $(n-2)$-dimensional submanifolds, smooth up to the boundary of W. The first step in the proof is therefore to approximate W and $\gamma ^{\pm }$. $\square$

Proposition 9.1

Given $\epsilon , \delta >0$, there exist a smooth, strictly geodesically convex set $W_{\epsilon }\subset W$, and $(n-2)$-dimensional manifolds $\gamma ^{\pm }_{\delta }\subset {\bar{W}}^{\pm }$, smooth up to the boundary of $\partial W$, such that

$$\begin{aligned} Haus _{\mathbb {S}^{n-1}}(W_{\epsilon },W)<\epsilon , \qquad Haus _{\mathbb {S}^{n-1}}(\gamma ^{\pm }_{\delta },\gamma ^{\pm }) <\delta . \end{aligned}$$

Here $Haus _{\mathbb {S}^{n-1}}(\cdot ,\cdot )$ measures the Hausdorff distance between the sets in $\mathbb {S}^{n-1}$.

Proof of Proposition 9.1

We first construct the set $W_{\epsilon }$. Since W is a proper, convex subset of $\mathbb {S}^{n-1}$, it must be contained in a hemisphere, say $\mathbb {S}^{n-1}\cap \{x_{n}\ge 0\}$. We use $W\subset \mathbb {S}^{n-1}$ to form a n-dimensional cone R, with vertex at the origin. By the convexity of W, R is a Euclidean convex subset of $\mathbb {R}^{n}$, and we set K to be the $(n-1)$-dimensional cross section $K = R\cap \{x_{n} =1\}$. We can therefore form a sequence $K_{\epsilon }\subset K$ of smooth, strictly convex, bounded sets, which converge to K in Hausdorff distance on compact sets as $\epsilon$ tends to 0, [25]. Using $K_{\epsilon }$ to form the sequence of strictly Euclidean convex cones $R_{\epsilon }$, and then restricting to $\mathbb {S}^{n-1}$, we obtain a sequence of strictly convex, smooth sets $W_{\epsilon } \subset W$, converging to W in Hausdorff measure on $\mathbb {S}^{n-1}$ as $\epsilon$ tends to 0.

To construct the smooth sets $\gamma ^{\pm }_\delta$ we proceed as follows: Given $\eta >0$, since $w\in C^{\alpha }(W)$, the distance between $\{w > \eta \}$ and $\partial \{w > 0\}$ is strictly positive, and tends to zero as $\eta$ tends to 0. We approximate w from below by a sequence $w_n\in C^{\infty }(W)$, converging uniformly to w on W. Therefore, by choosing $n = n(\delta )$ sufficiently large and $\eta = \eta (\delta )>0$ sufficiently small and using Sard’s lemma, we can construct a smooth set $\gamma ^{+}_\delta = \{w_n > \eta \}$ contained in $\{w>0\}$, with the required properties, and analogously for $\gamma ^{-}_{\delta }$. $\square$

Suppose first that one of $\gamma _{\delta }^{\pm }$ does not intersect $W_{\epsilon }$ for some $\delta$, $\epsilon >0$. Then, one of $W^{\pm }$ is contained in $W\backslash W_{\epsilon }$. If this holds for $\delta$ and $\epsilon >0$ sufficiently small, then since

$$\begin{aligned} \lim _{\epsilon \rightarrow 0} \text {Vol}_{\mathbb {S}^n}(W\backslash W_{\epsilon }) =0, \qquad \lim _{\delta \rightarrow 0}\text {Haus}_{\mathbb {S}^n}(\gamma ^{\pm }_{\delta },\gamma ^{\pm }) =0, \end{aligned}$$

one of $\alpha (W^{\pm })$ must be larger than 2, and the estimate in Theorem 4.1 holds automatically. Therefore, from now on we will assume that $\gamma _{\delta }^{\pm }$ both intersect $W_{\epsilon }$.

To complete the proof of the theorem, we will show that the approximation statement in Proposition 9.1 carries over to the Dirichlet–Neumann eigenvalues.

Definition 9.2

Denoting $\gamma ^{\pm }_{\epsilon ,\delta }$ to be the part of $\gamma ^{\pm }_{\delta }$ contained in $W_{\epsilon }$, let $W^{\pm }_{\epsilon ,\delta } \subset W_{\epsilon }$ be the set with boundary consisting of $\gamma ^{\pm }_{\epsilon ,\delta }$ and the part of $\partial W_{\epsilon }$ contained in $W^{\pm }$. We also denote $W^{\pm }_{\epsilon }$ to be the sets $W_{\epsilon }\cap W^{\pm }$, which correspond to $W^{\pm }_{\epsilon ,\delta }$ with $\delta =0$.

Definition 9.3

Let $\mu ^{\pm }_{\epsilon ,\delta }$ be the first eigenvalue for $({W}^{\pm }_{\epsilon ,\delta },g_{\epsilon })$, with Dirichlet boundary conditions imposed on ${\gamma }^{\pm }_{\epsilon ,\delta }$,and Neumann boundary conditions elsewhere. Let $\mu ^{\pm }_{\epsilon }$ be the first eigenvalue for ${W}^{\pm }_{\epsilon }$, with Dirichlet boundary conditions imposed on $\gamma ^{\pm }$, and Neumann boundary conditions on the rest of $\partial W^{\pm }_{\epsilon }$.

The proof of Theorem 4.1 is completed by the following lemma:

Lemma 9.4

The limits $\lim _{\delta \rightarrow 0} \mu ^{\pm }_{\epsilon ,\delta } = \mu _{\epsilon }^{\pm }$, $\limsup _{\epsilon \rightarrow 0} \mu _{\epsilon }^{\pm } \le \mu ^{\pm }$ both hold.

Proof of Lemma 9.4

Since $\lim _{\epsilon \rightarrow 0} \text {Vol}_{\mathbb {S}^n}(W\backslash W_{\epsilon }) = 0$, the second limit in the lemma follows immediately from Lemma 5.3. To establish the first limit we proceed as follows. By the variational formulation of the first eigenvalue, we have

$$\begin{aligned} \mu ^{\pm }_{\epsilon ,\delta } = \inf _{\phi \in X^{\pm }_{\epsilon ,\delta }} \frac{\int _{{W}^{\pm }_{\epsilon ,\delta }} \left| \nabla _{g}\phi \right| ^2 \mathrm{{d}}\sigma _{g}}{\int _{{W}^{\pm }_{\epsilon ,\delta }} \phi ^2 \mathrm{{d}}\sigma _{g}}. \end{aligned}$$

for $X^{\pm }_{\epsilon ,\delta } = \{\phi \in C^{\infty }({W}^{\pm }_{\epsilon ,\delta }) : \text {supp}(\phi ) \cap \gamma ^{\pm }_{\epsilon ,\delta } =\emptyset , \phi \ne 0\}.$ Define the set of functions $X^{\pm }_{\epsilon }$ by $X^{\pm }_{\epsilon } = \{\phi \in C^{\infty }({W}^{\pm }_{\epsilon }) : \text {supp}(\phi ) \cap \gamma ^{\pm } =\emptyset , \phi \ne 0\}.$ Since $X^{\pm }_{\epsilon ,\delta }\subset X^{\pm }_{\epsilon }$, we immediately obtain $\mu ^{\pm }_{\epsilon ,\delta }\ge \mu ^{\pm }_{\epsilon }$. Moreover, given any $\phi \in X^{\pm }_{\epsilon }$, since the Hausdorff distance between $\gamma _{\epsilon ,\delta }$ and $\gamma$ tends to 0 as $\delta$ tends to 0 (uniformly in $\epsilon >0$), we see that $\phi \in X^{\pm }_{\epsilon ,\delta }$ for $\delta >0$ sufficiently small (uniformly in $\epsilon >0$). In particular, given $c>0$, we can choose $\delta$ sufficiently small so that $\mu ^{\pm }_{\epsilon ,\delta } \le \mu ^{\pm }_{\epsilon } +c$. Thus, $\lim _{\delta \rightarrow 0}\mu ^{\pm }_{\epsilon ,\delta } = \mu ^{\pm }_{\epsilon },$ as required. $\square$

Regularity of the spherical slices $\mathbf {V}_{\mathbf{t}}$: In Sect. 5, we required Lemma 5.4 to prove properties of the spherical sections ${V_{t}}$ for those values of t where $t^{-1}M_\mathbf{0 }(t)$ is sufficiently small. We now prove this lemma and its corollary, and we start by restating Lemma 5.4:

Lemma 9.5

There exists a constant $c>0$, depending only on the Lipschitz norm of $\partial \Omega$, and an orientation of $\Omega$ with the following property: For $t\in {S_{c}}$, the part of the boundary $\partial \Omega \cap \left( B_{2t}\backslash B_{t/2}\right)$ can be written as the graph $x_n = g(x')$ of a convex function g, with Lipschitz constant depending only on that of $\partial \Omega$. Here we have written $x = (x',x_n)\in \mathbb {R}^n$.

Proof of Lemma 9.5

We fix an orientation of $\Omega$ by looking at the slice $V_1$: This slice $V_1\subset \mathbb {S}^{n-1}$ contains a geodesic ball of radius $r^*>0$, and we rotate $\Omega$ so that the center of this geodesic ball is at the north pole $(0,\ldots ,0,1)\in \mathbb {S}^{n-1}$. Since the slices $V_t$ form a increasing sequencs as t decreases, $V_t$ contains this geodesic ball for all $t<1$. Moreover, since $V_t$ is always contained in a hemisphere, this means that $V_t$ cannot contain the corresponding neighborhood of the south pole.

Now let $y\in \partial \Omega \cap \left( B_{2t}\backslash B_{t/2}\right)$, with outward pointing unit normal $\nu (y)$. To prove the lemma, we will show that the component of $\nu (y)$ in the $x_n$-direction is bounded above by $-c^*$, for a constant $c^*>0$ depending only on the Lipschitz constant of $\partial \Omega$. After a rotation in the $x'$-variables, we can assume that y lies in the $(x_1x_n)$-plane. Writing $\nu (y) = (\nu _1,\ldots ,\nu _n)$, we first obtain a lower bound on $\nu _1^2+\nu _n^2$ as follows: Since $\Omega$ is convex, the tangent plane at y provides a supporting hyperplane for $\Omega$. Therefore, if $\nu _1^2+\nu _n^2$ is small relative to $r^*$, then this would contradict the spherical slices $V_t$ containing the geodesic ball of radius $r^*$ centered at the north pole.

Now that we have a lower bound on $\nu _1^2+\nu _n^2$, we use the upper bound on $s^{-1}M_\mathbf{0 }(s)$ for $s\in [\tfrac{1}{2}t,2t]$ to conclude the proof. Since $t\in S_{c}$, we have

$$\begin{aligned} |y|^{-1}\left( y_1\nu _1 + y_n\nu _n\right) = \frac{y}{|y|}\cdot \nu (y) \le c, \end{aligned}$$

so that the vectors $\tfrac{y}{|y|}$ and $(\nu _1,\nu _n)$ are almost orthogonal in the $(x_1x_n)$-plane. Since $\tfrac{y}{|y|}$ cannot be within distance $r^*$ from the north or south pole, by taking c sufficiently small depending on $r^*$, this provides a lower bound on $|\nu _n|$. Finally, $\nu _n$ must be negative, since otherwise this would again contradict $V_t$ containing the north pole. $\square$

Corollary 9.6

For c and the orientation of $\Omega$ as in Lemma 5.4, and for $s\in [\tfrac{1}{2}t,2t]$ with $t\in S_c$, the slices $V_s$ are star-shaped with respect to the north pole in $\mathbb {S}^{n-1}$. Moreover, there exists a neighborhood around each $y\in \partial V_s$ on which $\partial V_s$ can be parameterized as submanifold $(x'(\tau ),g(x'(\tau )))$ for $\tau$ in an open set in $\mathbb {R}^{n-2}$. The Lipschitz constant of this parameterization and the size of the neighborhood can be taken to only depend on that of $\partial \Omega$.

Proof of Corollary 9.6

To establish the star-shaped property, it is sufficient to show that every great circle passing through the north pole intersects $\partial V_s$ at precisely two points. Without loss of generality, after a rotation in the $x'$ variables, we may take this great circle to be in $(x_1x_{n})$-plane, given by $\{x_2=x_3=\cdots =x_{n-1}=0\}\cap \mathbb {S}^{n-1}$. Let $y \in \partial V_s$ be on this great circle, with $y_1>0$. Then $sy\in \partial \Omega$, and writing $\nu (sy) = (\nu _1,\ldots , \nu _n)$, from Lemma 5.4 we know that $\nu _n \le - c_n$ for some constant $c_n >0$, and $|y|^{-1}\left( y_1\nu _1 + y_n\nu _n\right) \le c$. Since as in the proof of Lemma 5.4, the tangent plane at y is a supporting hyperplane for $\Omega$, for $c>0$ sufficiently small there exists no point z in $\Omega$ on this great circle with $z_1>0$ and $z_n<y_n$. The analogous argument applies for the portion of the circle with $x_1<0$, and so this gives the star-shaped property.

We now establish the Lipschitz parameterization of $\partial V_s$. After a rotation in the $x'$-plane, let $y=(y_1,0,\ldots ,0,s^{-1}g(sy'))\in \partial V_s$ with $y_1>0$. Here g is the convex function from Lemma 5.4. Let ${v}(x_1,x_2)$ be given by

$$\begin{aligned} {v}(x') = x_1^2+\cdots +x_{n-1}^2 + s^{-2}g(sx')^2 - 1. \end{aligned}$$

Then, ${x}\in \partial V_s$ if and only if $x_n = s^{-1}g(sx')$ and $v(x') = 0$. In particular, $v(y') = 0$, and to establish the Lipschitz parameterization near this point, we will apply the implicit function theorem to the set $\{x':v(x') = 0\}$. We therefore, need to obtain a lower bound on

$$\begin{aligned} \partial _{x_1}v(x') = 2x_1 + 2s^{-1}g(sx')(\partial _{x_1}g)(sx'), \end{aligned}$$

(59)

for $x'$ near $y'$. To obtain a lower bound on this quantity, we will use $M_\mathbf{0 }(t)$. For $|x| = s\in [\tfrac{1}{2}t,2t]$, we have

$$\begin{aligned} \nu (x)\cdot x = (1+|\nabla g(x')|^2)^{-1/2}(\partial _{x_1}g(x'),\ldots , \partial _{x_{n-1}}g(x'),-1)\cdot (x',g(x')) \le cs, \end{aligned}$$

which we can rearrange as $g(x') \ge \nabla g(x')\cdot x' - cs(1+|\nabla g(x')|^2)^{1/2}.$ Inserting this in (59) we have

$$\begin{aligned} \partial _{x_1}v(x')&\ge 2x_1 + 2\partial _{x_1}g(sx')\left( \nabla g(sx')\cdot x'\right) - 2c\partial _{x_1}g(sx')(1+|\nabla g(sx')|^2)^{1/2} \\&= 2x_1 + 2x_1(\partial _{x_1}g(sx'))^2 + \text { Error}. \end{aligned}$$

Here the error terms consist of terms involving a factor of $x_2,\ldots ,x_{n-1}$ or c. In particular, for $x'$ sufficiently near the point $y' = (y_1,0,\ldots ,0)$ and for $c>0$ sufficiently small, the Error term is smaller than $x_1$, and we obtain the desired lower bound on $\partial _{x_1}v(x')$. $\square$

Proof of Proposition 7.2

We end by proving Proposition 7.2, which establishes a subdomain of $\Omega$ satisfying an $L^{\infty }$ harmonic measure estimate. $\square$

Proposition 9.7

There exist constants $c_1$, $C_1$, depending only on the Lipschitz norm of $\partial \Omega$, such that for each ${x}_0\in \Omega \cap B_{1/2}$, we can form a convex domain $\Omega _{{x}_{0}}\subset \Omega$, with the following properties:

• (i) The boundary of $\Omega _{{x}_{0}}$ consists of two parts $\partial \Omega _{{x}_{0},N}$ and $\partial \Omega _{{x}_{0},D}$. The first part is a (possibly empty) subset of $\partial \Omega$, and the second part ensures that $B_{c_1}({x}_{0}) \cap \Omega \subset \Omega _{{x}_{0}}$.

• (ii) Let G be the Green’s function for $\Omega _{{x}_{0}}$, with pole at ${x}_{0}$, with zero Dirichlet boundary conditions on $\partial \Omega _{{x}_{0},D}$, and zero Neumann boundary conditions (weakly) on $\partial \Omega _{{x}_{0},N}$. Then,

  $$\begin{aligned} {\left\Vert G\right\Vert }_{L^{\infty }\left( \Omega _{{x}_{0}}\backslash B_{c_1}({x}_0)\right) } \le C_1, \qquad {\left\Vert \nabla G\right\Vert }_{L^{\infty }\left( \partial \Omega _{{x}_{0},D}\right) } \le C_1 . \end{aligned}$$

Proof of Proposition 9.7

Let $y_0$ be the closest point to $x_0$ on $\partial \Omega$. After a translation, we set ${y}_0 = \mathbf{0}$. Now, let $c>0$ be a small constant, as in the statement of Lemma 5.4, and fix $c_1$ with $2c_1\in S_{c}$. If $|x_0|\ge c_1$, we can take $\Omega _{{x}_0} = B_{c_1}(x_0)$. Otherwise, choose $z_0 = (0,\ldots ,0,-|z_0|)$, with $|z_0| = \tfrac{1}{10}c_1$, and set $\Omega _{{x}_0} = B_{2c_1}(z_0)\cap \Omega$. In either, case part i) of the lemma is satisfied.

To obtain the estimates on G, we show that the Dirichlet, $\partial B_{2c_1}(z_0)\cap \Omega$, and Neumann, $B_{2c_1}(z_0)\cap \partial \Omega$, parts of the boundary meet at a strictly acute angle at each point of their intersection: For $y\in \partial B_{2c_1}(z_0)\cap \partial \Omega$, we have

$$\begin{aligned} (y-z_0)\cdot \nu (y) = y\cdot \nu (y) - z_0\cdot \nu (y) \le |y|M_\mathbf{0 }(|y|) - z_0\cdot \nu (y). \end{aligned}$$

We have $|y|^{-1}M_\mathbf{0 }(|y|) \le c$, and by Lemma 5.4, there exists a constant $c^*$, independent of c, such that $z_0\cdot \nu (y) \ge c^*|z_0|$. Therefore, choosing $c<\tfrac{1}{20}c^*$, we have

$$\begin{aligned} (y-z_0)\cdot \nu (y) \le - \tfrac{1}{20}c^*c_1. \end{aligned}$$

Since $|y-z_0|$ is comparable to $c_1$, this shows that $\partial \Omega$ and $B_{2c_1}(z_0)$ meet at a strictly acute angle at y. With this property, we can therefore use the maximum principle with a linear function to show that the Green’s function G(x) decays (at least) linearly as x approaches each point $y\in \partial B_{2c_1}(z_0)\cap \partial \Omega$. Since near $\partial B_{2c_1}(z_0)\cap \partial \Omega$, the function G is a harmonic function on a Lipschitz domain, this implies the desired estimate for $\nabla G$. $\square$