An inspection game of internal audit and the influence of whistle-blowing

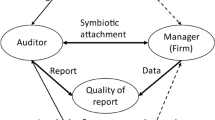
Abstract

In this paper we analyse how whistle-blowing affects fraudulent behaviour of managers while the company instigates imperfect internal audit to detect the fraud. To do so, we employ in a first step a non-cooperative inspection game to analyse fraudulent behaviour of a manager controlled by an internal auditor. In a second step we introduce exogenous whistle-blowing of a manager’s employee to aid the auditor to reveal the fraud. In a third step, the two-person inspection game is extended to a three-person approach with endogenous whistle-blowing. Our novel results are that the intensity of internal audit is always lower with whistle-blowing than without and that whistle-blowing renders the manager to act less fraudulently than compared to the basic inspection game if and only if she is unaware of the whistle-blower’s expected pay-off and the efficacy of internal audit is sufficiently low.

Appendices

Appendix

Comparative static analysis of (31) and (32)

Differentiating (31) with respect to the according parameters while taking account of (1), (2) and (28) provides

$$\begin{aligned} \frac{\partial \tilde{p}_h^*}{\partial \Gamma }&= \frac{1}{p_r \cdot (\Delta - \rho ^-) \cdot (1 - p_d)} > 0, \\ \frac{\partial \tilde{p}_h^*}{\partial \Delta }&= - \frac{\Gamma - \rho ^-}{p_r \cdot (\Delta - \rho ^-)^2 \cdot (1 - p_d)}< 0,\\ \frac{\partial \tilde{p}_h^*}{\partial \rho ^-}&= \frac{\Gamma - \Delta }{p_r \cdot (\Delta - \rho ^-)^2 \cdot (1 - p_d)}< 0, \\ \frac{\partial \tilde{p}_h^*}{\partial p_r}&= - \frac{\Gamma - \rho ^- - (\Delta - \rho ^-) \cdot p_d}{p_r^2 \cdot (\Delta - \rho ^-) \cdot (1 - p_d)}< 0, \\ \frac{\partial \tilde{p}_h^*}{\partial p_d}&= \frac{\Gamma - \Delta }{p_r \cdot (\Delta - \rho ^-) \cdot (1 - p_d)^2} < 0. \end{aligned}$$

Let $M \equiv \left( (1 - p_d) \cdot p_r \cdot (R^+ + R^-) + p_d \cdot (1 - z) \cdot R^+ \right) ^2$. Differentiating (32) with respect to the relevant parameters subject to (16) leads to

$$\begin{aligned} \frac{\partial \tilde{p}_f^*}{\partial K}&= \frac{1}{M}> 0,\\ \frac{\partial \tilde{p}_f^*}{\partial R^+}&= - \frac{K \cdot \left( (1 - p_d) \cdot p_r + p_d \cdot (1 - z) \right) }{M}< 0,\\ \frac{\partial \tilde{p}_f^*}{\partial R^-}&= - \frac{(1 - p_d) \cdot p_r \cdot K}{M}< 0,\\ \frac{\partial \tilde{p}_f^*}{\partial p_r}&= - \frac{(1 - p_d) \cdot K \cdot (R^+ + R^-)}{M}< 0,\\ \frac{\partial \tilde{p}_f^*}{\partial p_d}&= \frac{K \cdot \left( p_r \cdot (R^+ + R^-) - (1 - z) \cdot R^+ \right) }{M} \begin{Bmatrix}< \\> \end{Bmatrix} 0 \ \text { if } \ p_r \begin{Bmatrix} < \\> \end{Bmatrix} \frac{(1 - z) \cdot R^+}{R^+ + R^-},\\ \frac{\partial \tilde{p}_f^*}{\partial z}&= \frac{K \cdot R^+}{M} > 0. \end{aligned}$$

2.1 Proof of proposition 1

To prove proposition 1 (i), exploit (1), (17) and (31) and simplify to obtain

$$\begin{array}{lrcl} & {p}_h^* & > & \tilde{p}_h^* \\ \\ \Leftrightarrow & \frac{\Gamma - \rho^-}{p_r \cdot (\Delta - \rho^-)} & > &\frac{\Gamma - \rho^- - (\Delta - \rho^-) \cdot p_d}{p_r \cdot (\Delta - \rho^-) \cdot (1 - p_d)} \\ \\ \Leftrightarrow & (1 - p_d) \cdot (\Gamma - \rho^-) & > & \Gamma - \rho^- - (\Delta - \rho^-) \cdot p_d \\ \\ \Leftrightarrow & - p_d \cdot (\Gamma - \rho^-) & > & -(\Delta - \rho^-) \cdot p_d \\ \\ \Leftrightarrow & \Gamma & < & \Delta, \end{array}$$

which is true by (2).

Proposition 1 (ii) is proven by comparing (18) and (32) subject to (16). This leads to

$$\begin{array}{lrcl} & {p}_f^* & > & \tilde{p}_f^* \\ \\ \Leftrightarrow & \frac{K}{p_r \cdot (R^+ + R^-)} & >& \frac{K}{(1 - p_d) \cdot p_r \cdot (R^+ + R^-) + p_d \cdot (1- z) \cdot R^+} \\ \\ \Leftrightarrow & p_r \cdot (R^+ + R^-) & <& (1 - p_d) \cdot p_r \cdot (R^+ + R^-) + p_d \cdot (1 - z) \cdot R^+ \\ \\ \Leftrightarrow & p_d \cdot p_r \cdot (R^+ + R^-) & <& p_d \cdot (1 - z) \cdot R^+ \\ \\ \Leftrightarrow & p_r & <& \frac{(1 - z) \cdot R^+}{R^+ + R^-} \end{array}$$

where due to the general assumption of $z \in (0,1)$, we have $\frac{(1 - z) \cdot R^+}{R^+ + R^-} \in (0,1)$. The opposite case of $p_f^* < \tilde{p}_f^*$, consequently, holds if $p_r > \frac{(1 - z) \cdot R^+}{R^+ + R^-}$.

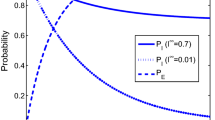
Discussion of strategy tuples from Fig. 1 in the three-person inspection game with endogenous whistle-blowing

As each player aims at maximising its own pay-off according to Nash and since we intend to solve the optimisation problem in accordance with an inspection game approach, it is necessary to figure out, if there is a Nash equilibrium in pure strategies in the first place. We successively verify whether or not one of the strategy tuples defined in Fig. 1 results in the highest pay-off for each player subject to the quantities given in Table 2. In particular, we investigate for each player’s possible strategy if she has an incentive to change her strategy taking as given the choices of the other players. Recall, that $v^g \equiv v + b_b$.

1.1 Strategy tuple ${{s}}_{{1}} =$ (fraud/high level/hide):

• The manager prefers no fraud as in $s_5$ to fraud as in $s_1$, since ${\mathcal {A}}^M < {\mathcal {C}}^M$ by (13).

• The auditor prefers high level as in $s_1$ to low level as in $s_3$, since ${\mathcal {A}}^A > {\mathcal {B}}^A$ by (15).

$\Rightarrow s_1$ cannot be a Nash equilibrium in pure strategies irrespective of the strategy choice of the employee.

1.2 Strategy tuple ${{s}}_{{2}} =$ (fraud/high level/disclose):

• There is no alternative for the manager. He, therefore, chooses fraud as in $s_2$.

• The auditor prefers high level as in $s_2$ to low level as in $s_4$, i.e. $s_2 \succ s_4$, and vice versa if

  $$\begin{aligned} s_2 \begin{Bmatrix} \succ \\ \prec \end{Bmatrix} s_4 \quad \Leftrightarrow \quad {\mathcal {A}}^A_d \begin{Bmatrix}> \\< \end{Bmatrix} {\mathcal {B}}^A_d \quad \Leftrightarrow \quad z \begin{Bmatrix} < \\ > \end{Bmatrix} 1 - \frac{K}{R^+}, \end{aligned}$$

  (54)

  where, due to (15), the bound on z from (54) is less than one.

• The employee prefers disclose as in $s_2$ to hide as in $s_1$, i.e. $s_2 \succ s_1$, and vice versa, if

  $$\begin{aligned} s_2 \begin{Bmatrix} \succ \\ \prec \end{Bmatrix} s_1 \quad \Leftrightarrow \quad {\mathcal {A}}^E_d \begin{Bmatrix}> \\< \end{Bmatrix} {\mathcal {A}}^E \quad \Leftrightarrow \quad (1 - \mu ) \cdot b_b + \mu \cdot b_\mu \begin{Bmatrix} > \\ < \end{Bmatrix} \mu \cdot (v + c_\mu ) + (1 - p_r) \cdot m + c_s \end{aligned}$$

  (55)

  and we conclude

Lemma 1

Suppose that the manager, auditor and employee simultaneously choose one from their respective two strategies. Then, there exists a Nash equilibrium in the pure strategies (fraud/high level/disclose) if and only if

$$\begin{aligned} z&< 1 - \frac{K}{R^+}, \\ (1 - \mu ) \cdot b_b + \mu \cdot b_\mu&> \mu \cdot (v + c_\mu ) + (1 - p_r) \cdot m + c_s. \end{aligned}$$

Given (30), (fraud/high level/disclose) is not a Nash equilibrium in pure strategies if either

$$\begin{aligned} z \in \left( 1 - \frac{K}{R^+}, \ \min \left\{ 1, \ 1 + \frac{(1 - p_d) \cdot p_r \cdot (R^+ + R^-) - K}{p_d \cdot R^+} \right\} \right) \end{aligned}$$

(56)

or

$$\begin{aligned} (1 - \mu ) \cdot b_b + \mu \cdot b_\mu < \mu \cdot (v + c_\mu ) + (1 - p_r) \cdot m + c_s \end{aligned}$$

or both.

1.3 Strategy tuple ${{s}}_{{3}} =$ (fraud/low level/hide):

• The manager prefers fraud as in $s_3$ to no fraud as in $s_6$, since ${\mathcal {B}}^M > {\mathcal {D}}^M$ by (14).

• The auditor prefers high level as in $s_1$ to low level as in $s_2$, since ${\mathcal {A}}^A > {\mathcal {B}}^A$ by (15).

$\Rightarrow s_3$ cannot be a Nash equilibrium in pure strategies irrespective of the strategy choice of the employee.

1.4 Strategy tuple ${{s}}_{{4}} =$ (fraud/low level/disclose):

• There is no alternative for the manager. He, therefore, chooses fraud as in $s_4$.

• The considerations of the auditor read along the line of the strategy tuple $s_2$, see (54).

• The employee prefers disclose as in $s_4$ to hide as in $s_3$, i.e. $s_4 \succ s_3$, and vice versa, if

  $$\begin{aligned} s_4 \begin{Bmatrix} \succ \\ \prec \end{Bmatrix} s_3 \quad \Leftrightarrow \quad {\mathcal {B}}^E_d \begin{Bmatrix}> \\< \end{Bmatrix} {\mathcal {B}}^E \quad \Leftrightarrow \quad (1 - \mu ) \cdot b_b + \mu \cdot b_\mu \begin{Bmatrix} > \\ < \end{Bmatrix} \mu \cdot (v + c_\mu ) + m + c_s \end{aligned}$$

  (57)

  and we conclude

Lemma 2

Suppose that the manager, auditor and employee simultaneously choose one from their respective two strategies. Then, there exists a Nash equilibrium in the pure strategies (fraud/low level/disclose) if and only if

$$\begin{aligned} z&> 1 - \frac{K}{R^+}, \\ (1 - \mu ) \cdot b_b + \mu \cdot b_\mu&> \mu \cdot (v + c_\mu ) + m + c_s .\end{aligned}$$

(fraud/low level/disclose) is not a Nash equilibrium in pure strategies if either

$$\begin{aligned} z < 1 - \frac{K}{R^+} \end{aligned}$$

or

$$\begin{aligned} (1 - \mu ) \cdot b_b + \mu \cdot b_\mu < \mu \cdot (v + c_\mu ) + m + c_s \end{aligned}$$

or both.

1.5 Strategy tuple ${s}_{{5}} =$ (no fraud/high level/hide):

• The manager prefers no fraud as in $s_5$ to fraud as in $s_1$, since ${\mathcal {A}}^M < {\mathcal {C}}^M$ by (13).

• The auditor prefers low level as in $s_6$ to high level as in $s_5$, since ${\mathcal {C}}^A < {\mathcal {D}}^A$ by (16).

• The employee has no alternative and chooses hide as in $s_5$.

$\Rightarrow s_5$ cannot be a Nash equilibrium in pure strategies.

1.6 Strategy tuple ${{s}}_{{6}} =$ (no fraud/low level/hide):

• The manager prefers fraud as in $s_3$ to no fraud as in $s_6$, since ${\mathcal {B}}^M > {\mathcal {D}}^M$ by (14).

• The auditor prefers low level as in $s_6$ to high level as in $s_5$, since ${\mathcal {C}}^A < {\mathcal {D}}^A$ by (16).

• The employee has no alternative and chooses hide as in $s_6$.

$\Rightarrow s_6$ cannot be a Nash equilibrium in pure strategies.

Discussion of Nash equilibrium probabilities in the three-person inspection game with endogenous whistle-blowing

Recall $v^g \equiv v + b_b$. Notice from (48) that $\hat{p}_h^* \in (0,1)$ requires the probable appropriation of the board $b_b$ and the possible national protection of the whistle-blower $b_\mu$ to be such that

$$\begin{aligned} \mu \cdot (v + c_\mu ) + (1 - p_r) \cdot m + c_s< (1 - \mu ) \cdot b_b + \mu \cdot b_\mu < \mu \cdot (v + c_\mu ) + m + c_s, \end{aligned}$$

(58)

according to which $s_4$ will not arise as a Nash equilibrium in pure strategies, see Lemma 2. In order to avoid that $s_2$ may represent the Nash equilibrium, see Lemma 1, we consider (56) to hold in terms of $z > 1 - \frac{K}{R^+}$.

To check for $\hat{p}_d^*$ from (49) to attain a value between zero and one, be aware that its denominator is negative, see

$$\begin{array}{c} Y < 0\\ \Leftrightarrow \\ p_r \cdot \underbrace{(\Delta - \rho ^-)}_{> 0 \text { by (1)}} \cdot \underbrace{(\mu \cdot (v^g - b_\mu ^n) + c_s - b_b)}_{< 0 \text { by (58)}} - \kappa \cdot \underbrace{(\mu \cdot (v^g - b_\mu ^n) + m + c_s - b_b)}_{> 0 \text { by (58)}} < 0.\end{array}$$

(59)

The numerator, thus, must be negative as well so that $\hat{p}_d^* > 0$. To guarantee this, recall that the manager’s costs in case of her fraud being revealed ($\Delta$) are assumed to exceed her long-term reputation loss if there is only the internal audit and it does not reveal the fraud ($\rho ^-$), see (1). A sufficient condition for a negative numerator is, then, given if $\Delta$ is not too high in terms of

$$\begin{aligned} X< 0 \quad \Leftrightarrow \quad \Delta < \ \rho ^- + \underbrace{\frac{m}{\mu \cdot (v^g - b_\mu ^n) + m + c_s - b_b}}_{>1 \text { due to (58)}} \cdot \underbrace{(\Gamma - \rho ^-)}_{> 0 \text { by (14)}} \equiv \bar{\Delta }. \end{aligned}$$

(60)

For $\hat{p}_d^* < 1$ to hold, we find that Z from (40) must be positive, i.e.

$$\begin{aligned} Z> 0 \quad \Leftrightarrow \quad \underbrace{\Gamma - \Delta }_{< 0 \text { by (2)}} < \underbrace{\frac{\kappa \cdot (\mu \cdot (v^g - b_\mu ^n) + m + c_s - b_b)}{p_r \cdot m}}_{> 0 \text { by (58)}}. \end{aligned}$$

(61)

Since the left-hand side is negative while the right-hand side is positive, $Z > 0$ applies and $\hat{p}_d^* \in (0,1)$ follows from (60).

Closer inspection of (50) shows that the numerator of $\hat{p}_f^*$ is negative because of (16) and $Z > 0$. Hence, in order for $\hat{p}_f^* > 0$ to hold, the denominator must be negative as well. Since we generally have

$$\begin{aligned} \underbrace{((1-z) \cdot R^+ - K)}_{< 0 \text { since } z> 1 - \frac{K}{R^+} \text { by (56)}} \cdot \underbrace{X}_{< 0 \text { by (60)}} - \ \ \underbrace{(R^+ + R^-)}_{> 0 \text { by (15)}} \cdot \underbrace{Z}_{> 0 \text { by (61)}} \gtrless 0, \end{aligned}$$

it turns out that a sufficient condition for a negative denominator in (50) is

$$\begin{aligned} \frac{X}{Z} > \frac{R^+ + R^-}{(1 - z) \cdot R^+ - K}. \end{aligned}$$

(62)

Moreover, $\hat{p}_f^* < 1$ requires that

$$\begin{aligned} \frac{X}{Z} > \frac{p_r \cdot (R^+ + R^-) - K}{p_r \left( (1 - z) \cdot R^+ - K \right) .} \end{aligned}$$

(63)

As the lower bound on $X/Z$ from (63) is less negative than that in (62), we obtain $\hat{p}_f^* \in (0,1)$ subject to (63), which is equivalent to

$$\begin{aligned}\Delta > \rho^-& + \frac{m}{\mu \cdot (v^g - b_{\mu} ^n) + m + c_s - b_b} \cdot (\Gamma - \rho ^-) \\ & + \frac{p_r \cdot (R^+ + R^-) - K}{p_r \cdot ((1 - z) \cdot R^+ -K)} \cdot \left(\kappa - \frac{p_r \cdot m \cdot (\Gamma - \Delta)}{\mu \cdot (v^g - b_{\mu} ^n) + m + c_s - b_b}\right) \equiv \underline{\Delta}.\end{aligned}$$

(64)

Notice that $\underline{\Delta}$ from (64) is smaller than $\bar{\Delta }$ from (60) as the third addend of the former is negative. It is, however, unclear whether or not $\underline{\Delta}$ is in line with (1) via $\underline{\Delta} > \rho ^-$. A feasible domain for $\Delta$ subject to (1) is, therefore, given by $\Delta\,\in\,\left (\max\{\rho ^-, \underline{\Delta}\}, \bar{\Delta }\right)$.

Comparative static analysis of (48), (49) and (50)

Recall $v^g \equiv v + b_b$ and $b_\mu ^n \equiv b_\mu - c_\mu$. Differentiating (48) with respect to the relevant parameters while taking account of (58) gives

$$\begin{aligned} \frac{\partial \hat{p}_h^*}{\partial m}&= - \frac{\mu \cdot (v^g - b_\mu ^n) + c_s - b_b}{p_r \cdot m^2}> 0, \\ \frac{\partial \hat{p}_h^*}{\partial v}&= - \frac{\partial \hat{p}_h^*}{\partial b_\mu ^n} = \frac{\mu }{p_r \cdot m}> 0, \\ \frac{\partial \hat{p}_h^*}{\partial b_b}&= - \frac{1 - \mu }{p_r \cdot m}< 0, \\ \frac{\partial \hat{p}_h^*}{\partial c_s}&= \frac{1}{p_r \cdot m}> 0, \\ \frac{\partial \hat{p}_h^*}{\partial p_r}&= - \frac{\mu \cdot (v^g - b_\mu ^n) + m + c_s - b_b}{p_r^2 \cdot m}< 0, \\ \frac{\partial \hat{p}_h^*}{\partial \mu }&= \frac{v^g - b_\mu ^n}{p_r \cdot m} \begin{Bmatrix}> \\< \end{Bmatrix} 0 \ \text { if } \ v^g + c_\mu \begin{Bmatrix} > \\ < \end{Bmatrix} b_\mu . \end{aligned}$$

Differentiating (49) with respect to the relevant parameters subject to (1), (2), (14), (58), $X$ from (38) with $X < 0$, see (60), $Y$ from (39) with $Y < 0$, see (59), leads to

$$\begin{aligned} \frac{\partial \hat{p}_d^*}{\partial \Gamma }&= - \frac{p_r \cdot m}{Y}> 0, \\ \frac{\partial \hat{p}_d^*}{\partial p_r}&= - \frac{X \cdot \kappa \cdot (\mu \cdot (v^g - b_\mu ^n) + m + c_s - b_b)}{Y^2}> 0, \\ \frac{\partial \hat{p}_d^*}{\partial \Delta }&= \frac{p_r \cdot \left( m \cdot (\Gamma - \rho ^-) \cdot p_r \cdot (\mu \cdot (v^g - b_\mu ^n) + c_s - b_b) - \kappa \cdot (\mu \cdot (v^g - b_\mu ^n) + m + c_s - b_b) \right) }{Y^2}< 0,\\ \frac{\partial \hat{p}_d^*}{\partial \rho ^-}&= \frac{p_r \cdot (\mu \cdot (v^g - b_\mu ^n) + c_s - b_b) \cdot \left( m \cdot p_r \cdot (\Delta - \Gamma ) + \kappa \cdot (\mu \cdot (v^g - b_\mu ^n) + m + c_s - b_b)\right) }{Y^2}< 0,\\ \frac{\partial \hat{p}_d^*}{\partial \kappa }&= \frac{p_r \cdot X \cdot (\mu \cdot (v^g - b_\mu ^n) + m + c_s - b_b)}{Y^2}< 0, \\ \frac{\partial \hat{p}_d^*}{\partial v}&= - \frac{\partial \hat{p}_d^*}{\partial b_\mu ^n} = - \frac{p_r \cdot m \cdot \mu \cdot \left( p_r \cdot (\Delta - \rho ^-) \cdot ( \Delta - \Gamma ) + \kappa \cdot (\Gamma - \rho ^-) \right) }{Y^2}< 0, \\ \frac{\partial \hat{p}_d^*}{\partial b_b}&= \frac{p_r \cdot (1 - \mu ) \cdot m \cdot \left( p_r \cdot (\Delta - \rho ^-)\cdot (\Delta - \Gamma ) +\kappa \cdot (\Gamma - \rho ^-) \right) }{Y^2}> 0, \\ \frac{\partial \hat{p}_d^*}{\partial c_s}&= - \frac{p_r \cdot m \cdot \left( p_r \cdot (\Delta - \rho ^-) \cdot (\Delta - \Gamma ) + \kappa \cdot (\Gamma - \rho ^-) \right) }{Y^2}< 0,\\ \frac{\partial \hat{p}_d^*}{\partial \mu }&= - \frac{p_r \cdot m \cdot (v^g - b_\mu ^n) \cdot \left( p_r \cdot (\Delta - \rho ^-) \cdot (\Delta - \Gamma ) + \kappa \cdot (\Gamma - \rho ^-) \right) }{Y^2} \begin{Bmatrix}< \\> \end{Bmatrix} 0 \ \text { if } \ v^g + c_\mu \begin{Bmatrix} > \\< \end{Bmatrix} b_\mu, \\ \frac{\partial \hat{p}_d^*}{\partial m}&= \frac{p_r \cdot \left( (\Delta - \Gamma ) \cdot Y + X \cdot \kappa \right) }{Y^2} < 0. \\ \end{aligned}$$

Let $W \equiv \left( (1 - z) \cdot R^+ - K\right) \cdot X - (R^+ + R^-) \cdot Z$ with $W < 0$ because of (62). Differentiating (50) with respect to the relevant parameters while exploiting (1), (2), (15), (16), $z > 1 - \frac{K}{R^+}$ from (56), (58), $X$ from (38) with $X < 0$, see (60), $Z$ from (40) with $Z > 0$, see (61), yields

$$\begin{aligned} \frac{\partial \hat{p}_f^*}{\partial K}&= \frac{- Z \cdot W - K \cdot Z \cdot X}{p_r \cdot W^2}> 0,\\ \frac{\partial \hat{p}_f^*}{\partial z}&= - \frac{K \cdot Z \cdot R^+ \cdot X}{p_r \cdot W^2}> 0, \\ \frac{\partial \hat{p}_f^*}{\partial R^+}&= \frac{K \cdot Z \cdot \left( (1 - z) \cdot X - Z \right) }{p_r \cdot W^2}< 0,\\ \frac{\partial \hat{p}_f^*}{\partial R^-}&= - \frac{K \cdot Z^2}{p_r \cdot W^2}< 0,\\ \frac{\partial \hat{p}_f^*}{\partial p_r}&= \frac{K \cdot m \cdot (\Gamma - \Delta ) \cdot ((1 - z) \cdot R^+ - K) \cdot X}{p_r \cdot W^2}< 0, \\ \frac{\partial \hat{p}_f^*}{\partial \Gamma }&= - \frac{K \cdot m \cdot ((1 - z) \cdot R^+ - K) \cdot (Z - p_r \cdot X)}{p_r \cdot W^2}> 0,\\ \frac{\partial \hat{p}_f^*}{\partial \Delta }&= - \frac{K \cdot ((1 - z) \cdot R^+ - K) \cdot \left( p_r \cdot m \cdot X - Z \cdot (\mu \cdot (v^g - b_\mu ^n) + m + c_s - b_b) \right) }{p_r \cdot W^2}< 0, \\ \frac{\partial \hat{p}_f^*}{\partial \rho ^-}&= - \frac{K \cdot Z \cdot ((1 - z) \cdot R^+ - K) \cdot (\mu \cdot (v^g - b_\mu ^n) + c_s - b_b)}{p_r \cdot W^2}< 0,\\ \frac{\partial \hat{p}_f^*}{\partial \kappa }&= - \frac{K \cdot ((1 - z) \cdot R^+ - K) \cdot ( \mu \cdot (v^g - b_\mu ^n) + m + c_s - b_b) \cdot X}{p_r \cdot W^2}< 0, \\ \frac{\partial \hat{p}_f^*}{\partial v}&= - \frac{\partial \hat{p}_f^*}{\partial b_\mu ^n} = - \frac{K \cdot \mu \cdot ((1 - z) \cdot R^+ - K) \cdot (\kappa \cdot X - Z \cdot (\Delta - \rho ^-))}{p_r \cdot W^2}< 0,\\ \frac{\partial \hat{p}_f^*}{\partial c_s}&= - \frac{K \cdot ((1 - z) \cdot R^+ - K) \cdot (\kappa \cdot X - Z \cdot (\Delta - \rho ^-))}{p_r \cdot W^2}< 0, \\ \frac{\partial \hat{p}_f^*}{\partial b_b}&= \frac{K \cdot (1 - \mu ) \cdot \left( (1 - z) \cdot R^+ - K \right) \cdot \left( \kappa \cdot X - Z \cdot (\Delta - \rho ^-) \right) }{p_r \cdot W^2}> 0, \\ \frac{\partial \hat{p}_f^*}{\partial \mu }&= - \frac{K \cdot (v^g - b_\mu ^n) \cdot ((1 - z) \cdot R^+ - K) \cdot (\kappa \cdot X - Z \cdot (\Delta - \rho ^-))}{p_r \cdot W^2} \begin{Bmatrix}< \\> \end{Bmatrix} 0 \ \text { if } \ v^g + c_\mu \begin{Bmatrix} > \\< \end{Bmatrix} b_\mu, \\ \frac{\partial \hat{p}_f^*}{\partial m}&= - \frac{K \cdot ((1 - z) \cdot R^+ - K) \cdot \left( Z \cdot (\Gamma - \Delta ) + (\kappa + p_r \cdot (\Delta - \Gamma )) \cdot X \right) }{p_r \cdot W^2} < 0. \\ \end{aligned}$$

Proof of proposition 2

To prove proposition 2 (i), compare (17) and (48). Taking account of (1) and (58) results in

$$\begin{array}{lrcl} \hat{p}_h^* < p_h^* \\ \\ \Leftrightarrow \frac{\mu \cdot v + m + c - (1 - \mu) \cdot b_b - \mu \cdot b_s}{p_r \cdot m} < \frac{\Gamma - \rho^-}{p_r \cdot (\Delta - \rho^-)} \\ \\ \Leftrightarrow \Delta < \rho^- + \frac{m}{\mu \cdot v + m + c - (1 - \mu) \cdot b_b - \mu \cdot b_s} \cdot (\Gamma - \rho^-), \end{array}$$

(65)

which is true by (60).

We prove proposition 2 (ii) by comparing (18) and (50) conditional upon (15), the assumption of $z > 1 - \frac{K}{R^+}$ from (56), $X < 0$ from (60) and (62). We obtain

$$\begin{array}{lrcl} & \hat{p}_f^* & > & p_f^* \\ \\ \Leftrightarrow & \frac{- K \cdot Z}{p_r \cdot \left[ ((1 - z) \cdot R^+ - K) \cdot X - (R^+ + R^-) \cdot Z \right]} & > & \frac{K}{p_r \cdot (R^+ + R^-)} \\ \\ \Leftrightarrow & - Z \cdot (R^+ + R^-) & < & ((1 - z) \cdot R^+ - K) \cdot X - (R^+ + R^-) \cdot Z \\ \\ \Leftrightarrow & 0 & < & ((1 - z) \cdot R^+ - K) \cdot X, \end{array}$$

which is true.