Bifurcations and dynamical analysis of Coriolis-stabilized spherical lagging pendula

Abstract

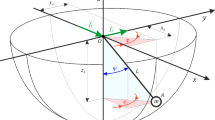
The dynamics of a spherical pendulum with a horizontally rotating support, exhibiting regimes of intriguing lagging behaviour in the presence of aerodynamic drag, is analysed in the present study. The dynamic equilibria of the pendulum are derived through both numerical and analytical means, with excellent experiment agreement across key system parameters. System attractors indicate the critical role played by drag in convergence towards the various equilibria. The stability of the various equilibria is also investigated. Interestingly, one of the folded states is found to be dynamically stabilized by the Coriolis force, with the resulting stability bound by a saddle-node bifurcation from below and a Hamiltonian Hopf bifurcation from above; multiple limit cycles are discovered past the Hopf point. Lastly, a theoretical analogy between the pendulum system and the triangular $\text {L}_4$/$\text {L}_5$ Lagrange points in celestial mechanics is identified. The presented results are of relevance to crane systems and the mechanical modelling of cable or truss-supported structures.

Appendices

Appendix A: Stability threshold for $\theta _{e_1}$

A derivation similar to Ghigliazza and Holmes [25] is followed. At the saddle-node bifurcation, $q\equiv {b/\ell }=-\sin ^3{\theta }$. The stability threshold for $\theta _{e_1}$ occurs when the eigenvalues in Eq. (8) are degenerate, disregarding sign; this occurs when $p\equiv {\alpha -\sqrt{\beta }}=0$, with solution $q=(4/5)^{3/2}\equiv \xi$. Recall that $\lim _{\Omega \rightarrow \infty } \theta _{e_1} = -\arcsin {b/\ell }=\zeta$, and therefore $(b/\ell )^{1/3}\le {-\sin {\theta }}<b/\ell$. When $q>\xi$, $p<0\,\forall \, \theta \in \left( -\arcsin {b/\ell },-\arcsin {(b/\ell )^{1/3}}\right)$, as can be deduced by noting that $p'(\theta )<0$ within the same domain. This yields complex eigenvalues and indicates instability. On the other hand, $q<\xi$ yields imaginary eigenvalues and indicates stability.

Fig. 11

Potential landscapes for $b=15\hbox { cm}$, $\ell =47\hbox { cm}$, $g=9.78\hbox { m/s}^{2}$, $\Omega _{\text {SN}}=7.31\hbox { rad/s}$. a$\Omega =5\hbox { rad/s}<\Omega _{\text {SN}}$b$\Omega =15\hbox { rad/s}>\Omega _{\text {SN}}$

Appendix B: Potential stability analysis

The stability of the various dynamic equilibria can alternatively be analysed by examining the dimensionless potential of the system, given by

$$\begin{aligned} V(\theta ,\phi )&=A^{-2}B(1-\cos {\theta })\nonumber \\&\quad -\frac{1}{2}\left( 1+A^{-2} \sin ^2{\theta }+2A^{-1}\sin {\theta }\cos {\phi }\right) , \end{aligned}$$

(B1)

where A and B are defined in Eq. (3), and negligible drag has been assumed. The potential landscapes of the system are shown in Fig. 11, plotted for $\Omega <\Omega _{\text {SN}}$ and $\Omega >\Omega _{\text {SN}}$, respectively. The three dynamic equilibria are, if present, aligned on the $\phi =0$ plane. When $\Omega <\Omega _{\text {SN}}$, it is clear that there exists no equilibria for $\theta \in (-\pi /2,0)$. On the other hand, when $\Omega >\Omega _{\text {SN}}$, we observe the formation of the two discrete equilibria $e_1$ and $e_2$. In particular, note that $e_0$ is always within a potential well, consistent with its apparent stability observed in experiments. Similarly, $e_2$ is located on a potential saddle, corroborating its instability in experiments. However, $e_1$ is located atop a potential hill, contradictory to its stability in experiments. This suggests that the stability of $e_1$ cannot be attributed to conservative forces.

Appendix C: Drag characterization

Figure 12 illustrates the stationary ($\Omega =0$) decay envelope of the system perturbed from equilibrium in the x–z plane. Numerical nonlinear regression of Eq. (5) against the experimentally measured decay envelope allows the determination of appropriate values for the drag coefficients $\mu _1$ and $\mu _2$, which are then consistently used throughout this paper.

Fig. 12

Stationary decay envelope for $m=19\hbox { g}$, $\ell =4\hbox { cm}$, $\mu _1=\kappa \cdot 1.52\hbox { mg/s}$ and $\mu _2=\kappa \cdot 4.56\hbox { mg/m}$, where $\kappa =200$. Experiment error bars are too small to be visible

Fig. 13

Potential landscape of a two-body celestial system in the rotating frame. The $\text {L}_1$–$\text {L}_3$ Lagrange points are located on potential saddles, while the $\text {L}_4$/$\text {L}_5$ Lagrange points are located on Coriolis-stabilized hills

Appendix D: $\text {L}_4$/$\text {L}_5$ Lagrange points

Figure 13 illustrates the potential landscape due to gravitational and centrifugal forces in the rotating frame of two masses orbiting about their barycenter; the $\text {L}_1$–$\text {L}_5$ Lagrange points are the five equilibrium positions.

A comparison can be made between the linearized equations of motion of the lagging pendulum, as expressed in Eq. (7), and that of objects in the neighbourhood of the $\text {L}_4$/$\text {L}_5$ Lagrange points:

$$\begin{aligned} \begin{pmatrix} \dot{x} \\ \ddot{x} \\ \dot{y} \\ \ddot{y} \end{pmatrix}&= \begin{bmatrix} 0&1&0&0\\ \frac{3}{4}\Omega&0&\eta \Omega ^{2}&{2\Omega } \\ 0&0&0&1 \\ \eta \Omega ^{2}&{-2\Omega }&\frac{9}{4}\eta ^{2}&0 \end{bmatrix}\times \begin{pmatrix} x \\ \dot{x} \\ y \\ \dot{y} \end{pmatrix};\nonumber \\ \eta&=\frac{3\sqrt{3}}{4}\cdot \frac{m_1-m_2}{m_1+m_2}, \end{aligned}$$

(D1)

where the terms $\mathbf {J}_{24}$ and $\mathbf {J}_{42}$ in Eq. (7), as well as the $\pm {\,}{2\Omega }$ terms in Eq. (D1), are the Coriolis terms. It is immediately apparent that the two systems share fundamental similarities. Indeed, dynamics in the vicinity of the $\text {L}_4$/$\text {L}_5$ Lagrange points are Coriolis-stabilized and also undergo a Hamiltonian Hopf bifurcation when the mass ratio parameter exceeds $m_2/m_1>2/(25+3\sqrt{69})$ [34, 35].