Comprehensive theoretical digging performance analysis for hydraulic excavator using convex polytope method

Abstract

This paper proposes a method for analyzing comprehensive theoretical digging performance of an excavator based on the convex polytope. The convex polytope is generated by using Newton–Euler’s equations to establish dynamic relationships between the digging capability of the bucket and the driving capability of hydraulic cylinders with the consideration of the excavator tipping and slipping constraints, and is used to identify the excavator’s output capability for digging forces and moments in the bucket force space. A set of indices for theoretically quantifying the digging capability, digging efficiency, as well as the matchability between the manipulator mechanism and the driving capability of hydraulic cylinders are proposed based on the polytope, and they are used to assess the digging trajectory characteristics and the dynamic digging performance of the entire excavator workspace. Two case studies for the excavator’s digging performance assessment and optimal digging trajectory generation are conducted to test and validate this method. The proposed method contributes to comprehensively and deeply understand the design principles of an excavator, and shows the promising application prospect for guiding the design and development of new excavators.

Appendices

Appendix A: Forward kinematics of the driving mechanisms of the manipulator

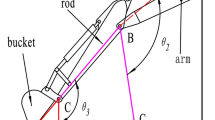
Figure 10 shows the schematics of the driving mechanisms of the manipulator. Considering the boom cylinder driving loop $\Delta\mathit{AFC}$, one has

$$ \theta _{2} = \cos^{ - 1} ( \alpha _{1} ) - \angle \mathit{CAB} - \angle X_{1}\mathit{AF}, \quad \alpha _{1} = \frac{\overline{\mathit{AF}}^{2} + \overline{\mathit{AC}}^{2} - r_{2}^{2}}{2 \overline{\mathit{AF}} \cdot \overline{\mathit{AC}}}, $$

(A.1)

where $\angle X_{1}\mathit{AF}$ is the angle that $\overline{\mathit{AF}}$ makes with the $x_{1}$-axis. By the time derivative of Eq. (A.1), the joint velocity $\dot{\theta }_{2}$ is obtained:

$$ \dot{\theta }_{2} = \frac{r_{2}\dot{r}_{2}}{\overline{\mathit{AF}} \cdot \overline{\mathit{AC}} \cdot \sqrt{1 - \alpha _{1}^{2}}}. $$

(A.2)

Fig. 10

Driving mechanisms of the manipulator. (a) Boom cylinder driving loop; (b) stick cylinder driving loop; (c) bucket cylinder driving loop

By further calculating the second derivative of joint angle $\theta _{2}$ versus time, following equation can be derived:

$$ \ddot{\theta }_{2} = \frac{\dot{r}_{2}^{2} + r_{2}\ddot{r}_{2}}{ \overline{\mathit{AF}} \cdot \overline{\mathit{AC}} \cdot \sqrt{1 - \alpha _{1}^{2}}} - \frac{\alpha _{1}}{\sqrt{1 - \alpha _{1}^{2}}} \dot{\theta }_{2}^{2}. $$

(A.3)

After substituting the detailed representations of $\alpha _{1}$ and $\dot{\theta }_{2}$ into Eq. (A.3), joint acceleration $\ddot{\theta }_{2}$ can be explicitly written as the function of $r_{2}$, $\dot{r}_{2}$ and $\ddot{r}_{2}$. Equations (A.1)–(A.3) establish the forward kinematics of the boom cylinder driving mechanism.

For the stick cylinder loop $\Delta\mathit{DBH}$, joint angle $\theta _{3}$ is given by

$$ \theta _{3} = \pi - \cos^{ - 1} ( \alpha _{2} ) - \angle \mathit{GBH} - \angle \mathit{ABD}, \quad \alpha _{2} = \frac{\overline{\mathit{DB}}^{2} + \overline{\mathit{BH}}^{2} - r_{3}^{2}}{2 \overline{\mathit{DB}} \cdot \overline{\mathit{BH}}}. $$

(A.4)

The joint velocity $\dot{\theta }_{3}$ is then given by differentiating the above expression, and it is written as

$$ \dot{\theta }_{3} = - \frac{r_{3}\dot{r}_{3}}{\overline{\mathit{DB}} \cdot \overline{\mathit{BH}} \cdot \sqrt{1 - \alpha _{2}^{2}}}. $$

(A.5)

Further the time derivative of $\dot{\theta }_{3}$ is given by

$$ \ddot{\theta }_{3} = \frac{ - ( \dot{r}_{3}^{2} + r_{3}\ddot{r} _{3} )}{\overline{\mathit{DB}} \cdot \overline{\mathit{BH}} \cdot \sqrt{1 - \alpha _{2}^{2}}} + \frac{\alpha _{2}}{\sqrt{1 - \alpha _{2}^{2}}} \dot{\theta }_{3}^{2}. $$

(A.6)

Thus, giving the length of stick cylinder and the ram velocity and acceleration, forward motion relations of stick cylinder driving loop can be determined by Eqs. (A.4)–(A.6).

Bucket cylinder driving mechanism includes loop $\Delta \mathit{ENK}$ and a four-bar linkage $\Box \mathit{KNGL}$. Based on the geometry of the mechanism, it can be obtained

$$ \theta _{4} = \pi - \angle \mathit{JGL} - \angle \mathit{BGN} - \angle \mathit{KGN} - \angle \mathit{LGK}, $$

(A.7)

where

$$\begin{aligned} &\angle \mathit{KGN} = \cos^{ - 1} ( \alpha _{3} ),\qquad \alpha _{3} = \frac{\overline{\mathit{KG}}^{2} + \overline{\mathit{NG}}^{2} - \overline{\mathit{KN}}^{2}}{2 \overline{\mathit{KG}} \cdot \overline{\mathit{NG}}},\qquad \angle \mathit{LGK} = \cos^{ - 1} ( \alpha _{4} ),\\ &\alpha _{4} = \frac{\overline{\mathit{GL}} ^{2} + \overline{\mathit{KG}}^{2} - \overline{\mathit{KL}}^{2}}{2\overline{\mathit{GL}} \cdot \overline{\mathit{KG}}},\qquad \overline{\mathit{KG}} = \sqrt{\overline{\mathit{KN}}^{2} + \overline{\mathit{NG}}^{2} - 2 \overline{\mathit{KN}} \cdot \overline{\mathit{NG}} \cdot \cos ( \angle \mathit{KNG} )},\\ &\angle \mathit{KNG} = 2\pi - \angle \mathit{ENK} - \angle \mathit{ENB} - \angle \mathit{GNB}, \qquad \angle \mathit{ENK} = \cos^{ - 1} ( \alpha _{5} ),\\ &\alpha _{5} = \frac{\overline{\mathit{EN}}^{2} + \overline{\mathit{KN}}^{2} - r_{4}^{2}}{2 \overline{\mathit{EN}} \cdot \overline{\mathit{KN}}}. \end{aligned}$$

According to the derivation rules of the function of functions, one has

$$ \dot{\theta }_{4} = - \alpha _{8} \cdot \dot{r}_{4} - \alpha _{9} \cdot \dot{r}_{4}, $$

(A.8)

where

$$\begin{aligned} &\alpha _{8} = - \frac{\alpha _{7} \cdot ( \overline{\mathit{KG}}^{2} - \overline{\mathit{NG}}^{2} + \overline{\mathit{KN}}^{2} )}{2\overline{\mathit{KG}}^{2} \cdot \overline{\mathit{NG}} \cdot \sqrt{1 - \alpha _{3}^{2}}},\qquad \alpha _{7} = - \frac{\overline{\mathit{KN}} \cdot \overline{\mathit{NG}} \cdot \sin ( \angle \mathit{KNG} ) \cdot \alpha _{6}}{\overline{\mathit{KG}}}, \\ &\alpha _{6} = \frac{r_{4}}{\overline{\mathit{EN}} \cdot \overline{\mathit{KN}} \cdot \sqrt{1 - \alpha _{5}^{2}}},\qquad\alpha _{9} = - \frac{\alpha _{7} \cdot ( \overline{\mathit{KG}}^{2} - \overline{\mathit{GL}}^{2} + \overline{\mathit{KL}}^{2} )}{2\overline{\mathit{GL}} \cdot \overline{\mathit{KG}}^{2}\sqrt{1 - \alpha _{4}^{2}}}. \end{aligned}$$

Taking the derivative of Eq. (A.8) with respect to time, one obtains

$$ \ddot{\theta }_{4} = - \alpha _{10} \cdot \dot{r}_{4} - \alpha _{8} \cdot \ddot{r}_{4} - \alpha _{11} \cdot \dot{r}_{4} - \alpha _{9} \cdot \ddot{r}_{4}. $$

(A.9)

Then the various variants in Eq. (A.9) are given by

$$\begin{aligned} \alpha _{10} =& \frac{ - ( \alpha _{12} \cdot ( \overline{\mathit{KG}}^{2} - \overline{\mathit{NG}}^{2} + \overline{\mathit{KN}}^{2} ) + \alpha _{7}^{2} \cdot 2\overline{\mathit{KG}} \cdot \dot{r}_{4} )}{2 \overline{\mathit{KG}}^{2} \cdot \overline{\mathit{NG}} \cdot \sqrt{1 - \alpha _{3} ^{2}}} \\ &{}+ \frac{\alpha _{7} \cdot ( \overline{\mathit{KG}}^{2} - \overline{\mathit{NG}} ^{2} + \overline{\mathit{KN}}^{2} ) \cdot ( 2\alpha _{7} \cdot \dot{r}_{4} \cdot \sqrt{1 - \alpha _{3}^{2}} - \overline{\mathit{KG}} \cdot \frac{ ( \alpha _{3}\alpha _{15} )}{\sqrt{1 - \alpha _{3}^{2}}} )}{2 \overline{\mathit{KG}}^{3} \cdot \overline{\mathit{NG}} \cdot ( 1 - \alpha _{3} ^{2} )} \\ \alpha _{11} =& \frac{ - ( \alpha _{12} \cdot ( \overline{\mathit{KG}}^{2} - \overline{\mathit{GL}}^{2} + \overline{\mathit{KL}}^{2} ) + \alpha _{7}^{2} \cdot 2\overline{\mathit{KG}} \cdot \dot{r}_{4} )}{2 \overline{\mathit{KG}}^{2} \cdot \overline{\mathit{GL}} \cdot \sqrt{1 - \alpha _{4} ^{2}}} \\ &{}+ \frac{\alpha _{7} \cdot ( \overline{\mathit{KG}}^{2} - \overline{\mathit{GL}} ^{2} + \overline{\mathit{KL}}^{2} ) \cdot ( 2\alpha _{7} \cdot \dot{r}_{4} \cdot \sqrt{1 - \alpha _{4}^{2}} - \overline{\mathit{KG}} \cdot \frac{ ( \alpha _{4}\alpha _{16} )}{\sqrt{1 - \alpha _{4}^{2}}} )}{2 \overline{\mathit{KG}}^{3} \cdot \overline{\mathit{GL}} \cdot ( 1 - \alpha _{4} ^{2} )} \\ \alpha _{12} =& \frac{ - \overline{\mathit{KN}} \cdot \overline{\mathit{NG}} \cdot ( \alpha _{13} \cdot \sin ( \angle \mathit{KNG} ) - \alpha _{6}^{2} \cdot \dot{r}_{4} \cdot \cos ( \angle \mathit{KNG} ) ) - \alpha _{7}^{2} \cdot \dot{r}_{4}}{\overline{\mathit{KG}}} \\ \alpha _{13} =& \frac{\dot{r}_{4}}{\overline{\mathit{EN}} \cdot \overline{\mathit{KN}} \cdot \sqrt{1 - \alpha _{5}^{2}}} + \frac{\alpha _{6}\alpha _{5}\alpha _{14}}{ ( 1 - \alpha _{5}^{2} )},\qquad \alpha _{14} = - \frac{r _{4} \cdot \dot{r}_{4}}{\overline{\mathit{EN}} \cdot \overline{\mathit{KN}}} \\ \alpha _{15} =& - \alpha _{8} \cdot \sqrt{1 - \alpha _{3}^{2}} \cdot \dot{r}_{4},\qquad \alpha _{16} = - \alpha _{9} \cdot \sqrt{1 - \alpha _{4}^{2}} \cdot \dot{r}_{4}. \end{aligned}$$

Joint angle, angular velocity and acceleration of the bucket can be described by substituting above expressions of various variants into Eqs. (A.7)–(A.9), respectively.

Appendix B: Inverse kinematics of the driving mechanisms of the manipulator

Inverse kinematics equations of the driving mechanisms of the manipulator can be obtained by calculating the inverse functions of Eqs. (A.1)–(A.9). For the boom cylinder driving mechanism, the cylinder length, the ram velocity and acceleration are described in the following equations:

$$\begin{aligned} r_{2} =& \sqrt{\overline{\mathit{AF}}^{2} + \overline{\mathit{AC}}^{2} - 2 \overline{\mathit{AF}} \cdot \overline{\mathit{AC}} \cdot \alpha _{1}}, \end{aligned}$$

(B.1)

$$\begin{aligned} \dot{r}_{2} =& \frac{\overline{\mathit{AF}} \cdot \overline{\mathit{AC}} \cdot \sin ( \theta _{2} + \angle \mathit{CAB} + \angle X_{1}\mathit{AF} ) \cdot \dot{\theta }_{2}}{r_{2}}, \end{aligned}$$

(B.2)

$$\begin{aligned} \ddot{r}_{2} =& \frac{\overline{\mathit{AF}} \cdot \overline{\mathit{AC}} \cdot ( \alpha _{1} \cdot \dot{\theta }_{2}^{2} + \sin ( \theta _{2} + \angle \mathit{CAB} + \angle X_{1}\mathit{AF} ) \cdot \ddot{\theta }_{2} )}{r _{2}} - \frac{\dot{r}_{2}^{2}}{r_{2}}. \end{aligned}$$

(B.3)

Thus, Eqs. (B.1)–(B.3) establish the inverse kinematics of the boom cylinder driving mechanism.

Similarly, the corresponding inverse kinematics equations of the stick cylinder driving mechanism are written

$$\begin{aligned} r_{3} =& \sqrt{\overline{\mathit{DB}}^{2} + \overline{\mathit{BH}}^{2} - 2 \overline{\mathit{DB}} \cdot \overline{\mathit{BH}} \cdot \alpha _{2}}, \end{aligned}$$

(B.4)

$$\begin{aligned} \dot{r}_{3} =& - \frac{\overline{\mathit{DB}} \cdot \overline{\mathit{BH}} \cdot \sin ( \theta _{3} + \angle \mathit{GBH} + \angle \mathit{ABD} ) \cdot \dot{\theta }_{3}}{r_{3}}, \end{aligned}$$

(B.5)

$$\begin{aligned} \ddot{r}_{3} =& - \frac{\overline{\mathit{DB}} \cdot \overline{\mathit{BH}} \cdot ( - \alpha _{2} \cdot \dot{\theta }_{3}^{2} + \sin ( \theta _{3} + \angle \mathit{GBH} + \angle \mathit{ABD} ) \cdot \ddot{\theta }_{3} )}{r _{3}} - \frac{\dot{r}_{3}^{2}}{r_{3}}. \end{aligned}$$

(B.6)

Equations (B.4)–(B.6) can specify $r_{3}$, $\dot{r}_{3}$ and $\ddot{r}_{3}$, once the joint angle, angular velocity and acceleration are given.

Finally, for the bucket cylinder driving mechanism, the bucket cylinder length is determined by

$$ r_{4} = \sqrt{\overline{\mathit{EN}}^{2} + \overline{\mathit{KN}}^{2} - 2 \overline{\mathit{EN}} \cdot \overline{\mathit{KN}} \cdot \alpha _{5}}, $$

(B.7)

where $\alpha _{5}$ is the cosine of $\angle \mathit{ENK}$, and $\angle \mathit{ENK}$ is given by

$$\begin{aligned} &\angle \mathit{ENK} = 2\pi - \angle \mathit{KNG} - \angle \mathit{ENB} - \angle \mathit{GNB},\\ &\angle \mathit{KNG} = \cos^{ - 1} ( \beta _{1} ),\qquad \beta _{1} = \frac{ \overline{\mathit{KN}}^{2} + \overline{\mathit{NG}}^{2} - \overline{\mathit{KG}}^{2}}{2 \overline{\mathit{KN}} \cdot \overline{\mathit{NG}}}, \\ &\overline{\mathit{KG}} = \sqrt{\overline{\mathit{GL}}^{2} + \overline{\mathit{KL}}^{2} - 2 \overline{\mathit{GL}} \cdot \overline{\mathit{KL}} \cdot \cos ( \angle \mathit{GLK} )}, \qquad \angle \mathit{GLK} = \angle \mathit{NLK} + \angle \mathit{GLN}, \\ &\angle \mathit{NLK} = \cos ^{ - 1} ( \beta _{2} ),\qquad \beta _{2} = \frac{\overline{\mathit{LN}}^{2} + \overline{\mathit{KL}}^{2} - \overline{\mathit{KN}}^{2}}{2 \overline{\mathit{LN}} \cdot \overline{\mathit{KL}}},\\ &\overline{\mathit{LN}} = \sqrt{ \overline{\mathit{NG}}^{2} + \overline{\mathit{GL}}^{2} - 2\overline{\mathit{NG}} \cdot \overline{\mathit{GL}} \cdot \cos ( \angle \mathit{LGN} )}, \\ &\angle \mathit{LGN} = \angle \mathit{LGK} + \angle \mathit{KGN} = \pi - \angle \mathit{JGL} - \angle \mathit{BGN} - \theta _{4},\qquad \beta _{3} = \frac{\overline{\mathit{LN}}^{2} + \overline{\mathit{GL}} ^{2} - \overline{\mathit{NG}}^{2}}{2\overline{\mathit{LN}} \cdot \overline{\mathit{GL}}}, \\ &\angle \mathit{GLN} = \left \{ \textstyle\begin{array}{l@{\quad}l} \cos ^{ - 1} ( \beta _{3} ),&\angle \mathit{LGN} \le \pi\\ - \cos ^{ - 1} ( \beta _{3} ),& \angle \mathit{LGN} \ge \pi. \end{array}\displaystyle \right . \end{aligned}$$

Further, the ram velocity of the bucket cylinder can be calculated by

$$ \dot{r}_{4} = \frac{\overline{\mathit{EN}} \cdot \overline{\mathit{KN}} \cdot \sin ( \angle \mathit{KNG} + \angle \mathit{ENB} + \angle \mathit{GNB} ) \cdot \beta _{4}}{r _{4}}, $$

(B.8)

where

$$\begin{aligned} &\beta _{4} = \frac{\overline{\mathit{KG}} \cdot \beta _{5}}{\overline{\mathit{KN}} \cdot \overline{\mathit{NG}} \cdot \sqrt{1 - \beta _{1}^{2}}},\qquad \beta _{5} = \frac{ \overline{\mathit{GL}} \cdot \overline{\mathit{KL}} \cdot \sin ( \angle \mathit{GLK} ) \cdot \beta _{6}}{\overline{\mathit{KG}}},\qquad \beta _{6} = \beta _{7} + \beta_{8}, \\ &\beta _{7} = - \frac{\beta _{10}}{\sqrt{1 - \beta _{2}^{2}}},\qquad \beta _{9} = - \frac{\overline{\mathit{NG}} \cdot \overline{\mathit{GL}} \cdot \sin ( \angle \mathit{LGN} ) \cdot \dot{\theta }_{4}}{\overline{\mathit{LN}}}, \qquad \beta _{10} = \frac{\beta _{9}}{\overline{\mathit{KL}}} - \frac{\beta _{2} \beta _{9}}{\overline{\mathit{LN}}}, \\ &\beta _{11} = \frac{\beta _{9}}{\overline{\mathit{GL}}} - \frac{\beta _{3}\beta _{9}}{\overline{\mathit{LN}}},\qquad \beta _{8} = \left \{ \textstyle\begin{array}{ll} - \frac{\beta _{11}}{\sqrt{1 - \beta _{3}^{2}}},& \angle \mathit{LGN} \le \pi\\ \frac{\beta _{11}}{\sqrt{1 - \beta _{3}^{2}}},& \angle \mathit{LGN} \ge \pi. \end{array}\displaystyle \right . \end{aligned}$$

The ram acceleration of the bucket cylinder has the following expression:

$$ \ddot{r}_{4} = \frac{\overline{\mathit{EN}} \cdot \overline{\mathit{KN}} \cdot ( \alpha _{5} \cdot \beta _{4}^{2} + \sin ( \angle \mathit{KNG} + \angle \mathit{ENB} + \angle \mathit{GNB} ) \cdot \beta _{12} )}{r_{4}} - \frac{ \dot{r}_{4}^{2}}{r_{4}}, $$

(B.9)

where

$$\begin{aligned} &\beta _{12} = \frac{\beta _{5}^{2} + \overline{\mathit{KG}} \cdot \beta _{13}}{ \overline{\mathit{KN}} \cdot \overline{\mathit{NG}} \cdot \sqrt{1 - \beta _{1}^{2}}} + \beta _{4} \cdot \frac{\beta _{1}\beta _{14}}{ ( 1 - \beta _{1}^{2} )}, \qquad \beta _{14} = \frac{ - \overline{\mathit{KG}} \cdot \beta _{5}}{ \overline{\mathit{KN}} \cdot \overline{\mathit{NG}}}, \\ &\beta _{13} = \frac{\overline{\mathit{GL}} \cdot \overline{\mathit{KL}} \cdot ( \cos ( \angle \mathit{GLK} ) \cdot \beta _{6}^{2} + \sin ( \angle \mathit{GLK} ) \cdot \beta _{15} ) \cdot \overline{\mathit{KG}} - \overline{\mathit{GL}} \cdot \overline{\mathit{KL}} \cdot \sin ( \angle \mathit{GLK} ) \cdot \beta _{6} \cdot \beta _{5}}{\overline{\mathit{KG}}^{2}}, \\ &\beta _{15} = \beta _{16} + \beta _{17},\qquad \beta _{16} = - \frac{\beta _{2}\beta _{10}^{2}}{ ( 1 - \beta _{2}^{2} )^{3/2}} - \frac{ \beta _{18}}{\sqrt{1 - \beta _{2}^{2}}},\\ &\beta _{18} = \frac{ \beta _{19}}{\overline{\mathit{KL}}} - \frac{\overline{\mathit{LN}} \cdot ( \beta _{10}\beta _{9} + \beta _{2}\beta _{19} ) - \beta _{2}\beta _{9} ^{2}}{\overline{\mathit{LN}}^{2}}, \\ &\beta _{19} = \frac{\overline{\mathit{NG}} \cdot \overline{\mathit{GL}} \cdot ( \cos ( \angle \mathit{LGN} ) \cdot \dot{\theta }_{4}^{2} - \sin ( \angle \mathit{LGN} ) \cdot \ddot{\theta }_{4} )}{ \overline{\mathit{LN}}} - \frac{\beta _{9}^{2}}{\overline{\mathit{LN}}},\\ &\beta _{21} = \frac{\beta _{19}}{\overline{\mathit{GL}}} - \frac{\overline{\mathit{LN}} \cdot ( \beta _{11}\beta _{9} + \beta _{3}\beta _{19} ) - \beta _{3}\beta _{9}^{2}}{\overline{\mathit{LN}}^{2}}, \\ &\beta _{20} = \left \{ \textstyle\begin{array}{l@{\quad}l} - \frac{\beta _{3}\beta _{11}^{2}}{ ( 1 - \beta _{3}^{2} ) ^{3/2}} - \frac{\beta _{21}}{\sqrt{1 - \beta _{3}^{2}}},& \angle \mathit{LGN} \le \pi\\ \frac{\beta _{3}\beta _{11}^{2}}{ ( 1 - \beta _{3}^{2} )^{3/2}} + \frac{\beta _{21}}{\sqrt{1 - \beta _{3}^{2}}},& \angle \mathit{LGN} \ge \pi. \end{array}\displaystyle \right . \end{aligned}$$

Therefore, Eqs. (B.7)–(B.9) and the various variants included in them specify the inverse kinematics of the bucket cylinder driving mechanism.