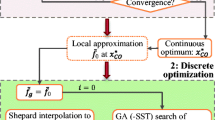
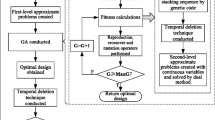
A special type of new, continuous design variables is introduced in order to find optimum solutions for discrete fiber orientations. In the buckling analysis, as two or three different fiber orientations are considered, optimum solutions can be found in an analytical way. For a greater number of discrete fiber orientations, a special optimization algorithm based on the evolution strategy is used to solve the combinatorial optimization problems dealing with the optimum design of the laminate stacking sequence. The analysis is conducted with the example of simply supported rectangular multilayered composite plates subjected to buckling and the first-ply-failure constraints. The results demonstrate the effectiveness, simplicity, and advantages of the use of continuous design variables in the discrete optimization problem.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
A. Muc, “Optimization of composite topology for doubly-curved laminated shells under buckling constraints,” Proc. IUTAM Sym. “Optimal design with advanced materials” (Ed. by P. Pedersen), Elsevier, Amsterdam, pp.474-489 (1993).
G. Verchery, “Design rules for the laminate stiffness,” Mechanics of Composite Materials, 47, 47-58 (2011),
A. Muc, “Optimal fiber orientations for simply-supported plates under compression,” Compos. Struct., 9, No. 2, 161-172 (1988).
R. Le Riche and R. T. Haftka, “Optimization of laminate stacking sequence for buckling load maximization by genetic algorithm,” AIAA J., 31, No. 5, 951-956 (1993).
S. Karakaya, and O. Soykasap, “Buckling optimization of laminated composite plates using genetic algorithm and generalized pattern search algorithm,” Structural and Multidisciplinary Optimization, 39, No. 5, 477-486 (2009).
Z. Gurdal and R. Olmedo, “In-plane response of laminates with spatially varying fiber orientations: variable stiffness concept,” AIAA J., 31, No. 4, 751–758 (1993).
A. Muc and A. Ulatowska, “Design of plates with curved fiber format,” Compos. Struct., 92, No. 7, 1728-1733 (2010).
M. Miki, Optimum Design of Fibrous Laminated Plates Subjected to Axial Compression, in: Eds. K. Kawata, S. Umekawa, and A. Kobayashi, Composites ‘86: recent advances in Japan and the United States: Proc. of the third Japan-U.S. Conf. on Composite Materials, Tokyo, Japan, 673-681 (1986).
H.Fukunaga and G. N. Vanderplaats, “Stiffness optimization of orthotropic laminated composites using lamination parameters,” AIAA J., 29, No. 4,641-648 (1991).
C. G. Diaconu, M. Sato, and H. Sekine, “Buckling characteristics and layup optimization of long laminated composite cylindrical shells subjected to combined loads using lamination parameters,” Compos. Struct., 58, No. 4, 423–433 (2002).
M. W. Bloomfield, C. G. Diaconu, and P. M. Weaver, “On feasible regions of lamination parameters for lay-up optimization of laminated composites,” Proc. Royal Soc. A: Mathematical, Physical and Engineering Sci., 465, 1123-1143 (2009).
A. Muc and W. Gurba, “Genetic algorithms and finite element analysis in optimization of composite structures.” Compos. Struct., 54. Nos. 2-3, 275-281 (2001).
A. Muc and M. Muc-Wierzgoń, “An evolution strategy in structural optimization problems for plates and shells,” Compos. Struct., 94, No. 4, 1461-1470 (2012).
Acknowledgments
The support of the NCN under the grant UMO-2013/09/B/ST8/00178 is gratefully acknowledged.
Author information
Authors and Affiliations
Corresponding author
Additional information
Russian translation published in Mekhanika Kompozitnykh Materialov, Vol. 52, No. 2, pp. 305-324, March-April, 2016.
Rights and permissions
About this article
Cite this article
Muc, A. Choice of Design Variables in the Stacking Sequence Optimization for Laminated Structures. Mech Compos Mater 52, 211–224 (2016). https://doi.org/10.1007/s11029-016-9574-3
Received:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11029-016-9574-3