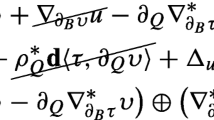Abstract
We propose a definition of symplectic 2-groupoid which includes integrations of Courant algebroids that have been recently constructed. We study in detail the simple but illustrative case of constant symplectic 2-groupoids. We show that the constant symplectic 2-groupoids are, up to equivalence, in one-to-one correspondence with a simple class of Courant algebroids that we call constant Courant algebroids. Furthermore, we find a correspondence between certain Dirac structures and Lagrangian sub-2-groupoids.
Similar content being viewed by others
Notes
Recently, Ševera and Širaň [17] described a general construction for local integration and showed that the local integrations can be glued together up to coherent homotopy.
The extension of the proof to the general case \(\overline{W}ND\), where D is a symplectic double Lie groupoid, is similar.
We note that we are using the condition \(D^\perp = D\) in place of the usual requirement that D be maximally isotropic. In most cases of interest, E has signature (n, n), and the two conditions are equivalent. However, if E does not have signature (n, n), then, according to the definition we are using, there do not exist any Dirac structures in E.
References
Coste, A., Dazord, P., Weinstein, A.: Groupoïdes symplectiques, Publications du Département de Mathématiques. Nouvelle Série. A, Vol. 2, Publ. Dép. Math. Nouvelle Sér. A, vol. 87, Univ. Claude-Bernard, Lyon, pp. i–ii, 1–62 (1987)
Courant, T., Weinstein, A.: Beyond Poisson structures, Action hamiltoniennes de groupes. Troisième théorème de Lie (Lyon, 1986), Travaux en Cours, vol. 27, Hermann, Paris, pp. 39–49 (1988)
Courant, T.J.: Dirac manifolds. Trans. Am. Math. Soc. 319(2), 631–661 (1990)
Dorfman, I.: Dirac Structures and Integrability of Nonlinear Evolution Equations, Nonlinear Science: Theory and Applications. Wiley, Chichester (1993)
Duskin, J.: Higher-dimensional torsors and the cohomology of topoi the abelian theory, Applications of sheaves. In: Proceedings of the Research Symposium on Applications of Sheaf Theory to Logic, Algebra and Analysis, University Durham, Durham (1977). Lecture Notes in Mathemathics, vol. 753, pp. 255–279. Springer, Berlin (1979)
Getzler, E.: Differential forms on stacks, 2014, Slides from minicourse at Winter School in Mathematical Physics, Les Diablerets (2014)
Goerss, Paul G., Jardine, John F.: Simplicial Homotopy Theory. Birkhäuser, Basel (2009)
Henriques, A.: Integrating \(L_\infty \)-algebras. Compos. Math. 144(4), 1017–1045 (2008)
Li-Bland, D., Ševera, P.: Integration of exact Courant algebroids. Electron. Res. Announc. Math. Sci. 19, 58–76 (2012)
Liu, Z., Weinstein, A., Xu, P.: Manin triples for Lie bialgebroids. J. Differ. Geom. 45(3), 547–574 (1997)
Mehta, R.A., Tang, X.: Symplectic Structures on the Integration of Exact Courant Algebroids. arXiv:1310.6587
Mehta, R.A., Tang, X.: From double Lie groupoids to local Lie 2-groupoids. Bull. Braz. Math. Soc. (N.S.) 42(4), 651–681 (2011)
Pantev, T., Toën, M., Vaquié, B., Vezzosi, G.: Shifted symplectic structures. Publ. Math. Inst. Hautes Études Sci. 117, 271–328 (2013)
Roytenberg, D.: On the structure of graded symplectic supermanifolds and Courant algebroids. In: Quantization, Poisson Brackets and Beyond (Manchester, 2001) Contemporary Mathematics, vol. 315, pp. 169–185. American Mathematical Society, Providence, RI (2002)
Ševera, P.: Some title containing the words “homotopy” and “symplectic”, e.g. this one, Travaux mathématiques. Fasc. XVI, Trav. Math., XVI, Univ. Luxemb., Luxembourg, pp. 121–137 (2005)
Sheng, Y., Zhu, C.: Higher Extensions of Lie Algebroids and Application to Courant Algebroids (2011). arXiv:1103.5920
Ševera, P., Širaň, M.: Integration of Differential Graded Manifolds (2015). arXiv:1506.04898
Xu, P.: Momentum maps and Morita equivalence. J. Differ. Geom. 67(2), 289–333 (2004)
Zhu, C.: Kan replacement of simplicial manifolds. Lett. Math. Phys. 90(1–3), 383–405 (2009)
Acknowledgements
We would like to thank Ezra Getzler for inspiring discussions and explanations about symplectic structures on differentiable n-stacks. We would like to thank Damien Calaque for a discussion about the relation between integration of Courant algebroids and derived symplectic geometry. The research of the second author is partially supported by NSF Grant DMS 1363250.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Mehta, R.A., Tang, X. Constant symplectic 2-groupoids. Lett Math Phys 108, 1203–1223 (2018). https://doi.org/10.1007/s11005-017-1026-z
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11005-017-1026-z