Skip to main content
SpringerLink
Log in

Contact Process in a Wedge

  • Published:
Journal of Statistical Physics Aims and scope Submit manuscript

Abstract

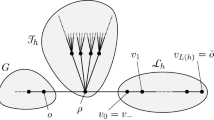
We prove that the supercritical one-dimensional contact process survives in certain wedge-like space-time regions, and that when it survives it couples with the unrestricted contact process started from its upper invariant measure. As an application we show that a type of weak coexistence is possible in the nearest-neighbor “grass-bushes-trees” successional model introduced in Durrett and Swindle (Stoch. Proc. Appl. 37:19–31, 1991).

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Alarcon, T., Byrne, H.M., Maini, P.K.: A cellular automaton for tumour growth in inhomogeneous environment. J. Theor. Biol. 225, 257–274 (2003)

    Article  MathSciNet  Google Scholar 

  2. Campanino, M., Klein, A.: Decay of two-point functions for (d+1)-dimensional percolation, Ising and Potts models with d-dimensional disorder. Commun. Math. Phys. 135, 489–497 (1991)

    ADS  Google Scholar 

  3. Chayes, J.T., Chayes, L.: Critical points and intermediate phases on wedges of ℤd. J. Phys. A, Math. Gen 19, 3033–3048 (1986)

    Article  MATH  MathSciNet  ADS  Google Scholar 

  4. Durrett, R., Swindle, G.: Are there bushes in a forest? Stoch. Proc. Appl 37, 19–31 (1991)

    Article  MATH  MathSciNet  Google Scholar 

  5. Grimmett, G.: Bond percolation on subsets of the square lattice, and the transition between one-dimensional and two-dimensional behavior. J. Phys. A, Math. Gen 16, 599–604 (1983)

    Article  MATH  MathSciNet  ADS  Google Scholar 

  6. Harris, T.E.: Contact interactions on a lattice. Ann. Probab. 2, 969–988 (1974)

    Article  MATH  Google Scholar 

  7. Liggett, T.M.: Interacting Particle Systems. Springer, Berlin (1985)

    MATH  Google Scholar 

  8. Liggett, T.M.: Stochastic Interacting Systems: Contact, Voter and Exclusion Processes. Springer, Berlin (1999)

    MATH  Google Scholar 

  9. Madras, N., Schinazi, R., Schonmann, R.: On the critical behavior of the contact process in deterministic inhomogeneous environments. Ann. Probab. 22, 1140–1159 (1994)

    Article  MATH  MathSciNet  Google Scholar 

  10. McCoy, B., Wu, T.T.: Theory of the two-dimensional Ising model with random impurities. I. Thermodynamics. Phys. Rev. B 76, 631–643 (1968)

    MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics, Syracuse University, Syracuse, NY, 13244, USA

    J. Theodore Cox

  2. Department of Mathematics and CS, University of Missouri- St. Louis, St. Louis, MO, 63121, USA

    Nevena Marić

  3. Department of Mathematics, University of Colorado-Colorado Springs, Colorado Springs, CO, 80933, USA

    Rinaldo Schinazi

Authors
  1. J. Theodore Cox
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Nevena Marić
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Rinaldo Schinazi
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Nevena Marić.

Additional information

J.T. Cox was supported in part by NSF Grant No. 0803517.

N. Marić was supported in part by NSF Grant No. 0803517.

R. Schinazi was supported in part by NSF Grant No. 0701396.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Cox, J.T., Marić, N. & Schinazi, R. Contact Process in a Wedge. J Stat Phys 139, 506–517 (2010). https://doi.org/10.1007/s10955-010-9950-7

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10955-010-9950-7

Keywords

Search

Navigation