Skip to main content
SpringerLink
Log in

A High Order Semi-Lagrangian Discontinuous Galerkin Method for the Two-Dimensional Incompressible Euler Equations and the Guiding Center Vlasov Model Without Operator Splitting

  • Published:
Journal of Scientific Computing Aims and scope Submit manuscript

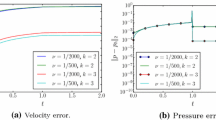
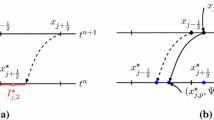
Abstract

In this paper, we generalize a high order semi-Lagrangian (SL) discontinuous Galerkin (DG) method for multi-dimensional linear transport equations without operator splitting developed in Cai et al. (J Sci Comput 73(2–3):514–542, 2017) to the 2D time dependent incompressible Euler equations in the vorticity-stream function formulation and the guiding center Vlasov model. We adopt a local DG method for Poisson’s equation of these models. For tracing the characteristics, we adopt a high order characteristics tracing mechanism based on a prediction-correction technique. The SLDG with large time-stepping size might be subject to extreme distortion of upstream cells. To avoid this problem, we propose a novel adaptive time-stepping strategy by controlling the relative deviation of areas of upstream cells.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15

Similar content being viewed by others

References

  1. Arnold, D., Brezzi, F., Cockburn, B., Marini, L.: Unified analysis of discontinuous Galerkin methods for elliptic problems. SIAM J. Numer. Anal. 39(5), 1749–1779 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  2. Bell, J., Colella, P., Glaz, H.: A second-order projection method for the incompressible Navier–Stokes equations. J. Comput. Phys. 85(2), 257–283 (1989)

    Article  MathSciNet  MATH  Google Scholar 

  3. Bonaventura, L., Ferretti, R., Rocchi, L.: A fully semi-Lagrangian discretization for the 2D incompressible Navier–Stokes equations in the vorticity-streamfunction formulation. Appl. Math. Comput. 323, 132–144 (2018)

    MathSciNet  MATH  Google Scholar 

  4. Cai, X., Guo, W., Qiu, J.-M.: A high order conservative semi-Lagrangian discontinuous Galerkin method for two-dimensional transport simulations. J. Sci. Comput. 73(2–3), 514–542 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  5. Cai, X., Guo, W., Qiu, J.-M.: A high order semi-Lagrangian discontinuous Galerkin method for Vlasov-Poisson simulations without operator splitting. J. Comput. Phys. 354, 529–551 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  6. Castillo, P., Cockburn, B., Perugia, I., Schötzau, D.: An a priori error analysis of the local discontinuous Galerkin method for elliptic problems. SIAM J. Numer. Anal. 38(5), 1676–1706 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  7. Christlieb, A., Guo, W., Morton, M., Qiu, J.-M.: A high order time splitting method based on integral deferred correction for semi-Lagrangian Vlasov simulations. J. Comput. Phys. 267, 7–27 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  8. Cockburn, B., Shu, C.-W.: The local discontinuous Galerkin method for time-dependent convection-diffusion systems. SIAM J. Numer. Anal. 35(6), 2440–2463 (1998)

    Article  MathSciNet  MATH  Google Scholar 

  9. Cockburn, B., Shu, C.-W.: Runge–Kutta discontinuous Galerkin methods for convection-dominated problems. J. Sci. Comput. 16(3), 173–261 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  10. Crouseilles, N., Mehrenberger, M., Sonnendrücker, E.: Conservative semi-Lagrangian schemes for Vlasov equations. J. Comput. Phys. 229(6), 1927–1953 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  11. Fischer, P., Mullen, J.: Filter-based stabilization of spectral element methods. Comptes Rendus de l’Académie des Sci.-Ser. I-Math. 332(3), 265–270 (2001)

    MathSciNet  MATH  Google Scholar 

  12. Frenod, E., Hirstoaga, S.A., Lutz, M., Sonnendrücker, E.: Long time behaviour of an exponential integrator for a Vlasov–Poisson system with strong magnetic field. Commun. Comput. Phys. 18(2), 263–296 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  13. Guo, W., Nair, R., Qiu, J.-M.: A conservative semi-Lagrangian discontinuous Galerkin scheme on the cubed-sphere. Mon. Weather Rev. 142(1), 457–475 (2013)

    Article  Google Scholar 

  14. Guo, Y., Xiong, T., Shi, Y.: A maximum-principle-satisfying high-order finite volume compact WENO scheme for scalar conservation laws with applications in incompressible flows. J. Sci. Comput. 65(1), 83–109 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  15. Lauritzen, P., Nair, R., Ullrich, P.: A conservative semi-Lagrangian multi-tracer transport scheme (CSLAM) on the cubed-sphere grid. J. Comput. Phys. 229(5), 1401–1424 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  16. Liu, J.-G., Shu, C.-W.: A high-order discontinuous Galerkin method for 2D incompressible flows. J. Comput. Phys. 160(2), 577–596 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  17. Liu, J.-G., Weinan, E.: Simple finite element method in vorticity formulation for incompressible flows. Math. Comput. 70(234), 579–593 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  18. Qiu, J.-M., Russo, G.: A high order multi-dimensional characteristic tracing strategy for the Vlasov–Poisson system. J. Sci. Comput. 71(1), 414–434 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  19. Qiu, J.-M., Shu, C.-W.: Conservative high order semi-Lagrangian finite difference WENO methods for advection in incompressible flow. J. Comput. Phys. 230(4), 863–889 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  20. Qiu, J.-M., Shu, C.-W.: Positivity preserving semi-Lagrangian discontinuous Galerkin formulation: theoretical analysis and application to the Vlasov–Poisson system. J. Comput. Phys. 230(23), 8386–8409 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  21. Russo, G.: A deterministic vortex method for the Navier–Stokes equations. J. Comput. Phys. 108(1), 84–94 (1993)

    Article  MathSciNet  MATH  Google Scholar 

  22. Shoucri, M.M.: A two-level implicit scheme for the numerical solution of the linearized vorticity equation. Int. J. Numer. Methods Eng. 17(10), 1525–1538 (1981)

    Article  MATH  Google Scholar 

  23. Souli, M.: Vorticity boundary conditions for Navier–Stokes equations. Comput. Methods Appl. Mech. Eng. 134(3–4), 311–323 (1996)

    Article  MathSciNet  MATH  Google Scholar 

  24. Weinan, E., Liu, J.-G.: Essentially compact schemes for unsteady viscous incompressible flows. J. Comput. Phys. 126(1), 122–138 (1996)

    Article  MathSciNet  MATH  Google Scholar 

  25. Weinan, E., Liu, J.-G.: Vorticity boundary condition and related issues for finite difference schemes. J. Comput. Phys. 124(2), 368–382 (1996)

    Article  MathSciNet  MATH  Google Scholar 

  26. Weinan, E., Shu, C.-W.: A numerical resolution study of high order essentially non-oscillatory schemes applied to incompressible flow. J. Comput. Phys. 110(1), 39–46 (1994)

    Article  MATH  Google Scholar 

  27. Xiong, T., Russo, G., Qiu, J.-M.: Conservative multi-dimensional semi-Lagrangian finite difference scheme: stability and applications to the kinetic and fluid simulations. arXiv preprint arXiv:1607.07409 (2016)

  28. Xiong, T., Russo, G., Qiu, J.-M.: High order multi-dimensional characteristics tracing for the incompressible Euler equation and the guiding-center Vlasov equation. J. Sci. Comput. to appear (2018)

  29. Xu, C.: Stabilization methods for spectral element computations of incompressible flows. J. Sci. Comput. 27(1–3), 495–505 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  30. Yang, C., Filbet, F.: Conservative and non-conservative methods based on Hermite weighted essentially non-oscillatory reconstruction for Vlasov equations. J. Comput. Phys. 279, 18–36 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  31. Zhang, X., Shu, C.-W.: On maximum-principle-satisfying high order schemes for scalar conservation laws. J. Comput. Phys. 229, 3091–3120 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  32. Zhong, X., Shu, C.-W.: A simple weighted essentially nonoscillatory limiter for Runge–Kutta discontinuous Galerkin methods. J. Comput. Phys. 232(1), 397–415 (2013)

    Article  MathSciNet  Google Scholar 

  33. Zhu, H., Qiu, J., Qiu, J.-M.: An h-adaptive RKDG method for the two-dimensional incompressible Euler equations and the Guiding Center Vlasov Model. J. Sci. Comput. 73(2–3), 1316–1337 (2017)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematical Sciences, University of Delaware, Newark, DE, 19716, USA

    Xiaofeng Cai & Jing-Mei Qiu

  2. Department of Mathematics and Statistics, Texas Tech University, Lubbock, TX, 70409, USA

    Wei Guo

Authors
  1. Xiaofeng Cai
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Wei Guo
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Jing-Mei Qiu
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Jing-Mei Qiu.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

W. Guo: Research is supported by NSF Grant NSF-DMS-1620047. J.-M. Qiu: Research of first and last author is supported by NSF Grant NSF-DMS-1522777 and 1818924, Air Force Office of Scientific Computing FA9550-18-1-0257 and University of Delaware.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Cai, X., Guo, W. & Qiu, JM. A High Order Semi-Lagrangian Discontinuous Galerkin Method for the Two-Dimensional Incompressible Euler Equations and the Guiding Center Vlasov Model Without Operator Splitting. J Sci Comput 79, 1111–1134 (2019). https://doi.org/10.1007/s10915-018-0889-1

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10915-018-0889-1

Keywords

Search

Navigation