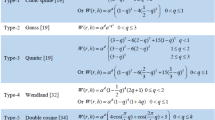Skip to main content
SpringerLink
Log in

Evaluation of Accuracy and Stability of the Classical SPH Method Under Uniaxial Compression

  • Published:
Journal of Scientific Computing Aims and scope Submit manuscript

Abstract

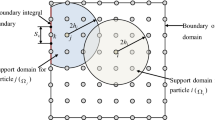
The accuracy and stability of the classical formulation of the smoothed particle hydrodynamics (SPH) method for modelling compression of elastic solids is studied to assess its suitability for predicting solid deformation. SPH has natural advantages for simulating problems involving compression of deformable solids arising from its ability to handle large deformation without re-meshing, complex free surface behaviour and tracking of multiple material interfaces. The ‘classical SPH method’, as originally proposed by Monaghan (in Ann Rev Astron 30:543–574, 1992, Rep Prog Phys 68:1703–1759, 2005), has become broadly established as a robust method in different areas, especially involving fluid flows. However, limited attention has been paid to understanding of its numerical performance for elastic deformation problems. To address this, we evaluate the classical SPH method to explore its stability, accuracy and convergence and the effect of numerical parameters on elastic solutions using a generic uniaxial stress test. Short term transient and long term uniform state SPH solutions agree well with those from the finite element method (FEM). The SPH elastic deformation solution showed good convergence with increasing particle resolution. The tensile instability stabilisation method was found to have little impact on the solution, except for higher values of the correction factor which then produce small amplitude benign artificial banded stress patterns. The use of artificial viscosity is able to eliminate the instability and improve the accuracy of the solutions. Overall, the classical SPH method appears to be robust and suitable for accurate modelling of elastic solids under compression.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16
Fig. 17
Fig. 18
Fig. 19
Fig. 20
Fig. 21
Fig. 22
Fig. 23
Fig. 24
Fig. 25
Fig. 26
Fig. 27

Similar content being viewed by others

References

  1. Monaghan, J.J.: Smoothed particle hydrodynamics. Ann. Rev. Astron. Astrophys. 30, 543–574 (1992)

    Article  Google Scholar 

  2. Monaghan, J.J.: Smoothed particle hydrodynamics. Rep. Prog. Phys. 68, 1703–1759 (2005)

    Article  MathSciNet  Google Scholar 

  3. Gingold, R.A., Monaghan, J.J.: Smoothed particle hydrodynamics—theory and application to non-spherical stars. MNRAS 181, 375–389 (1977)

    Article  MATH  Google Scholar 

  4. Lucy, L.B.: A numerical approach to the testing of the fission hypothesis. Astron. J. 82, 1013–1024 (1977)

    Article  Google Scholar 

  5. Monaghan, J.J., Price, D.J.: Variational principles for relativistic smoothed particle hydrodynamics. Mon. Not. R. Astron. Soc. 328(2), 381–392 (2001)

    Article  Google Scholar 

  6. Cleary, P.W.: Modelling confined multi-material heat and mass flows using SPH. Appl. Math. Model. 22(12), 981–993 (1998)

    Article  Google Scholar 

  7. Cleary, P.W., Ha, J., Prakash, M., Nguyen, T.: Simulation of casting complex shaped objects using SPH. In: In San Francisco, CA, United States. pp. 317–326. Minerals, Metals and Materials Society, Warrendale, PA 15086, United States (2005)

  8. Cummins, S.J., Rudman, M.J.: Truly incompressible SPH. In: In Washington, DC, USA. p. 8. ASME, Fairfield, NJ, USA (1998)

  9. Cleary, P.W., Monaghan, J.J.: Conduction modelling using smoothed particle hydrodynamics. J. Comput. Phys. 148(1), 227–264 (1999)

    Article  MathSciNet  MATH  Google Scholar 

  10. Bonet, J., Kulasegaram, S.: A simplified approach to enhance the performance of smooth particle hydrodynamics methods. Appl. Math. Comput. (N. Y.) 126(2–3), 133–155 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  11. Cleary, P., Ha, J., Alguine, V., Nguyen, T.: Flow modelling in casting processes. Appl. Math. Model. 26(2), 171–190 (2002)

    Article  MATH  Google Scholar 

  12. Cleary, P.W., Prakash, M., Ha, J., Stokes, N., Scott, C.: Smooth particle hydrodynamics: status and future potential. Prog. Comput. Fluid Dyn. 7(2–4), 70–90 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  13. Libersky, L.D., Petschek, A.G.: Smooth particle hydrodynamics with strength of materials. In: Trease, H., Crowley, W.P. (eds.) Advances in the Free-Lagrange Method. Springer, Berlin (1990)

    Google Scholar 

  14. Wingate, C.A., Fisher, H.N.: Strength Modeling in SPHC. Los Alamos National Laboratory, Report No. LA-UR-93-3942 (1993)

  15. Gray, J.P., Monaghan, J.J., Swift, R.P.: SPH elastic dynamics. Comput. Methods Appl. Mech. Eng. 190(49–50), 6641–6662 (2001)

    Article  MATH  Google Scholar 

  16. Cleary, P.W., Prakash, M., Ha, J.: Novel applications of smoothed particle hydrodynamics (SPH) in metal forming. J. Mater. Process. Technol. 177(1–3), 41–48 (2006)

    Article  Google Scholar 

  17. Das, R., Cleary, P.W.: The potential for SPH modelling of solid deformation and fracture. In: Reddy, D. (ed.) IUTAM Proceedings Book Series Volume on “Theoretical, Computational and Modelling Aspects of Inelastic Media”, pp. 287–296. Springer, Capetown (2008)

    Google Scholar 

  18. Karekal, S., Das, R., Mosse, L., Cleary, P.W.: Application of a mesh-free continuum method for simulation of rock caving processes. Int. J. Rock Mech. Min. Sci. 48(5), 703–711 (2011)

    Article  Google Scholar 

  19. Cleary, P.W., Prakash, M., Das, R., Ha, J.: Modelling of metal forging using SPH. Appl. Math. Model. 36(8), 3836–3855 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  20. Das, R., Cleary, P.W.: A mesh-free approach for fracture modelling of gravity dams under earthquake. Int. J. Fract. 179(1–2), 9–33 (2013)

    Article  Google Scholar 

  21. Das, R., Cleary, P.W.: Effect of rock shapes on brittle fracture using Smoothed Particle Hydrodynamics. Theor. Appl. Fract. Mech. 53(1), 47–60 (2010)

    Article  Google Scholar 

  22. Fagan, T., Das, R., Lemiale, V., Estrin, Y.: Modelling of equal channel angular pressing using a mesh-free method. J. Mater. Sci. 47 (11), 4514–4519 (2012)

  23. Islam, S., Ibrahim, R., Das, R., Fagan, T.: Novel approach for modelling of nanomachining using a mesh-less method. Appl. Math. Model. 36 (11), 5589–5602 (2012)

  24. Bradley, G.L., Chang, P.C., McKenna, G.B.: Rubber modeling using uniaxial test data. J. Appl. Polym. Sci. 81(4), 837–848 (2001)

    Article  Google Scholar 

  25. Liu, W.K., Jun, S., Li, S., Adee, J., Belytschko, T.: Reproducing kernel particle methods for structural dynamics. Int. J. Numer. Methods Eng. 38(10), 1655–1679 (1995)

    Article  MathSciNet  MATH  Google Scholar 

  26. Chen, J.K., Beraun, J.E., Jih, C.J.: Improvement for tensile instability in smoothed particle hydrodynamics. Comput. Mech. 23(4), 279–287 (1999)

    Article  MATH  Google Scholar 

  27. Liu, M.B., Liu, G.R.: Restoring particle consistency in smoothed particle hydrodynamics. Appl. Numer. Math. 56(1), 19–36 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  28. Bonet, J., Kulasegaram, S.: Correction and stabilization of smooth particle hydrodynamics methods with applications in metal forming simulations. Int. J. Numer. Methods Eng. 47(6), 1189–1214 (2000)

    Article  MATH  Google Scholar 

  29. Bonet, J., Kulasegaram, S.: Remarks on tension instability of Eulerian and Lagrangian corrected smooth particle hydrodynamics (CSPH) methods. Int. J. Numer. Methods Eng. 52(11), 1203–1220 (2001)

    Article  MATH  Google Scholar 

  30. Vidal, Y., Bonet, J., Huerta, A.: Stabilized updated Lagrangian corrected SPH for explicit dynamic problems. Int. J. Numer. Methods Eng. 69(13), 2687–2710 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  31. Dyka, C.T., Ingel, R.P.: An approach for tension instability in smoothed particle hydrodynamics. Comput. Struct. 57, 573–580 (1995)

    Article  MATH  Google Scholar 

  32. Dyka, C.T., Randles, P.W., Ingel, R.P.: Stress points for tension instability in SPH. Int. J. Numer. Methods Eng. 40(13), 2325–2341 (1997)

    Article  MATH  Google Scholar 

  33. Randles, P.W., Libersky, L.D.: Normalized SPH with stress points. Int. J. Numer. Methods Eng. 48(10), 1445–1462 (2000)

    Article  MATH  Google Scholar 

  34. Vignjevic, R., Campbell, J., Libersky, L.: A treatment of zero-energy modes in the smoothed particle hydrodynamics method. Comput. Methods Appl. Mech. Eng. 184(1), 67–85 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  35. Belytschko, T., Xiao, S.: Stability analysis of particle methods with corrected derivatives. Comput. Math. Appl. 43(3–5), 329–350 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  36. Xiao, S.R., Belytschko, T.: Material stability analysis of particle methods. Adv. Comput. Math. 23(1–2), 171–190 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  37. Shaw, A., Roy, D.: Stabilized SPH-based simulations of impact dynamics using acceleration-corrected artificial viscosity. Int. J. Impact Eng 48, 98–106 (2012)

    Article  Google Scholar 

  38. Shaw, A., Roy, D., Reid, S.R.: Optimised form of acceleration correction algorithm within SPH-based simulations of impact mechanics. Int. J. Solids Struct. 48(25–26), 3484–3498 (2011)

    Article  Google Scholar 

  39. Shaw, A., Reid, S.R.: Applications of SPH with the acceleration correction algorithm in structural impact computations. Curr. Sci. 97(8), 1177–1186 (2009)

    MathSciNet  Google Scholar 

  40. Shaw, A., Reid, S.R.: Heuristic acceleration correction algorithm for use in SPH computations in impact mechanics. Comput. Methods Appl. Mech. Eng. 198(49–52), 3962–3974 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  41. Wang, S.L.N.: A large-deformation Galerkin SPH method for fracture. J. Eng. Math. 71(3), 305–318 (2011)

    Article  MATH  Google Scholar 

  42. Wong, S., Shie, Y.: Large deformation analysis with Galerkin based smoothed particle hydrodynamics. CMES 36(2), 97–118 (2008)

    MathSciNet  MATH  Google Scholar 

  43. Gray, J.P., Monaghan, J.J.: Numerical modelling of stress fields and fracture around magma chambers. J. Volcanol. Geotherm. Res. 135, 259–283 (2004)

    Article  Google Scholar 

  44. Swegle, J.W., Hicks, D.L., Attaway, S.W.: Smoothed particle hydrodynamics stability analysis. J. Comput. Phys. 116(1), 123–134 (1995)

    Article  MathSciNet  MATH  Google Scholar 

  45. Morris, J.P.: A study of the stability properties of smooth particle hydrodynamics. Publ. Astron. Soc. Aust. 13(1), 97–102 (1996)

    Google Scholar 

  46. Monaghan, J.J.: SPH without a tensile instability. J. Comput. Phys. 159(2), 290–311 (2000)

    Article  MATH  Google Scholar 

  47. Melean, Y., Sigalotti, L.D.G., Hasmy, A.: On the SPH tensile instability in forming viscous liquid drops. Comput. Phys. Commun. 157(3), 191–200 (2004)

    Article  Google Scholar 

  48. Liu, Z.S., Swaddiwudhipong, S., Koh, C.G.: High velocity impact dynamic response of structures using SPH method. Int. J. Comput. Eng. Sci. 5(2), 315–326 (2004)

    Article  Google Scholar 

  49. Monaghan, J.J.: Simulating free surface flows with SPH. J. Comput. Phys. 110, 399–406 (1994)

    Article  MATH  Google Scholar 

  50. Kulasegaram, S., Bonet, J., Lewis, R.W., Profit, M.: High pressure die casting simulation using a Lagrangian particle method. Commun. Numer. Methods Eng. 19(9), 679–687 (2003)

    Article  MATH  Google Scholar 

  51. Cedric, T., Janssen, L.P.B.M., Pep, E.: Smoothed particle hydrodynamics model for phase separating fluid mixtures. I. General equations. Phys. Rev. E Stat. Nonlin. Soft Matter Phys. 72, 016713 (2005)

    Article  Google Scholar 

  52. Cleary, P.W., Ha, J., Prakash, M., Nguyen, T.: 3D SPH flow predictions and validation for high pressure die casting of automotive components. Appl. Math. Model. 30(11), 1406–1427 (2006)

    Article  Google Scholar 

  53. Fang, J., Owens, R.G., Tacher, L., Parriaux, A.: A numerical study of the SPH method for simulating transient viscoelastic free surface flows. J. Nonnewton. Fluid Mech. 139(1–2), 68–84 (2006)

    Article  MATH  Google Scholar 

  54. Imaeda, Y., Inutsuka, S.-I.: Shear flows in smoothed particle hydrodynamics. Astrophys. J. 569(1), 501–518 (2002)

    Article  Google Scholar 

  55. Monaghan, J.J., Gingold, R.A.: Shock simulation by the particle method SPH. J. Comput. Phys. 52(2), 374–389 (1983)

    Article  MATH  Google Scholar 

  56. Monaco, A.D., Manenti, S., Gallati, M., Sibilla, S., Agate, G., Guandalini, R.: SPH modeling of solid boundaries through a semi-analytic approach. Eng. Appl. Comput. Fluid Mech. 5(1), 1–15 (2011)

    Google Scholar 

  57. Libersky, L.D., Randles, P.W., Carney, T.C., Dickinson, D.L.: Recent improvements in SPH modeling of hypervelocity impact. Int. J. Impact Eng 20(6–10 pt 2), 525–532 (1997)

    Article  Google Scholar 

  58. Libersky, L.D., Petscheck, A.G., Carney, T.C., Hipp, J.R., Allahdadi, F.A.: High strain Lagrangian hydrodynamics—a three dimensional SPH code for dynamic material response. J. Comput. Phys. 109(1), 67–75 (1993)

    Article  MATH  Google Scholar 

  59. Randles, P.W., Libersky, L.D.: Smoothed particle hydrodynamics: some recent improvements and applications. Comput. Methods Appl. Mech. Eng. 139(1–4), 375–408 (1996)

    Article  MathSciNet  MATH  Google Scholar 

  60. Mayrhofer, A., Rogers, B.D., Violeau, D., Ferrand, M.: Investigation of wall bounded flows using SPH and the unified semi-analytical wall boundary conditions. Comput. Phys. Commun. 184(11), 2515–2527 (2013)

    Article  MathSciNet  Google Scholar 

  61. Wu, B., Tan, C.P.: Sand production prediction of gas field: methodology and laboratory verification. In: SPE Asia Pacific Oil & Gas Conference and Exhibition, Melbourne, Australia (2002)

  62. Timoshenko, S., Goodier, J.N.: Theory of Elasticity, 3rd edn. McGraw-Hill, New York (1984)

    Google Scholar 

  63. Bathe, K.J.: Finite Element Procedures. Prentice-Hall, Englewood Cliffs (1995)

    Google Scholar 

  64. Babuska, I., Suri, M.: On locking and robustness in the finite element method. SIAM J. Numer. Anal. 29(5), 1261–1293 (1992)

    Article  MathSciNet  MATH  Google Scholar 

  65. Suri, M., Babuska, I., Schwab, C.: Locking effects in the finite element approximation of plate models. Math. Comput. 64(210), 461 (1995)

    Article  MathSciNet  MATH  Google Scholar 

  66. Ozkul, T.A., Ture, U.: The transition from thin plates to moderately thick plates by using finite element analysis and the shear locking problem. Thin-Walled Struct. 42(10), 1405–1430 (2004)

    Article  Google Scholar 

  67. Suri, M.: Analytical and computational assessment of locking in the hp finite element method. Comput. Methods Appl. Mech. Eng. 133(3–4), 347–371 (1996)

    Article  MathSciNet  MATH  Google Scholar 

  68. Hansbo, P.: New approach to quadrature for finite elements incorporating hourglass control as a special case. Comput. Methods Appl. Mech. Eng. 158(3–4), 301–309 (1998)

    Article  MathSciNet  MATH  Google Scholar 

  69. Reese, S., Wriggers, P.: Stabilization technique to avoid hourglassing in finite elasticity. Int. J. Numer. Methods Eng. 48(1), 79–109 (2000)

    Article  MATH  Google Scholar 

  70. Fernandez-Mendez, S., Bonet, J., Huerta, A.: Continuous blending of SPH with finite elements. Comput. Struct. 83, 1448–1458 (2005)

    Article  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mechanical Engineering, Centre for Advanced Composite Materials, University of Auckland, Auckland, 1010, New Zealand

    R. Das

  2. CSIRO Mathematics, Informatics and Statistics, Normanby Road, Clayton, VIC, 3168, Australia

    P. W. Cleary

Authors
  1. R. Das
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. P. W. Cleary
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to R. Das.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Das, R., Cleary, P.W. Evaluation of Accuracy and Stability of the Classical SPH Method Under Uniaxial Compression. J Sci Comput 64, 858–897 (2015). https://doi.org/10.1007/s10915-014-9948-4

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10915-014-9948-4

Keywords

Search

Navigation