Modelling equilibrium grain boundary segregation, grain boundary energy and grain boundary segregation transition by the extended Butler equation

Abstract

The Butler equation is extended to model equilibrium grain boundary (GB) energy and the equilibrium GB composition of a polycrystal, as a function of the following state parameters: bulk composition, temperature, pressure and the five degrees of freedom of the GB. In the simplest case of an ideal solution and equal atomic sizes of the components, the Butler equation reduces back to the well-known McLean equation of GB segregation. When the components repulse each other in the solid solution, grain boundary segregation transition (GBST) appears below the critical temperature of the bulk solid miscibility gap. The GBST line is a new equilibrium line in equilibrium phase diagrams. This new model is demonstrated for copper (Cu) segregation to the GBs in nickel (Ni) and for the phosphorous (P) segregation to the GBs in bcc iron (Fe). The GBST line appears in the Ni-rich (Fe-rich) corner of the Ni–Cu (Fe–P) phase diagram in coordinates of bulk Cu (P) mole fraction vs temperature at fixed pressure. The mole fraction of the solute (Cu or P), corresponding to the GBST line steadily increases with temperature. At a lower solute content (Cu or P), or at a higher temperature compared to the GBST line, the GB is composed mostly of the solvent atoms (Ni or Fe). Contrariwise, at a higher solute content (Cu or P), or at a lower temperature compared to the GBST line, the GB is composed mostly of the solute atoms (Cu or P). These low-segregation and high-segregation states of the GB are transformed into each other via a reversible first-order GBST. This latter process takes place when the GBST line is crossed by changing the bulk composition or the temperature. The results, theoretically estimated, are in agreement with earlier experimental results.

Appendices

Appendix A: estimation of model parameters for the binary Cu–Ni system

The integral molar excess Gibbs energy of the solid fcc Cu–Ni solution is written by the following equation [89, 90] (where x is the bulk mole fraction of Ni):

$$ \Delta G_{m}^{{\text{E}}} = x \cdot \left( {1 - x} \right) \cdot \left[ {L_{o} + L_{1} \cdot \left( {1 - 2 \cdot x} \right)} \right] \cdot \exp \left( { - \frac{T}{{\tau 1}}} \right) + R \cdot T \cdot f(\tau ) \cdot \ln (\beta _{m} + 1) $$

(41)

where L ₀ (J/mol) and L ₁ (J/mol) are the interaction energies between the components in the fcc Cu–Ni solid solution; the exponential term with $\tau 1 = 3000 \;{\text{K}}$ ensures that the molar excess Gibbs energy of the solution tends to zero at high temperatures [91, 92], while the last term of Eq. (41) is due to magnetic ordering, where $\beta_{m}$ (dimensionless) is the average magnetic moment per atom, $\tau \equiv T/T_{\text{mo}}$, with $T_{\text{mo}}$ (K) the critical temperature of magnetic ordering (the Curie temperature), $f(\tau )$ (dimensionless) is the following polynomial [93, 94]:

$$ {\text{at}}\;\tau \le 1:f\left( \tau \right) = 1 - \frac{1}{D} \cdot \left[ {\frac{{79 \cdot \tau ^{{ - 1}} }}{{140 \cdot p}} + \frac{{474}}{{497}} \cdot \left( {\frac{1}{p} - 1} \right) \cdot \left( {\frac{{\tau ^{3} }}{6} + \frac{{\tau ^{9} }}{{135}} + \frac{{\tau ^{{15}} }}{{600}}} \right)} \right] $$

(42)

$$ {\text{at}}\;\tau {\text{ > }}1:\;f\left( \tau \right) = - \frac{1}{D} \cdot \left( {\frac{{\tau ^{{ - 5}} }}{{10}} + \frac{{\tau ^{{ - 15}} }}{{315}} + \frac{{\tau ^{{ - 25}} }}{{1500}}} \right) $$

(43)

where p = 0.28 and D = 2.3425 for the fcc solid solution [93, 94]. The interaction energies of the fcc Cu–Ni solution are found in this paper using a method described in Appendix B. From the coordinates of the critical point of solid miscibility, x _c = 0.673 and T _c = 628 K [88]: L ₀ = 10658.7 J/mol, L ₁ = −3821.9 J/mol. The magnetic parameters are taken from [89] as

$$ \beta _{m} = 0.52 \cdot x + x \cdot \left( {1 - x} \right) \cdot \left[ { - 0.732 - 0.317 \cdot \left( {1 - 2 \cdot x} \right)} \right] $$

(44)

$$ T_{{{\text{mo}}}} = 633 \cdot x + x \cdot (1 - x) \cdot \left[ { - 935.5 - 594.9 \cdot \left( {1 - 2 \cdot x} \right)} \right]\;({\text{K}}) $$

(45)

The partial molar excess Gibbs energies of the two components can be found from Eq. (41) using the following general thermodynamic equations [73, 74]:

$$ \Delta G_{{m,{\text{Cu}}}}^{{\text{E}}} = \Delta G_{m}^{{\text{E}}} - x \cdot \frac{{d\Delta G_{m}^{{\text{E}}} }}{{dx}} $$

(46)

$$ \Delta G_{{m,{\text{Ni}}}}^{{\text{E}}} = \Delta G_{m}^{{\text{E}}} + (1 - x) \cdot \frac{{d\Delta G_{m}^{{\text{E}}} }}{{dx}} $$

(47)

The partial molar excess Gibbs energies of the components in the GB region are expressed by the same Eqs. (41)–(47), but x (the mole fraction of Ni in the bulk solid solution) should be replaced by x _GB (the mole fraction of Ni in the GB region) and Eq. (41) should be multiplied by parameter $\beta$ = 0.92, valid for fcc solid solutions (see above).

The high-angle GB energies of the two pure components are estimated using Eq. (33) and the data of [75, 76, 95] as

$$ \sigma _{{{\text{Cu}}}}^{{\text{o}}} \cong 0.68 - 1.1 \cdot 10^{{ - 4}} \cdot T \left( {{\text{J}}/{\text{m}}^{2} } \right) $$

(48)

$$ \sigma _{{{\text{Ni}}}}^{{\text{o}}} \cong 0.87 - 1.0 \cdot 10^{{ - 4}} \cdot T \left( {{\text{J}}/{\text{m}}^{2} } \right) $$

(49)

The molar volumes of the pure fcc metals Cu and Ni are quite close to each other; moreover, the lattice constant of the Cu–Ni solid solution changes linearly as a function of the mole fraction of Cu [96], and so the partial molar volumes will be taken equal to the molar volumes of the pure elements. That is why the simplified Eq. (37) applies: $\omega_{i} \cong \omega_{i}^{o}$. The molar GB areas are calculated using Eq. (34) with f ≅ 1.24, valid for GBs of fcc crystals (see above). The molar volumes of pure components are taken from [97]. The final equations are as follows:

$$ \omega _{{{\text{Cu}}}}^{{\text{o}}} \cong \omega _{{{\text{Cu}}}} \cong 1.047 \cdot 10^{4} \cdot \left( {7.042 + 3.159 \cdot 10^{{ - 5}} \cdot T^{{1.355}} } \right)^{{2/3}} \left( {{\text{m}}^{2} /{\text{mol}}} \right) $$

(50)

$$ \omega _{{{\text{Ni}}}}^{{\text{o}}} \cong \omega _{{{\text{Ni}}}} \cong 1.047 \cdot 10^{4} \cdot \left( {6.542 + 2.306 \cdot 10^{{ - 5}} \cdot T^{{1.355}} } \right)^{{2/3}} \;\left( {{\text{m}}^{2} /{\text{mol}}} \right) $$

(51)

Appendix B: a method to find the parameters of Eq. (41) from the critical parameters of the miscibility gap

Let us consider a binary A–B solid or liquid solution with a miscibility gap, with the known measured coordinates of its maximum critical point: T _c, K (=the absolute temperature of the critical point) and x _c (=the mole fraction of component B in the critical point). Suppose by definition that the standard Gibbs energies of pure components equal zero. Then, the integral molar Gibbs energy of the solution in the two-parameter approximation of the Redlich–Kister polynomial is written as [91, 92]

$$ G = R \cdot T \cdot \left[ {x \cdot \ln x + \left( {1 - x} \right) \cdot \ln \left( {1 - x} \right)} \right] + x \cdot (1 - x) \cdot \left[ {L_{0} + L_{1} \cdot (1 - 2 \cdot x)} \right] \cdot \exp \left( {\frac{{ - T}}{\tau }} \right) $$

(52)

where x (dimensionless) is the mole fraction of component B, L ₀ (J/mol) and L ₁ (J/mol) are the two interaction energies of the Redlich–Kister polynomial, valid at zero Kelvin; the exponential term is responsible for their temperature dependence, with $\tau$ ≅ 3000 K [91, 92]. The condition of the critical point of the miscibility gap is that the second and third derivatives of Eq. (52) at T = T _c and at x = x _c will equal zero:

$$ \frac{{{\text{d}}^{2} G}}{{{\text{d}}x^{2} }} = \frac{{R \cdot T_{c} }}{{x_{c} \cdot (1 - x_{c} )}} - 2 \cdot L_{{o,T{\text{c}}}} + 6 \cdot L_{{1,T{\text{c}}}} \cdot \left( {2 \cdot x_{c} - 1} \right) = 0 $$

(53a)

$$ \frac{{d^{3} G}}{{dx^{3} }} = \frac{{R \cdot T_{c} \cdot \left( {2 \cdot x_{c} - 1} \right)}}{{x_{c}^{2} \cdot (1 - x_{c} )^{2} }} + 12 \cdot L_{{1,T{\text{c}}}} = 0 $$

(53b)

where L _0,Tc (J/mol) and L _1,Tc (J/mol) are the two interaction energies of the Redlich–Kister polynomial, valid at the critical temperature. The two parameters of Eqs. (53a) and (53b) can be expressed from these two equations as

$$ L_{{o,T{\text{c}}}} = \frac{{R \cdot T_{{\text{c}}} }}{4} \cdot \frac{{6 \cdot x_{c} - 1 - 6 \cdot x_{c}^{2} }}{{x_{c}^{2} \cdot \left( {1 - x_{c} } \right)^{2} }} $$

(54a)

$$ L_{{1,T{\text{c}}}} = \frac{{R \cdot T_{c} }}{{12}} \cdot \frac{{\left( {1 - 2 \cdot x_{c} } \right)}}{{x_{c}^{2} \cdot (1 - x_{c} )^{2} }} $$

(54b)

If x _c = 0.5, then L _1,Tc = 0 from Eq. (54a) and $L_{{o,T{\text{c}}}} = 2RT_{\text{c}}$ from Eq. (54b), which is a well-known equation for the regular solution model. It should be mentioned that $L_{o,Tc}$ has a positive solution only, if $0.211 < x_{c} < 0.789$. As $L_{o,Tc}$ should have a positive value for the thermodynamic properties to be consistent with the miscibility gap, Eqs. (54a) and (54b) can be used only within the interval of $0.3 < x_{c} < 0.7$. If x _c is out of this interval, then a more complex form of the Redlich–Kister polynomial Eq. (52) should be used.

The connection between the values of the interaction energies between zero Kelvin and the critical temperature is given using Eqs. (55a) and (55b) as

$$ L_{0} = L_{{0,T{\text{c}}}} \cdot \exp \left( {\frac{{T_{{\text{c}}} }}{\tau }} \right)$$

(55a)

$$ L_{1} = L_{{1,T{\text{c}}}} \cdot \exp \left( {\frac{{T_{{\text{c}}} }}{\tau }} \right) $$

(55b)

The method developed here can be applied to the Cu–Ni fcc solid solution, as x _c = 0.673 [88], i.e. it is within the requested interval of $0.3 < x_{\text{c}} < 0.7$. Substituting the data of x _c = 0.673 and T _c = 628 K [88] into Eqs. (54a), (54b), (55a) and (55b), the following results are obtained: L ₀ = 10648 J/mol, L ₁ = −3832 J/mol (at fixed $\tau$ = 3000 K).

The heat of mixing of the alloy as a function of composition and temperature can be obtained from the above equations as [91]:

$$ \Delta H \cong x \cdot (1 - x) \cdot \left[ {L_{0} + L_{1} \cdot (1 - 2 \cdot x)} \right] \cdot \left( {1 + \frac{T}{\tau }} \right) \cdot \exp \left( { - \frac{T}{\tau }} \right) $$

(56)

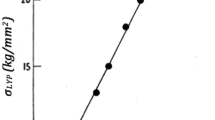
In Fig. 10, the experimental heat of mixing values measured at T = 1350 K [99] are compared with the results calculated by Eq. (56) and the parameters: L ₀ = 10648 J/mol, L ₁ = − 3832 J/mol, $\tau$ = 3000 K, T = 1350 K. As the agreement is reasonably good, model Eq. (52) and the above model parameters describe both the thermodynamic properties and the phase diagram features reasonably well in the Cu–Ni system.

Fig. 10

Comparison of the experimental heat of mixing values (dots) measured at T = 1350 K [99] with the results, calculated by Eq. (56) and the parameters: L ₀ = 10648 J/mol, L ₁ = −3832 J/mol, $\tau$ = 3000 K, T = 1350 K (line)

Appendix C: estimation of model parameters for the binary Fe–P bcc solution

Although the Fe–P system is very complex, only the bcc solid solution and the Fe₃P stoichiometric compound will be considered here. As explained above (see “A second example of calculations: the Fe-P system” section), the A–B model system in this case will be A = Fe and B = Fe₃P. That is why we need a relationship between the mole fraction of P in the real system and the mole fraction of Fe₃P in the model system. In one mole of the Fe–P real solid solution, the number of moles of P atoms are x _P (=the mole fraction of P in the binary Fe–P system). It is supposed here that the Gibbs energy change of the Fe₃P complex formation from the elements Fe and P is so strong that more than 99 % of the P atoms within the bcc matrix are actually in the form of Fe₃P complexes. Thus, the number of these molecules also equals x _P (as each of these molecules contains one P atom). Then, the number of the remaining (free) Fe-atoms will be $1 - 4x_{\text{P}}$, as each Fe₃P molecule contains four atoms. Therefore, the mole fraction of component B (Fe₃P) in the model alloy is written as

$$ x_{{\text{B}}} \equiv x_{{{\text{Fe}}_{3} {\text{P}}}} = \frac{{x_{{\text{P}}} }}{{1 - 3 \cdot x_{{\text{P}}} }} $$

(57)

The value of x _P = 0.25 corresponds to the stoichiometry of the Fe₃P compound. If this value is substituted into Eq. (57), $x_{{{\text{Fe}}_{ 3} {\text{P}}}} = 1$ is obtained, confirming that Eq. (57) obeys the reasonable boundary condition.

According to the phase diagram, the solid bcc Fe–P solution is in equilibrium with the stoichiometric compound Fe₃P below 1321 K [88], with an eutectic point between these two phases at 1232 K. In the present calculation, the liquid Fe–P phase will be thermodynamically “suppressed” (neglected), and thus the bcc/Fe₃P equilibrium will be extrapolated to the temperature of our interest, 1723 K. The thermodynamic properties of the phases in question are taken from [117] (all Gibbs energy values in J/mol-atom, temperature in K, $x \equiv x_{\text{P}}$):

$$ G_{{m,{\text{P,bcc}}}}^{o} = 44769 - 13.26 \cdot T $$

(58)

$$ G_{{m,{\text{bcc}}}} = x \cdot G_{{m,{\text{P,bcc}}}}^{{\text{o}}} + \left( {1 - x} \right) \cdot G_{{m,{\text{Fe,bcc}}}}^{{\text{o}}} + R \cdot T \cdot \left[ {x \cdot \ln x + \left( {1 - x} \right) \cdot \ln \left( {1 - x} \right)} \right] + G_{{m,{\text{bcc}}}}^{{\text{E}}} $$

(59)

$$ \Delta G_{{m,{\text{bcc}}}}^{{\text{E}}} = x \cdot \left( {1 - x} \right) \cdot \left( { - 200300 + 9.0 \cdot T} \right) $$

(60)

$$ G_{{m,{\text{Fe}}_{3} {\text{P}}}}^{{\text{o}}} = 0.25 \cdot \left( { - 193600 + 9.75 \cdot T} \right) $$

(61)

with the following simplifying conditions:

$$ G_{{m,{\text{P,red}}}}^{\text{o}} \equiv 0 $$

(62)

$$ G_{{m,{\text{Fe,bcc}}}}^{\text{o}} \equiv 0 $$

(63)

Let me mention that below 1043 K Eqs. (59) and (63) also contain magnetic terms, but they are ignored here as our calculations are performed at 1723 K. The condition of formal thermodynamic equilibrium between the Fe–P solid solution and the stoichiometric compound Fe₃P is

$$ G_{{m,{\text{bcc}}}} + \left( {0.25 - x_{\text{P}}^{\text{sat}} } \right) \cdot \frac{{{\text{d}}G_{{m,{\text{bcc}}}} }}{{{\text{d}}x}} = G_{{m,{\text{Fe}}_{ 3} {\text{P}}}}^{\text{o}} $$

(64)

where $x_{\text{P}}^{\text{sat}}$ is the saturation mole fraction of P in the bcc Fe–P solid solution, keeping equilibrium with phase Fe₃P. If Eqs. (58)–(63) are substituted into Eq. (64), the resulting equation can be solved for $x_{\text{P}}^{\text{sat}}$ at any given T. At T = 1321 K (the eutectic temperature) the result is $x_{\text{P}}^{\text{sat}} = 0.0477$. This corresponds to 2.7 w % of P, which is in good agreement with the measured value of 2.8 w % P [88]. This agreement validates the method described by Eqs. (58)–(64). For our temperature of interest T = 1723 K: $x_{\text{P}}^{\text{sat}} = 0.0875$. Substituting this value into Eq. (57): $x_{{{\text{Fe}}_{ 3} {\text{P}}}}^{\text{sat}} = 0.1186$.

Now, let us suppose that the Fe–Fe₃P model system is described by the regular solution model. Then, the interaction energy within the framework of the regular solution model is written as

$$ \Omega = \frac{{{\text{R}} \cdot T}}{{2 \cdot x_{{{\text{Fe}}_{3} {\text{P}}}}^{{{\text{sat}}}} - 1}} \cdot \ln \left( {\frac{{x_{{{\text{Fe}}_{3} {\text{P}}}}^{{{\text{sat}}}} }}{{1 - x_{{{\text{Fe}}_{3} {\text{P}}}}^{{{\text{sat}}}} }}} \right) $$

(65)

Substituting T = 1723 K and $x_{{{\text{Fe}}_{ 3} {\text{P}}}}^{\text{sat}} = 0.1186$ into Eq. (65), the value of the interaction energy in the model system Fe–Fe₃P is found as ${\Omega } = 37669$ J/mol. One can see that this value is positive, i.e. the Fe and the Fe₃P components repulse each other, and so they form a miscibility gap with the following critical temperature:

$$ T_{{\text{c}}} = \frac{\Omega }{{2 \cdot R}} $$

(66)

Substituting ${\Omega } = 37699$ J/mol into Eq. (66), $T_{\text{c}} = 2265$ K is found. Multiplying this value by $\beta = 0.92$, $T_{\text{GBST,max}} = 2084$ K is obtained from Eq. (39). As our T = 1723 K is below this maximum temperature, the formation of GBST is expected in the Fe–Fe₃P model system at T = 1723 K. Therefore, the value of ${\Omega } = 37669$ J/mol will be used together with Eqs. (38a)–(38d) for modelling the excess Gibbs energies in the Fe–Fe₃P model system.

The molar volume of bcc Fe crystal at 298 K is [118]: $V_{{{\text{Fe,bcc,}}298\;{\text{K}}}}^{\text{o}} = 7.09\; \times \;10^{ - 6}$ m³/mol. Between T = 298 and 1723 K the bcc Fe crystal exhibits a linear expansion of about 2.4 % [119]. Then, the molar volume of bcc Fe at 1723 K: $V_{{{\text{Fe,bcc,}}1723\;{\text{K}}}}^{o} = 7.61\; \times \;10^{ - 6}$ m³/mol. Substituting this value and $f = 1.24$ into Eq. (34): $\omega_{{{\text{Fe,bcc,}}1723{\text{K}}}}^{\text{o}} = 4.05\; \times \;10^{4}$ m²/mol.

The molar volume of Fe₃P crystal at 298 K is [96]: $V_{{{\text{Fe}}_{ 3} {\text{P,298K}}}}^{\text{o}} = 2.87\; \times \;10^{ - 5}$ m³/mol. Its linear expansion is not known. Let us suppose that it is half that of the bcc Fe crystal, as compounds usually expand less than elements. Then: $V_{{{\text{Fe}}_{ 3} {\text{P,1723}}}}^{\text{o}} = 2.97\; \times \;10^{ - 5}$ m³/mol. Substituting this value and $f = 1.24$ into Eq. (34): $\omega_{{{\text{Fe}}_{ 3} {\text{P,1723}}\;{\text{K}}}}^{\text{o}} = 1.00\; \times \;10^{5}$ m²/mol.

The T dependence of the surface tension of pure liquid iron is written approximately as $\sigma_{\text{Fe,l/g}}^{\text{o}} \cong 2.76 - 4.5\; \times \;10^{ - 4} \;T$ (J/m²) [75, 76]. Substituting this equation into Eq. (33), the interfacial high-angle GB energy of pure bcc iron is estimated as

$$ \sigma _{{{\text{Fe,bcc}}}}^{{\text{o}}} \cong 1.054 - 1.7 \cdot 10^{{ - 4}} \cdot T $$

(67)

Substituting T = 1723 K into Eq. (67): $\sigma_{{{\text{Fe,bcc,1723}}\;{\text{K}}}}^{\text{o}} \cong 0.761$ J/m². The final task is to estimate the GB energy of a pure Fe₃P compound. Due to the lack of a suitable theoretical equation, only the following relationship can be written: $\omega_{{{\text{Fe}}_{ 3} {\text{P,1723}}\;{\text{K}}}}^{\text{o}} \cdot \sigma_{{{\text{Fe}}_{ 3} {\text{P,1723}}\;{\text{K}}}}^{\text{o}} \ll \omega_{{{\text{Fe,bcc,1723}}\;{\text{K}}}}^{\text{o}} \cdot \sigma_{{{\text{Fe,bcc,1723}}\;{\text{K}}}}^{\text{o}}$. Substituting the above values into this condition: $\sigma_{\text{Fe3P,1723K}}^{\text{o}} \ll 0.308$ J/m². A more precise value is found semi-empirically in “A second example of calculations: the Fe-P system” section.