Abstract
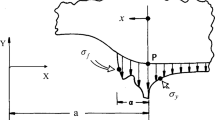
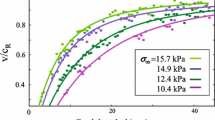
On the base of many experimental results, e.g., Ravi-Chandar and Knauss (Int. J. Fract. 26:65–80, 1984), Sharon et al. (Phys. Rev. Lett. 76(12):2117–2120, 1996), Hauch and Marder (Int. J. Fract. 90:133–151, 1998), the object of our analysis is a rate-dependent model for the propagation of a crack in brittle materials. Restricting ourselves to the quasi-static framework, our goal is a mathematical study of the evolution equation in the geometries of the ‘Single Edge Notch Tension’ and of the ‘Compact Tension’. Besides existence and uniqueness, emphasis is placed on the regularity of the evolution making reference also to the ‘velocity gap’. The transition to the rate-independent model of Griffith is obtained by time rescaling, proving convergence of the rescaled evolutions and of their energies. Further, the discontinuities of the rate-independent evolution are characterized in terms of unstable points of the free energy. Results are illustrated by a couple of numerical examples in the above mentioned geometries.
Similar content being viewed by others
References
Ambrosio, L., Fusco, N., Pallara, D.: Functions of Bounded Variation and Free Discontinuity Problems. Oxford University Press, New York (2000)
Anderson, T.: Fracture Mechanics: Fundamentals and Applications. CRC Press, Boca Raton (1995)
Aubin, J.P., Cellina, A.: Differential Inclusions. Springer, Berlin (1984)
Bourdin, B., Francfort, G., Marigo, J.J.: The variational approach to fracture. J. Elast. 91, 5–148 (2008)
Cherepanov, G.P., Germanovich, L.N.: An employment of the catastrophe theory in fracture mechanics as applied to brittle strength criteria. J. Mech. Phys. Solids 41(10), 1637–1649 (1993)
Ciarlet, P.: Mathematical Elasticity. Three-Dimensional Elasticity. North-Holland, Amsterdam (1988)
Dal Maso, G., Rampazzo, F.: On systems of ordinary differential equations with measures as controls. Differ. Integral Equ. 4(4), 739–765 (1991)
Dal Maso, G., DeSimone, A., Mora, M., Morini, M.: A vanishing viscosity approach to quasistatic evolution in plasticity with softening. Arch. Ration. Mech. Anal. 189(3), 469–544 (2008)
Destuynder, P., Djaoua, M.: Sur une interprétation mathématique de l’intégrale de Rice en théorie de la rupture fragile. Math. Methods Appl. Sci. 3(1), 70–87 (1981)
Evans, L.C., Gariepy, R.F.: Measure Theory and Fine Properties of Functions. Studies in Advanced Mathematics. CRC Press, Boca Raton (1992)
Francfort, G., Marigo, J.J.: Revisiting brittle fracture as an energy minimization problem. J. Mech. Phys. Solids 46(8), 1319–1342 (1998)
Freund, L.: Dynamic Fracture Mechanics. Cambridge University Press, Cambridge (1990)
Griffith, A.: The phenomena of rupture and flow in solids. Philos. Trans. R. Soc. Lond. 18, 163–198 (1920)
Hauch, J., Marder, M.: Energy balance in dynamic fracture, investigated by a potential drop technique. Int. J. Fract. 90, 133–151 (1998)
Knees, D., Mielke, A., Zanini, C.: On the inviscid limit of a model for crack propagation. Math. Models Methods Appl. Sci. 18(9), 1529–1569 (2008)
Larsen, C., Ortiz, M., Richardson, C.: Fracture paths from front kinetics: relaxation and rate-independence. Arch. Ration. Mech. Anal. 193, 539–583 (2009)
Negri, M.: A comparative analysis on variational models for quasi-static brittle crack propagation. Adv. Calc. Var. (submitted)
Negri, M., Ortner, C.: Quasi-static propagation of brittle fracture by Griffith’s criterion. Math. Models Methods Appl. Sci. 18(11), 1895–1925 (2008)
Puglisi, G., Truskinovsky, L.: Thermodynamics of rate-independent plasticity. J. Mech. Phys. Solids 53(3), 655–679 (2005)
Ravi-Chandar, K., Knauss, W.: An experimental investigation into dynamic fracture: II. Microstructural aspects. Int. J. Fract. 26, 65–80 (1984)
Rice, J.: Mathematical analysis in the mechanics of fracture. In: Liebowitz, H. (ed.) Fracture: An Advanced Treatise, pp. 192–308. Academic Press, San Diego (1968)
Sharon, E., Gross, S., Fineberg, J.: Energy dissipation in dynamic fracture. Phys. Rev. Lett. 76(12), 2117–2120 (1996)
Toader, R., Zanini, C.: An artificial viscosity approach to quasi-static crack growth. Boll. Unione Mat. Ital. 2(1), 1–35 (2009)
Zanini, C.: Singular perturbations of finite dimensional gradient flows. Discrete Contin. Dyn. Syst. 18(4), 657–675 (2007)
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Negri, M. From Rate-Dependent to Rate-Independent Brittle Crack Propagation. J Elast 98, 159–187 (2010). https://doi.org/10.1007/s10659-009-9223-3
Received:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10659-009-9223-3