Skip to main content
SpringerLink
Log in

Refinement on non-hydrostatic shallow granular flow model in a global Cartesian coordinate system

  • Original Paper
  • Published:
Computational Geosciences Aims and scope Submit manuscript

Abstract

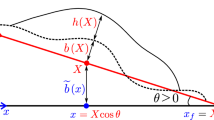
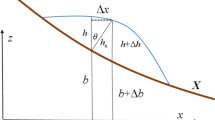
Current shallow granular flow models suited to arbitrary topography can be divided into two types, those formulated in bed-fitted curvilinear coordinates and those formulated in global Cartesian coordinates. The shallow granular flow model of Denlinger and Iverson (J. Geophys. Res. 109, F01014, 2004) and the Boussinesq-type shallow granular flow theory of Castro-Orgaz et al. (2014) are formulated in a Cartesian coordinate system (with z vertical), and both account for the effect of nonzero vertical acceleration on depth-averaged momentum fluxes and stress states. In this paper, we first reformulate the vertical normal stress of Castro-Orgaz et al. (2014) in a quadratic polynomial in the relative elevation η. This form allows for analytical depth integration of the vertical normal stress. We then calculate the basal normal stress based on the basal friction law and scaling analysis. These calculations, plus certain constitutive relations, lead to a refined full non-hydrostatic shallow granular flow model, which is further rewritten in a form of Boussinesq-type water wave equations for future numerical studies. In the present numerical study, we apply the open-source code TITAN2D for numerical solution of a low-order version of the full model involving only a mean vertical acceleration correction term. To cure the numerical instability related with discretization of the enhanced gravity, we propose an approximate formula for the enhanced gravity by utilizing the hydrostatic pressure assumption in the bed normal direction. Numerical calculations are conducted for several test cases involving steep slopes. Comparison with a bed-fitted model shows that even the simplified non-hydrostatic Cartesian model can be used to simulate shallow granular flows over arbitrary topography.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Denlinger, R.P., Iverson, R.M.: Granular avalanches across irregular three-dimensional terrain: 1. Theory and computation. J. Geophys. Res. 109, F01014 (2004). doi:10.1029/2003JF000085

    Article  Google Scholar 

  2. Castro-Orgaz, O., Hutter, K., Giraldez, J.V., Hager, W.H.: Non-hydrostatic granular flow over 3D terrain: new Boussinesq-type gravity waves?. J. Geophy. Res. Earth Surf., 120(1), 10.1002/2014JF003279 (2014)

  3. Pudasaini, S., Hutter, K.: Avalanche dynamics: dynamics of rapid flows of dense granular avalanches, p. 47. Springer-Verlag, Berlin (2007)

    Google Scholar 

  4. Grigorian, S.S., Eglit, M.E., Yakimov, I.L.: A new formulation and solution of the problem of snow avalanche motion. Snow, Avalanches & Glaciers. Tr. Vysokogorn. Geofiz. Inst. 12, 104–113 (1967)

    Google Scholar 

  5. Savage, S., Hutter, K.: The motion of a finite mass of granular material down a rough incline. J. Fluid Mech. 199, 177–215 (1989)

    Article  Google Scholar 

  6. Iverson, R.M.: The physics of debris flows. Rev. Geophys. 35, 245–296 (1997)

    Article  Google Scholar 

  7. Wieland, M., Gray, J.M.N.T., Hutter, K.: Channelized free-surface flow of cohesionless granular avalanches in a chute with shallow lateral curvature. J. Fluid Mech. 392, 73–100 (1999)

    Article  Google Scholar 

  8. Denlinger, R.P., Iverson, R.M.: Flow of variably fluidized granular masses across three-dimensional terrain: 2. Numerical predictions and experimental tests. J. Geophys. Res. 106, 553–566 (2001)

    Article  Google Scholar 

  9. Pouliquen, O., Forterre, Y.: Friction law for dense granular flows: application to the motion of a mass down a rough inclined plane. J. Fluid Mech. 453, 133–151 (2002)

    Article  Google Scholar 

  10. MiDi, G.D.R.: On dense granular flows. Eur. Phys. J. E: Soft Matter 14(4), 341–365 (2004)

    Article  Google Scholar 

  11. Mangeney, A., Bouchut, F., Thomas, N., Vilotte, J.P., Bristeau, M.O.: Numerical modeling of self-channeling granular flows and of their levee-channel deposits. J. Geophys. Res. 112, F02017 (2007). doi:10.1029/2006JF000469

    Article  Google Scholar 

  12. Maeno, F., Hogg, A.J., Sparks, R.S., Matson, G.P.: Unconfined slumping of a granular mass on a slope. Phys. Fluids 25, 023302 (2013). doi:10.1063/1.4792707

    Article  Google Scholar 

  13. McDougall, S., Pirulli, M., Hungr, O., Scavia, C.: Advances in landslide continuum dynamic modelling. In: Proceedings of the Tenth International Symposium on Landslides and Engineered Slopes (Volume 1). Taylor and Francis Group, London, UK (2008)

  14. Luca, I., Hutter, K., Tai, Y.C., Kuo, C.Y.: A hierarchy of avalanche models on arbitrary topography. Acta Mech. 205, 121–149 (2009)

    Article  Google Scholar 

  15. Kuo, C., Tai, Y.C., Bouchut, F., Maneney, A., Pelanti, M., Chen, R., Chang, K.: Simulation of Tsaoling landslide, Taiwan, based on Saint Venant equations over general topography. Engng Geol. 104(3-4), 181–189 (2009)

    Article  Google Scholar 

  16. Takahashi, T., Nakagawa, H., Harada, T., Yamashiki Y.: Routing debris flows with particle segregation. J. Hydr. Res. 118(11), 1490–1507 (1992)

    Google Scholar 

  17. Wu, J., Chen, G.Q., Zhang, L., Zhang, Y.B.: GIS-Based numerical modelling of debris flow motion across three-dimensional terrain. J. Mt. Sci. 10(4), 522–531 (2013)

    Article  Google Scholar 

  18. Patra, A.K., Bauer, A.C., Nichita, C.C., Pitman, E.B., et al.: Parallel adaptive numerical simulation of dry avalanches over natural terrain. J. Volcanol. Geotherm. Res. 139(1), 1–21 (2005)

    Article  Google Scholar 

  19. Chau, K.T., Lo, K.H.: Hazard assessment of debris flows for Leung King estate of Hong Kong by incorporating GIS with numerical simulations. Nat. Hazards Earth Syst. Sci. 4, 103–116 (2004). doi:10.5194/nhess-4-103-2004

    Article  Google Scholar 

  20. Hergarten, S., Robl, J.: Modelling rapid mass movements using the shallow water equations in Cartesian coordinates. Nat. Hazards Earth Syst. Sci. 15, 671–685 (2015). doi:10.5194/nhess-15-671-2015

    Article  Google Scholar 

  21. Gray, J.M.N.T., Wieland, M., Hutter, K.: Free surface flow of cohesionless granular avalanches over complex basal topography. Proc. Roy. Soc. London A 455, 1841–1874 (1999)

    Article  Google Scholar 

  22. Pudasaini, S., Hutter, K., Eckart, W.: Gravity-driven rapid shear flows of dry granular masses in topographies with orthogonal and non-orthogonal metrics. In: Hutter, K., Kirchner, N. (eds.) Dynamic response of granular and porous materials under large and catastrophic deformation. Lecture Notes in Applied and Computational Mechanics, vol. 11, pp. 43–82. Springer, Berlin (2003)

  23. Bouchut, F., Mangeney-Castelnau, A., Perthame, B., Vilotte, J.P.: A new model of Saint-Venant and Savage-Hutter type for gravity driven shallow water flows. C. R. Acad. Sci. Paris Ser. I 336, 531–536 (2003)

    Article  Google Scholar 

  24. Bouchut, F., Westdickenberg, M.: Gravity driven shallow water models for arbitrary topography. Commun. Math. Sci. 2(3), 359–389 (2004)

    Article  Google Scholar 

  25. Green, A., Naghdi, P.: A derivation of equations for wave propagation in water of variable depth. J. Fluid Mech. 78, 237–246 (1976)

    Article  Google Scholar 

  26. Bristeau, M.-O., Mangeney, A., Sainte-Marie, J., Seguin, N.: An energy-consistent depth-averaged Euler system: derivation and properties. Discret. Contin. Dyn. Syst. Ser. B 20 (4), 961–988 (2015). doi:10.3934/dcdsb.2015.20.961

    Article  Google Scholar 

  27. Gray, J.M.N.T.: Rapid granular avalanches. In: Hutter, K., Kirchner, N. (eds.) Dynamic response of granular and porous material under large and catastrophic deformations. Lecture Notes in Applied and Computational Mechanics, vol. 11, pp. 3–42. Springer (2003)

  28. Mangeney-Castelnau, A., Vilotte, J.P., Bristeau, M.O., Perthame, B., Bouchut, F., Simeoni, C., Yerneni, S.: Numerical modeling of avalanches based on Saint Venant equations using a kinetic scheme. J. Geophys. Res. 108(B11), 2527 (2003). doi:10.1029/2002JB002024

    Article  Google Scholar 

  29. GMFG: Titan2d: A open-source simulation code for dry guanlar avalanche flow over natual terrian. http://www.gmfg.buffalo.edu (Unknown Month 2007)

  30. Wang, Y.Q., Hutter, K., Pudasaini, S.P.: The Savage-Hutter theory: A system of partial differential equations for avalanche flows of snow, debris, and mud. Z. Angew. Math. Mech. 84(8), 507–527 (2004). doi:10.1002/zamm.200310123

    Article  Google Scholar 

  31. De Toni, S., Scotton, P.: Two-dimensional mathematical and numerical model for the dynamics of granular avalanches. Cold. Reg. Sci. Tech. 43, 36–48 (2005)

    Article  Google Scholar 

  32. Kelfoun, K., Druitt, T.H.: Numerical modeling of the emplacement of Socompa rock avalanche, Chile. J. Geophys. Res. 110, B12202 (2005)

    Article  Google Scholar 

  33. Chen, W.-C., Kuo, C.Y., Shyue, K.M., Tai, Y.-C.: Gas kinetic scheme for anisotropic Savage-Hutter model. Commun. Comput. Phys. 13(5), 1432–1454 (2013). doi:10.4208/cicp.190112.250512a

    Article  Google Scholar 

  34. Iverson, R.M., Denlinger, R.P.: Flow of variably fluidized granular masses across three-dimensional terrain: 1. Coulomb mixture theory. J. Geophys. Res. 106, 537–552 (2001)

    Article  Google Scholar 

  35. Kim, D.H., Lynett, P.J., Socolofsky, S.: A depth-integrated model for weakly dispersive, turbulent, and rotational fluid flows. Oc. Model. 27(3-4), 198–214 (2009)

    Article  Google Scholar 

  36. Fang, K.Z., Zhang, Z., Zou, Z., Liu, Z., Sun, J.W.: Modelling of 2-D extended Boussinesq equations using a hybrid numerical scheme. J. Hydrodyn. 26(2), 187–198 (2014). doi:10.1016/S1001-6058(14)60021-4

    Article  Google Scholar 

  37. Pitman, E.B., Le, L.: A two-fluid model for avalanche and debris flows. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 363(1832), 1573–1601 (2005)

    Article  Google Scholar 

  38. Juez, C., Murillo, J., García-navarro, P.: 2D simulation of granular flow over irregular steep slopes using global and local coordinates. J. Comput. Phys. 255, 166–204 (2013)

    Article  Google Scholar 

  39. Davis, S.F.: Simplified second order Godunov type methods. SIAM J. Sci. Statist. Comput. 9, 445–473 (1988)

    Article  Google Scholar 

  40. Mangeney, A., Heinrich, P., Roche, R.: Analytical solution for testing debris avalanche numerical models. Pure. Appl. Geophys. 157, 1081–1096 (2000)

    Article  Google Scholar 

  41. Ritter, A.: Die Fortpflanzung der Wasserwelle. Ver. Deutsch. Ing. Z. 36, 947–954 (1892)

    Google Scholar 

Download references

Acknowledgements

L. Yuan, W. Liu, J. Zhai thank the support of state key program for developing basic sciences (2010CB731505, MJ-F-2012-04) and Natural Science Foundation of China (11321061, 11261160486, 91641107). S. Wu thanks the support of the Department of Education of Guangdong Province (2014KQNCX175). A. Patra and E. Pitman acknowledge the support of NSF grants 0620991, 0757367, 0711497, 1228217, and NSF/OAC-1339765.

The modified TITAN2D code can be downloaded from http://lsec.cc.ac.cn/∼lyuan/code.html.

Author information

Authors and Affiliations

  1. LSEC and Institute of Computational Mathematics and Scientific/Engineering Computing, Academy of Mathematics and Systems Science, University of Chinese Academy of Sciences, Chinese Academy of Sciences, Beijing, 100190, China

    L. Yuan, W. Liu & J. Zhai

  2. Department of Computer Science, Guangdong Polytechnic Normal University, Guangzhou, 510665, China

    S. F. Wu

  3. Department of Mechanical and Aerospace Engineering, University at Buffalo SUNY, Buffalo, NY, 14260, USA

    A. K. Patra

  4. Department of Mathematics, University at Buffalo SUNY, Buffalo, NY, 14260, USA

    E. B. Pitman

Authors
  1. L. Yuan
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. W. Liu
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. J. Zhai
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. S. F. Wu
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. A. K. Patra
    View author publications

    You can also search for this author in PubMed Google Scholar

  6. E. B. Pitman
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to L. Yuan.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Yuan, L., Liu, W., Zhai, J. et al. Refinement on non-hydrostatic shallow granular flow model in a global Cartesian coordinate system. Comput Geosci 22, 87–106 (2018). https://doi.org/10.1007/s10596-017-9672-x

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10596-017-9672-x

Keywords

Search

Navigation