An analytical approach to the analysis of an electrically permeable interface crack in a 1D piezoelectric quasicrystal

Abstract

A plane problem is analysed for an electrically permeable crack in a bi-material composed of two semi-infinite 1D piezoelectric quasicrystals bonded together. The polarization direction coincides with the quasiperiodic direction of the materials and is orthogonal to the interface. Uniformly distributed phonon normal and shear in-plane stresses and also phason stress and electric displacement are applied at infinity. The matrix–vector representations for the phonon and phason stresses, the electrical displacement and for the derivatives of the phonon and phason displacements and electrical potentials jumps via the sectional-holomorphic vector-function are derived. Using these relations and satisfying the conditions at the crack faces, the problems of linear relationship are formulated and solved exactly. All required phonon and phason characteristics are given in the form of simple analytical expressions. A numerical analysis is carried out for two different 1D piezoelectric quasicrystals bonded together. The obtained results are presented in graph and table forms.

Appendices

Appendix 1: General solution of Eq. (6)

Assuming that all fields are independent on the coordinate $x_{2}$, the solution of Eq. (6) according to the method suggested in [41] can be presented in the form:

$$\begin{aligned} {\mathbf{V}}={\mathbf{a}}\;{\mathbf{f}}(z), \end{aligned}$$

(A.1)

where $z=x_{1} +p\,x_{3}$, and the vector ${\mathbf{a}} =[a_{1}, a_{2}, a_{3}, a_{4}]^{T}$ can be found from the relation

$$\begin{aligned} \left[ {{\mathbf{Q}}+p({\mathbf{E}}+{\mathbf{E}}^{T})+p^{2}{\mathbf{T}}}\right] \,{\mathbf{a}}=0. \end{aligned}$$

(A.2)

The elements of the $5\times 5$ matrices Q, E, and T are defined as

$$\begin{aligned} {\mathbf{Q}}=\left[ \begin{array}{lll} {c_{1jk1} } &{}\quad {e_{1j1} } &{}\quad {R_{1j31} } \\ {e_{1k1} } &{}\quad {-\xi _{11} } &{}\quad {\tilde{{e}}_{131} } \\ {R_{k131} } &{}\quad {\tilde{{e}}_{131} } &{}\quad {-K_{3131} } \\ \end{array}\right] , {\mathbf{E}}=\left[ \begin{array}{lll} {c_{1jk2} } &{}\quad {e_{21j1} } &{}\quad {R_{j132} } \\ {e_{1k2} } &{}\quad {-\xi _{12} } &{}\quad {\tilde{{e}}_{132} } \\ {R_{k231} } &{}\quad {\tilde{{e}}_{132} } &{}\quad {K_{3132} } \\ \end{array}\right] , {\mathbf{T}}=\left[ \begin{array}{lll} {c_{2jk2} } &{}\quad {e_{2j2} } &{}\quad {R_{j232} } \\ {e_{2k2} } &{}\quad {-\xi _{22} } &{}\quad {\tilde{{e}}_{232} } \\ {R_{k232} } &{}\quad {\tilde{{e}}_{232} } &{}\quad {K_{3232} } \\ \end{array}\right] . \end{aligned}$$

A nontrivial solution of Eq. (A.2) exists if p is a root of the equation

$$\begin{aligned} \det \,\left[ {{\mathbf{Q}}+p({\mathbf{E}}+{\mathbf{E}}^{T})+p^{2}{\mathbf{T}}} \right] =0. \end{aligned}$$

(A.3)

Since Eq. (A.3) has no real roots [42] we denote the roots of Eq. (A.3) with positive imaginary parts as $p_{\alpha }$ and the associated eigenvectors of (A.2) as ${\mathbf{a}}_{\alpha }$ (subscript $\alpha$ here and afterwards takes the numbers 1–5). The most general real solution of Eq. (6) can be presented as [42]

$$\begin{aligned} {\mathbf{V}}={\mathbf{A}}\,{\mathbf{f}}(z)+{\bar{{\mathbf{A}}}}\,{\bar{{\mathbf{f}}}}(\bar{{z}}), \end{aligned}$$

(A.4)

where ${\mathbf{A}}=[{{\mathbf{a}}_{1}, {\mathbf{a}}_{2}, {\mathbf{a}}_{3}, {\mathbf{a}}_{4}, {\mathbf{a}}_{5}}]$ is a matrix composed of eigenvectors, ${\mathbf{f}}(z)=[f_{1} (z_{1} ),f_{2} (z_{2} ), f_{3} (z_{3}), f_{4} (z_{4}), f_{5} (z_{5} )]^{\mathrm{T}}$ is an arbitrary vector function, $z_{\alpha } =x_{1} +p_{\alpha }\,x_{3}$, and the overbar stands for the complex conjugate.

Using Eqs. (1)–(3) the vector t introduced by Eq. (7) can be represented in the form

$$\begin{aligned} \mathbf{t}=\mathbf{B}\,\mathbf{f}'(z)+\bar{\mathbf{B}}\,\bar{\mathbf{f}}'(\bar{z}), \end{aligned}$$

(A.5)

where the $5\times 5$ matrix B is defined as

$$\begin{aligned} {\mathbf{B}}=[{{\mathbf{b}}_{1} ,{\mathbf{b}}_{2} ,{\mathbf{b}}_{3}, {\mathbf{b}}_{4} ,{\mathbf{b}}_{5}}] \end{aligned}$$

with

$$\begin{aligned} {\mathbf{b}}_{\alpha }= & {} ({\mathbf{R}}^{T}+p_{\alpha } {\mathbf{T}}){\mathbf{a}}_{\alpha }\ (\hbox {not summed over index}\ \alpha ) \end{aligned}$$

(A.6)

and

$$\begin{aligned} \mathbf{f}'(z)=\left[ {\frac{\mathrm{d}f_{1} (z_{1} )}{\mathrm{d}z_{1}}, \frac{\mathrm{d}f_{2} (z_{2} )}{\mathrm{d}z_{2} },\frac{\mathrm{d}f_{3} (z_{3} )}{\mathrm{d}z_{3}}, \frac{\mathrm{d}f_{4} (z_{4} )}{\mathrm{d}z_{4} },\frac{\mathrm{d}f_{5} (z_{5} )}{\mathrm{d}z_{5} }}\right] ^{T}. \end{aligned}$$

(A.7)

Appendix 2: Solution for a composite of two 1D hexagonal piezoelectric QCs with mixed boundary conditions at the interface

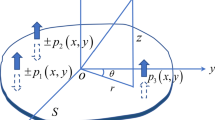
A bimaterial composed of two different semi-infinite 1D hexagonal piezoelectric quasicrystalline spaces $x_{3} >0$ and $x_{3} <0$, with properties defined by Eqs. (1)–(3) for each material, is considered (a cross-section orthogonal to the axis $x_{2}$ is shown in Fig. 1). We assume that the vector t is continuous across the whole bimaterial interface and the part $L=\{(-\infty ,-b)\cup (b,\infty )\}$ of the interface $-\propto< x_{1}<\propto$, $x_{3}=0$ is mechanically and electrically bounded, i.e. the boundary conditions at the interface $x_{3} =0$ are the following ones:

$$\begin{aligned}&{\mathbf{t}}^{(1)}(x_{1} ,0)={\mathbf{t}}^{(2)}(x_{1} ,0) \quad for \quad x_{1} \in (-\infty ,\infty ), \end{aligned}$$

(A.8)

$$\begin{aligned}&{\mathbf{V}}^{({\mathbf{1}})}(x_{1} ,0)={\mathbf{V}}^{(2)}(x_{1} ,0) \quad for\quad x_{1} \in L. \end{aligned}$$

(A.9)

In this case according to Eqs. (A.4), (A.5), the solution of Eqs. (6) can be written for each subdomain in the form

$$\begin{aligned}&{\mathbf{V}}^{(j)}(x_{1} ,x_{3} )={\mathbf{A}}^{(j)}\,{\mathbf{f}}^{(j)}(z) +{\bar{{\mathbf{A}}}}^{(j)}\,{\bar{{\mathbf{f}}}}^{(j)}(\bar{{z}}), \end{aligned}$$

(A.10)

$$\begin{aligned}&{\mathbf{t}}^{(j)}(x_{1} ,x_{3} )={\mathbf{B}}^{(j)}\,{\mathbf{f}'}^{(j)}(z) +{\bar{{\mathbf{B}}}}^{(j)}\,\bar{\mathbf{f}}'^{(j)}(\bar{{z}}), \end{aligned}$$

(A.11)

where $j=1$ for $x_{3}>0$ and $j=2$ for $x_{3}<0$; the vector functions ${\mathbf{f}}^{(1)}(z)$ and ${\mathbf{f}}^{(2)}(z)$ are analytic in the upper ($x_{3}>0$) and the lower ($x_{3}<0$) domains, respectively.

Equation (A.11) and the boundary condition (A.8) give

$$\begin{aligned} {\mathbf{B}}^{(1)}\,{\mathbf{f}'}^{(1)}(x_{1} )-{\bar{{\mathbf{B}}}}^{(2)} \,\bar{\mathbf{f}}'^{(2)}(x_{1})= {\mathbf{B}}^{(2)}\,\mathbf{f}'^{(2)} (x_{1} )-{\bar{{\mathbf{B}}}}^{(1)}\,\bar{\mathbf{f}}'^{(1)}(x_{1}) \ \hbox {for} \quad -\infty<x_{1} <\infty . \end{aligned}$$

(A.12)

The left-hand side of Eq. (A.12) is the boundary value of a function analytic in the domain $x_{3}>0$ and the right-hand side of Eq. (A.12) is a boundary value of another function analytic in the domain $x_{3}<0$. Equation (A.12) means that both functions can be analytically continued into the entire plane, i.e. they are equal for $x_{3}>0$ and $x_{3}<0$, respectively, to a function M(z) analytic in the whole plane. Taking into account that the phonon and phason stresses and the electric displacement are bounded at infinity one gets from Eq. (A.11) that $\mathbf{M} ({z})|_{{z}\rightarrow \propto }=\mathbf{M} ^{(\mathbf 0 )} =\mathbf{const }$. But it means that $\mathbf{M} ({z})=\mathbf{M} ^{(\mathbf 0 )}$ holds true in the whole plane. Thus from Eq. (A.12) it follows

$$\begin{aligned}&{\mathbf{B}}^{(1)}{\mathbf{f}}'^{(1)}\;(z)-{\bar{{\mathbf{B}}}}^{(2)}{\bar{{\mathbf{f}}}}'^{(2)} \;(\bar{{z}})=\mathbf{M} ^{(\mathbf 0 )}\ \hbox {for}\quad x_{3}> 0, \end{aligned}$$

(A.13)

$$\begin{aligned}&{\mathbf{B}}^{(2)}{\mathbf{f}}'^{(2)}\;(z)-{\bar{{\mathbf{B}}}}^{(1)}{\bar{{\mathbf{f}}}}'^{(1)} \;(\bar{{z}})=\mathbf{M} ^{(\mathbf 0 )}\ \hbox {for}\quad x_{3}< 0, \end{aligned}$$

(A.14)

where $\mathbf{M} ^{(\mathbf 0 )}$ is an arbitrary constant vector. Assuming that the eigenvalues are distinct and taking into account that the matrices in Eqs. (A.13), (A.14) are non-singular [40], one obtains

$$\begin{aligned} {\bar{{\mathbf{f}}}}'^{(2)}\;(\bar{{z}})= & {} ({\bar{{\mathbf{B}}}}^{(2)})^{-1} {\mathbf{B}}^{(1)}{\mathbf{f}}'^{(1)}\;(z)-({\bar{{\mathbf{B}}}}^{(2)})^{-1} {\mathbf{M}}^{{\mathbf{(0)}}}\ \hbox {for}\quad x_{3}>0,\nonumber \\ {\bar{{\mathbf{f}}}}'^{(1)}\;(\bar{{z}})= & {} ({\bar{{\mathbf{B}}}}^{(1)})^{-1} {\mathbf{B}}^{(2)}{\mathbf{f}}'^{(2)}\;(z)-({\bar{{\mathbf{B}}}}^{(1)})^{-1} {\mathbf{M}}^{{\mathbf{(0)}}}\ \hbox {for} \quad x_{3}< 0. \end{aligned}$$

(A.15)

Since ${\mathbf{f}}'^{(1)}\;(z)$ and ${\mathbf{f}}'^{(2)}\;(z)$ are arbitrary functions, one can set $\mathbf{M} ^{(\mathbf 0 )} =\mathbf 0$, and Eq. (A.15) gets the form

$$\begin{aligned} {\bar{{\mathbf{f}}}}'^{(2)}\;(\bar{{z}})= & {} ({\bar{{\mathbf{B}}}}^{(2)})^{-1} {\mathbf{B}}^{(1)}{\mathbf{f}}'^{(1)}\;(z)\ \hbox {for}\quad x_{3}> 0,\nonumber \\ {\bar{{\mathbf{f}}}}'^{(1)}\;(\bar{{z}})= & {} ({\bar{{\mathbf{B}}}}^{(1)})^{-1} {\mathbf{B}}^{(2)}{\mathbf{f}}'^{(2)}\;(z)\ \hbox {for}\quad x_{3}< 0. \end{aligned}$$

(A.16)

Consider further the vector

$$\begin{aligned} \left\langle {\mathbf{V}'}(x_{1}) \right\rangle ={\mathbf{V}'}^{(1)}(x_{1} ,0)-{\mathbf{V}'}^{(2)}(x_{1} ,0) \end{aligned}$$

(A.17)

of the derivatives of the jumps of phonon and phason displacements and electric potential across the material interface. By using Eqs. (A.10) and (A.16), it can be written as

$$\begin{aligned} \langle \mathbf{V}'(x_{1})\rangle =\mathbf{Df}'^{(1)}(x_{1}) +\bar{\mathbf{D}}\bar{f}'^{(1)}(x_{1}), \end{aligned}$$

(A.18)

with the definition ${\mathbf{D}}={\mathbf{A}}^{(1)}-{\bar{{\mathbf{A}}}}^{(2)} ({\bar{{\mathbf{B}}}}^{(2)})^{-1}{\mathbf{B}}^{(1)}$.

    From Eq. (A.11), the vector $\mathbf{t} ^{(1)}$ on the material interface can be written as

$$\begin{aligned} \mathbf{t}^{(1)}(x_{1}, 0)={\mathbf{B}}^{(1)}\,{\mathbf{f}'}^{(1)}(x_{1}) +{\bar{\mathbf{B}}}^{(1)}\,{\bar{\mathbf{f}}}'^{(1)}(x_{1}). \end{aligned}$$

(A.19)

Introducing the vector function ${\varvec{\upomega }}(z)$ by the formula

$$\begin{aligned} {\varvec{\upomega }}(z) =\left\{ \begin{array}{ll} \mathbf{D}\,\mathbf{N} (z) &{} \quad \hbox {for}\quad x_{3} >0 \\ -\bar{\mathbf{D}}\,\bar{\mathbf{N}}(z) &{} \quad \hbox {for}\quad x_{3} <0 \\ \end{array}\right. , \end{aligned}$$

(A.20)

with ${\mathbf{N}}(z)=[{f_{1}'^{(1)}(z),\,f_{2}'^{(1)}(z), \,f_{3}'^{(1)}(z),\,f_{4}'^{(1)}(z),f_{5}'^{(1)}(z)}]^{T}$, leads to the following expressions:

$$\begin{aligned} \langle \mathbf{V}'(x_{1})\rangle= & {} {\varvec{\upomega }}^{+} (x_{1})-{\varvec{\upomega }}^{-}(x_{1}), \end{aligned}$$

(A.21)

$$\begin{aligned} \mathbf{t}^{(1)}(x_{1}, 0)= & {} \mathbf{G}{\varvec{\upomega }}^{+} (x_{1})-\bar{\mathbf{G}}{\varvec{\upomega }}^{-}(x_{1}) \end{aligned}$$

(A.22)

where ${\mathbf{G}}={\mathbf{B}}^{(1)}{\mathbf{D}}^{-1}$ and ${\varvec{\upomega }}^{+} (x_{1} )={\varvec{\upomega }}(x_{1} +i0), {\varvec{\upomega }}^{-}(x_{1}) ={\varvec{\upomega }}(x_{1}-i0)$.

    Equations (A.21) and (A.22) can be used for the analysis of compositions of different semi-infinite 1D hexagonal piezoelectric quasicrystals with cracks at their interface.

Appendix 3: Constants of 1D piezoelectric QCs poling in the $x_{3}$-direction [28]

	Upper material	Lower material
Phonon elastic	$c_{11} =150$, $c_{12} =100$, $c_{13} =90$	$c_{11} =234.33$, $c_{12} =57.41$, $c_{13} =66.63$
constants (GPa)	$c_{33} =130$, $c_{44} =50$	$c_{33} =232.22$, $c_{44} =70.19$
Phason elastic constants (GPa)	$K_{1} =0.18$, $K_{2} =0.3$	$K_{1} =122$, $K_{2} =24$
Coupling constants (GPa)	$R_{1} =-1.50$, $R_{2} =1.20$, $R_{3} =1.20$	$R_{1} =R_{2} =R_{3} =0.8846$
Piezoelectric constants	$e_{31} =\tilde{{e}}_{15} =-0.160$, $e_{33} =0.347$,	$e_{31} =-4.4$, $e_{33} =18.6$, $e_{15} =11.6$
(C m$^{-2}$)	$e_{15} =-0.138$, $\tilde{{e}}_{33} =0.350$	$\tilde{{e}}_{15} =1.16$, $\tilde{{e}}_{33} =1.86$
Dielectric constants ($10^{-9}$ C$^{2}$ N$^{-1}$ m$^{-2}$)	$\xi _{11} =0.0826$, $\xi _{33} =0.0903$	$\xi _{11} =5$, $\xi _{33} =10$