u–w formulation for dynamic problems in large deformation regime solved through an implicit meshfree scheme

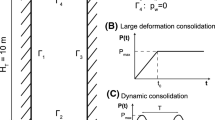
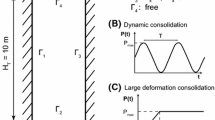
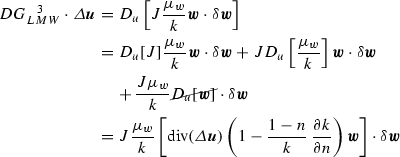Abstract

Solving dynamic problems for fluid saturated porous media at large deformation regime is an interesting but complex issue. An implicit time integration scheme is herein developed within the framework of the u–w (solid displacement–relative fluid displacement) formulation for the Biot’s equations. In particular, liquid water saturated porous media is considered and the linearization of the linear momentum equations taking into account all the inertia terms for both solid and fluid phases is for the first time presented. The spatial discretization is carried out through a meshfree method, in which the shape functions are based on the principle of local maximum entropy LME. The current methodology is firstly validated with the dynamic consolidation of a soil column and the plastic shear band formulation of a square domain loaded by a rigid footing. The feasibility of this new numerical approach for solving large deformation dynamic problems is finally demonstrated through the application to an embankment problem subjected to an earthquake.

A Appendix: Consistent linearization

As the linearization is referred to the undeformed domain, $B_0$, since it is time independent, it is necessary to move Eqs. (31–32) to the reference configuration. From the transport theorems we know that $dv=J \, dV$ and $ds=J\varvec{F}^{-T}dS$ and the Piola transformation states that $Div(\varvec{u})=J\, div(\varvec{u})$ (See [24, 35] for more information). Starting from these points, the equations to be linearized yield

$$\begin{aligned}&-\alpha _7\int _{B_0}\varvec{\tau }':\text{ grad }(\delta \varvec{u}) \, dV \; -\alpha _7\int _{B_0} Q \, \text{ Div }(\varvec{u}) \text{ div }(\delta \varvec{u}) \, dV\nonumber \\&\quad -\, \alpha _7 \int _{B_0} Q \, \text{ Div }(\varvec{w}) \text{ div }(\delta \varvec{u}) \, dV - \alpha _1 \int _{B_0} \left[ \rho _0\varvec{u}+J\rho _w \varvec{w}\right] \cdot \delta \varvec{u} \, dV \nonumber \\&\quad +\, \alpha _8 \int _{B_0} \rho _0\varvec{g}\cdot \delta \varvec{u} \, dV+ \alpha _8 \int _{\delta B_0} {\overline{\varvec{T}}}\cdot \delta \varvec{u} \, dS =0 \end{aligned}$$

(A.1)

$$\begin{aligned}&-\,\alpha _7 \int _{B_0} Q \, \text{ Div } (\varvec{u}) \text{ div }(\delta \varvec{w}) \, dV -\alpha _7 \int _{B_0} Q \, \text{ Div } (\varvec{w}) \text{ div }(\delta \varvec{w}) \, dV\nonumber \\&\quad - \,\alpha _4 \int _{B_0} \frac{J\mu _w}{k}\varvec{w} \cdot \delta \varvec{w} \, dV- \alpha _1 \int _{B_0} \frac{J\rho _w}{n}\varvec{w}\cdot \delta \varvec{w} \, dV\nonumber \\&\quad -\,\alpha _1 \int _{B_0} J \rho _w\varvec{u} \cdot \delta \varvec{w} \, dV + \alpha _8\int _{B_0} J \rho _w\varvec{g} \cdot \delta \varvec{w} \, dV\nonumber \\&\quad -\,\alpha _8\int _{\delta B_0}{\overline{\varvec{T}}}_w\cdot \delta \varvec{w} \, dS =0, \end{aligned}$$

(A.2)

where $\varvec{\tau }'$ is the effective Kirchhoff stress tensor and ${\overline{\varvec{T}}}$ and ${\overline{\varvec{T}}}_w$ are respectively the traction vectors of solid and fluid phases computed respect the undeformed configuration.

Before linearizing the different terms of the target equation, the linearization of some useful terms is carried out against $\varDelta \varvec{u}$:

$$\begin{aligned} D_u\left[ J\right] =J \, \text{ div }(\varDelta \varvec{u}) \end{aligned}$$

(A.3)

(From [16]) Also the linearization of n will be useful for the derivation of other quantities:

$$\begin{aligned} D_u\left[ n\right]= & {} D_u\left[ 1-\frac{1-n_0}{J}\right] =-(1-n_0)\frac{-1}{J^2}D_u\left[ J\right] \nonumber \\= & {} \frac{1-n_0}{J}\text{ div }(\varDelta \varvec{u})=(1-n) \text{ div }(\varDelta \varvec{u}) \end{aligned}$$

(A.4)

From tensor analysis [15] we determine that:

$$\begin{aligned} D_u\left[ \text{ grad }(\varvec{u})\right]= & {} D_u\left[ \text{ Grad }(\varvec{u}) \, \varvec{F}^{-1} \right] \nonumber \\= & {} \text{ Grad }(D_u\left[ \varvec{u} \right] ) \varvec{F}^{-1} + \text{ Grad }(\delta \varvec{u}) D_u\left[ \varvec{F}^{-1} \right] \nonumber \\= & {} \text{ Grad }(\varDelta \varvec{u}) \varvec{F}^{-1} - \text{ Grad }(\varvec{u}) \varvec{F}^{-1} \text{ grad }(\varDelta \varvec{u}) \nonumber \\= & {} \text{ grad }(\varDelta \varvec{u}) - \text{ grad }(\varvec{u}) \text{ grad }(\varDelta \varvec{u}) \end{aligned}$$

(A.5)

(A.6)

$$\begin{aligned} D_u\left[ \text{ Div }(\varvec{u})\right]= & {} D_u\left[ \varvec{I}: \text{ Grad }(\varvec{u})\right] =\varvec{I}:D_u \left[ \text{ Grad }(\varvec{u})\right] \nonumber \\= & {} \varvec{I}:\text{ Grad }(D_u\left[ \varvec{u}\right] )= \varvec{I}:\text{ Grad }(\varDelta \varvec{u})=\text{ Div } (\varDelta \varvec{u})\nonumber \\ \end{aligned}$$

(A.7)

So, the term we can see in the linear momentum balance equations is linearized as follows:

$$\begin{aligned} D_u\left[ \text{ Div }(\varvec{u})\text{ div }(\delta \varvec{u})\right]= & {} D_u\left[ \text{ Div }(\varvec{u})\right] \text{ div }(\delta \varvec{u})\nonumber \\&+\, \text{ Div }(\varvec{u})D_u\left[ \text{ div }(\delta \varvec{u})\right] \nonumber \\= & {} \text{ grad }(\delta \varvec{u}) : [ \text{ Div }(\varDelta \varvec{u})\varvec{I}\nonumber \\&-\, \text{ Div }(\varvec{u}) \text{ grad }^T(\varDelta \varvec{u})] \end{aligned}$$

(A.8)

Other important linearizations can be derived from Eq. A.4:

$$\begin{aligned} D_u\left[ k\right] =(1-n) \, \frac{\partial k}{\partial n}\, \text{ div }(\varDelta \varvec{u}) \end{aligned}$$

(A.9)

(See also [35])

$$\begin{aligned} D_u\left[ J\frac{\mu }{k}\right]= & {} \frac{\mu }{k}J \,\text{ div }(\varDelta \varvec{u})-J\frac{\mu }{k^2}D_u\left[ k\right] \nonumber \\= & {} J\frac{\mu }{k}\left[ 1-\frac{1-n}{k} \frac{\partial k}{\partial n} \right] \text{ div }(\varDelta \varvec{u}) \end{aligned}$$

(A.10)

$$\begin{aligned} D_u\left[ Q\right]= & {} D_u\left[ \frac{K_w}{n}\right] = K_w\frac{\partial }{\partial n}\left[ \frac{1}{n}\right] \, \frac{\partial n}{\partial \varvec{u}}=-\frac{K_w}{n^2}D_u[n]\nonumber \\= & {} -\frac{K_w}{n^2}(1-n) \text{ div }(\varDelta \varvec{u}) \end{aligned}$$

(A.11)

$$\begin{aligned} D_u\left[ J \frac{\rho _w}{n}\right]= & {} \frac{\rho _w}{n} J \, \text{ div }(\varDelta \varvec{u}) -J\frac{\rho _w}{n^2}(1-n) \text{ div }(\varDelta \varvec{u})\nonumber \\= & {} J \frac{\rho _w}{n}\frac{2n-1}{n}\text{ div }(\varDelta \varvec{u}) \end{aligned}$$

(A.12)

As the reference density is defined as

$$\begin{aligned} \rho _0= & {} J\rho =J \, n\rho _{w}+J(1-n)\rho _s\nonumber \\= & {} J \rho _{w}-(1-n_0)\rho _{w}+(1-n_0)\rho _s, \end{aligned}$$

(A.13)

the linearization of the density yields:

$$\begin{aligned} D_u\left[ \rho _0\right] =D_u\left[ J\rho _{w}\right] =J\rho _w \text{ div }(\varDelta \varvec{u}). \end{aligned}$$

(A.14)

The linearization will be stated for the weak form with respect to the reference configuration. Hereinafter the linearization of the terms that upon the deformation field are presented. All other terms will take part of the Newton scheme in the sense that it presented in Sect. 3. In the following equations superscripts represent the different terms of both Linear Momentum Balance equation of mixture and fluid phases respectively.

• $DG_{LMS} \cdot \varDelta \varvec{u}$:

  $$\begin{aligned} DG_{LMS}^{\; \;\;1} \cdot \varDelta \varvec{u}= & {} D_u\left[ \varvec{\tau }':\text{ grad }(\delta \varvec{u})\right] \nonumber \\= & {} \text{ grad }(\varDelta \varvec{u})\varvec{\tau }': \text{ grad }(\delta \varvec{u})\nonumber \\&+ \,J\,\text{ grad }(\varDelta \varvec{u}):\varvec{C}^{ep}: \text{ grad }(\delta \varvec{u})\nonumber \\ \end{aligned}$$

  (A.15)

  where $\varvec{C}^{ep}$ is the material elasto-plastic constitutive tangent operator. This linearization is widely developed in literature [40].

  $$\begin{aligned} DG_{LMS}^{\; \;\;2} \cdot \varDelta \varvec{u}= & {} D_u\left[ Q \, \text{ Div }(\varvec{u})\text{ div }(\delta \varvec{u}) \right] \nonumber \\= & {} D_u\left[ Q\right] \text{ Div }(\varvec{u})\text{ div } (\delta \varvec{u})\nonumber \\&+ \,Q \,D_u\left[ \text{ Div }(\varvec{u})\text{ div }(\delta \varvec{u})\right] \nonumber \\= & {} Q\,\left( -\frac{1-n}{n}\text{ div }(\varDelta \varvec{u}) \text{ Div }(\varvec{u})\text{ div }(\delta \varvec{u})\right. \nonumber \\&+\left. \text{ grad }(\delta \varvec{u}) : \left[ \text{ Div }(\varDelta \varvec{u})\varvec{I}- \text{ Div }(\varvec{u}) \text{ grad }^T(\varDelta \varvec{u})\right] \right) \nonumber \\= & {} J\,Q \,\text{ grad }\bigg (\delta \varvec{u}) :(\text{ div }(\varDelta \varvec{u})\varvec{I}\nonumber \\&-\, \text{ div }(\varvec{u}) \left[ \text{ grad }^T(\varDelta \varvec{u})+\frac{1-n}{n} \text{ div }(\varDelta \varvec{u})\varvec{I}\right] \bigg ) \nonumber \\\end{aligned}$$

  (A.16)
  $$\begin{aligned} DG_{LMS}^{\; \;\;3} \cdot \varDelta \varvec{u}= & {} D_u\left[ Q\, \text{ Div }(\varvec{w})\text{ div }(\delta \varvec{u}) \right] \nonumber \\= & {} D_u\left[ Q\,\right] \text{ Div }(\varvec{w})\text{ div }(\delta \varvec{u})\nonumber \\&+\, Q\, D_u\left[ \text{ Div }(\varvec{w})\right] \text{ div }(\delta \varvec{u})\nonumber \\&+\, Q \, \text{ Div }(\varvec{w})D_u\left[ \text{ div }(\delta \varvec{u})\right] \nonumber \\= & {} -J\,Q\,\text{ grad }(\delta \varvec{u}) :( \text{ div }(\varvec{w}) [ \text{ grad }^T(\varDelta \varvec{u}) \nonumber \\&+ \frac{1-n}{n}\text{ div }(\varDelta \varvec{u})\varvec{I} ] ) \end{aligned}$$

  (A.17)
  $$\begin{aligned} DG_{LMS}^{\; \;\;4} \cdot \varDelta \varvec{u}= & {} D_u\left[ \rho _0\varvec{u}+J\rho _w\varvec{w}\right] \cdot \delta \varvec{u}\\= & {} D_u\left[ J\rho _w\right] \left( \varvec{u}+\varvec{w}\right) \cdot \delta \varvec{u} + J\rho \, D_u[\varvec{u}]\cdot \delta \varvec{u}\nonumber \\= & {} J \left[ \rho \varDelta \varvec{u} + \rho _w \text{ div }(\varDelta \varvec{u})\left( \varvec{u}+ \varvec{w}\right) \right] \cdot \delta \varvec{u}\nonumber \end{aligned}$$

  (A.18)

• $DG_{LMS} \cdot \varDelta \varvec{w}$:

  $$\begin{aligned} DG_{LMS}^{\; \;\;2} \cdot \varDelta \varvec{w}= & {} D_w\left[ Q \, \text{ Div }(\varvec{u})\text{ div }(\delta \varvec{u}) \right] =0 \end{aligned}$$

  (A.19)
  $$\begin{aligned} DG_{LMS}^{\; \;\;3} \cdot \varDelta \varvec{w}= & {} D_w\left[ Q \, \text{ Div }(\varvec{w})\text{ div }(\delta \varvec{u}) \right] \nonumber \\= & {} J\,Q \, \text{ grad }(\delta \varvec{u}) : \text{ div }(\varDelta \varvec{w})\varvec{I} \end{aligned}$$

  (A.20)

• $DG_{LMW} \cdot \varDelta \varvec{u}$:

  $$\begin{aligned} DG_{LMW}^{\; \;\;1} \cdot \varDelta \varvec{u}= & {} D_u\left[ Q \,\text{ div }(\varvec{u})\text{ div }(\delta \varvec{w}) \right] \nonumber \\= & {} J\,Q \,\text{ grad }(\delta \varvec{w}) :\left( \text{ div }(\varDelta \varvec{u})\varvec{I}\right. \nonumber \\&- \left. \text{ div }(\varvec{u}) \left[ \text{ grad }^T(\varDelta \varvec{u})+\frac{1-n}{n} \text{ div }(\varDelta \varvec{u})\varvec{I}\right] \right) \end{aligned}$$

  (A.21)
  $$\begin{aligned} DG_{LMW}^{\; \;\;2} \cdot \varDelta \varvec{u}= & {} D_u\left[ Q\, \text{ div }(\varvec{w})\text{ div }\delta \varvec{w} \right] \nonumber \\= & {} -J\,Q\,\text{ grad }(\delta \varvec{w}) :( \text{ div }(\varvec{w}) [ \text{ grad }^T(\varDelta \varvec{u})\nonumber \\&+\,\frac{1-n}{n}\text{ div }(\varDelta \varvec{u})\varvec{I} ] ) \end{aligned}$$

  (A.22)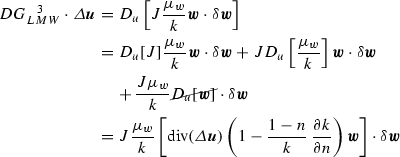
  

  (A.23)
  

  (A.24)
  $$\begin{aligned} DG_{LMW}^{\; \;\;5} \cdot \varDelta \varvec{u}= & {} D_u\left[ J\rho _w \varvec{u}\cdot \delta \varvec{w} \right] \nonumber \\= & {} \left[ D_u[J] \rho _w \varvec{u} + J\rho _w D_u[\varvec{u}]\right] \cdot \delta \varvec{w} \nonumber \\= & {} J \rho _w \left[ \varDelta \varvec{u}-\text{ div }(\varDelta \varvec{u})\varvec{u}\right] \cdot \delta \varvec{w} \end{aligned}$$

  (A.25)

• $DG_{LMW} \cdot \varDelta \varvec{w}$:

  $$\begin{aligned} DG_{LMW}^{\; \;\;1} \cdot \varDelta \varvec{w}= & {} D_w\left[ Q \text{ div }(\varvec{u})\text{ div }(\delta \varvec{w}) \right] =0 \end{aligned}$$

  (A.26)
  $$\begin{aligned} DG_{LMW}^{\; \;\;2} \cdot \varDelta \varvec{w}= & {} D_w\left[ Q \text{ div }(\varvec{w})\text{ div }(\delta \varvec{w}) \right] \nonumber \\= & {} J\,Q \, \text{ grad }(\delta \varvec{w}) : \text{ div }(\varDelta \varvec{w})\varvec{I} \end{aligned}$$

  (A.27)

Finally, using the different terms carried out in the Eqs. (A.15–A.27), the linearization of Eqs. (A.1–A.2) gives the following result:

$$\begin{aligned}&-\,\alpha _7 \int _B \text{ grad }(\delta \varvec{u}):\varvec{c}^{ep}: \text{ grad }(\varDelta \varvec{u}) \, dv\nonumber \\&-\,\alpha _7 \int _B \varvec{\sigma }':\text{ grad }^T (\delta \varvec{u} )\, \text{ grad }(\varDelta \varvec{u}) \, dv\nonumber \\&-\,\alpha _7 \int _B\text{ grad }(\, \delta \varvec{u}) :\left( Q\left[ \text{ div }(\varDelta \varvec{u})+\text{ div } (\varDelta \varvec{w}) \right] \varvec{I}\right) dv\nonumber \\&-\,\alpha _7 \int _B\text{ grad }(\, \delta \varvec{u}) : \left( p_w \left[ \text{ grad }^T(\varDelta \varvec{u})+\frac{1-n}{n}\text{ div } (\varDelta \varvec{u})\varvec{I} \right] \right) dv\nonumber \\&-\, \alpha _1\int _B \delta \varvec{u} \cdot \left[ \rho \varDelta \varvec{u} + \rho _w \varDelta \varvec{w} + \rho _w \text{ div }(\varDelta \varvec{u}) \left( \varvec{u}+\varvec{w}\right) \right] dv\nonumber \\&+ \,\alpha _8\int _B \rho _w \delta \varvec{u} \cdot \varvec{g} \, \text{ div }(\varDelta \varvec{u})\, dv \end{aligned}$$

(A.28)

$$\begin{aligned}&-\,\alpha _7 \int _B \text{ grad }(\, \delta \varvec{w}) :\left( Q\left[ \text{ div }(\varDelta \varvec{u})+ \text{ div }(\varDelta \varvec{w}) \right] \varvec{I}\right) dv\nonumber \\&-\,\alpha _7 \int _B \text{ grad }(\, \delta \varvec{w}) :\left( p_w \left[ \text{ grad }^T(\varDelta \varvec{u}) +\frac{1-n}{n}\text{ div }(\varDelta \varvec{u})\varvec{I} \right] \right) dv\nonumber \\&-\, \alpha _4 \int _B \frac{\mu _w}{k} \delta \varvec{w} \cdot \left[ \varDelta \varvec{w} + \text{ div }(\varDelta \varvec{u})\left( 1-\frac{1-n}{k} \, \frac{\partial k}{\partial n}\right) \varvec{w}\right] \, dv\nonumber \\&- \,\alpha _1\int _B\frac{\rho _w}{n} \delta \varvec{w} \cdot \left[ \varDelta \varvec{w} + \frac{2n-1}{n}\text{ div }(\varDelta \varvec{u})\varvec{w}\right] \, dv\nonumber \\&- \,\alpha _1 \int _B \rho _w \delta \varvec{w} \cdot \left[ \varDelta \varvec{u} + \text{ div }(\varDelta \varvec{u}) \varvec{u}\right] \, dv\nonumber \\&+\, \alpha _8\int _B \rho _w \delta \varvec{w} \cdot \varvec{g} \, \text{ div }(\varDelta \varvec{u})\, dv \end{aligned}$$

(A.29)