Optimal waste control with abatement capital

Abstract

In this paper, we address the question of how “green” growth differs from other patterns of economic growth. To this aim we analyze the control problem of a social optimum with waste, abatement and productive capital stocks. Consumption generates waste. We have two main results: (1) An environmental Keynes-Ramsey rule showing how along the transitional path, consumption dynamics is affected by capital and waste. One crucial implication is that faster waste emissions do not always call for faster abatement investment, and this effect can generate an overshooting in waste and productive capital stock, not present in the standard Keynes-Ramsey model. (2) In the steady state both productive capital stock and output are unchanged relative to the standard Ramsey model; nonetheless, the output composition changes since, to make room for abatement investment, steady state consumption must be reduced. We stress that, when abatement activities are treated as flows, the benefits and costs of abatement capital are greatly undervalued. It is the marginal impact of the whole abatement stock that impinges upon waste accumulation and current consumption, not only the additional unit of abatement activity.

Appendix: The analysis of the dynamic system

We now show that the system with the three state variables (M, T, K) is conditionally stable. Linearizing the differential system (21) around its steady state (remembering that μ = ψ, and thus omitting the equation for $\dot {\mu }$),

$$\left\{ \begin{array}{l} \dot{\psi}=\psi \left( \delta +\rho \right) +s\lambda \\ \dot{T}=K^{\varepsilon} -C-\delta T \\ \dot{\lambda}=\lambda \left( v+\rho \right) +\gamma M^{\gamma -1} \\ \dot{M}=hC-s\left( T-K\right) -vM \\ \dot{K}=-\frac{1}{1-\varepsilon} \left( K\varepsilon K^{\varepsilon -1}+K\gamma \frac{M^{\gamma -1}}{\lambda} +\left( v-\delta \right) K\right) \end{array} \right. $$

we get the corresponding Jacobian matrix:

$$J=\left( \begin{array}{lllll} \rho +\delta & 0 & s & 0 & 0 \\ G & -\delta & -hG & 0 & \rho +\delta\\ 0 & 0 & \nu +\rho & \gamma \left( \gamma -1\right) M^{\ast \gamma -2} & 0\\ -hG & -s & h^{2}G & -\nu & s\\ 0 & 0 & \frac{1}{\varepsilon -1}\frac{K^{\ast} }{\lambda^{\ast} } \left( \rho +v\right) & -\frac{\gamma -1}{\varepsilon -1}\frac{K^{\ast} }{ M^{\ast} }\left( \rho +v\right) & \rho +\delta \end{array} \right) $$

where use has been made of the fact that in steady state $\rho +v=-\frac { \gamma } {\lambda ^{\ast } }M^{\ast ^{\gamma -1}}$ (from $\dot {\lambda }=0$) and $\rho +\delta =\varepsilon \left (K^{\ast } \right )^{\varepsilon -1}$ (from $\dot {\mu }=0$).

The characteristic equation for the linearized system is

$$ \left\vert \begin{array}{lllll} \delta +\rho -x & 0 & s & 0 & 0 \\ G & -\delta -x & -hG & 0 & \rho +\delta\\ 0 & 0 & v+\rho -x & \gamma \left( \gamma -1\right) M^{\ast \gamma -2} & 0 \\ -hG & -s & h^{2}G & -v-x & s\\ 0 & 0 & \frac{1}{\varepsilon -1}\frac{K^{\ast} }{\lambda^{\ast} } \left( \rho +v\right) & -\frac{\gamma -1}{\varepsilon -1}\frac{K^{\ast} }{ M^{\ast} }\left( \rho +v\right) & \rho +\delta -x \end{array} \right\vert =0 $$

(37)

We proceed by expanding it:

$$\begin{array}{@{}rcl@{}} &&\left( \rho +\delta -x\right) \left\{ \left( \rho +\delta -x\right) \left[ \left( -\delta -x\right) \left[ \left( \nu +\rho -x\right) \left( -\nu -x\right) -L\right] +s\frac{L}{h}\right]+s\left[ \left( \delta +x\right) \frac{L}{h}+\frac{s}{h^{2}}L\right] \right\} \\ &&-\left( \delta +x\right) \left( \rho +\delta -x\right) N\left[ \frac{ \left( \nu +\rho -x\right)} {M^{\ast} }+\frac{\gamma M^{\ast \gamma -2}}{ \lambda^{\ast} }\right] \\ &&+\left( \rho +\delta \right) \left( \rho +\delta -x\right) N\left[ \frac{ \left( \nu +\rho -x\right)} {M^{\ast} }+\frac{\gamma M^{\ast \gamma -2}}{ \lambda^{\ast} }\right] =0 \end{array} $$

where $G=\frac {1}{\alpha \left (1-\alpha \right )} \left (\frac {\psi ^{\ast } -h\lambda ^{\ast } }{\alpha } \right )^{\frac {1}{\alpha -1}-1},$ $L=h^{2}G\gamma \left (\gamma -1\right ) M^{\ast \gamma -2}$ and $N=s\frac { \gamma -1}{\varepsilon -1}K^{\ast } \left (\rho +v\right ) <0.$

Remembering that $\rho +v=-\frac {\gamma } {\lambda ^{\ast } }M^{\ast ^{\gamma -1}},$ we simplify the last expression by writing

$$\begin{array}{ll} \left( \rho +\delta -x\right) \left\{ \left( \rho +\delta -x\right) \left[ \left( -\delta -x\right) \left[ \left( v+\rho -x\right) \left( -v-x\right) -L \right] +s\frac{L}{h}\right] +\right. \\ \left. +s\left[ \left( \delta +x\right) \frac{L}{h}+\frac{s}{h^{2}}L\right] +Nx\frac{x}{M^{\ast} }-N\rho \frac{x}{M^{\ast} }\right\} {\small =0} \end{array} $$

Hence, a root of the characteristic equation is ρ + δ. The remaining four eigenvalues are the roots of the following quartic equation:

$$ R\left( x\right) =x^{4}+bx^{3}+cx^{2}+dx+e=0 $$

(38)

where

$$\begin{array}{@{}rcl@{}} b &=&-2\rho,\\ c &=&\rho^{2}-\rho \left( \delta +v\right) -\left( \delta^{2}+v^{2}+L- \frac{N}{M^{\ast} }\right) , \\ d &=&\rho^{2}\left( \delta +v\right) +\rho \left( \delta^{2}+v^{2}+L-\frac{ N}{M^{\ast} }\right) ,\text{} \\ e &=&\left( \rho +\delta \right) \left[ -\delta \left( -v\left( \rho +v\right) -L\right) +\frac{s}{h}L\right] +\frac{s}{h}L\left( \delta +\frac{s} {h}\right) \end{array} $$

From the mathematical point of view, it is remarkable that the structure of our intertemporal problem provides a solution of the linearized system in the presence of three state variables, namely, waste, abatement and productive capital stocks.

Our quartic Eq. 10 has the special property that can be reduced to a biquadratic equation by a double change of variable: first, from x to

$$y=x+\frac{b}{4}, $$

and then from y to z = y ².

The first change produces the following equation:

$$P\left( y\right) =\left( y-\frac{b}{4}\right)^{4}+b\left( y-\frac{b}{4} \right)^{3}+c\left( y-\frac{b}{4}\right)^{2}+d\left( y-\frac{b}{4}\right) +e=0 $$

that is

$$P\left( y\right) =y^{4}+\left( c-\frac{3b^{2}}{8}\right) y^{2}+\left( d- \frac{bc}{2}+\frac{b^{3}}{8}\right) y-\frac{3b^{4}}{256}+\frac{cb^{2}}{16}- \frac{db}{4}+e=0. $$

Notice that the coefficient of the term of first degree in y is identically equal to zero since

$$d+\frac{b^{3}}{8}-\frac{bc}{2}=\rho^{2}\left( \delta +v\right) +\rho \left( \delta^{2}+v^{2}+L-\frac{N}{M^{\ast} }\right) -\rho^{3}+\rho^{3}-\rho^{2}\left( \delta +v\right) -\rho \left( \delta^{2}+v^{2}+L-\frac{N}{ M^{\ast} }\right) =0 $$

Hence, we can rewrite $P\left (y\right )$ as:

$$P\left( y\right) =y^{4}+\theta y^{2}+\phi =0 $$

where $\theta =c-\frac {3b^{2}}{8}$ and $\phi =-\frac {3b^{4}}{256}+\frac { cb^{2}}{16}-\frac {db}{4}+e.$ Making the change of variable

$$y^{2}=z, $$

we get

$${\Omega} \left( z\right) =z^{2}+\theta z+\phi =0. $$

Notice that 𝜃 is always negative (remember that N < 0) since:

$$\begin{array}{@{}rcl@{}} \theta &=&c-\frac{3b^{2}}{8}=\rho^{2}-\rho \left( \delta +v\right) -\left( \delta^{2}+v^{2}+L-\frac{N}{M^{\ast} }\right) -\frac{3}{8}4\rho^{2} \\ &=&\left( 1-\frac{3}{2}\right) \rho^{2}-\rho \left( \delta +v\right) -\left( \delta^{2}+v^{2}+L-\frac{N}{M^{\ast} }\right) \\ &=&-\left[ \frac{1}{2}\rho^{2}+\rho \left( \delta +v\right) +\left( \delta^{2}+v^{2}+L-\frac{N}{M^{\ast} }\right) \right] <0 \end{array} $$

while ϕ is always positive:

$$\phi =-\frac{3b^{4}}{256}+\frac{cb^{2}}{16}-\frac{db}{4}+e>0 $$

Indeed, e is greater than zero and so are the first three addends of ϕ:

$$\begin{array}{@{}rcl@{}} &&-\frac{3b^{4}}{256}+\frac{cb^{2}}{16}-\frac{db}{4} \\ &=&-\frac{3\cdot 16}{256}\rho^{4}+\frac{\rho^{2}}{4}\left[ \rho^{2}-\rho \left( \delta +v\right) -\left( \delta^{2}+v^{2}+L-\frac{N}{M^{\ast} } \right) \right] \\&&+\frac{\rho} {2}\left[ \rho^{2}\left( \delta +v\right) +\rho \left( \delta^{2}+v^{2}+L-\frac{N}{M^{\ast} }\right) \right] \\ &=&\left( \frac{1}{4}-\frac{3}{16}\right) \rho^{4}+\frac{1}{4}\rho^{3}\left( \delta +v\right) +\frac{1}{4}\rho^{2}\left( \delta^{2}+v^{2}+L- \frac{N}{M^{\ast} }\right) \\ &=&\frac{1}{4}\rho^{2}\left[ \frac{1}{4}\rho^{2}+\rho \left( \delta +v\right) +\left( \delta^{2}+v^{2}+L-\frac{N}{M^{\ast} }\right) \right] >0 \end{array} $$

1.1 A.1 Solving the biquadratic equation

We now solve the biquadratic equation. Its solutions are:

$$z_{1,2}=\frac{-\theta \pm \sqrt{\theta^{2}-4\phi} }{2}=\frac{-\theta \pm \sqrt{\left( \theta -2\sqrt{\phi} \right) \left( \theta +2\sqrt{\phi} \right)} }{2} $$

Since 𝜃 is negative, the radicand will be positive, and the roots real, if and only if $\theta +2\sqrt {\phi }$ is negative, i.e. if and only if $\theta <-2\sqrt {\phi }$ or equivalently $-\theta >2\sqrt {\phi }$. In such a case, both z ₁ and z ₂ are positive. The corresponding negative roots for x are:

$$\begin{array}{@{}rcl@{}} x_{1} &=&-\sqrt{\frac{-\theta +\sqrt{\theta^{2}-4\phi} }{2}}-\frac{b}{4}=- \sqrt{\frac{-\theta +\sqrt{\theta^{2}-4\phi} }{2}}+\frac{\rho} {2} \\ x_{2} &=&-\sqrt{\frac{-\theta -\sqrt{\theta^{2}-4\phi} }{2}}-\frac{b}{4}=- \sqrt{\frac{-\theta -\sqrt{\theta^{2}-4\phi} }{2}}+\frac{\rho} {2} \end{array} $$

while the positive ones are:

$$\begin{array}{@{}rcl@{}} x_{3} &=&\sqrt{\frac{-\theta +\sqrt{\theta^{2}-4\phi} }{2}}-\frac{b}{4}= \sqrt{\frac{-\theta +\sqrt{\theta^{2}-4\phi} }{2}}+\frac{\rho} {2} \\ x_{4} &=&\sqrt{\frac{-\theta -\sqrt{\theta^{2}-4\phi} }{2}}-\frac{b}{4}= \sqrt{\frac{-\theta -\sqrt{\theta^{2}-4\phi} }{2}}+\frac{\rho} {2} \end{array} $$

If instead $\theta >-2\sqrt {\phi } ,$ z ₁ and z ₂ are complex conjugates. In this case the x solutions are:

$$\begin{array}{@{}rcl@{}} x_{1,2} &=&-\frac{1}{2}\sqrt{z_{1}}-\frac{b}{4}\pm \sqrt{z_{2}}i; \\ x_{3,4} &=&\frac{1}{2}\sqrt{z_{1}}-\frac{b}{4}\pm \sqrt{z_{2}}i. \end{array} $$

In any case, to these four solutions, be they real or complex, we must add the fifth solution x ₅ = ρ + δ.

1.2 A.2 The dynamics of the system

Having determined the characteristic roots, we now focus on the dynamics of the system. Let x ₁ and x ₂ be the two characteristic negative and stable roots. For convenience, we will assume that $\left \vert x_{1}\right \vert >\left \vert x_{2}\right \vert$. Correspondingly, we have two characteristic vectors, v ¹ and v ²:

$$Jv_{1}=x_{1}v_{1}\text{ and } Jv_{2}=x_{2}v_{2} $$

Hence, we can write the linearized version of the five differential equations as follows:

$$\begin{array}{@{}rcl@{}} \psi \left( t\right) &=&a_{1}{v_{1}^{1}}\exp (x_{1}t)+a_{2}{v_{1}^{2}}\exp (x_{2}t)+\psi^{\ast} \\ T\left( t\right) &=&a_{1}{v_{2}^{1}}\exp (x_{1}t)+a_{2}{v_{2}^{2}}\exp (x_{2}t)+T^{\ast} \\ \lambda \left( t\right) &=&a_{1}{v_{3}^{1}}\exp (x_{1}t)+a_{2}{v_{3}^{2}}\exp (x_{2}t)+\lambda^{\ast} \\ M\left( t\right) &=&a_{1}{v_{4}^{1}}\exp (x_{1}t)+a_{2}{v_{4}^{2}}\exp (x_{2}t)+M^{\ast} \\ K\left( t\right) &=&a_{1}{v_{5}^{1}}\exp (x_{1}t)+a_{2}{v_{5}^{2}}\exp (x_{2}t)+K^{\ast} \end{array} $$

(39)

where a ₁ and a ₂ are two arbitrary constants. Assuming as given by history $K\left (0\right )$ and $M\left (0\right ) ,$ we can determine these two constants using the second and fourth equation of the previous system:

$$\begin{array}{@{}rcl@{}} M\left( 0\right) &=&a_{1}{v_{4}^{1}}+a_{2}{v_{4}^{2}}+M^{\ast} \\ K\left( 0\right) &=&a_{1}{v_{5}^{1}}+a_{2}{v_{5}^{2}}+K^{\ast} \end{array} $$

(40)

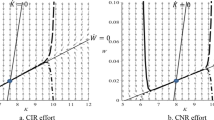
Without any loss of generality, suppose $K\left (0\right ) =K^{\ast }$ and $M\left (0\right ) >M^{\ast } .$ This is the situation depicted by point B in Fig. 1. Then,

$$\begin{array}{@{}rcl@{}} a_{1} &=&\frac{\left\vert \begin{array}{cc} M\left( 0\right) -M^{\ast} & {v_{4}^{2}} \\ K\left( 0\right) -K^{\ast} & {v_{5}^{2}} \end{array} \right\vert} {\Delta} =\frac{\left( M\left( 0\right) -M^{\ast} \right) {v_{5}^{2}}}{\Delta} , \\ a_{2} &=&\frac{\left\vert \begin{array}{cc} {v_{4}^{1}} & \left( M\left( 0\right) -M^{\ast} \right) \\ {v_{5}^{1}} & \left( K\left( 0\right) -K^{\ast} \right) \end{array} \right\vert} {\Delta} =-\frac{\left( M\left( 0\right) -M^{\ast} \right) {v_{5}^{1}}}{\Delta} \end{array} $$

(41)

where

$$ {\Delta} =\left\vert \begin{array}{cc} {v_{4}^{1}} & {v_{4}^{2}} \\ {v_{5}^{1}} & {v_{5}^{2}} \end{array} \right\vert ={v_{4}^{1}}{v_{5}^{2}}-{v_{4}^{2}}{v_{5}^{1}}. $$

(42)

Note that since by assumption $M\left (0\right ) >M^{\ast }$, $a_{1}{v_{4}^{1}}+a_{2}{v_{4}^{2}}$ must be positive, that is

$$\frac{\left( M\left( 0\right) -M^{\ast} \right) {v_{5}^{2}}}{\Delta} {v_{4}^{1}}- \frac{\left( M\left( 0\right) -M^{\ast} \right) {v_{5}^{1}}}{\Delta} {v_{4}^{2}}>0 $$

Simplifying, we get

$$\frac{{v_{5}^{2}}{v_{4}^{1}}-{v_{5}^{1}}{v_{4}^{2}}}{\Delta} =\frac{\Delta} {\Delta} >0 $$

Thus, Δ ha an ambiguous sign. Without loss of generality, we assume that Δ > 0, i.e. ${v_{5}^{2}}{v_{4}^{1}}>{v_{5}^{1}}{v_{4}^{2}}$ (otherwise, interchange the rows in Eq. 42). The dynamics of $A\left (t\right )$ is calculated residually since $A\left (t\right ) =T\left (t\right ) -K\left (t\right )$.

1.3 A.3 The overshooting of the stock of pollution

We can consider the curve in Fig. 1 as a parametric curve the component functions of which are $\left (M\left (t\right ) ,K\left (t\right ) \right )$. Even if we do not know the equation of the curve, we can calculate its derivative, since

$$\frac{dK}{dM}=\frac{\frac{dK\left( t\right)} {dt}}{\frac{dM\left( t\right)} { dt}}=\frac{K^{\prime} \left( t\right)} {M^{\prime} \left( t\right)} . $$

When the position of the curve is D, the derivative $K^{\prime } \left (t\right )$ must be equal to 0, or from the system (39)

$$K^{\prime} \left( t\right) =x_{1}a_{1}{v_{5}^{1}}\exp (x_{1}t)+x_{2}a_{2}{v_{5}^{2}}\exp (x_{2}t)=0, $$

that is,

$$x_{1}a_{1}{v_{5}^{1}}\exp (x_{1}t)=-x_{2}a_{2}{v_{5}^{2}}\exp (x_{2}t). $$

Substituting the solutions for a ₁ and a ₂ from Eq. 41, we can solve this equation for t:

$$\begin{array}{@{}rcl@{}} \ln \left( x_{1}\right) +x_{1}t &=&\ln \left( x_{2}\right) +x_{2}t \\ \hat{t} &=&\frac{\ln \left( \frac{x_{1}}{x_{2}}\right)} {x_{2}-x_{1}}. \end{array} $$

Notice that $\hat {t}$ is positive since $\left \vert x_{1}\right \vert >\left \vert x_{2}\right \vert$, which entails $\frac {x_{1}}{x_{2}}>1$. Substituting $\hat {t}$ in the equation for $M\left (t\right ) ,$we obtain

$$\begin{array}{@{}rcl@{}} M\left( \hat{t}\right) &=&a_{1}{v_{4}^{1}}\exp (x_{1}\hat{t} )+a_{2}{v_{4}^{2}}\exp (x_{2}\hat{t})+M^{\ast} \\ &=&\frac{\left( M\left( 0\right) -M^{\ast} \right) {v_{5}^{2}}}{\Delta} {v_{4}^{1}}\left( \frac{x_{1}}{x_{2}}\right)^{\frac{x_{1}}{x_{2}-x_{1}}}- \frac{\left( M\left( 0\right) -M^{\ast} \right) {v_{5}^{1}}}{\Delta} {v_{4}^{2}}\left( \frac{x_{1}}{x_{2}}\right)^{\frac{x_{2}}{x_{2}-x_{1}}} +M^{\ast} \\ &=&\frac{\left( M\left( 0\right) -M^{\ast} \right)} {\Delta} \left( \frac{ x_{1}}{x_{2}}\right)^{\frac{x_{1}}{x_{2}-x_{1}}}\left( {v_{5}^{2}}{v_{4}^{1}}- \frac{x_{1}}{x_{2}}{v_{5}^{1}}{v_{4}^{2}}\right) +M^{\ast} \\ &=&\frac{\left( M\left( 0\right) -M^{\ast} \right)} {\Delta} \left( \frac{ x_{1}}{x_{2}}\right)^{\frac{x_{1}}{x_{2}-x_{1}}}\left( {v_{5}^{2}}{v_{4}^{1}}- \frac{x_{1}}{x_{2}}{v_{5}^{1}}{v_{4}^{2}}\right) +M^{\ast} \end{array} $$

(43)

We now show that $M\left (\hat {t}\right ) <M^{\ast }$. To put it in words, there is always overshooting.

The product on the right-hand side of Eq. 43 is composed of three terms: the first two are positive (since Δ > 0,and $M\left (0\right ) >M^{\ast } )$, and the two roots x ₁ and x ₂ are both negative. As for the third term, we claim that

$${v_{5}^{2}}{v_{4}^{1}}-{v_{5}^{1}}{v_{4}^{2}}\frac{x_{1}}{x_{2}}<0 $$

To see this, we first determine the (finite) time t ^∗ at which the stock of pollution reaches its steady state value, i.e. $M\left (t\right ) =M^{\ast }$ (see Fig. 1). In such a case, it must be that

$$a_{1}{v_{4}^{1}}\exp (x_{1}t^{\ast} )+a_{2}{v_{4}^{2}}\exp (x_{2}t^{\ast} )=0. $$

Substituting a ₁ and a ₂ from Eq. 41, we get

$$\frac{\left( M\left( 0\right) -M^{\ast} \right)} {\Delta} {v_{5}^{2}}{v_{4}^{1}}\exp (x_{1}t^{\ast} )-\frac{\left( M\left( 0\right) -M^{\ast} \right)} {\Delta} {v_{5}^{1}}{v_{4}^{2}}\exp (x_{2}t^{\ast} )=0 $$

Rearranging and simplifying,

$$\exp (x_{1}t^{\ast} )\left( {v_{5}^{2}}{v_{4}^{1}}-{v_{5}^{1}}{v_{4}^{2}}\exp (\left( x_{2}-x_{1}\right) t^{\ast} )\right) =0 $$

we can solve for t ^∗

$$t^{\ast} =\frac{\ln \left( \frac{{v_{5}^{2}}{v_{4}^{1}}}{{v_{5}^{1}}{v_{4}^{2}}} \right)} {x_{2}-x_{1}} $$

In order that t ^∗ be positive, it must be that $\frac { {v_{5}^{2}}{v_{4}^{1}}}{{v_{5}^{1}}{v_{4}^{2}}}>1$.

Next, we determine the time t _m at which $M\left (t\right )$ reaches a minimum. The first-order condition is

$$M^{\prime} \left( t\right) =x_{1}a_{1}{v_{4}^{1}}\exp (x_{1}t_{m})+x_{2}a_{2}{v_{4}^{2}}\exp (x_{2}t_{m})=0 $$

Again, substituting a ₁ and a ₂ from Eq. 41 and simplifying, we obtain

$$\frac{x_{1}}{x_{2}}=\frac{{v_{5}^{1}}{v_{4}^{2}}}{{v_{5}^{2}}{v_{4}^{1}}}\exp (\left( x_{2}-x_{1}\right) t_{m}) $$

whence

$$t_{m}=\frac{\ln \left( \frac{x_{1}}{x_{2}}\right) -\ln \left( \frac{ {v_{5}^{1}}{v_{4}^{2}}}{{v_{5}^{2}}{v_{4}^{1}}}\right)} {x_{2}-x_{1}} $$

The second-order condition requires that

$$M^{\prime \prime} \left( t\right) =\left( x_{1}\right)^{2}a_{1}{v_{4}^{1}}\exp (x_{1}t_{m})+\left( x_{2}\right) ^{2}a_{2}{v_{4}^{2}}\exp (x_{2}t_{m})>0 $$

or, after some algebra,

$$M^{\prime \prime} \left( t\right) =\frac{\left( M\left( 0\right) -M^{\ast} \right)} {\Delta} {v_{5}^{2}}{v_{4}^{1}}\left( \frac{x_{1}}{x_{2}}\frac{ {v_{5}^{1}}{v_{4}^{2}}}{{v_{5}^{2}}{v_{4}^{1}}}\right)^{\frac{x_{1}}{x_{2}-x_{1}}} x_{1}\left( x_{1}-x_{2}\right) >0 $$

To be satisfied, the second-order condition requires that ${v_{5}^{2}}{v_{4}^{1}}$ be positive. This is because the product $x_{1}\left (x_{1}-x_{2}\right )$ is positive. If we evaluate the stock of pollution at t _m , i.e. at its minimum, we get

$$ M\left( t_{m}\right) =\frac{\left( M\left( 0\right) -M^{\ast} \right)} { {\Delta}} \left( \frac{x_{1}}{x_{2}}\frac{{v_{5}^{1}}{v_{4}^{2}}}{ {v_{5}^{2}}{v_{4}^{1}}}\right)^{\frac{x_{1}}{x_{2}-x_{1}}}\left( {v_{5}^{2}}{v_{4}^{1}}-\frac{x_{1}}{x_{2}}{v_{5}^{1}}{v_{4}^{2}}\right) +M^{\ast} $$

(44)

Notice that this expression is very similar to $M\left (\hat {t}\right )$ of Eq. 43. There is just one difference: the second term on the right-hand side is multiplied by $\frac {{v_{5}^{1}}{v_{4}^{2}}}{ {v_{5}^{2}}{v_{4}^{1}}}$, which as seen above is greater than 1. How can we know that the last term in parentheses is negative? Intuition suggests that if there is a finite time t ^∗ at which $M\left (t\right )$ equals its steady state value, the minimum stock of pollution should be less than that.

Formally, this requires that at t ^∗ the stock of pollution is decreasing, or that

$$M^{\prime} \left( t^{\ast} \right) =a_{1}{v_{4}^{1}}\exp (x_{1}t^{\ast} )+a_{2}{v_{4}^{2}}\exp (x_{2}t^{\ast} )<0 $$

After substitution and some simplification, this condition becomes

$$\frac{\left( M\left( 0\right) -M^{\ast} \right)} {\Delta} \left( \frac{ {v_{5}^{2}}{v_{4}^{1}}}{{v_{5}^{1}}{v_{4}^{2}}}\right)^{\frac{x_{1}}{x_{2}-x_{1}}} {v_{5}^{2}}{v_{4}^{1}}\left( x_{1}-x_{2}\right) <0 $$

This condition is satisfied since x ₁ − x ₂ < 0 while, as seen above, ${v_{5}^{2}}{v_{4}^{1}}$ is positive.

Thus, at point such as D in Fig. 1 the stock of pollution is always greater than at the steady state.