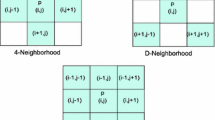
Abstract
Grayscale skeletonization offers an interesting alternative to traditional skeletonization following a binarization. It is well known that parallel algorithms for skeletonization outperform sequential ones in terms of quality of results, yet no general and well defined framework has been proposed until now for parallel grayscale thinning. We introduce in this paper a parallel thinning algorithm for grayscale images, and prove its topological soundness based on properties of the critical kernels framework. The algorithm and its proof, given here in the 2D case, are also valid in 3D. Some applications are sketched in conclusion.
Chapter PDF
Similar content being viewed by others
Keywords
These keywords were added by machine and not by the authors. This process is experimental and the keywords may be updated as the learning algorithm improves.
References
Abeysinghe, S.S., Baker, M., Chiu, W., Ju, T.: Segmentation-free skeletonization of grayscale volumes for shape understanding. In: IEEE International Conference on Shape Modeling and Applications, SMI 2008, pp. 63–71 (2008)
Arcelli, C., Ramella, G.: Finding grey-skeletons by iterated pixel removal. Image and Vision Computing 13(3), 159–167 (1995)
Bertrand, G.: On critical kernels. Comptes Rendus de l’Académie des Sciences, Série Math. I(345), 363–367 (2007)
Bertrand, G., Couprie, M.: Two-dimensional thinning algorithms based on critical kernels. J. of Mathematical Imaging and Vision 31(1), 35–56 (2008)
Bertrand, G., Couprie, M.: Powerful parallel and symmetric 3d thinning schemes based on critical kernels. J. of Mathematical Imaging and Vision (2012) (to appear), doi:10.1007/s10851-012-0402-7
Bertrand, G., Everat, J.C., Couprie, M.: Topological approach to image segmentation. In: SPIE Vision Geometry V, vol. 2826, pp. 65–76 (1996)
Bertrand, G., Everat, J.C., Couprie, M.: Image segmentation through operators based upon topology. J. of Electronic Imaging 6(4), 395–405 (1997)
Beucher, S.: Segmentation d’images et morphologie mathématique. PhD thesis, École des Mines de Paris, France (1990)
Costes, C., Garello, R., Mercier, G., Artis, J.-P., Bon, N.: Convective clouds modelling and tracking by an airborne radar. In: OCEANS 2008, pp. 1–5 (September 2008)
Couprie, M., Bezerra, F.N., Bertrand, G.: Topological operators for grayscale image processing. J. of Electronic Imaging 10(4), 1003–1015 (2001)
Dyer, C., Rosenfeld, A.: Thinning algorithms for gray-scale pictures. IEEE Trans. on Pattern An. and Machine Int. 1(1), 88–89 (1979)
Jang, J.-H., Hong, K.-S.: A pseudo-distance map for the segmentation-free skeletonization of gray-scale images. In: Proceedings of the Eighth IEEE International Conference on Computer Vision, ICCV 2001, vol. 2, pp. 18–23 (2001)
Kang, K.W., Suh, J.W., Kim, J.H.: Skeletonization of grayscale character images using pixel superiority index. In: IAPR Workshop on Document Analysis Systems (1998)
Kong, T.Y.: On topology preservation in 2D and 3D thinning. Int. J. on Pattern Recognition and Artificial Intelligence 9, 813–844 (1995)
Kong, T.Y., Rosenfeld, A.: Digital topology: introduction and survey. Computer Vision, Graphics and Image Processing 48, 357–393 (1989)
Le Bourgeois, F., Emptoz, H.: Skeletonization by gradient diffusion and regularization. In: IEEE International Conference on Image Processing, ICIP 2007, vol. 3, pp. 33–36 (2007)
Lohou, C., Bertrand, G.: New parallel thinning algorithms for 2d grayscale images. In: Latecki, L.J., Mount, D.M., Wu, A.Y. (eds.) Society of Photo-Optical Instrumentation Engineers (SPIE) Conference Series, vol. 4117, pp. 58–69 (2000)
Mersa, S.S., Darwish, A.M.: A new parallel thinning algorithm for gray scale images. In: IEEE Nonlinear Signal and Image Proc. Conf., pp. 409–413 (1999)
Nedzved, A., Uchida, S., Ablameyko, S.: Gray-scale thinning by using a pseudo-distance map. In: 18th International Conference on Pattern Recognition, ICPR 2006, vol. 2, pp. 239–242 (2006)
Pal, S.K.: Fuzzy skeletonization of an image. Pattern Recognition Letters 10, 17–23 (1989)
Ranwez, V., Soille, P.: Order independent homotopic thinning for binary and grey tone anchored skeletons. Pattern Recognition Letters 23(6), 687–702 (2002); Discrete Geometry for Computer Imagery
Rosenfeld, A.: The fuzzy geometry of image subsets. Pattern Recognition Letters 2, 311–317 (1984)
Saleh, A.M., Bahaa Eldin, A.M., Wahdan, A.-M.A.: A modified thinning algorithm for fingerprint identification systems. In: International Conference on Computer Engineering Systems, ICCES 2009, pp. 371–376 (2009)
Whitehead, J.H.C.: Simplicial spaces, nuclei and m-groups. Proceedings of the London Mathematical Society 45(2), 243–327 (1939)
Yu, S.-S., Tsai, W.-H.: A new thinning algorithm for gray-scale images by the relaxation technique. Pattern Recognition 23(10), 1067–1076 (1990)
Author information
Authors and Affiliations
Editor information
Editors and Affiliations
Rights and permissions
Copyright information
© 2013 Springer-Verlag Berlin Heidelberg
About this paper
Cite this paper
Couprie, M., Bezerra, N., Bertrand, G. (2013). A Parallel Thinning Algorithm for Grayscale Images. In: Gonzalez-Diaz, R., Jimenez, MJ., Medrano, B. (eds) Discrete Geometry for Computer Imagery. DGCI 2013. Lecture Notes in Computer Science, vol 7749. Springer, Berlin, Heidelberg. https://doi.org/10.1007/978-3-642-37067-0_7
Download citation
DOI: https://doi.org/10.1007/978-3-642-37067-0_7
Publisher Name: Springer, Berlin, Heidelberg
Print ISBN: 978-3-642-37066-3
Online ISBN: 978-3-642-37067-0
eBook Packages: Computer ScienceComputer Science (R0)