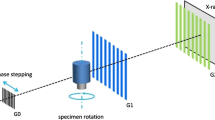
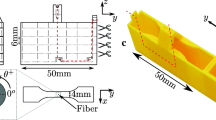
Abstract
The potential of full-field low coherence interferometric techniques for imaging internal structures, such as fibers, interfaces, or inclusions in technical materials is demonstrated by our coherence probe microscopy (CPM) setup. However, the huge amount of recorded data demand for an automatized enhancement and evaluation of the image data. We propose an automatic image analysis procedure adapted for full-field coherence probe microscopy, which we tested on fiber composite materials. The performed image enhancement and orientation analysis finally allow to cluster the internal fiber structures, to detect outliers and enable an improved characterization of investigated specimens supporting a sophisticated material design for the future.
Chapter PDF
Similar content being viewed by others
References
Drexler, W., Fujimoto, J.G.: Optical coherence tomography. Springer, Berlin (2008)
Dubois, A., Vabre, L., Boccara, A., Beaurepaire, E.: High-resolution full-field optical coherence tomography with a Linnik microscope. Appl. Opt. 41, 805–812 (2002)
Stifter, D.: Beyond biomedicine: a review of alternative applications and developments for optical coherence tomography. Appl. Phys. 88, 337–357 (2007)
Latour, G., Echard, J.P., Soulier, B., Emond, I., Vaiedelich, S., Elias, M.: Structural and optical properties of wood and wood finishes studied using optical coherence tomography. Appl. Optics 48, 6485–6491 (2009)
Wiesauer, K., Pircher, M., Gotzinger, E., Hitzenberger, C.K., Engelke, R., Ahrens, G., Gruetzner, G., Stifter, D.: Transversal ultrahigh-resolution polarization-sensitive optical coherence tomography for strain mapping in materials. Optics Express 14, 5945–5953 (2006)
Schausberger, S.E., Heise, B., Maurer, C., Bernet, S., Ritsch-Marte, M., Stifter, D.: Flexible contrast for low-coherence interference microscopy by Fourier-plane filtering with a SLM. Opt. Lett. 35, 4154–4156 (2010)
Stifter, D., Leiss-Holzinger, E., Major, Z., Baumann, B., Pircher, M., Götzinger, E., Hitzenberger, C.K., Heise, B.: Dynamic optical studies in materials testing with spectral-domain polarization-sensitive OCT. Opt. Express 18, 25712–25725 (2010)
Gallagher, N.C., Wise, G.L.: Median filters: a tutorial. In: Proc. IEEE ISCAS, vol. 88, pp. 1737–1744 (1988)
Candes, E.J., Demanet, L., Donoho, D.L., Ying, L.: Fast Discrete Curvelet Transform (2005)
Shensa, M.J.: The discrete wavelet transform: Wedding the Á trous and Mallat algorithms. IEEE Trans. Signal Proc. 40, 2464–2482 (1992)
Sternberg, S.R.: Biomedical image processing. Computer 16, 22–34 (1983)
Held, S., Storath, M., Massopust, P., Forster, B.: Steerable wavelet frames based on the Riesz transform. IEEE Trans. Image Proc. 19, 653–667 (2010) ; Monogenic wavelet toolbox, http://www.mamebia.de/Software
Jähne, B.: Digital Image Processing. Springer, Heidelberg (2002)
Movellan, J.R.: Tutorial on Gabor Filters
Unser, M., Sage, D., Ville, D.V.D.: Multiresolution monogenic signal analysis using the Riesz-Laplace wavelet transform. IEEE Trans. Image Proc. 18, 2402–2418 (2009); MonogenicJ toolbox, http://bigwww.epfl.ch/demo/monogenic/
Felsberg, M., Sommer, G.: The monogenic signal. IEEE Trans. Image Proc. 49, 3136–3144 (2001)
Author information
Authors and Affiliations
Editor information
Editors and Affiliations
Rights and permissions
Copyright information
© 2011 Springer-Verlag Berlin Heidelberg
About this paper
Cite this paper
Schlager, V., Schausberger, S.E., Stifter, D., Heise, B. (2011). Coherence Probe Microscopy Imaging and Analysis for Fiber-Reinforced Polymers. In: Heyden, A., Kahl, F. (eds) Image Analysis. SCIA 2011. Lecture Notes in Computer Science, vol 6688. Springer, Berlin, Heidelberg. https://doi.org/10.1007/978-3-642-21227-7_40
Download citation
DOI: https://doi.org/10.1007/978-3-642-21227-7_40
Publisher Name: Springer, Berlin, Heidelberg
Print ISBN: 978-3-642-21226-0
Online ISBN: 978-3-642-21227-7
eBook Packages: Computer ScienceComputer Science (R0)