Abstract
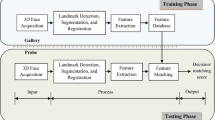
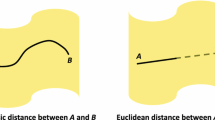
Stemming from a sound mathematical framework dating back to the beginning of the 20th century, this paper introduces a novel approach for 3D face recognition. The proposed technique is based on joint differential invariants, projecting a 3D shape in a 9-dimensional space where the effect of rotation and translation is removed. As a consequence, the matching between two different 3D samples can be directly performed in the invariant space. Thus the matching score can be effectively used to detect surfaces or parts of surfaces characterised by similar when not identical 3D structure. The paper details an efficient procedure for the generation of the invariant signature in the 9-dimensional space, carefully discussing a number of significant implications related to the application of the mathematical framework to the discrete, non-rigid case of interest. Experimental evaluation of the proposed approach is performed over the widely known 3D_RMA database, comparing results to the well established Iterative Closest Point (ICP)-based matching approach.
Chapter PDF
Similar content being viewed by others
References
Bowyer, K., Chang, K., Flynn, P.: A survey of approaches and challenges in 3d and multi-modal 2D+3D face recognition. Computer Vision and Image Understanding 101(1), 1–15 (2006)
Chang, K., Bowyer, K., Flynn, P.: An evaluation of multimodal 2d+3d face biometrics. IEEE Trans. Pattern Anal. Mach. Intell. 27(4), 619–624 (2005)
Gokberk, B., Akarun, L.: Comparative analysis of decision-level fusion algorithms for 3d face recognition. In: Proc. of Int. Conf. on Pattern Recognition, pp. 1018–1021 (2006)
Besl, P., McKay, N.: A method for registration of 3D shapes. IEEE Trans. on Pattern Analysis and Machine Intelligence 14, 239–256 (1992)
Chang, K., Bowyer, K., Flynn, P.: Adaptive rigid miltiregion selection for handling expression variations in 3D face recognition. In: IEEE Workshop on FRGC (2005)
Irfanoglu, M., Gokberk, B., Akarun, L.: 3D shape-based face recognition using automatically registered facial surfaces. In: Proc. of Int. Conf. on Pattern Recognition, pp. 183–186 (2004)
Lu, X., Jain, A.: Deformation analysis for 3d face matching. In: Proc. Int. Workshop on Applications of Computer Vision, pp. 99–104 (2005)
Lu, X., Jain, A., Colbry, D.: Matching 2.5D Face Scans to 3D Models. IEEE Trans. on Pattern Analysis and Machine Intelligence 28, 31–43 (2006)
Beumier, C., Acheroy, M.: Automatic 3d face authentication. Image and Vision Computing 18(4), 315–321 (2000)
Olver, P.J.: Joint Invariants Signatures. Found. Comput. Math. 1, 3–67 (2001)
Olver, P.J.: A survey of moving frames. In: Li, H., J. Olver, P., Sommer, G. (eds.) IWMM-GIAE 2004. LNCS, vol. 3519, pp. 105–138. Springer, Heidelberg (2005)
Cartan, É.: La Méthode du Repère Mobile, la Théorie des Groups Continue, et les Espaces Géneralisés. Hermann, Paris, France (1935)
Cadoni, M.I., Chimienti, A., Nerino, R.: Automatic Coarse Registration by Invariant Features. In: The 7th International Symposium on Virtual Reality, Archaeology and Cultural Heritage VAST (2006)
Do Carmo, M.: Differential Geometry of Curves and Surfaces. Prentice-Hall, Englewood Cliffs (1976)
de Berg, M., van Kreveld, M., Overmars, M., Schwarzkopf, O.: Computational Geometry, 2nd revised edn., pp. 99–105. Springer, Heidelberg (2000)
Author information
Authors and Affiliations
Editor information
Editors and Affiliations
Rights and permissions
Copyright information
© 2009 Springer-Verlag Berlin Heidelberg
About this paper
Cite this paper
Cadoni, M., Bicego, M., Grosso, E. (2009). 3D Face Recognition Using Joint Differential Invariants. In: Tistarelli, M., Nixon, M.S. (eds) Advances in Biometrics. ICB 2009. Lecture Notes in Computer Science, vol 5558. Springer, Berlin, Heidelberg. https://doi.org/10.1007/978-3-642-01793-3_29
Download citation
DOI: https://doi.org/10.1007/978-3-642-01793-3_29
Publisher Name: Springer, Berlin, Heidelberg
Print ISBN: 978-3-642-01792-6
Online ISBN: 978-3-642-01793-3
eBook Packages: Computer ScienceComputer Science (R0)