Abstract
In the introduction of this paper we discuss a possible approach to the unitarizability problem for classical p-adic groups. In this paper we give some very limited support that such approach is not without chance. In a forthcoming paper we shall give additional evidence in generalized cuspidal rank (up to) three.
Access this chapter
Tax calculation will be finalised at checkout
Purchases are for personal use only
Notes
- 1.
For \(\delta \in D(\mathcal A)_u\) denote by \(\nu _\delta :=\nu ^{s_\delta }\), where s δ is the smallest non-negative number such that \(\nu ^{s_\delta }\delta \times \delta \) reduces. Introduce u(δ, n) in the same way as above, except that we use ν δ in the definition of u(δ, n) instead of ν. Then the expected answer is the same as in the Theorem 1.1, except that one replaces ν by ν δ in the definition of \(B(\mathcal A)\).
- 2.
In the field case it reduces if and only if ρ′≅ν ±1 ρ.
- 3.
By classical groups we mean symplectic, orthogonal, and unitary groups (see the following sections for more details). In this introduction and in the most of the paper we shall deal with symplectic and orthogonal groups. The case of unitary groups is discussed in the last section of the paper.
- 4.
It is there denoted by E 1,2.
- 5.
- 6.
As we already noted, this is known to hold if char(F) = 0.
- 7.
- 8.
- 9.
These are irreducible representations which become square integrable modulo center after twist by a (not necessarily unitary) character of the group.
- 10.
One can find in [53] matrix realizations of the symplectic and split odd-orthogonal groups. In a similar way one can make matrix realizations also for other orthogonal groups (and for unitary groups which are discussed a little bit later).
- 11.
It is easy to see that Langlands parameter of γ must be of above form. Namely, for the beginning, the tempered piece of the Langlands parameter must be square integrable (this follows from the fact that ρ is self-contragredient and the fact that
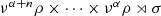 is a regular representations, i.e. all the Jacquet modules of it are multiplicity one representations). Further, one directly sees that this square integrable representation must be some δ( Δk+1; σ). Now considering the support, and using the fact that \(\mathfrak c(\Delta _i)>0\), we get that the Langlands parameter of γ must be of the above form.
is a regular representations, i.e. all the Jacquet modules of it are multiplicity one representations). Further, one directly sees that this square integrable representation must be some δ( Δk+1; σ). Now considering the support, and using the fact that \(\mathfrak c(\Delta _i)>0\), we get that the Langlands parameter of γ must be of the above form. - 12.
- 13.
Recall, \(\mathcal C\) is the set of all irreducible cuspidal representations of general linear groups.
- 14.
Then X 2 is also self-contragredient.
- 15.
Clearly, Ξ does not need to be irreducible.
- 16.
- 17.
As we already noted, this is known if char(F) = 0.
- 18.
References
Arthur, J., The Endoscopic Classification of Representations: Orthogonal and Symplectic Groups, American Mathematical Society Colloquium Publications 61, American Mathematical Society, Providence, RI, 2013.
Aubert, A. M., Dualité dans le groupe de Grothendieck de la catégorie des représentations lisses de longueur finie d’un groupe réductif p-adique, Trans. Amer. Math. Soc. 347 (1995), 2179–2189; Erratum, Trans. Amer. Math. Soc 348 (1996), 4687–4690.
Badulescu, A. I., On p-adic Speh representations, Bull. Soc. Math. France 142 (2014), 255–267.
Badulescu, A. I.; Henniart, G.; Lemaire, B. and Sécherre, V., Sur le dual unitaire de GL r(D), Amer. J. Math. 132 (2010), no. 5, 1365–1396.
Badulescu, A. I. and Renard, D. A., Sur une conjecture de Tadić, Glasnik Mat. 39 no. 1 (2004), 49–54.
Badulescu, A. I. and Renard, D. A., Unitary dual of GL n at archimedean places and global Jacquet-Langlands correspondence, Compositio Math. 146, vol. 5 (2010), 1115–1164.
Baruch, D., A proof of Kirillov’s conjecture, Ann. of Math. (2) 158, no. 1 (2003), 207–252.
Bernstein, J., P-invariant distributions on GL(N) and the classification of unitary representations of GL(N) (non-archimedean case), Lie Group Representations II, Lecture Notes in Math. 1041, Springer-Verlag, Berlin, 1984, 50–102.
Borel, A. and Wallach, N., Continuous cohomology, discrete subgroups, and representations of reductive groups, Annals of Mathematics Studies, vol. 94, Princeton University Press, Princeton, N.J.; University of Tokyo Press, Tokyo, 1980.
Casselman, W., Introduction to the theory of admissible representations of p-adic reductive groups, preprint (http://www.math.ubc.ca/~cass/research/pdf/p-adic-book.pdf).
Casselman, W., A new nonunitarity argument for p-adic representations, J. Fac. Sci. Univ. Tokyo Sect. IA Math. 28, no. 3 (1981), 907–928 (1982).
Gelfand, I. M. and Naimark, M. A., Unitary representations of semi simple Lie groups 1, Unitary representations of complex unimodular group, M. Sbornik 21 (1947), 405–434.
Gelfand, I. M. and Naimark, M. A., Unitäre Darstellungen der Klassischen Gruppen (German translation of Russian publication from 1950), Akademie Verlag, Berlin, 1957.
Hanzer, M., The unitarizability of the Aubert dual of the strongly positive discrete series, Israel J. Math. 169 (2009), no. 1, 251–294.
Hanzer, M. and Tadić, M., A method of proving non-unitarity of representations of p-adic groups I, Math. Z. 265 (2010), no. 4, 799–816.
Hanzer, M. and Jantzen, C., A method of proving non-unitarity of representations of p-adic groups, J. Lie Theory 22 (2012), no. 4, 1109–1124.
Harris, M. and Taylor, R., On the geometry and cohomology of some simple Shimura varieties, Princeton University Press, Annals of Math. Studies 151, 2001.
Henniart, G., Une preuve simple des conjectures de Langlands pour GL(n) sur un corps p-adique, Invent. Math. 139 (2000), 439–455.
Howe, R. and Moore, C., Asymptotic properties of unitary representations, J. Funct. Anal. 32 (1979), no. 1, 72–96.
Jacquet, H., On the residual spectrum of GL(n), Lie Group Representations II, Lecture Notes in Math. 1041, Springer-Verlag, Berlin, 1984, 185–208.
Jantzen, C., On supports of induced representations for symplectic and odd-orthogonal groups, Amer. J. Math. 119 (1997), 1213–1262.
Jantzen, C., Discrete series for p-adic SO(2n) and restrictions of representations of O(2n), Canad. J. Math. 63, no. 2 (2011), 327–380.
Jantzen, C., Tempered representations for classical p-adic groups, Manuscripta Math. 145 (2014), no. 3–4, 319–387.
Kazhdan, D., Connection of the dual space of a group with the structure of its closed subgroups, Functional Anal. Appl. 1 (1967), 63–65.
Kirillov, A. A., Infinite dimensional representations of the general linear group, Dokl. Akad. Nauk SSSR 114 (1962), 37–39; Soviet Math. Dokl. 3 (1962), 652–655.
Konno, T., A note on the Langlands classification and irreducibility of induced representations of p-adic groups, Kyushu J. Math. 57 (2003), no. 2, 383–409.
Kudla, S. S., Notes on the local theta correspondence, lectures at the European School in Group Theory, 1996, preprint (http://www.math.toronto.edu/~skudla/castle.pdf).
Lapid, E. and Mínguez, A., On parabolic induction on inner forms of the general linear group over a non-archimedean local field, Selecta Math. (N.S.) to appear, arXiv:1411.6310.
Lapid, E., Muić, G. and Tadić, M., On the generic unitary dual of quasisplit classical groups, Int. Math. Res. Not. no. 26 (2004), 1335–1354.
Laumon, G., Rapoport, M. and Stuhler, U., P-elliptic sheaves and the Langlands correspondence, Invent. Math. 113 (1993), 217–338.
Matić, I. and Tadić, M., On Jacquet modules of representations of segment type, Manuscripta Math. 147 (2015), no. 3–4, 437–476.
Mautner, F., Spherical functions over p-adic fields I, Amer. J. Math. 80 (1958). 441–457.
Mœglin, C., Sur certains paquets d’Arthur et involution d’Aubert-Schneider-Stuhler généralisée, Represent. Theory 10 (2006), 86–129.
Mœglin, C., Multiplicité 1 dans les paquets d’Arthur aux places p-adiques, in “On certain L-functions”, Clay Math. Proc. 13 (2011), 333–374.
Mœglin, C., Paquets stables des séries discrètes accessibles par endoscopie tordue; leur paramètre de Langlands, Contemp. Math. 614 (2014), pp. 295–336.
Mœglin, C. and Renard, D., Paquet d’Arthur des groupes classiques non quasi-déployś, preprint.
Mœglin, C. and Tadić, M., Construction of discrete series for classical p-adic groups, J. Amer. Math. Soc. 15 (2002), 715–786.
Mœglin, C. and Waldspurger, J.-L., Sur le transfert des traces tordues d’un group linéaire à un groupe classique p-adique, Selecta mathematica 12 (2006), pp. 433–516.
Muić, G. and Tadić, M., Unramified unitary duals for split classical p–adic groups; the topology and isolated representations, in “On Certain L-functions”, Clay Math. Proc. vol. 13, 2011, 375–438.
Renard, D., Représentations des groupes réductifs p-adiques, Cours Spécialisés 17, Société Mathématique de France, Paris, 2010.
Rodier, F., Whittaker models for admissible representations, Proc. Sympos. Pure Math. AMS 26 (1983), pp. 425–430.
Schneider, P. and Stuhler, U., Representation theory and sheaves on the Bruhat-Tits building, Publ. Math. IHES 85 (1997), 97–191.
Sécherre, V., Proof of the Tadić conjecture (U0) on the unitary dual of GL m(D), J. reine angew. Math. 626 (2009), 187–203.
Silberger, A., The Langlands quotient theorem for p-adic groups, Math. Ann. 236 (1978), no. 2, 95–104.
Silberger, A., Special representations of reductive p-adic groups are not integrable, Ann. of Math. 111 (1980), 571–587.
Speh, B., Unitary representations of \(GL(n, \mathbb R)\) with non-trivial (g, K)- cohomology, Invent. Math. 71 (1983), 443–465.
Tadić, M., Unitary representations of general linear group over real and complex field, preprint MPI/SFB 85–22 Bonn (1985), (http://www.mpim-bonn.mpg.de/preblob/5395).
Tadić M. Unitary dual of p-adic GL(n), Proof of Bernstein Conjectures, Bulletin Amer. Math. Soc. 13 (1985), 39–42.
Tadić, M., Classification of unitary representations in irreducible representations of general linear group (non-archimedean case), Ann. Sci. École Norm. Sup. 19 (1986), 335–382.
Tadić, M., Induced representations of GL(n, A) for p-adic division algebras A, J. reine angew. Math. 405 (1990), 48–77.
Tadić, M., An external approach to unitary representations, Bull. Amer. Math. Soc. (N.S.) 28, no. 2 (1993), 215–252.
Tadić, M., Representations of p-adic symplectic groups, Compositio Math. 90 (1994), 123–181.
Tadić, M., Structure arising from induction and Jacquet modules of representations of classical p-adic groups, J. of Algebra 177 (1995), 1–33.
Tadić, M. On regular square integrable representations of p-adic groups, Amer. J. Math. 120, no. 1 (1998), 159–210.
Tadić, M., On reducibility of parabolic induction, Israel J. Math. 107 (1998), 29–91.
Tadić, M., Square integrable representations of classical p-adic groups corresponding to segments, Represent. Theory 3 (1999), 58–89.
Tadić, M., On classification of some classes of irreducible representations of classical groups, in book Representations of real and p-adic groups, Singapore University Press and World Scientific, Singapore, 2004, 95–162.
Tadić, M., \(GL(n,\mathbb C)\hat {\ }\) and \(G L(n,\mathbb R)\hat {\ }\), in “Automorphic Forms and L-functions II, Local Aspects”, Contemp. Math. 489 (2009), 285–313.
Tadić, M., On reducibility and unitarizability for classical p-adic groups, some general results, Canad. J. Math. 61 (2009), 427–450.
Tadić, M., On automorphic duals and isolated representations; new phenomena, J. Ramanujan Math. Soc. 25, no. 3 (2010), 295–328.
Tadić, M., On tempered and square integrable representations of classical p-adic groups, Sci. China Math., 56 (2013), 2273–2313.
Tadić, M., Remark on representation theory of general linear groups over a non-archimedean local division algebra, Rad Hrvat. Akad. Znan. Umjet. Mat. Znan. 19(523) (2015), 27–53.
Vogan, D. A., The unitary dual of GL(n) over an archimedean field, Invent. Math. 82 (1986), 449–505.
Zelevinsky, A. V., Induced representations of reductive p-adic groups II. On irreducible representations of GL(n), Ann. Sci. École Norm. Sup. 13 (1980), 165–210.
Acknowledgements
This work has been supported by Croatian Science Foundation under the project 9364.
Author information
Authors and Affiliations
Corresponding author
Editor information
Editors and Affiliations
Rights and permissions
Copyright information
© 2018 Springer International Publishing AG, part of Springer Nature
About this paper
Cite this paper
Tadić, M. (2018). On Unitarizability in the Case of Classical p-Adic Groups. In: Müller, W., Shin, S., Templier, N. (eds) Geometric Aspects of the Trace Formula. SSTF 2016. Simons Symposia. Springer, Cham. https://doi.org/10.1007/978-3-319-94833-1_13
Download citation
DOI: https://doi.org/10.1007/978-3-319-94833-1_13
Published:
Publisher Name: Springer, Cham
Print ISBN: 978-3-319-94832-4
Online ISBN: 978-3-319-94833-1
eBook Packages: Mathematics and StatisticsMathematics and Statistics (R0)


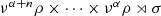 is a regular representations, i.e. all the Jacquet modules of it are multiplicity one representations). Further, one directly sees that this square integrable representation must be some δ( Δk+1; σ). Now considering the support, and using the fact that
is a regular representations, i.e. all the Jacquet modules of it are multiplicity one representations). Further, one directly sees that this square integrable representation must be some δ( Δk+1; σ). Now considering the support, and using the fact that