Abstract
This chapter formulates a flight optimization problem to characterize the optimal flight of a jet aircraft subject to mission constraints and constraints that arise from the basic physics of flight of the aircraft. A hierarchical optimization approach is suggested: a flight plan is first developed, specified by way points and segments of helical paths between these waypoints, that meet the mission constraints; then an optimal steady flight problem is formulated and solved for each of the helical flight path segments. The required background in flight physics is summarized and optimal flight conditions for several categories of steady flight are described. Finally, a specific fuel optimal flight optimization problem for an example of a jet aircraft is introduced as a case study. The hierarchical optimization approach is followed and a fuel optimal flight plan is obtained and its flight performance is analyzed.
This is a preview of subscription content, log in via an institution to check access.
Access this chapter
Tax calculation will be finalised at checkout
Purchases are for personal use only
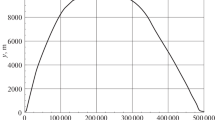
Similar content being viewed by others
References
Anderson, J. D. (2000). Introduction to flight (4th edn.) Boston: McGraw-Hill.
Betts, J. (2001). Practical methods for optimal control using nonlinear programming, advances in design and control. Philadelphia: SIAM.
Bryson, A., & Ho, Y. C. (1975). Applied optimal control. Washington, DC: Hemisphere.
DIDO optimal control software. Mathworks. http://www.mathworks.com/products/connections/product_detail/product_61633.html.
MATLAB optimization toolbox. Mathworks. http://www.mathworks.com/help/optim/.
McClamroch, N. H. (2011). Steady aircraft flight and performance. Princeton: Princeton University Press.
Ross, I. M., & D’Souza, C. N. (2005). A hybrid optimal control framework for mission planning. Journal of Guidance, Control and Dynamics, 28(4), 686–697.
Author information
Authors and Affiliations
Corresponding author
Editor information
Editors and Affiliations
1 Electronic supplementary material
Rights and permissions
Copyright information
© 2015 Springer Science+Business Media New York
About this chapter
Cite this chapter
McClamroch, H., Lee, T. (2015). Optimal Flight Planning for a Jet Aircraft. In: Murty, K. (eds) Case Studies in Operations Research. International Series in Operations Research & Management Science, vol 212. Springer, New York, NY. https://doi.org/10.1007/978-1-4939-1007-6_15
Download citation
DOI: https://doi.org/10.1007/978-1-4939-1007-6_15
Publisher Name: Springer, New York, NY
Print ISBN: 978-1-4939-1006-9
Online ISBN: 978-1-4939-1007-6
eBook Packages: Business and EconomicsBusiness and Management (R0)