Skip to main content
SpringerLink
Log in

Interferometry in Acoustic-Data Processing Using Extended Antennas. Space–Time Analogy

  • UNDERWATER ACOUSTICS
  • Published:
Physics of Wave Phenomena Aims and scope Submit manuscript

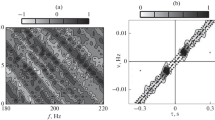
Abstract

A theory of interferometric processing of hydroacoustic data using an extended antenna is presented. An explicit relationship between the 2D spectral density of double Fourier transform of the interference pattern formed by a moving source and the aperture and angular dependence of received field is derived. The gain, antenna directional characteristic, and processing noise immunity are estimated. The results of numerical simulation are presented and discussed. Some ways to adapt the results obtained to the problems of solid-state acoustics using the space–time analogy are proposed and outlined.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1.
Fig. 2.
Fig. 3.
Fig. 4.
Fig. 5.
Fig. 6.

Similar content being viewed by others

REFERENCES

  1. G. N. Kuznetsov, V. M. Kuz’kin, and S. A. Pereselkov, “Spectrogram and localization of a sound source in shallow water,” Acoust. Phys. 63 (4), 449–461 (2017). https://doi.org/10.1134/S1063771017040078

    Article  ADS  Google Scholar 

  2. I. V. Kaznacheev, G. N. Kuznetsov, V. M. Kuz’kin, and S. A Pereselkov, “An interferometric method for detecting a moving sound source with a vector-scalar receiver,” Acoust. Phys. 64 (1), 37–48 (2018). https://doi.org/10.1134/S1063771018010104

    Article  ADS  Google Scholar 

  3. E. S. Kaznacheeva, G. N. Kuznetsov, V. M. Kuz’kin, G. A. Lyakhov, and S. A. Pereselkov, “Measurement capability of the interferometric method of sound source localization in the absence of data on the waveguide transfer function,” Phys. Wave Phenom. 27 (1), 73–78 (2019). https://doi.org/10.3103/S1541308X19010126

    Article  ADS  Google Scholar 

  4. E. S. Kaznacheeva, V. M. Kuz’kin, G. A. Lyakhov, S. A. Pereselkov, and S. A. Tkachenko, “Adaptive algorithms for interferometric processing,” Phys. Wave Phenom. 28 (3), 267–273 (2020). https://doi.org/10.3103/S1541308X20030103

    Article  ADS  Google Scholar 

  5. S. D. Chuprov, Interference Structure of Sound in a Layered Ocean. Ocean Acoustics: Current State (Nauka, Moscow, 1982) [in Russian].

    Google Scholar 

  6. S. A. Akhmanov and R. V. Khokhlov, Problems of Nonlinear Optics (Electromagnetic Waves in Nonlinear Dispersive Media) (Gordon and Breach Sci., New York, 1972).

    Google Scholar 

  7. L. M. Brekhovskikh and Yu. P. Lysanov, Fundamentals of Ocean Acoustics (Springer, 2003).

    MATH  Google Scholar 

  8. G. S. Landsberg, Optics (Nauka, Moscow, 1976) [in Russian].

    Google Scholar 

  9. G. N. Kuznetsov, V. M. Kuz’kin, G. A. Lyakhov, S. A. Pereselkov, and D. Yu. Prosovetskiy, “Direction finding of a noise sound source,” Phys. Wave Phenom. 27 (3), 237–241 (2019). https://doi.org/10.3103/S1541308X19030117

    Article  ADS  Google Scholar 

  10. V. M. Agranovich and V. L. Ginzburg, Crystal Optics with Spatial Dispersion, and Exitons (Interscience, London, 1966).

    Google Scholar 

  11. H. Chen and C. T. Chan, “Acoustic cloaking in three dimensions using acoustic metamaterials,” Appl. Phys. Lett. 91 (18), 183518 (2007). https://doi.org/10.1063/1.2803315

    Article  ADS  Google Scholar 

  12. B.-I. Popa, L. Zigoneanu, and S. A. Cummer, “Experimental acoustic ground cloak in air,” Phys. Rev. Lett. 106 (25), 253901 (2011). https://doi.org/10.1103/PhysRevLett.106.253901

    Article  ADS  Google Scholar 

Download references

Funding

This study was supported in part by the Russian Foundation for Basic Research, project nos. 19-08-00941 and 19-29-06075.

I.V. Kaznacheev acknowledges the support of the President of the Russian Federation (grant no. MK-933.2019.8).

Author information

Authors and Affiliations

  1. Voronezh State University, 394006, Voronezh, Russia

    I. V. Kaznacheev, M. V. Kutsov & S. A. Pereselkov

  2. Prokhorov General Physics Institute of the Russian Academy of Sciences, 119991, Moscow, Russia

    V. M. Kuz’kin & G. A. Lyakhov

Authors
  1. I. V. Kaznacheev
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. V. M. Kuz’kin
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. M. V. Kutsov
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. G. A. Lyakhov
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. S. A. Pereselkov
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to V. M. Kuz’kin.

Additional information

Translated by Yu. Sin’kov

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Kaznacheev, I.V., Kuz’kin, V.M., Kutsov, M.V. et al. Interferometry in Acoustic-Data Processing Using Extended Antennas. Space–Time Analogy. Phys. Wave Phen. 28, 326–332 (2020). https://doi.org/10.3103/S1541308X20040068

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.3103/S1541308X20040068

Search

Navigation