Skip to main content
SpringerLink
Log in

Thermoelastic Characteristics of a Composite with Anisotropic Platelike Inclusions

  • Reliability, Strength, and Wear Resistance of Machines and Structures
  • Published:
Journal of Machinery Manufacture and Reliability Aims and scope Submit manuscript

Abstract

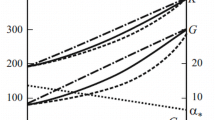
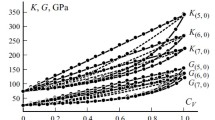
A mathematical model is constructed describing the thermomechanical action of the elements of a composite structure (platelike inclusion and matrix particles) and isotropic elastic medium with the required thermomechanical characteristics. The model is used at the first stage to obtain the matrix relations by the self-consistent method to find the elastic modulus of the composite. At the second stage, it is used to determine the temperature coefficient of linear expansion. Using the variation approach for the composite considered, the two-way estimates of the volumetric elasticity modulus, shearing modulus, and temperature coefficient of linear expansion are determined. The estimated dependences presented allow forecasting the thermoelastic characteristics of the composite, which is reinforced with the anisotropic platelike inclusions (including in the form of nanostructural elements).

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Komkov, M.A. and Tarasov, V.A., Tekhnologiya namotki kompozitnykh konstruktsii raket i sredstv porazheniya (Winding Technology of Composite Structures, and Missile Weapons), Moscow: MGTU im. N.E. Baumana, 2011.

    Google Scholar 

  2. Shermergor, T.D., Teoriya uprugosti mikroneodnorodnykh sred (Theory of Elasticity of Micro-Inhomoheneous Media), Moscow: Nauka, 1977.

    Google Scholar 

  3. Christensen, R.M., Mechanics of Composite Materials, New York: Wiley-Interscience, 1979.

    Google Scholar 

  4. Arzamasov, B.N., Krasheninnikov, A.I., Pastukhova, Zh.P., and Rakhshtadt, A.G., Nauchnye osnovy materialovedeniya (Scientific Principles of Material Science), Moscow: MGTU im. N.E. Baumana, 1994.

    Google Scholar 

  5. Kats, E.A., Fullereny, uglerodnye nanotrubki i nanoklastery. Rodoslovnaya form i idei (Fullerenes, Carbon Nanotubes and Nanoclusters. Genealogy of Forms and Ideas), Moscow: LKI, 2008.

    Google Scholar 

  6. Stankovich, S., Dikin, D.A., Dommett, G.H.B., Kohlhaas, K.M., Zimmey, E.J., Stach, E.A., Piner, R.D., Nguyen, S.T., and Ruoff, R.S., Graphene-based composite materials, Nature (London, U.K.), 2006, vol. 442, pp. 282–286.

    Article  Google Scholar 

  7. Eletskii, A.V., Iskandarova, I.M., Knizhnik, A.A., and Krasikov, D.N., Graphene: fabrication methods and thermophysical properties, Phys. Usp., 2011, vol. 54, no. 3, pp. 227–258.

    Article  Google Scholar 

  8. Berinskii, I.E. and Krivtsov, A.M., On using many-particle interatomic potentials to compute elastic properties of graphene and diamond, Mech. Solids, 2010, vol. 45, no. 6, pp. 815–834.

    Article  Google Scholar 

  9. Zarubin, V.S., Kuvyrkin, G.N., and Savel’eva, I.Yu., Thermal conductivity of the textured composite with anisotropic lamellar inclusions, Kompoz. Nanostrukt., 2015, vol. 7, no. 1, pp. 1–13.

    MATH  Google Scholar 

  10. Eshelby, J.D., The continuum theory of lattice defects, in Progress in Solid State Physics, Seitz, F. and Turnbull, D., Eds., New York: Academic, 1956, vol. 3, pp. 79–303.

    Google Scholar 

  11. Zarubin, V.S. and Kuvyrkin, G.N., Matematicheskie modeli mekhaniki i elektrodinamiki sploshnoi sredy (Mathematical Models of Mechanics and Electrodynamics of Continuous Media), Moscow: MGTU im. N.E. Baumana, 2008.

    Google Scholar 

  12. Zarubin, V.S., Kuvyrkin, G.N., and Savel’eva, I.Yu., Comparative analysis estimates of elastic moduli for composite. Isotropic spherical inclusions, Vestn. MGTU Baumana, Ser. Mashinostr., 2014, no. 5, pp. 53–69.

    Google Scholar 

  13. Golovin, N.N., Zarubin, V.S., and Kuvyrkin, G.N., Mixture models of composite mechanics. 1. Thermal mechanics and thermoelasticity of multicomponent mixture, Vestn. MGTU Baumana, Ser. Estestv. Nauki, 2009, no. 3, pp. 36–49

    Google Scholar 

  14. Frantsevich, I.N., Voronov, F.F., and Bakuta, S.A., Uprugie postoyannye i moduli uprugosti metallov i nemetallov. Spravochnik (Handbook on Elastic Constants and Moduli of Elasticity for Metals and Nonmetals), Kiev: Naukova Dumka, 1982.

    Google Scholar 

  15. Fizicheskie velichiny: Spravochnik (Physical Values, the Handbook), Grigor’ev, I.S. and Melikhov, E.Z., Eds., Moscow: Energoatomizdat, 1991.

Download references

Author information

Authors and Affiliations

  1. Bauman Moscow State Technical University, Moscow, Russia

    V. S. Zarubin, G. N. Kuvyrkin & I. Yu. Savel’eva

Authors
  1. V. S. Zarubin
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. G. N. Kuvyrkin
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. I. Yu. Savel’eva
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to I. Yu. Savel’eva.

Additional information

Original Russian Text © V.S. Zarubin, G.N. Kuvyrkin, I.Yu. Savel’eva, 2018, published in Problemy Mashinostroeniya i Nadezhnosti Mashin, 2018, No. 3, pp. 59–69.

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Zarubin, V.S., Kuvyrkin, G.N. & Savel’eva, I.Y. Thermoelastic Characteristics of a Composite with Anisotropic Platelike Inclusions. J. Mach. Manuf. Reliab. 47, 256–265 (2018). https://doi.org/10.3103/S1052618818030159

Download citation

  • Received:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.3103/S1052618818030159

Search

Navigation