Abstract
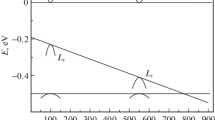
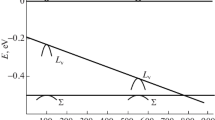
The thermoelectric properties of heavily doped p-type PbTe have been theoretically studied in the temperature range from 300 to 900 K. The calculations are based on a three-band model of the PbTe electron energy spectrum taking into account the transport of electrons and light holes in the L-extrema and heavy holes in the Σ-extrema. The thermoelectric quantities turned out to be very sensitive to the parameters of the heavy-hole band. The best agreement with the experiment is reached at mhh = 5m0 and EgΣ = 0.5 eV, where all calculated thermoelectric quantities agree well with the available experimental data within the entire interval from 300 to 900 K. The calculation confirms a significant increase of the value of the thermoelectric figure-of-merit to ZT = 1.2 that has been recently observed experimentally in heavily doped p-PbTe samples. The maximum of ZT corresponds to the temperature at which the band edges of light and heavy holes coincide in energy so that the steepest singularity in the density of states in the valence band is formed.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
A. Ishida, T, Yamada, D. Cao, et al., J. Appl. Phys. 106, 023718 (2009).
J. Andrulakis, I. Todorov, D.-Y. Chung, et al., Phys. Rev. B 82, 115209 (2010).
Y. Pei, A. LaLonde, S. Iwanga, and G. J. Snyder, Energy Environ. Sci. 4, 2085 (2011).
N. F. Mott and H. Jones, The Theory of the Properties of Metals and Alloys (Clarendon, Oxford, 1936).
N. I. Babenko and A. V. Dmitriev, Moscow Univ. Phys. Bull. (72, 582 (2017)).
A. V. Dmitriev and E. S. Tkacheva, J. Electron. Mater. 43, 1280 (2014).
A. V. Dmitriev and E. S. Tkacheva, Moscow Univ. Phys. Bull. 69, 243 (2014). https://doi.org/10.3103/S0027134914030072
H. Sitter, K. Lishka, and H. Heinrich, Phys. Rev. B 16, 680 (1977).
R. Dornhaus, G. Nimtz, and B. Schlicht, Narrow-Gap Semiconductors (Springer, Berlin, 1983).
Z. Gibbs, H. Kim, H. Wang, et al., Appl. Phys. Lett. 103, 262109 (2013).
R. N. Tauber, A. A. Machonis, and I. B. Cadoff, J. Appl. Phys. 37, 4855 (1966).
Y. Pei, X. Shi, A. LaLonde, et al., Nature 473, 66 (2011).
Author information
Authors and Affiliations
Corresponding author
Additional information
Original Russian Text © N.I. Babenko, A.V. Dmitriev, 2017, published in Vestnik Moskovskogo Universiteta, Seriya 3: Fizika, Astronomiya, 2017, No. 6, pp. 85–88.
About this article
Cite this article
Babenko, N.I., Dmitriev, A.V. The Effect of the Heavy-Hole Band on the Thermoelectric Figure-of-Merit of Heavily Doped p-Type Lead Telluride. Moscow Univ. Phys. 72, 587–590 (2017). https://doi.org/10.3103/S0027134917060030
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.3103/S0027134917060030