Skip to main content
SpringerLink
Log in

Composition-dependent hardness and Young’s modulus in fcc Ni–X (X = Rh, Ta, W, Re, Os, and Ir) alloys: Experimental measurements and CALPHAD modeling

  • Novel Synthesis and Processing of Metals
  • Article
  • Published:
Journal of Materials Research Aims and scope Submit manuscript

Abstract

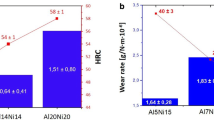
In this paper, the hardness and Young’s moduli along the diffusion paths in fcc Ni–X (X = Rh, Ta, W, Re, Os, and Ir) binary diffusion couples were measured by using the nanoindentation technique. Hardness increases gradually from the pure Ni to the fcc Ni–X alloys, except for the Ni–Os system. While the Young’ moduli in fcc Ni–X alloys scatter much larger and do not show noticeable variation with the addition of element X. After that, the CALPHAD models for description of the composition-dependent hardness and Young’s modulus were proposed, and an in-house code was developed. Based on the present experimental data, the CALPHAD-type descriptions for hardness and Young’s modulus in fcc Ni–X (X = Rh, Ta, W, Re, Os, and Ir) systems were obtained. The model-predicted hardness and Young’s moduli of composition dependence agree with the experimental data in general. It is anticipated that the presently obtained CALPHAD-type hardness and Young’s modulus descriptions, together with the previous thermodynamic and atomic mobility databases, can be used for the future alloy design of novel Ni-based superalloys.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Figure 1
Figure 2
Figure 3
Figure 4
Figure 5
Figure 6

Similar content being viewed by others

References

  1. Ł. Rakoczy, G. Cempura, A. Kruk, A. Czyrska-Filemonowicz, and A. Zielińska-Lipiec: Evolution of γ′ morphology and γ/γ′ lattice parameter misfit in a nickel-based superalloy during non-equilibrium cooling. J. Mater. Res. 110, 66 (2019).

    Article  CAS  Google Scholar 

  2. M. Huo, L. Liu, W.C. Yang, Y.F. Li, S.S. Hu, H.J. Su, J. Zhang, and H.Z. Fu: Formation of low-angle grain boundaries under different solidification conditions in the rejoined platforms of Ni-based single crystal superalloys. J. Mater. Res. 34, 251 (2019).

    Article  CAS  Google Scholar 

  3. W.J. Sun, S. Kothari, and C.C. Sun: The relationship among tensile strength, Young’s modulus, and indentation hardness of pharmaceutical compacts. Powder Technol. 331, 1 (2018).

    Article  CAS  Google Scholar 

  4. M. Liu, J.Y. Lin, C. Lu, K.A. Tieu, K. Zhou, and T. Koseki: Progress in indentation study of materials via both experimental and numerical methods. Crystals 7, 258 (2017).

    Article  CAS  Google Scholar 

  5. J.C. Zhao, M.R. Jackson, L.A. Peluso, and L.N. Brewer: A diffusion-multiple approach for mapping phase diagrams, hardness, and elastic modulus. JOM 54, 42 (2002).

    Article  CAS  Google Scholar 

  6. L. You and X.P. Song: First principles study of low Young’s modulus Ti–Nb–Zr alloy system. Mater. Lett. 80, 165 (2012).

    Article  CAS  Google Scholar 

  7. R.M. Hu, Z.B. Zhou, X.L. Zhou, J. Yu, and K.H. Zhang: Phase stability, electronic structure, elastic properties and hardness of Ru–Ir alloys: First-principles calculations. Mater. Res. Express 4, 076512 (2017).

    Article  CAS  Google Scholar 

  8. X. Li, X.Q. Tu, B.Q. Liu, J.M. Song, W. Luo, Y. Lei, G.A. Sun, B. Chen, and Q.M. Hu: Composition-dependent elastic properties in Ti–Ni–Nb from first principle calculations. J. Alloys Compd. 706, 260 (2017).

    Article  CAS  Google Scholar 

  9. S.Y. Wen, Y. Tang, J. Zhong, L.J. Zhang, Y. Du, and F. Zheng: High-throughput measurements of interdiffusivity matrices in face centered cubic Ni–Al–Mo alloys at 1273–1473 K. J. Mater. Res. 32, 2188 (2017).

    Article  CAS  Google Scholar 

  10. J. Chen, C. Zhang, J. Wang, W.M. Chen, Y. Tang, L.J. Zhang, and Y. Du: Thermodynamic description, diffusivities and atomic mobilities in binary Ni–Os system. Calphad 50, 118 (2015).

    Article  CAS  Google Scholar 

  11. L. Kaufman and H. Bernstein: Computer Calculation of Phase Diagrams (Academic Press, New York, 1970).

    Google Scholar 

  12. L.J. Zhang, J. Wang, Y. Du, R. Hu, P. Nash, X.G. Lu, and C. Jiang: Thermodynamic properties of the Al–Fe–Ni system acquired via a hybrid approach combining calorimetry, first-principles and CALPHAD. Acta Mater. 57, 5324 (2009).

    Article  CAS  Google Scholar 

  13. L.J. Zhang, Y. Du, I. Steinbach, Q. Chen, and B. Huang: Diffusivities of an Al–Fe–Ni melt and their effects on the microstructure during solidification. Acta Mater. 58, 3664 (2010).

    Article  CAS  Google Scholar 

  14. Available at: https://www.thermocalc.com/products-services/databases (accessed April 10, 2019).

  15. Z.K. Liu, H. Zhang, S. Ganeshan, Y. Wang, and S.N. Mathaudhu: Computational modeling of effects of alloying elements on elastic coefficients. Scr. Mater. 63, 686 (2010).

    Article  CAS  Google Scholar 

  16. X.G. Lu, M. Selleby, and B. Sundman: Assessments of molar volume and thermal expansion for selected bcc, fcc and hcp metallic elements. Calphad 29, 68 (2005).

    Article  CAS  Google Scholar 

  17. A.E. Gheribi and P. Chartrand: Application of the CALPHAD method to predict the thermal conductivity in dielectric and semiconductor crystals. Calphad 39, 70 (2012).

    Article  CAS  Google Scholar 

  18. X.T. Liu and K. Oikawa: Assessment of the temperature and pressure dependence of molar volume and phase diagrams of Cu and Zn. Calphad 47, 114 (2014).

    Article  CAS  Google Scholar 

  19. L.J. Zhang, M. Stratmann, Y. Du, B. Sundman, and I. Steinbach: Incorporating the CALPHAD sublattice approach of ordering into the phase-field model with finite interface dissipation. Acta Mater. 88, 156 (2015).

    Article  CAS  Google Scholar 

  20. J. Chen, L.J. Zhang, and X.G. Lu: Screening of possible Re-substitutional elements in single-crystal Ni-based superalloys: A viewpoint from interdiffusion coefficients in Ni–Al–X ternaries. Metall. Mater. Trans. A 49A, 2999 (2018).

    Article  CAS  Google Scholar 

  21. J. Chen, J.K. Xiao, L. Zhang, and Y. Du: Interdiffusion in fcc Ni–X (X = Rh, Ta, W, Re, and Ir) alloys. J. Alloys Compd. 657, 457 (2016).

    Article  CAS  Google Scholar 

  22. Y. Lin, M. Wei, G.D. Li, and L.J. Zhang: Phase equilibria and microhardness of as-cast and annealed Ni–Al–Os alloys in Ni-rich region. J. Phase Equilib. Diffus. 39, 944 (2018).

    Article  CAS  Google Scholar 

  23. J. Chen, J.R. Zhao, L.J. Zhang, X-G. Lu, and L. Liu: Atomic mobilities in fcc Ni-rich Ni–X (X = Rh, Ta, W, Re, and Ir) systems. Calphad 65, 316 (2019).

    Article  CAS  Google Scholar 

  24. S.H. Zhou, Y. Wang, L.Q. Chen, Z.K. Liu, and R.E. Napolitano: Solution-based thermodynamic modeling of the Ni–Ta and Ni–Mo–Ta systems using first-principle calculations. Calphad 33, 631 (2009).

    Article  CAS  Google Scholar 

  25. P. Gustafson: A thermodynamic evaluation of the Cr–Ni–W system. Calphad 12, 277 (1988).

    Article  CAS  Google Scholar 

  26. K. Yaqoob and J.M. Joubert: Experimental determination and thermodynamic modeling of the Ni–Re binary system. J. Solid State Chem. 196, 320 (2012).

    Article  CAS  Google Scholar 

  27. K. Durst, O. Franke, A. Böhner, and M. Göken: Indentation size effect in Ni–Fe solid solutions. Acta Mater. 55, 6825 (2007).

    Article  CAS  Google Scholar 

  28. R. Schwaiger, B. Moser, M. Dao, N. Chollacoop, and S. Suresh: Some critical experiments on the strain-rate sensitivity of nanocrystalline nickel. Acta Mater. 51, 5159 (2003).

    Article  CAS  Google Scholar 

  29. C.H. Wang, T.H. Fang, P.C. Cheng, C.C. Chiang, and K.C. Chao: Simulation and experimental analysis of nanoindentation and mechanical properties of amorphous NiAl alloys. J. Mol. Model. 21, 1 (2015).

    Article  CAS  Google Scholar 

  30. V. Petley, S. Sathishkumar, K.H.T. Raman, G.M. Rao, and U. Chandrasekhar: Microstructural and mechanical characteristics of Ni–Cr thin films. Mater. Res. Bull. 66, 59 (2015).

    Article  CAS  Google Scholar 

  31. S.H. Kim: Young’s Modulus Measurement of Electroplated Nickel Using AFM (2006 ASME International Mechanical Engineering Congress and Exposition, Chicago, Illinois, 2006).

    Book  Google Scholar 

  32. O. Hubert, X. Milhet, P. Gadaud, M. Tatat, P.O. Renault, and C. Coupeau: Modeling of Young’s modulus variations with temperature of Ni and oxidized Ni using a magneto-mechanical approach. Mater. Sci. Eng., A 633, 76 (2015).

    Article  CAS  Google Scholar 

  33. W. Köster: Temperaturabhangigkeit des Elastizitatsmoduls reiner Metalle. Z. Metallkd. 39, 1 (1948).

    Google Scholar 

  34. K. Honda and T. Terada: On the change of elastic constants of ferromagnetic substances by magnetization. Tokyo Sugaku-Butsurigakukwai Kiji-Gaiyo 2, 381 (1905).

    Google Scholar 

  35. N. Kurnakow and J. Rapke: Harte und Elastizitatsmodul isomorpher Gemische von Kupfer mit Nickel. Z. Anorg. Chem. 87, 269 (1914).

    Article  Google Scholar 

  36. W.A. Mudge and L.W. Luff: Some mechanical properties of nickel, manganese–nickel and copper–nickel alloys. Am. Soc. Test. Mater., Proc. 28, 278 (1928).

    Google Scholar 

  37. A. Jacquerod and H. Mügeli: Etude sur l’élasticité de flexion: Fer–cuivre–or–argent–platine–verre de silice–nickel. Helv. Phys. Acta 4, 3 (1931).

    Google Scholar 

  38. K. Nakamura: Unterschung der Variationen des Elastizitatskoeffizienten der Metallegierung Ni–Fe durch Magnetisierung. Z. Phys. 94, 707 (1935).

    Article  CAS  Google Scholar 

  39. R.M. Davies and I.H. Thomas: A dynamical method for the measurement of Young’s modulus for imperfectly elastic metals, and the application of the method to nickel and some of its alloys. Philos. Mag. 23, 361 (1937).

    Article  CAS  Google Scholar 

  40. O. Engler: Der Elastizitätsmodul ferromagnetischer Stoffe in Abhängigkeit von der Temperatur und vom Magnetfeld. Ann. Phys. 423, 145 (1938).

    Article  Google Scholar 

  41. R.I. Kimura: On the elastic moduli of ferromagnetic materials. Part I. Dynamical measurements of the elastic moduli of iron crystals. Proc. Phys.-Math. Soc. Jpn. 21, 686 (1939).

    Google Scholar 

  42. M. Yamamoto: Young’s modulus of elasticity and its variation with magnetization in ferromagnetic nickel–copper alloys. Nippon Kinzoku Gakkaishi 6, 249 (1942).

    Google Scholar 

  43. M. Yamamoto: On the ΔE-effect of iron, nickel and cobalt. Nippon Kinzoku Gakkaishi 5, 167 (1941).

    CAS  Google Scholar 

  44. H. Masumoto and H. Saito: On elasticity, its temperature coefficient, and heat expansion coefficient of the nickel–copper alloy system. Nippon Kinzoku Gakkaishi 8, 49 (1944).

    Google Scholar 

  45. H. M. Ledbetter and R. P. Reed: Elastic properties of metals and alloys. I. Iron, Nickel, and Iron–Nickel Alloys. J. Phys. Chern. Ref. Data 2, 531 (1973).

    CAS  Google Scholar 

  46. V.A. Pavlov, N.F. Kriutchkov, and I.D. Fedotov: Relationship of temperature to elastic modulus in nickel–copper alloys. Phys. Met. Metallogr. 5, 160 (1957).

    Google Scholar 

  47. W.H. Hill, K.D. Shimmin, and B.A. Wilcox: Elevated temperature dynamic moduli of metallic materials. Am. Soc. Test. Mater., Proc. 61, 890 (1961).

    Google Scholar 

  48. Y. Tino and T. Maeda: On the anomalous thermoelastic variation in the invar-type iron–nickel alloys. J. Phys. Soc. Jpn. 18, 955 (1963).

    Article  Google Scholar 

  49. A.F. Orlov and S.G. Fedotov: Temperature dependence of the Young’s and shear moduli of Ni–Cu alloys. Phys. Met. Metallogr. 22, 146 (1966).

    Google Scholar 

  50. G. Faninger: Die elastischen konstanten von Kupfer·Nickel-Vielkristallen. Z. Metallkd. 60, 601 (1969).

    CAS  Google Scholar 

  51. H. Masumoto, H. Saitô, and S. Sawaya: Thermal expansion and temperature dependence of Young’s modulus of nickel-copper alloys. Trans. Jpn. Inst. Met. 11, 88 (1970).

    Article  Google Scholar 

  52. J. Merker, D. Lupton, M. Töpfer, and H. Knake: High temperature mechanical properties of the platinum group metals. Platinum Met. Rev. 45, 74 (2001).

    CAS  Google Scholar 

  53. S.L. Shang, A. Saengdeejing, Z.G. Mei, D.E. Kim, H. Zhang, S. Ganeshan, Y. Wang, and Z.K. Liu: First-principles calculations of pure elements: Equations of state and elastic stiffness constants. Comput. Mater. Sci. 48, 813 (2010).

    Article  CAS  Google Scholar 

  54. S.L. Shang, D.E. Kim, C.L. Zacherl, Y. Wang, Y. Du, and Z.K. Liu: Effects of alloying elements and temperature on the elastic properties of dilute Ni-base superalloys from first-principles calculations. J. Appl. Phys. 112, 053515 (2012).

    Article  CAS  Google Scholar 

  55. D. Kim, S.L. Shang, and Z.K. Liu: Effects of alloying elements on elastic properties of Ni by first-principles calculations. Comput. Mater. Sci. 47, 254 (2009).

    Article  CAS  Google Scholar 

  56. R. Hill: The elastic behaviour of a crystalline aggregate. Proc. Phys. Soc., London, Sect. A 65, 349 (1952).

    Article  Google Scholar 

  57. W.C. Oliver and G.M. Pharr: An improved technique for determining hardness and elastic modulus using load and displacement sensing indentation experiments. J. Mater. Res. 7, 1564 (1992).

    Article  CAS  Google Scholar 

  58. W.C. Oliver and G.M. Pharr: Measurement of hardness and elastic modulus by instrumented indentation: Advances in understanding and refinements to methodology. J. Mater. Res. 19, 3 (2004).

    Article  CAS  Google Scholar 

Download references

Acknowledgments

The financial support from the Youth Talent Project of Innovation-driven Plan at Central South University (Grant No. 2019CX027), the Hunan Provincial Science and Technology Program of China (Grant No. 2017RS3002)—Huxiang Youth Talent Plan, Project Funded by China Postdoctoral Science Foundation (Grant No. 2018M640526), Jiangsu Planned Projects for Postdoctoral Research Funds (Grant No. 2018K073C) and Lvyangjinfeng Talent Program of Yangzhou are acknowledged. Lijun Zhang acknowledges the project supported by the State Key Laboratory of Powder Metallurgy Foundation, Central South University, Changsha, China.

Author information

Authors and Affiliations

  1. Testing Center, Yangzhou University, Yangzhou, 225009, People’s Republic of China

    Juan Chen

  2. School of Chemistry and Chemical Engineering, Yangzhou University, Yangzhou, 225009, China

    Juan Chen

  3. State Key Laboratory of Powder Metallurgy, Central South University, Changsha, Hunan, 410083, People’s Republic of China

    Lijun Zhang

Authors
  1. Juan Chen
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Lijun Zhang
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding authors

Correspondence to Juan Chen or Lijun Zhang.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Chen, J., Zhang, L. Composition-dependent hardness and Young’s modulus in fcc Ni–X (X = Rh, Ta, W, Re, Os, and Ir) alloys: Experimental measurements and CALPHAD modeling. Journal of Materials Research 34, 3104–3115 (2019). https://doi.org/10.1557/jmr.2019.220

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1557/jmr.2019.220

Search

Navigation