Abstract
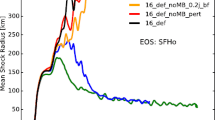
Test particle methods have been applied very successfully to the numerical simulation of heavy ion reactions at intermediate and high beam energies. Here we will show that the same techniques can be used successfully to simulate the dynamics of the collapse of type II supernovae precursors. We will focus special attention on the effects of collective angular momentum on the resulting supernova dynamics.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
C.Y. Wong, Phys. Rev. C 25 (1982) 1460.
J. Cugnon, T. Mizutani and J. Vandermeulen, Nucl. Phys. A352 (1981) 505.
J. Cugnon, D. Kinet, and J. Vandermeulen, Nucl. Phys. A379 (1982) 553.
G.F. Bertsch, H. Kruse and S. Das Gupta, Phys. Rev. C 29 (1984) 673.
W. Bauer, G.F. Bertsch, W. Cassing and U. Mosel, Phys. Rev. C 34 (1986) 2127.
G.F. Bertsch and S. Das Gupta, Phys. Rep. 160 (1988) 189.
P. Danielewicz and G.F. Bertsch, Nucl. Phys. A533 (1991) 712.
H. Kruse et al., Phys. Rev. Lett. 54 (1985) 289.
H. Stöcker and W. Greiner, Phys. Rep. 137 (1986) 277.
J. Cugnon and M.C. Lemaire, Nucl. Phys. A489 (1988) 781.
P. Schuck et al., Prog. Part Nucl. Phys. 22 (1989) 181.
W. Cassing and U. Mosel, Prog. Part. Nucl Phys. 25 (1990) 235.
B.A. Li and W. Bauer, Phys. Lett. B254 (1991) 335.
S.J. Wang et al., Ann. Phys. (N.Y.) 209 (1991) 251.
A. Ono et al., Prog. of Theor. Phys. 87 (1992) 1185.
W. Bauer, C.K. Gelbke and S. Pratt, Ann. Rev. Nucl. Part. Sci. 42 (1992) 77.
W. Bauer, Prog. in Part. and Nucl. Phys. 30 (1993) 45.
W. Bauer, Phys. Rev. Lett. 61 (1988) 2534.
A color version of this figure is on the back cover of the Proc. of the 18th Winter Workshop on Nuclear Dynamics, 2002.
J. Lattimer and F. Swesty, Nucl. Phys., A535 (1991) 331; Equation of state version 2.7 (ls eos v2.7), http://www.ess.sunysb.edu/dswesty/lseos.html.
F. Timmes and F. Swesty, Astrophys. J. Suppl. S 126 (2000) 501; http://flash.uchicago.edu/fxt/eos.shtml.
T. Bollenbach, M.S. Thesis, Michigan State University, 2002.
T. Bollenbach and W. Bauer, in Exotic Clustering, eds. S. Costa, A. Insolia and C. Tùve, American Institute of Physics Conference Proceedings, Vol. 644, Melville, New York, 2002, p. 219.
A. Heger, N. Langer and S. Woosley, Astrophys. J. 528 (2000) 368.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Bauer, W. Dynamical simulations of supernovae collapse and nuclear collisions via the test particle method — Similarities and differences. Acta Phys. Hung. A 21, 371–377 (2004). https://doi.org/10.1556/APH.21.2004.2-4.41
Received:
Issue Date:
DOI: https://doi.org/10.1556/APH.21.2004.2-4.41
Keywords
- heavy ion collision
- test particle simulation
- particle spectra
- flow
- interferometry
- supernova
- type II
- precursor
- phase space dynamics
- angular momentum