Abstract
Novel mathematical models were developed to predict inter-tablet coating uniformity in terms of coefficients of variation (CV) and acceptance values (AV) for cylindrical tablet pan coaters, operating in batch and continuous modes. The models, based on binomial coating spray and tablet movement distribution functions and on bed geometry, yielded equations and results that are in good agreement with previously reported experimental data, most empirical expressions, and more computationally intensive models. The new model equations are readily useable for process analysis, optimization, scale-up, and manufacturing design and control.

















Similar content being viewed by others
Abbreviations
- A bed :
-
cross-sectional bed area
- AV :
-
acceptance value (USP/EP/JP)
- \(\overline{c}\) :
-
average coating on tablets after the coating process
- c i :
-
coating received per pass through the spray zone
- \(\overline{c_i}\) :
-
average coating on tablets per cycle
- \(\overline{c_1}\) :
-
average coating from one continuous bed
- CV :
-
coefficient of variation
- CV B :
-
coefficient of variation for batch coating
- CV C :
-
coefficient of variation for continuous coating
- d p :
-
tablet diameter, Sauter or surface-volume diameter used
- D :
-
pan diameter
- f c :
-
Choi-Porter formulation factor
- f f :
-
pan loading = bed volume/pan volume
- f r :
-
ratio of bed cycle time to pan cycle time
- F B :
-
coating distribution for the batch process
- F C :
-
coating distribution for the continuous process
- F m :
-
coating distribution for the continuous process with m beds in series
- g :
-
gravitational acceleration
- h :
-
bed height
- i :
-
denotes the position in the number of cycles or the number of passes through the spray zone
- k, k”, k AV , k C , k e , k n , k P , k KW , k’ KW , k s1 , k s2 , k Δ :
-
equation coefficients
- L :
-
length of the coating pan
- m :
-
number of beds in series
- m C, bed :
-
steady-state bed weight in the continuous coating process
- \(\dot{m}\) :
-
bed circulation rate
- \(\dot{m_p}\) :
-
production rate
- \(\dot{m_{sp}}\) :
-
spray rate
- M :
-
reference value
- n :
-
number of cycles
- n c :
-
number of passes through the spray zone
- n s :
-
number of samples
- N :
-
number of tablets in the bed
- p at :
-
atomizing air pressure
- P i :
-
probability of i
- s :
-
sample standard deviation
- T :
-
drug dosage label claim in the coating
- x c :
-
probability of tablet going through the spray zone
- x e :
-
exit probability of tablets in continuous processes
- x m :
-
moisture content of API
- x p :
-
purity of API
- \(\overline{X}\) :
-
mean of individual content
- v w :
-
tablet velocity
- V bed :
-
bed volume
- V a :
-
volume of upflow
- V b :
-
volume of downflow
- V c :
-
volume of spray zone
- V d :
-
volume of bypass zone
- V T :
-
volume of the pan
- w Tab :
-
tablet weight
- w B :
-
batch weight
- δ slip :
-
slip between pan wall and tablet
- Δ:
-
depth of the spray zone
- \(\dot{\varphi}\) :
-
pan rotational speed
- η :
-
coating efficiency
- σ :
-
overall standard deviation
- σ i :
-
cycle standard deviation
- σ 1 :
-
standard deviation for one continuous bed
- ρ bed :
-
bed density
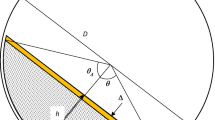
- θ :
-
angle shown in Fig. 3a
- θ Δ :
-
angle accounting for the difference in the spray zone depth in Fig. 3b and Eq. 25
- τ :
-
total coating time
References
United States Pharmacopeial Convention: General Chapters: <905> Uniformity of Dosage Units, Pharmacopeial Forum. 2020;35(3):6673,7079,7183.
Porter SC. Troubleshooting appearance-related problems of film coated tablets. Tablets and Capsules. 2008;6(3):16–22.
Porter SC. The role of high-solids coating systems in reducing process costs. Tablets & Capsules. 2010;8(3):10–15.
Rege BD, Gawel J, Kou JH. Identification of critical process variables for coating actives onto tablets via statistically designed experiments. Int J Pharm. 2002;237:87–94.
Choi M. Applications of process thermodynamics in pharmaceutical coating. Tablets & Capsules. 2007;5(3):12–24.
Suzzi D, Radl S, Khinast JG. Local analysis of the tablet coating process: impact of operation conditions on film quality. Chem Eng Sci. 2010;69:107–21.
Niblett D, Porter S, Reynolds G, Morgan T, Greenamoyer J, Hach R, et al. Development and evaluation of a dimensionless mechanistic pan coating model for the prediction of coated tablet appearance. Int J Pharm. 2017;528(1–2):180–201.
Freireich B, Wassgren C. Intra-particle coating variability: analysis and Monte-Carlo simulations. Chem Eng Sci. 2010;65:1117–24.
Brock D, Zeitler JA, Funke A, Knop K, Kleinebudde P. Evaluation of critical process parameters for intra-tablet coating uniformity using terahertz pulsed imaging. Eur J Pharm Biopharm. 2013;85(3):1122–9.
Porter SC. Scale-up of film coating. In: Levin M, editor. Pharmaceutical process scale-up. New York: Taylor & Francis; 2006. p. 435–85.
Mann U. Analysis of spouted-bed coating and granulation. 1. Batch operation. Ind Eng Chem Proc Des Dev. 1983;22(2):288–93.
Freireich B, Li J. A renewal approach to understanding interparticle coating variability. Powder Technol. 2013;249:330–8.
Pandey P, Katakdaunde M, Turton R. Modeling weight variability in a pan coating process using Monte Carlo simulations. AAPS PharmSciTech. 2006;7(4):E2–E11.
Kalbag A, Wassgren C. Inter-tablet coating variability: tablet residence time variability. Chem Eng Sci. 2009;64(11):2705–17.
Kureck H, Govender N, Siegmann E, Boehling P, Radeke C, Khinast J. Industrial scale simulations of tablet coating using GPU based DEM: a validation study. Chem Eng Sci. 2019;202:462–80.
Choi M, Meisen A. Sulfur coating of urea in shallow spouted beds. Chem Eng Sci. 1997;52(7):1073–86.
Kumar R, Freireich B, Wassgren C. DEM-compartment-population balance model for particle coating in a horizontal rotating drum. Chem Eng Sci. 2015;125:144–57.
Boehling P, Toschkoff G, Knop K, Kleinebudde P, Just S, Funke A, et al. Analysis of large-scale tablet coating: modeling, simulation and experiments. Eur J Pharm Sci. 2016;90:14–24.
Boehling P, Khinast JG, Jajcevic D, Davis C, Carmody A, Doshi P, et al. Computational fluid dynamics-discrete element method modeling of an industrial-scale Wurster coater. J Pharm Sci. 2019;108:538–50.
FDA. Guidance for Industry: PAT—A Framework for Innovative Pharmaceutical Development, Manufacturing, and Quality Assurance. Pharmaceutical CGMPs, 2004.
Porter SC. Continuous film coating processes: a review. Tablets & Capsules. 2007;5(3):26–39.
Thakral NK, Thakral S. Continuous tablet coaters: developments, advantages and limitations. Innovations Pharm Technol. 2009:70–3.
Barimani S, Sinbanc R, Kleinebudde P. Optimization of semi-batch tablet coating process for a continuous manufacturing line by design of experiments. Int J Pharm. 2018;539:95–103.
Suzzi D, Toschkoff G, Radl S, Machold D, Fraser SD, Glasser BJ, et al. DEM simulation of continuous tablet coating: effects of tablet shape and fill level on inter-tablet coating variability. Chem Eng Sci. 2012;69(1):107–21.
Choi M. Sulfur Coating of Urea in Shallow Spouted Beds. Ph.D. dissertation. The University of British Columbia. 1993. p. 56–73.
Choi M. Pan coating simulation for determining tablet coating uniformity. US Patent No. 7,634,390 B2, Washington, DC: U.S. Patent and Trademark Office. 2009.
Perry RH, Green DW, Maloney JO. Perry’s chemical engineering handbook. 6th ed. New York: McGraw-Hill; 1984. p. 2–38.
Chen W, Chang S-Y, Kiang S, Marchut A, Lyngberg O, Wang J, et al. Modeling of pan coating processes: prediction of tablet content uniformity and determination of critical process parameters. J Pharm Sci. 2010;9(7):3213–25.
Toschkoff G, Just S, Funke A, Djuric D, Knop K, Kleinebudde P, et al. Spray models for discrete element simulations of particle coating processes. Chem Eng Sci. 2013;101:603–14.
Hill CG Jr. An Introduction to Chemical Engineering Kinetics & Reactor Design. New York: Wiley & Sons; 1977.
Choi M. Determining the manufacturability of drug-layered tablets. Pharm Manuf. 2007;6(4):34–42.
Alexander A, Shinbrot T, Muzzio F. Scaling surface velocities in rotating cylinders as a function of vessel radius, rotation rate, and particle size. Powder Technol. 2002;126:174–90.
Chang R-K, Leonzio M. The effect of run time on the inter-unit uniformity of aqueous film coating applied to glass beads in a hi-coater. Drug Dev Ind Pharm. 1995;21(16):1895–9.
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher’s Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Appendices
Appendix 1
Derivation of Eq. 8 Using the Central Limit Theorem
The central limit theorem states that individual variances (\({\sigma}_i^2\)) from each cycle can be added to determine the overall variance (σ2). Since bed shape and movement are assumed to be the same throughout the coating process, the variance for each cycle also remains the same. Therefore,
For a binomial system, the variance per cycle consists of the variances for tablets entering the spray zone (\({\sigma}_c^2\)) and bypassing the spray zone (\({\sigma}_b^2\)) based on their probabilities:
Applying the definition of population standard deviation, this equation becomes
Substituting the expression for \({\sigma}_i^2\) in Eq. 50 into A1 results in
Substituting σ2 in Eq. 51 into Eq. 3 gives the following expression for CVB:
Appendix 2
Bridging Actual Spray Distribution to x c in Binomial Distribution
Despite the seemingly crude assumption that all tablets receive the same amount of coating, this allowed the use of binomial distribution to model the coating uniformity. Since batch coating processes are long processes with large number of cycles (n), the central limit theorem can be applied—i.e., all distributions approach normal distribution (see Fig. 5 for the binomial distribution for n = 100). It is then a matter of bridging the standard deviation between distributions to arrive at the matching xc. Table III shows this bridging approach for linear and parabolic distribution to the binomial distributions, which corresponded to xc values of 77% and 56%, respectively. This illustration used only 10 zeros and ones.
Alternatively, if coating per pass distribution information is not available, xc can be estimated using Eq. 24.
Coating Distribution on the Surface of the Bed
Coating per cycle distribution for the tablets traveling on the surface of the bed can be obtained by integrating through all possible tablet paths. If the tablet path is straight vertical from top to bottom as shown by the arrow in Fig. 18, the amount of coating received can be calculated by integrating along this path. In an example case where the spray pattern is circular, tablet velocity is constant, and there is no concentration gradient (i.e., evenly distributed spray within the pattern), integration of all possible paths shown in Fig. 18 results in the coating per pass distribution shown in Fig. 19. xc can be calculated by bridging the standard deviation of this coating per pass distribution as illustrated above.
Appendix 3
Cost Optimization Calculation
The data used in Fig. 17 assumes the base case coating process takes 2 h to set up and 2 h to coat to achieve a 10% average weight gain with CV = 15%. Five percent of the tablets are undercoated or defective, which corresponds to a weight gain of 7.5% (based on the mean of 10% and CV of 15%). To determine the quantities of coating material needed achieve CV values ranging from 4 to 30% at the same defect rate (i.e., 5% of tablets with less than 7.5% weight gain), the ratio of the coating weight gain that corresponds to the 5% of the population at each CV and that of the base case is used. For example, at CV = 4%, 5% of tablets have less than 9.3% weight gain when the same coating amount is applied as in the base case. This represents a weight gain that is 20% higher than the base case (i.e., 7.5% vs 9.3%); therefore, a process that yields CV of 4% can use 20% less coating amount relative to the base case with the same defect rate.
To calculate the operating and total costs, the coating times corresponding to these CV values (as determined by Eq. 24) are used. Table IV gives the calculated results for one of the scenarios shown in Fig. 17. The ratio of material cost to operating cost was 10, a ratio that approximates the current cost of controlled-release coating material (~ $1000/batch for a typical commercial batch size of ~ 400 kg) and total operating cost (~ $100/h).
Rights and permissions
About this article
Cite this article
Choi, M., Porter, S.C., Macht, B. et al. Novel Coating Uniformity Models for Tablet Pan Coaters. AAPS PharmSciTech 22, 7 (2021). https://doi.org/10.1208/s12249-020-01857-z
Received:
Accepted:
Published:
DOI: https://doi.org/10.1208/s12249-020-01857-z