Abstract
In the paper, we offer a set of inequalities involving delayed argument and offer their application for higher-order differential equations of the form
to be oscillatory. The conditions obtained essentially improve many other known results.
MSC:34K11, 34C10.
Similar content being viewed by others
1 Introduction
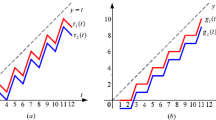
The paper is organized as follows. In the first part we consider only properties of functions and their derivatives, and later we connect the estimate obtained with properties of solutions of differential equations. We shall investigate the properties of a couple of functions and , .
Lemma 1 Assume that ℓ is a positive integer such that

eventually. Then for any constant and for every ,
eventually.
Proof Assume that holds for . Using the monotonicity of , it is easy to see that for any ,
eventually, let us say, for . We define a sequence of functions as follows:
It follows from (1.2) that . An integration of this from to t yields
On the other hand, since as , we see that
Combining the last two inequalities, we conclude that
Proceeding as above, we verify that , eventually, for all . Therefore,
or in other words
Setting , the last inequalities imply (1.1) and the proof is complete. □
Lemma 2 Assume that and that ℓ is a positive integer such that holds. Then, for any constant ,
eventually.
Proof Taylor’s theorem implies that
Employing (1.1), we have
The proof is complete. □
The obtained estimates can be used, e.g., in the theory of functional equations. In the paper, we present their application in discussing oscillatory and asymptotic properties of higher-order delay differential equations.
2 Main results
We consider higher-order delay differential equation

where
(H1) , .
Denote by the set of all nonoscillatory solutions of (E). It follows from the classical lemma of Kiguradze [1] that the set has the following decomposition:
where the nonoscillatory solution , let us say positive, satisfies
A nonoscillatory solution of (E) is said to be of degree ℓ if . Following Kondratiev and Kiguradze, we say that (E) has property (A) provided that
The investigation of oscillatory properties of the second- and higher-order linear differential equations started with the Sturm comparison theorem [2]. Later Mahfoud [3] essentially contributed to the subject and presented a very useful comparison technique for studying the properties of a delay differential equation from those of a differential equation without delay. A new impetus to investigation in this direction was given by papers of Chanturia and Kiguradze [4], Kusano and Naito [5] and Koplatadze et al. [6, 7]. See also [1–20]. In the paper, we employ Lemma 2 to establish new criteria for oscillation of (E).
It is interesting to note that the condition
is necessary for property (A) of (E). This fact has been observed in [6] and [7].
Theorem 1 Assume that (E) has a solution of degree , then for any so does the ordinary equation

Proof Assume that (E) possesses a nonoscillatory solution . We may assume that is positive. Then condition (1.3) of Lemma 1 implies that is a positive solution of the differential inequality
On the other hand, it follows from Theorem 2 of [5] that the corresponding equation () has also a solution of degree ℓ. The proof is complete. □
So, if we eliminate solutions of degree ℓ of equations (), we get property (A) of studied equation (E). To do it, we recall the following comparison result which is due to Chanturia [4].
Theorem 2 Assume that
If the differential equation
has no solution of degree ℓ, neither does the equation
In view of Theorem 1, we apply this comparison theorem to equations () and the Euler equation
to obtain new criteria for property (A) of (E). Properties of (2.3) are connected with properties of the polynomial . Let us denote
where for n odd, while for n even. In other words, represents all local maxima of the polynomial (see Figure 1). Then it is easy to verify (see also [15]) that the following criterion for the to be empty holds true.
Lemma 3 Let . If
where for n odd and for n even, then (2.3) has no solution of degree ℓ.
Employing Theorem 2 to (2.3) and (), in view of Theorem 1, one gets the following theorem.
Theorem 3 Assume that

Then (E) has property (A).
Proof Assume that n is odd. Observing that and for every , it follows from (P) that for every ,

On the other hand, () implies that there exists a couple of constants and such that
Since , Euler equation (2.3) has no solution of degree ℓ. On the other hand, taking (2.5) into account, Theorem 2 ensures that () has no solution of degree ℓ. Finally, Theorem 1 guarantees that (E) has property (A). The proof is complete. □
For , , the previous result simplifies to the following.
Corollary 1 Assume that

Then the delay differential equation

has property (A).
Theorem 4 Let n be odd. Assume that (E) has property (A). Then every nonoscillatory solution of (E) satisfies
Proof First note that property (A) of (E) implies (2.1). Moreover, it follows from the definition of property (A) that every nonoscillatory solution , which implies that there exists . We claim that . If not, then . An integration of (E) from t to ∞ yields
Having repeated this procedure, we are led to
which contradicts (2.1) and we conclude that . □
We support our results with the following illustrative example.
Example 1 Consider the fifth-order delay differential equation

The graph of the polynomial that corresponds to the fifth-order equation is presented in Figure 1. Employing Matlab, we easily evaluate that
Consequently, criterion () for property (A) of (E) reduces for () to
3 Comparison
Theorem 2 essentially improves Chanturia’s test [4] that guarantees property (A) of

provided that
Kiguradze’s test [1] that for property (A) of (E) requires
and Koplatadze’s test [7] for property (A) of (E) that claims the condition
where is nondecreasing.
We provide details while comparing those criteria with our one.
Example 2 Consider once more the fifth-order delay differential equation (). It is easy to see that Chanturia’s test can be applied only when and requires
for property (A) of (). Kiguradze’s test fails. On the other hand, Koplatadze’s test simplifies for and to
respectively, while our criterion needs only
respectively.
References
Kiguradze IT:On the oscillation of solutions of the equation . Mat. Sb. 1964, 65: 172-187. (Russian)
Sturm JCF: Mémoire sur les équations différentielles linéaires du second ordre. J. Math. Pures Appl. 1836, 1: 106-186.
Mahfoud WE: Oscillation and asymptotic behavior of solutions of n th order nonlinear delay differential equations. J. Differ. Equ. 1977, 24: 75-98. 10.1016/0022-0396(77)90171-1
Kiguradze IT, Chaturia TA: Asymptotic Properties of Solutions of Nonautonomous Ordinary Differential Equations. Kluwer Academic, Dordrecht; 1993.
Kusano T, Naito M: Comparison theorems for functional differential equations with deviating arguments. J. Math. Soc. Jpn. 1981, 3: 509-533. Zbl 0494.34049
Koplatadze RG: On differential equations with a delayed argument having properties A and B. Differ. Uravn. (Minsk) 1989, 25: 1897-1909.
Koplatadze RG, Kvinkadze G, Stavroulakis I: Properties A and B of n th order linear differential equations with deviating argument. Georgian Math. J. 1999, 6: 553-566. 10.1023/A:1022962129926
Agarwal RP, Grace SR, O’Regan D: Oscillation Theory for Difference and Functional Differential Equations. Kluwer Academic, Dordrecht; 2000.
Baculíková B, Džurina J: Oscillation of third-order neutral differential equations. Math. Comput. Model. 2010, 52: 215-226. 10.1016/j.mcm.2010.02.011
Baculíková B, Graef J, Džurina J: On the oscillation of higher order delay differential equations. Nonlinear Oscil. 2012, 15: 13-24.
Baculíková B, Džurina J: Oscillation of third-order nonlinear differential equations. Appl. Math. Lett. 2011, 24: 466-470. 10.1016/j.aml.2010.10.043
Baculíková B: Properties of third order nonlinear functional differential equations with mixed arguments. Abstr. Appl. Anal. 2011., 2011: Article ID 857860
Baculíková B, Džurina J, Rogovchenko Y: Oscillation of third order trinomial differential equations. Appl. Math. Comput. 2012, 218: 7023-7033. 10.1016/j.amc.2011.12.049
Baculíková B, Džurina J: Property (A) and oscillation of third order differential equations with mixed arguments. Funkc. Ekvacioj 2012, 55: 239-253.
Džurina J: Comparison theorems for nonlinear ODE’s. Math. Slovaca 1992, 42: 299-315.
Erbe L, Kong Q, Zhang BG: Oscillation Theory for Functional Differential Equations. Dekker, New York; 1995.
Ladde GS, Lakshmikantham V, Zhang BG: Oscillation Theory of Differential Equations with Deviating Arguments. Dekker, New York; 1987.
Zafer A: Oscillation criteria for even order neutral differential equations. Appl. Math. Lett. 1998, 11: 21-25.
Meng FW, Xu R: Oscillation criteria for certain even order quasi-linear neutral differential equations with deviating arguments. Appl. Math. Comput. 2007, 190: 458-464. 10.1016/j.amc.2007.01.040
Li T, Han Z, Zhao P, Sun S: Oscillation of even-order neutral delay differential equations. Adv. Differ. Equ. 2010., 2010: Article ID 184180
Acknowledgements
This work was supported by the Slovak Research and Development Agency under the contract No. APVV-0008-10.
Author information
Authors and Affiliations
Corresponding author
Additional information
Competing interests
The authors declare that they have no competing interests.
Authors’ contributions
The authors have made the same contribution. All authors read and approved the final manuscript.
Authors’ original submitted files for images
Below are the links to the authors’ original submitted files for images.
Rights and permissions
Open Access This article is distributed under the terms of the Creative Commons Attribution 2.0 International License (https://creativecommons.org/licenses/by/2.0), which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.
About this article
Cite this article
Baculíková, B., Džurina, J. On certain inequalities and their applications in the oscillation theory. Adv Differ Equ 2013, 165 (2013). https://doi.org/10.1186/1687-1847-2013-165
Received:
Accepted:
Published:
DOI: https://doi.org/10.1186/1687-1847-2013-165