Abstract
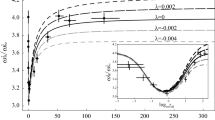
We study an interacting Bose gas at T = 0 under isotropic harmonic confinement within Density Functional Theory in the Local Density approximation. The energy density functional, which spans the whole range of positive scattering lengths up to the unitary regime (infinite scattering length), is obtained by fitting the recently calculated Monte Carlo bulk equation of state [Phys. Rev. A 89, 041602(R) (2014)]. We compare the density profiles of the trapped gas with those obtained by MC calculations. We solve the time-dependent Density Functional equation to study the effect of increasing values of the interaction strength on the frequencies of monopole and quadrupole oscillations of the trapped gas. We find that the monopole breathing mode shows a non-monotonous behavior as a function of the scattering length. We also consider the damping effect of three-body losses on such modes.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
W. Li, T.-L. Ho, Phys. Rev. Lett. 108, 195301 (2012)
B.S. Rem, A.T. Grier, I. Ferrier-Barbut, U. Eismann, T. Langen, N. Navon, L. Khaykovich, F. Werner, D.S. Petrov, F. Chevy, C. Salomon, Phys. Rev. Lett. 110, 163202 (2013)
P. Makotyn, C.E. Klauss, D.L. Goldberger, E.A. Cornell, D.S. Jin, Nat. Phys. 10, 116 (2014)
R.J. Fletcher, A.L. Gaunt, N. Navon, R.P. Smith, Z. Hadzibabic, Phys. Rev. Lett. 111, 125302 (2013)
M. Rossi, L. Salasnich, F. Ancilotto, F. Toigo, Phys. Rev. A 89, 041602(R) (2014)
W. Kohn, L.J. Sham, Phys. Rev. 140, A 1133 (1965)
E. Runge, E.K.U. Gross, Phys. Rev. Lett. 52, 997 (1984)
E. Lipparini, Modern Many-Particle Physics: Atomic Gases, Nanoparticles and Quantum Liquids, 2nd Ed. (World Scientific, Singapore, 2008)
M.L. Chiofalo, M.P. Tosi, Europh. Lett. 56, 326 (2001)
N.N. Bogoliubov, J. Phys. (USSR) 11, 23 (1947)
T.D. Lee, K. Huang, C.N. Yang, Phys. Rev. 106, 1135 (1957)
The coefficients c’s have been determined by ensuring continuity of the interpolating function and of its first three derivatives at x = 0.3 and x = 0.5 once a3 and the coefficients b’s have been fitted to the calculated values. This procedures automatically reproduces the MC values in the interval 0.3 < x < 0.5
E.P. Gross, Nuovo Cimento 20, 454 (1961)
L.P. Pitaevskii, Sov. Phys. JETP 13, 451 (1961)
J.L. DuBois, H.R. Glyde, Phys. Rev. A 63, 023602 (2001)
L.P. Pitaevskii, S. Stringari, Bose-Einstein Condensation (Oxford Univ. Press, Oxford, 2003)
Y. Castin, Comptes Rendus Phys. 5, 407 (2004)
T. Lahaye, J. Metz, B. Frolich, T. Koch, M. Meister, A. Griesmaier, T. Pfau, H. Saito, Y. Kawaguchi, M. Ueda, Phys. Rev. Lett. 101, 080401 (2008)
Author information
Authors and Affiliations
Rights and permissions
About this article
Cite this article
Rossi, M., Ancilotto, F., Salasnich, L. et al. Density functional theory of a trapped Bose gas with tunable scattering length: From weak-coupling to unitarity. Eur. Phys. J. Spec. Top. 224, 565–569 (2015). https://doi.org/10.1140/epjst/e2015-02387-9
Received:
Revised:
Published:
Issue Date:
DOI: https://doi.org/10.1140/epjst/e2015-02387-9