Induced Brownian motion by the Friedmann–Robertson–Walker spacetime in the presence of a cosmic string

Abstract

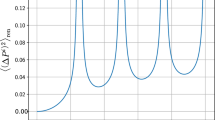
In this paper, we investigate the quantum Brownian motion of a massive scalar point particle induced by the FRW spacetime in the presence of a linear topological defect named cosmic string. In addition, we also consider a flat boundary orthogonal to the defect to analyze its effect on the particle’s motion. For both cases, we found exact expressions for the renormalized mean square deviation of the particle velocity, the quantity that measures the induced Brownian motion, and obtain asymptotic expressions when the point particle is near and far away from the cosmic string and the boundary. Furthermore, in both cases, there appear divergencies in the mean square deviation of the particle velocity coming from a time interval that correspond to a round trip of the massive point particle between its position and the cosmic string/flat boundary. The number of divergencies depends upon the radial position of the particle and the parameter associated with the cosmic string in the case without boundary, and upon this parameter and the radial and z positions of the particle in the case with boundary.

Appendix A: Wightman function

Let us here derive some important expressions which are necessary for the results obtained in the body of the text. The positive-energy Wightman function resulting from the solution (6), obeying Dirichlet boundary condition, can be written in the form [5, 35]:

$$\begin{aligned} W^{(\mathrm \pm )}(x,x') = \pm \frac{p}{8\pi ^2\rho \rho '}\int _{0}^{\infty }\mathrm{d}we^{-\frac{\zeta _{({\mp })}}{2\rho \rho '}w}\sum _{n=-\infty }^{\infty }e^{inp\Delta \phi }I_{p|n|}(w), \end{aligned}$$

(A1)

where the ‘plus’ sign in $W^{(\mathrm \pm )}(x,x')$ indicates the Wightman function due to only the cosmic string spacetime and the ‘minus’ sign indicates the Wightman function due to only a flat boundary [35] and:

$$\begin{aligned} \zeta _{({\mp })} = - \Delta \eta ^2 + (z{\mp } z')^2 + \rho ^2 + \rho '^2 . \end{aligned}$$

(A2)

Therefore, the total renormalized Wightman function associated with a massless scalar field in the cosmic string spacetime obeying the Dirichlet boundary condition on the flat boundary is given by:

$$\begin{aligned} W_{\text {r}}(x,x') = W_{\text {r}}^{(+)}(x,x') + W^{(-)}(x,x'), \end{aligned}$$

(A3)

where $W_{\text {r}}^{(+)}(x,x')$ is the renormalized Wightman function purely due to the cosmic string topology, i.e., the Wightman function with the Minkowski spacetime divergent contribution already subtracted. Also, $W^{(-)}(x,x')$ is the Wightman function induced by the plain boundary. Note that the Neumann boundary condition would only change the plus sign in Eq. (A3) by the minus sign.

Moreover, in Refs. [5, 36], a summation formula to perform the sum in n present in Eq. (A1) was derived and is given by:

$$\begin{aligned} \sum _{n=-\infty }^{\infty }e^{ipn\Delta \phi }I_{p|n|}(w)= & {} \frac{e^w}{p} + \frac{2}{p}\mathop {{\sum }'}\limits _{n=1}^{[q/2]}e^{w\cos \left( \frac{2\pi n}{p}-\Delta \phi \right) }\nonumber \\&- \frac{1}{2\pi }\sum _{j=+,-}\int _{0}^{\infty }d\xi \frac{\sin \left[ p\left( j\Delta \phi + \pi \right) \right] e^{-w\cosh (\xi )}}{[\cosh (p\xi ) - \cos (jp\Delta \phi + p\pi )]}, \end{aligned}$$

(A4)

where [p / 2] represents the integer part of p / 2, and the prime on the sign of summation means that in the case p is an integer number, the term $n=p/2$ should be taken with the coefficient 1 / 2. Note that, if $p<2$ the summation contribution should be omitted. Thereby, using (A4), the Wightman function is obtained as [5, 35]:

$$\begin{aligned} W^{(\mathrm \pm )}(x,x')= & {} \pm \frac{1}{4\pi ^2}\frac{1}{\sigma _0^{({\mp })}} \pm \frac{1}{2\pi ^2}\mathop {{\sum }'}\limits _{n=1}^{[p/2]}\frac{1}{\sigma _n^{({\mp })}}\nonumber \\&{\mp }\frac{q}{8\pi ^3}\sum _{j=+,-}\int _{0}^{\infty }d\xi \frac{\sin \left[ p\left( j\Delta \phi + \pi \right) \right] }{[\cosh (p\xi ) - \cos (jp\Delta \phi + p\pi )]}\frac{1}{\sigma _\xi ^{({\mp })}}, \end{aligned}$$

(A5)

where

$$\begin{aligned} \begin{aligned} \sigma _0^{({\mp })}&= \zeta _{({\mp })} - 2\rho \rho '\cos (\Delta \phi ),\\ \sigma _n^{({\mp })}&= \zeta _{({\mp })} - 2\rho \rho '\cos \left( \frac{2\pi n}{p}-\Delta \phi \right) ,\\ \sigma _{\xi }^{({\mp })}&= \zeta _{({\mp })} + 2\rho \rho '\cosh (\xi ). \end{aligned} \end{aligned}$$

(A6)

Another important relation to calculate the azimuthal component of the dispersion in the particle velocity is obtained by taking the derivatives in $\phi$ and $\phi '$ of Eq. (A1); that is:

$$\begin{aligned} \partial _{\phi }\partial _{\phi '}W^{(\mathrm \pm )}(x,x') = \pm \frac{p^3}{8\pi ^2\rho \rho '}\int _{0}^{\infty }dwe^{-\frac{\zeta _{({\mp })}}{2\rho \rho '}w}\sum _{n=-\infty }^{\infty }n^2I_{p|n|}(w). \end{aligned}$$

(A7)

Note that, after taking the derivatives in the azimuthal coordinates, we have taken the limit $\phi '\rightarrow \phi$. Next, we can make use of the modified Bessel differential equation:

$$\begin{aligned} \sum _{n=-\infty }^{\infty }n^2I_{p|n|}(w) = \frac{1}{p^2}\left( w^2\partial _w^2 + w\partial _w - w^2\right) \sum _{n=-\infty }^{\infty }I_{p|n|}(w), \end{aligned}$$

(A8)

to further work out the expression (A7). Thus:

$$\begin{aligned} \partial _{\phi }\partial _{\phi '}W^{(\mathrm \pm )}(x,x') = \pm \frac{p}{8\pi ^2\rho \rho '}\int _{0}^{\infty }dwe^{-\frac{\zeta _{({\mp })}}{2\rho \rho '}w}\left( w^2\partial _w^2 + w\partial _w - w^2\right) \sum _{n=-\infty }^{\infty }I_{p|n|}(w).\nonumber \\ \end{aligned}$$

(A9)

We can again use the summation formula (A4) for $\Delta \phi =0$ to get:

$$\begin{aligned}&\partial _{\phi }\partial _{\phi '}W^{(\mathrm \pm )}(x,x') \nonumber \\&\quad = \pm \frac{\rho \rho '}{2\pi ^2}\left[ \mathop {{\sum }'}\limits _{n=-[q/2]}^{[p/2]}M_n^{({\mp })}(x,x') - \frac{p}{\pi }\int _{0}^{\infty }d\xi \frac{\sin (p\pi )M_{\xi }^{({\mp })}(x,x')}{[\cosh (p\xi ) - \cos (p\pi )]}\right] , \end{aligned}$$

(A10)

where

$$\begin{aligned} M_{\gamma }^{({\mp })}(x,x') = 16s_{\gamma }^2(s_{\gamma }^2 - 1)\frac{\rho \rho '}{[\sigma _{\gamma }^{({\mp })}]^3} + (1 - 2s_{\gamma }^2)\frac{1}{[\sigma _{\gamma }^{({\mp })}]^2}. \end{aligned}$$

(A11)

Note that$\gamma$ stands for n in the first term and $\xi$ in the second term of Eq. (A10) and $s_n=\sin (n\pi /p)$ and $s_{\xi }=\cosh (\xi /2)$.

We want now to consider the integral in $\eta$ of the functions $M_{\gamma }^{({\mp })}(x,x')$ necessary for the calculation of the $\phi$ component of the velocity dispersion; that is:

$$\begin{aligned} \int _{0}^{\eta }\mathrm{d}\eta _1\int _{0}^{\eta }\mathrm{d}\eta _2M_{\gamma }^{(+)}(x,x) = \frac{1}{\eta ^2c^4}\left[ -\frac{(s_{\gamma }^2 - 1)}{R^2s_{\gamma }^2(1-R^2s_{\gamma }^2)} + \frac{(s_{\gamma }^2 - 2)}{4R^3s_{\gamma }^3}\ln \left( \frac{Rs_{\gamma } + 1}{Rs_{\gamma } - 1}\right) ^2\right] \nonumber \\ \end{aligned}$$

(A12)

and

$$\begin{aligned}&\int _{0}^{\eta }\mathrm{d}\eta _1\int _{0}^{\eta }\mathrm{d}\eta _2M_{\gamma }^{(-)}(x,x)\nonumber \\&\quad = \frac{1}{4\eta ^2c^4(R^2s_{\gamma }^2+Z^2)^{\frac{5}{2}}}\left[ \frac{4R^2s_{\gamma }^2(s_{\gamma }^2-1)\sqrt{R^2s_{\gamma }^2 +Z^2}}{(R^2s_{\gamma }^2 + Z^2 -1)}\right. \nonumber \\&\qquad \left. + [(1-2s_{\gamma }^2)Z^2 + (s_{\gamma }^2-2)R^2s_{\gamma }^2]\ln \left( \frac{\sqrt{R^2s_{\gamma }^2 +Z^2} + 1}{\sqrt{R^2s_{\gamma }^2 +Z^2} - 1}\right) ^2\right] . \end{aligned}$$

(A13)

Therefore, the expressions (A10)–(A13) are all necessary to calculate Eqs. (26) and (43).