Calculation of the persistence length of a flexible polymer chain with short-range self-repulsion

Abstract.

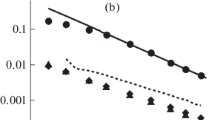
For a self-repelling polymer chain consisting of n segments we calculate the persistence length $L\left(j,n\right)$, defined as the projection of the end-to-end vector on the direction of the j-th segment. This quantity shows some pronounced variation along the chain. Using the renormalization group and $\epsilon$-expansion we establish the scaling form and calculate the scaling function to order $\epsilon^2$. Asymptotically, the simple result $L\left(j,n\right) \approx \mbox{const} \left(j\left(n-j\right)/n\right)^{2\nu-1}$ emerges for dimension d = 3. Also away from the excluded-volume limit $L\left(j,n\right)$ is found to behave very similar to the swelling factor of a chain of length $j \left(n-j\right)/n$. We carry through simulations which are found to be in good accord with our analytical results. For d = 2 both our and previous simulations as well as theoretical arguments suggest the existence of logarithmic anomalies.