Abstract.
We investigate theoretically the formation of a vortex lattice in a superfluid two-spin component Fermi gas in a rotating harmonic trap, in a BCS-type regime of condensed non-bosonic pairs. Our analytical solution of the superfluid hydrodynamic equations, both for the 2D BCS equation of state and for the 3D unitary quantum gas, predicts that the vortex free gas is subject to a dynamic instability for fast enough rotation. With a numerical solution of the full time dependent BCS equations in a 2D model, we confirm the existence of this dynamic instability and we show that it leads to the formation of a regular pattern of quantum vortices in the gas.
Similar content being viewed by others
References
K.M. O'Hara, S.L. Hemmer, M.E. Gehm, S.R Granade, J.E. Thomas, Science 298, 2179 (2002)
T. Bourdel, J. Cubizolles, L. Khaykovich, K.M.F. Magalhães, S.J.J.M.F. Kokkelmans, G.V. Shlyapnikov, C. Salomon, Phys. Rev. Lett. 91, 020402 (2003)
A.G. Leggett, J. Phys. (Paris) C 7, 19 (1980); P. Nozières, S. Schmitt-Rink, J. Low Temp. Phys. 59, 195 (1985); J.R. Engelbrecht, M. Randeria, C. Sá de Melo, Phys. Rev. B 55, 15153 (1997)
M. Greiner, C.A. Regal, D.S. Jin, Nature 426, 537 (2003); S. Jochim, M. Bartenstein, A. Altmeyer, G. Hendl, S. Riedl, C. Chin, J. Hecker Denschlag, R. Grimm, Science 302, 2101 (2003); M.W. Zwierlein, C.A. Stan, C.H. Schunck, S.M.F. Raupach, S. Gupta, Z. Hadzibabic, W. Ketterle, Phys. Rev. Lett. 91, 250401 (2003); T. Bourdel, L. Khaykovich, J. Cubizolles, J. Zhang, F. Chevy, M. Teichmann, L. Tarruell, S.J.J.M.F. Kokkelmans, C. Salomon, Phys. Rev. Lett. 93, 050401 (2004)
C.A. Regal, M. Greiner, D.S. Jin, Phys. Rev. Lett. 92, 040403 (2004); M.W. Zwierlein, C.A. Stan, C. H. Schunck, S.M.F. Raupach, A.J. Kerman, W. Ketterle, Phys. Rev. Lett. 92, 120403 (2004)
C. Chin, M. Bartenstein, A. Altmeyer, S. Riedl, S. Jochim, J. Hecker Denschlag, R. Grimm, Science 305, 1128 (2004)
P. Törmä, P. Zoller, Phys. Rev. Lett. 85, 487 (2000)
C. Menotti, P. Pedri, S. Stringari, Phys. Rev. Lett. 89, 250402 (2002)
M. Cozzini, S. Stringari, Phys. Rev. Lett. 91, 070401 (2003)
D.L. Feder, Phys. Rev. Lett. 93, 200406 (2004)
N. Nygaard, G.M. Bruun, C.W. Clark, D.L. Feder, Phys. Rev. Lett. 90, 210402 (2003)
T. Isoshima, K. Machida, Phys. Rev. A 60, 3313 (1999)
F. Dalfovo, S. Stringari, Phys. Rev. A 63, 011601(R) (2000)
K.W. Madison, F. Chevy, W. Wohlleben, J. Dalibard, Phys. Rev. Lett. 84, 806 (2000)
S. Sinha, Y. Castin, Phys. Rev. Lett. 87, 190402 (2001)
K. Madison, F. Chevy, V. Bretin, J. Dalibard, Phys. Rev. Lett. 86, 4443 (2001)
E. Hodby, G. Hechenblaikner, S.A. Hopkins, O.M. Maragò, C.J. Foot, Phys. Rev. Lett. 88, 010405 (2002)
D.L. Feder, A.A. Svidzinsky, A.L. Fetter, C.W. Clark, Phys. Rev. Lett. 86, 564 (2001)
C. Lobo, A. Sinatra, Y. Castin, Phys. Rev. Lett. 92, 020403 (2004)
D.S. Petrov, M. Holzmann, G. Shlyapnikov, Phys. Rev. Lett. 84, 2551 (2000); D.S. Petrov, G.V. Shlyapnikov, Phys. Rev. A 64, 012706 (2001); G. Shlyapnikov, J. Phys. IV France 116, 89 (2004)
L. Pricoupenko, M. Olshanii, arXiv:cond-mat/0205210
M. Randeria, J. Duan, L. Shieh, Phys. Rev. B 41, 327 (1990)
C. Mora, Y. Castin, Phys. Rev. A 67, 053615 (2003)
Y. Castin, J. Phys. IV France 116, 89 (2004)
G. Tonini, “Study of Ultracold Fermi gases: crystalline LOFF phase and nucleation of vortices”, Ph.D. thesis of the University of Florence (Italy)
At the border of the atomic cloud, the gap parameter assumes very small values, which locally (but not globally) invalidates the hydrodynamic approximation. Such a failure of hydrodynamics close to the boundaries of a trapped gas is well-known for Bose condensates Stringari. Moreover, hydrodynamics will fail when turbulence develops and vortices enter the cloud, since hydrodynamics does not include properly short length scale variations of |Δ|. For all these reasons, we have performed numerical simulations of the full time dependent BCS theory, as presented in this paper
S. Stringari, Phys. Rev. Lett. 77, 2360 (1996)
We note that the chemical potential μ is a function of the rotation frequency Ω, since we are here in a system with a fixed total number of particles. We do not need here the explicit expression for μ
A. Recati, F. Zambelli, S. Stringari, Phys. Rev. Lett. 86, 377 (2001)
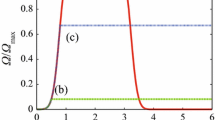
In this figure, it is not apparent that the very narrow tongues actually touch the epsilon=0 axis; but this can be shown analytically, and the contact points are \(\Omega=\omega/\sqrt{n}\), which is the value of the rotation frequency ensuring the resonance of the rotating anisotropy with the surface mode of frequency \(\sqrt{n}\omega\) in the lab frame Subhasis. Note also that the degrees n=6 and n=7 (not shown in the figure) have each an additional instability zone, however with very low values of the Lyapunov exponent (∼ 0.01ω) so of little physical relevance. We have not explored n≥8
S. Sinha, private communication
This value is obtained from the following result: the boundaries of the crescent of degree n=3 can be parametrized as epsilon=±z0 (-z0 2+2Ω2-1)/Ω where z0 is a root of the degree 5 polynomial P(z)=3 z5+19 Ωz4 +(6-13 Ω2) z3 +(26 Ω-25 Ω3) z2 + (3+3Ω4-10Ω2) z +3Ω5-22Ω3 + 7Ω, Ω being expressed in units of ω
J. Carlson, S.-Y. Chang, V.R. Pandharipande, K.E. Schmidt, Phys. Rev. Lett. 91, 050401 (2003)
G.E. Astrakharchik, J. Boronat, J. Casulleras, S. Giorgini, Phys. Rev. Lett. 93, 200404 (2004)
M. Bartenstein, A. Altmeyer, S. Riedl, S. Jochim, C. Chin, J. Hecker Denschlag, R. Grimm, Phys. Rev. Lett. 92, 120401 (2004)
M. Amoruso, I. Meccoli, A. Minguzzi, M.P. Tosi, Eur. Phys. J. D 7, 441 (1999)
J.-P. Blaizot, G. Ripka, Quantum Theory of Finite Systems (The MIT Press, Cambridge, Massachusetts, 1986)
The mean field term is simply g0 ρ/2. It tends to zero in the limit of a grid spacing l tending to zero, both in the 3D and 2D BCS theories. In 2D however, this convergence is only logarithmic so it can never be achieved in a practical numerical calculation. Keeping this mean field term introduces a spurious dependence on l in the BCS equation of state. This, as we have checked numerically, leads to a spurious dependence on l of the density profile and the gap parameter for a stationary gas in the trap in the BCS approximation: we have therefore removed the mean field term. In an exact many-body solution of the lattice model, not relying on the Born approximation as the Hartree-Fock mean field term does, we do not expect such a slow convergence in the l→0 limit
Shortly after submission of this paper, the observation of a vortex lattice in a 3D superfluid Fermi gas was reported, see M.W. Zwierlein, J.R. Abo-Shaeer, A. Schirotzek, C.H. Schunck, W. Ketterle, Nature 435, 1047 (2005)
G. Tonini, F. Werner, Y. Castin, arXiv:cond-mat/0504612 version 2
E.P. Wigner, Phys. Rev. 40, 749 (1932)
A. Messiah, in Quantum Mechanics (North Holland, 1961); Vol. II A.B. Migdal, in Qualitative methods in Quantum Theory (W.A. Benjamin, Massachusetts, 1977), p. 155
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Tonini, G., Werner, F. & Castin, Y. Formation of a vortex lattice in a rotating BCS Fermi gas. Eur. Phys. J. D 39, 283–294 (2006). https://doi.org/10.1140/epjd/e2006-00110-7
Received:
Published:
Issue Date:
DOI: https://doi.org/10.1140/epjd/e2006-00110-7