Nuclear mass form factors from coherent photoproduction of πmesons

Abstract.

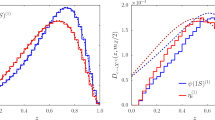
Data for coherent photoproduction of π⁰ mesons from nuclei ( ¹²C, ⁴⁰Ca, ⁹³Nb, ^natPb), recently measured with the TAPS detector at the Mainz MAMI accelerator, have been analyzed in view of the mass form factors of the nuclei. The form factors have been extracted in plane-wave approximation of the A(γ,π⁰)A reaction and corrected for final-state interaction effects with the help of distorted-wave impulse approximations. Nuclear mass rms radii have been calculated from the slope of the form factors for q² → 0. Furthermore, the Helm model (hard-sphere form factor folded with Gaussian) was used to extract diffraction radii from the zeroes of the form factor and skin thicknesses from the position and height of its first maximum. The diffraction radii from the Helm model agree with the corresponding charge radii obtained from electron scattering experiments within their uncertainties of a few per cent. The rms radii from the slope of the form factors are systematically lower by up to 5% for PWIA and up to 10% for DWIA. Also the skin thicknesses extracted from the Helm model are systematically smaller than their charge counterparts.