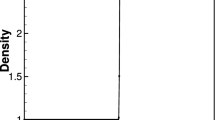
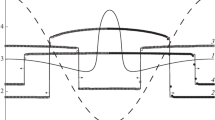
Abstract
Numerical analysis has shown that high order finite-volume WENO schemes have only the first order of convergence in the smooth part of a weak solution behind a shock front. The order of integral convergence of the difference solution is found to estimate the accuracy of the translation of the Rankine-Hugoniot conditions through the shock front.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
J. Casper and M. H. Carpenter, “Computational consideration for the simulation of shock-induced sound,” SIAM J. Sci. Comput. 19, 813–828 (1998).
B. Engquist and B. Sjogreen, “The convergence rate of finite difference schemes in the presence of shocks,” SIAM J. Numer. Anal. 34, 2464–2485 (1998).
V. V. Ostapenko, “Convergence of finite-difference schemes behind a shock front,” Comput. Math. Math. Phys. 37, 1161–1172 (1997).
B. L. Rozhdestvenskii and N. N. Yanenko, Systems of Quasilinear Equations and their Applications to Gas Dynamics (Nauka, Moscow, 1978; Amer. Math. Soc., Providence, 1983).
V. V. Ostapenko, “Finite-difference approximation of the Hugoniot conditions on a shock front propagating with variable velocity,” Comput. Math. Math. Phys. 38, 1299–1311 (1998).
V. V. Ostapenko, “On convergence of high order shock capturing difference schemes,” AIP Conf. Proc. 1301, 413–425 (2010).
C.-W. Shu, “Essentially non-oscillatory and weighted essentially non-oscillatory schemes for hyperbolic conservations laws,” ICASE Report No. 97–65 (1997).
N. K. Yamaleev and M. H. Carpenter, “A systematic methodology for constructing high-order energy stable WENO schemes,” J. Comput. Phys. 228, 4248–4272 (2009).
R. Borgers, M. Carmona, B. Costa, and W. S. Don, “An improved weighted essentially non-oscillatory scheme for hyperbolic conservation laws,” J. Comput. Phys. 227, 3191–3211 (2008).
E. F. Toro, Riemann Solvers and Numerical Methods for Fluid Dynamics. A Practical Introduction (Springer, Heidelberg, 2009).
S. Gottlieb, C.-W. Shu, and E. Tadmor, “Strong stability preserving high-order time discretization methods,” SIAM Rev. 43, 89–112 (2001).
V. V. Ostapenko, “Construction of high-order accurate shock-capturing finite difference schemes for unsteady shock waves,” Comput. Math. Math. Phys. 40, 1784–1800 (2000).
S. Gottlieb, D. Gottlieb, and C.-W. Shu, “Recovering high-order accuracy in WENO compuatations of steadystate hyperbolic systems,” J. Sci. Comput. 28, 307–318 (2006).
Author information
Authors and Affiliations
Corresponding author
Additional information
Original Russian Text © N.A. Mikhailov, 2015, published in Matematicheskoe Modelirovanie, 2015, Vol. 27, No. 2, pp. 129–138.
Rights and permissions
About this article
Cite this article
Mikhailov, N.A. The convergence order of weno schemes behind a shock front. Math Models Comput Simul 7, 467–474 (2015). https://doi.org/10.1134/S2070048215050075
Received:
Published:
Issue Date:
DOI: https://doi.org/10.1134/S2070048215050075