Abstract
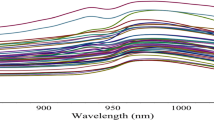
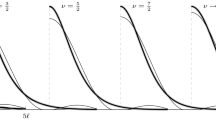
A smoother based on an adaptive cubic model [1, 2] and splines with free knots is proposed. The model uses three reference data points and two parameters of control for estimation of a near optimal position of knots at the axis x in autotracking mode. The data points are prethinned and corrected by local linear fitting. The coefficient table is obtained by standard spline procedure. The efficiency and the stability of the smoother w.r.t. random errors are shown on real noisy data.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
N. D. Dikoussar, “Local Cubic Approximation and Smoothing of Curves in Adaptation Mode,” JINR Commun. P10-99-168 (Dubna, 1999); N. D. Dikoussar, “A Local Cubic Smoothing in an Adaptation Mode,” JINR Preprint E10-2001-48 (Dubna, 2001).
N. D. Dikoussar and Cs. Török, “Automatic Knot Finding for Piecewise-Cubic Approximation,” Mathem. Model. 18(3), 23–40 (2006).
W. Härdle, Applied Nonparametric Regression (Cambridge Univ., Cambridge, 1990).
J. Friedman, A Variable Span Smoother, SLAC PUB-3477 (Stanford, 1984).
B. W. Silverman, “A Fast and Efficient Cross-Validation Method for Smoothing Parameter Choice in Spline Regression,” J. Amer. Statist. Assn. 19 (1984).
N. D. Dikoussar, “Discrete Projective Transformations on the Coordinate Plane,” Mathem. Model. 1(3), 50–64 (1991).
N. D. Dikoussar, “Adaptive Projective Filters for Track Finding,” Comp. Phys. Commun. 79, 39–51 (1994).
N. D. Dikoussar, “Function Parametrization by Using 4-Point Transforms,” Comp. Phys. Commun. 99, 235–254 (1997).
Cs. Török and N. D. Dikoussar, “MS.NET Components for Piecewise-Cubic Approximation,” JINR Commun. P10-2004-202 (Dubna, 2004).
M. Révayová and Cs. Török, “Piecewise Approximation and Neural Networks,” Kibernetika 43(4), (2007).
The European Phys. J. C. Review of Particle Physics (Springer, 2000), p. 235.
Author information
Authors and Affiliations
Additional information
The text was submitted by the authors in English.