Tomography of Qubit States and Implementation of Quantum Algorithms by Unipolar Pulses

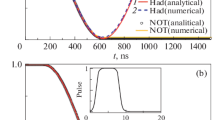
Abstract

The conventional technique for controlling the states of individual qubits and quantum registers is based on the use of high-frequency modulated pulses whose carrier frequency is close to the transition frequency between the ground states of “artificial atoms” and “molecules” (the Rabi technique). In this paper, an alternative concept for controlling superconducting qubits is developed that is based on the idea of fast excitation of a quantum system by solitary unmodulated pulses. The formation of entangled Bell states in a two-qubit superconducting register under the action of field pulses obtained by rapid single flux quantum (RSFQ) devices is demonstrated by numerical simulation. The tomography of quantum states is performed by solving the density matrix equation. It is shown that the technique of “unipolar pulses” allows one to speed up the formation of entangled states (at picosecond times) with a fidelity of 95–98%. The execution of the basic quantum algorithms is demonstrated: the Deutsch–Jozsa algorithm with an accuracy of more than 98% and Grover’s algorithm with an accuracy of 93%.

Appendices

APPENDIX A

In the case of identical qubits (Δ₁ = Δ₂ = Δ), the evolution operators U(τ₁), U(τ_J), and U(τ₂) are defined in matrix form as

$$U_{{{{\tau }_{1}}}}^{{(12)}} = \left( {\begin{array}{*{20}{c}} {{{e}^{{ - \frac{{i\Delta {{\tau }_{1}}}}{2}}}}\left( {\cos \frac{{{{\tau }_{1}}\Omega }}{2} - \frac{{i\Delta }}{\Omega }\sin \frac{{{{\tau }_{1}}\Omega }}{2}} \right)}&0&{ - \frac{{iA}}{\Omega }{{e}^{{ - \frac{{i\Delta {{\tau }_{1}}}}{2}}}}\sin \frac{{{{\tau }_{1}}\Omega }}{2}}&0 \\ 0&{{{e}^{{\frac{{i\Delta {{\tau }_{1}}}}{2}}}}\left( {\cos \frac{{{{\tau }_{1}}\Omega }}{2} - \frac{{i\Delta }}{\Omega }\sin \frac{{{{\tau }_{1}}\Omega }}{2}} \right)}&0&{ - \frac{{iA}}{\Omega }{{e}^{{\frac{{i\Delta {{\tau }_{1}}}}{2}}}}\sin \frac{{{{\tau }_{1}}\Omega }}{2}} \\ { - \frac{{iA}}{\Omega }{{e}^{{ - \frac{{i\Delta {{\tau }_{1}}}}{2}}}}\sin \frac{{{{\tau }_{1}}\Omega }}{2}}&0&{{{e}^{{\frac{{i\Delta {{\tau }_{1}}}}{2}}}}\left( {\cos \frac{{{{\tau }_{1}}\Omega }}{2} - \frac{{i\Delta }}{\Omega }\sin \frac{{{{\tau }_{1}}\Omega }}{2}} \right)}&0 \\ 0&{ - \frac{{iA}}{\Omega }{{e}^{{\frac{{i\Delta {{\tau }_{1}}}}{2}}}}\sin \frac{{{{\tau }_{1}}\Omega }}{2}}&0&{{{e}^{{\frac{{i\Delta {{\tau }_{1}}}}{2}}}}\left( {\cos \frac{{{{\tau }_{1}}\Omega }}{2} + \frac{{i\Delta }}{\Omega }\sin \frac{{{{\tau }_{1}}\Omega }}{2}} \right)} \end{array}} \right),$$

$$U_{{{{\tau }_{J}}}}^{{(12)}} = \left( {\begin{array}{*{20}{c}} {\cos \frac{{{{\tau }_{J}}{{\Omega }_{J}}}}{2} - \frac{{2i\Delta }}{{{{\Omega }_{J}}}}\sin \frac{{{{\tau }_{J}}{{\Omega }_{J}}}}{2}}&0&0&{ - \frac{{iJ}}{{{{\Omega }_{J}}}}\sin \frac{{{{\tau }_{J}}{{\Omega }_{J}}}}{2}} \\ 0&{\cos \frac{{{{\tau }_{J}}{{\Omega }_{J}}}}{2}}&{ - i\sin \frac{{{{\tau }_{J}}{{\Omega }_{J}}}}{2}}&0 \\ 0&{ - i\sin \frac{{{{\tau }_{J}}{{\Omega }_{J}}}}{2}}&{\cos \frac{{{{\tau }_{J}}{{\Omega }_{J}}}}{2}}&0 \\ { - \frac{{iJ}}{{{{\Omega }_{J}}}}\sin \frac{{{{\tau }_{J}}{{\Omega }_{J}}}}{2}}&0&0&{\cos \frac{{{{\tau }_{J}}{{\Omega }_{J}}}}{2} + \frac{{2i\Delta }}{{{{\Omega }_{J}}}}\sin \frac{{{{\tau }_{J}}{{\Omega }_{J}}}}{2}} \end{array}} \right),$$

$$U_{{{{\tau }_{2}}}}^{{(12)}} = \left( {\begin{array}{*{20}{c}} {{{e}^{{ - \frac{{i\Delta {{\tau }_{2}}}}{2}}}}\left( {\cos \frac{{{{\tau }_{2}}\Omega }}{2} - \frac{{i\Delta }}{\Omega }\sin \frac{{{{\tau }_{2}}\Omega }}{2}} \right)}&{ - \frac{{iA}}{\Omega }{{e}^{{ - \frac{{i\Delta {{\tau }_{2}}}}{2}}}}\sin \frac{{{{\tau }_{2}}\Omega }}{2}}&0&0 \\ { - \frac{{iA}}{\Omega }{{e}^{{ - \frac{{i\Delta {{\tau }_{2}}}}{2}}}}\sin \frac{{{{\tau }_{2}}\Omega }}{2}}&{{{e}^{{ - \frac{{i\Delta {{\tau }_{2}}}}{2}}}}\left( {\cos \frac{{{{\tau }_{2}}\Omega }}{2} + \frac{{i\Delta }}{\Omega }\sin \frac{{{{\tau }_{2}}\Omega }}{2}} \right)}&0&0 \\ 0&0&{{{e}^{{\frac{{i\Delta {{\tau }_{2}}}}{2}}}}\left( {\cos \frac{{{{\tau }_{2}}\Omega }}{2} - \frac{{i\Delta }}{\Omega }\sin \frac{{{{\tau }_{2}}\Omega }}{2}} \right)}&{ - \frac{{iA}}{\Omega }{{e}^{{\frac{{i\Delta {{\tau }_{2}}}}{2}}}}\sin \frac{{{{\tau }_{2}}\Omega }}{2}} \\ 0&0&{ - \frac{{iA}}{\Omega }{{e}^{{\frac{{i\Delta {{\tau }_{2}}}}{2}}}}\sin \frac{{{{\tau }_{2}}\Omega }}{2}}&{{{e}^{{\frac{{i\Delta {{\tau }_{2}}}}{2}}}}\left( {\cos \frac{{{{\tau }_{2}}\Omega }}{2} + \frac{{i\Delta }}{\Omega }\sin \frac{{{{\tau }_{2}}\Omega }}{2}} \right)} \end{array}} \right),$$

where Ω = $\sqrt {{{A}^{2}} + {{\Delta }^{2}}}$ and Ω_J = $\sqrt {{{J}^{2}} + 4{{\Delta }^{2}}}$.

The transition probabilities between the ground states of the two-qubit system, W_x = |〈x|$U_{{{{\tau }_{2}}}}^{{(12)}}U_{{{{\tau }_{J}}}}^{{(12)}}U_{{{{\tau }_{1}}}}^{{(12)}}$|00〉|², where x = 1 corresponds to the ground state |x〉 = |0₁0₂〉, x = 2, to |x〉 = |0₁1₂〉, x = 3, to |x〉 = |1₁0₂〉, and x = 4, to |x〉 = |1₁1₂〉, are defined as

$$\begin{gathered} {{W}_{2}} = \frac{{{{A}^{2}}}}{{{{\Omega }^{4}}\Omega _{J}^{2}}}\left( {{{{\sin }}^{2}}\frac{{{{\tau }_{1}}\Omega }}{2}{{{\left( {2{{\Delta }^{2}}\sin \frac{{{{\tau }_{1}}\Omega }}{2}\sin \frac{{{{\tau }_{J}}{{\Omega }_{J}}}}{2} + \left( { - \Delta \sin \frac{{{{\tau }_{J}}J}}{2}\sin \frac{{{{\tau }_{1}}\Omega }}{2} + \Omega \cos \frac{{{{\tau }_{1}}\Omega }}{2}\cos \frac{{{{\tau }_{J}}{{\Omega }_{J}}}}{2}} \right){{\Omega }_{J}}} \right)}}^{2}}} \right. \\ \left. { + {{{\left( {2\Delta \cos \frac{{{{\tau }_{1}}\Omega }}{2}\sin \frac{{{{\tau }_{2}}\Omega }}{2}\sin \frac{{{{\tau }_{J}}{{\Omega }_{J}}}}{2}\Omega + \sin \frac{{{{\tau }_{1}}\Omega }}{2}\left( {\Delta \cos \frac{{{{\tau }_{J}}{{\Omega }_{J}}}}{2}\sin \frac{{{{\tau }_{2}}\Omega }}{2} + \cos \frac{{{{\tau }_{2}}\Omega }}{2}\sin \frac{{{{\tau }_{J}}J}}{2}\Omega } \right){{\Omega }_{J}}} \right)}}^{2}}} \right), \\ \end{gathered} $$

(A.1)

$$\begin{gathered} {{W}_{3}} = \frac{{{{A}^{2}}}}{{{{\Omega }^{4}}\Omega _{J}^{2}}}\left( {{{{\sin }}^{2}}\frac{{{{\tau }_{2}}\Omega }}{2}{{{\left( {J\Omega \cos \frac{{{{\tau }_{1}}\Omega }}{2}\sin \frac{{{{\tau }_{J}}{{\Omega }_{J}}}}{2} + \Delta {{\Omega }_{J}}\cos \frac{{{{\tau }_{J}}\Omega }}{2}\sin \frac{{{{\tau }_{1}}\Omega }}{2}} \right)}}^{2}}} \right. \\ \left. { + {{{\sin }}^{2}}\frac{{{{\tau }_{1}}\Omega }}{2}{{{\left( {J\Delta \sin \frac{{{{\tau }_{2}}\Omega }}{2}\sin \frac{{{{\tau }_{J}}{{\Omega }_{J}}}}{2} - \Omega {{\Omega }_{J}}\cos \frac{{{{\tau }_{J}}J}}{2}\cos \frac{{{{\tau }_{2}}\Omega }}{2}} \right)}}^{2}}} \right), \\ \end{gathered} $$

(A.2)

$$\begin{gathered} {{W}_{4}} = \frac{1}{{4{{\Omega }^{4}}\Omega _{J}^{2}}}\left( {{{J}^{2}}{{{\left( {({{A}^{2}} + 2{{\Delta }^{2}})\cos \frac{{({{\tau }_{1}} - {{\tau }_{2}})\Omega }}{2} + {{A}^{2}}\cos \frac{{({{\tau }_{1}} + {{\tau }_{2}})\Omega }}{2}} \right)}}^{2}}{{{\sin }}^{2}}\frac{{({{\tau }_{1}} - {{\tau }_{2}})\Delta }}{2}} \right.{{\sin }^{2}}\frac{{{{\tau }_{J}}{{\Omega }_{J}}}}{2} \\ \left. { + 4{{{\cos }}^{2}}\frac{{({{\tau }_{1}} - {{\tau }_{2}})\Delta }}{2}{{{\left( { - {{A}^{2}}{{\Omega }_{J}}\cos \frac{{{{\tau }_{J}}J}}{2}\sin \frac{{{{\tau }_{1}}\Omega }}{2}\sin \frac{{{{\tau }_{2}}\Omega }}{2} - J\Delta \Omega \sin \frac{{({{\tau }_{1}} - {{\tau }_{2}})\Omega }}{2}\sin \frac{{{{\tau }_{J}}{{\Omega }_{J}}}}{2}} \right)}}^{2}}} \right). \\ \end{gathered} $$

(A.3)

APPENDIX B

For numerical calculations, it is convenient to represent the density matrix as an expansion in terms of the full set of matrices of the SU(4) group, which represent the Kronecker (direct) product of matrices for the SU(2) group: ρ = $\sum\nolimits_{\alpha ,\beta }^{} {{{R}_{{\alpha ,\beta }}}{\boldsymbol{\sigma }}_{\alpha }^{{(1)}}} \otimes {\boldsymbol{\sigma }}_{\beta }^{{(2)}}$, R_{α, β} = Tr(${\boldsymbol{\sigma }}_{\alpha }^{{(1)}} \otimes {\boldsymbol{\sigma }}_{\beta }^{{(2)}}$ρ) are the vector components (16 elements) and ${\boldsymbol{\sigma }}_{\alpha }^{{(i)}}$ = {$\sigma _{0}^{{(i)}}$, $\sigma _{x}^{{(i)}}$, $\sigma _{y}^{{(i)}}$, $\sigma _{z}^{{(i)}}$} is the set of matrices, where $\sigma _{0}^{{(i)}}$ = I⁽ⁱ⁾ is the 2 × 2 identity matrix and $\sigma _{{x,y,z}}^{{(i)}}$ are the Pauli matrices. The density matrix equation (13) is equivalent to the equations for the vector components R_{α, β} (15 nonzero components and R_{0, 0} = 0):

$$\begin{gathered} {{{\dot {R}}}_{{x,0}}} = 4(J{{R}_{{y,z}}} - {{R}_{{x,0}}}{{\gamma }_{1}} + {{R}_{{y,0}}}{{\varepsilon }_{1}}(t)), \\ {{{\dot {R}}}_{{y,0}}} = - 4(J{{R}_{{x,z}}} + {{R}_{{y,0}}}{{\gamma }_{1}} - {{R}_{{z,0}}}{{\Delta }_{1}} + {{R}_{{x,0}}}{{\varepsilon }_{1}}(t)), \\ {{{\dot {R}}}_{{z,0}}} = - 4{{R}_{{y,0}}}{{\Delta }_{1}}, \\ \end{gathered} $$

$$\begin{gathered} {{{\dot {R}}}_{{0,x}}} = 4(J{{R}_{{z,y}}} - {{R}_{{0,x}}}{{\gamma }_{2}} + {{R}_{{0,y}}}{{\varepsilon }_{2}}(t)), \\ {{{\dot {R}}}_{{0,y}}} = - 4(J{{R}_{{z,x}}} + {{R}_{{0,y}}}{{\gamma }_{2}} - {{R}_{{0,z}}}{{\Delta }_{2}} + {{R}_{{0,x}}}{{\varepsilon }_{2}}(t)), \\ {{{\dot {R}}}_{{0,z}}} = - 4{{R}_{{0,y}}}{{\Delta }_{2}}, \\ \end{gathered} $$

$$\begin{gathered} {{{\dot {R}}}_{{x,x}}} = 4( - {{R}_{{x,x}}}({{\gamma }_{1}} + {{\gamma }_{2}}) + {{R}_{{y,x}}}{{\varepsilon }_{1}}(t) + {{R}_{{x,y}}}{{\varepsilon }_{2}}(t)), \\ {{{\dot {R}}}_{{x,y}}} = - 4({{R}_{{x,y}}}({{\gamma }_{1}} + {{\gamma }_{2}}) - {{R}_{{x,z}}}{{\Delta }_{2}} - {{R}_{{y,y}}}{{\varepsilon }_{1}}(t) + {{R}_{{x,x}}}{{\varepsilon }_{2}}(t)), \\ {{{\dot {R}}}_{{x,z}}} = 4(J{{R}_{{y,0}}} - {{R}_{{x,z}}}{{\gamma }_{1}} - {{R}_{{x,y}}}{{\Delta }_{2}} + {{R}_{{y,z}}}{{\varepsilon }_{1}}(t)), \\ \end{gathered} $$

(B.1)

$$\begin{gathered} {{{\dot {R}}}_{{y,x}}} = - 4({{R}_{{y,x}}}({{\gamma }_{1}} + {{\gamma }_{2}}) - {{R}_{{z,x}}}{{\Delta }_{1}} + {{R}_{{x,x}}}{{\varepsilon }_{2}}(t) - {{R}_{{y,y}}}{{\varepsilon }_{2}}(t)), \\ {{{\dot {R}}}_{{y,y}}} = - 4({{R}_{{y,y}}}({{\gamma }_{1}} + {{\gamma }_{2}}) - {{R}_{{z,y}}}{{\Delta }_{1}} - {{R}_{{y,z}}}{{\Delta }_{2}} + {{R}_{{x,y}}}{{\varepsilon }_{1}}(t) + {{R}_{{y,x}}}{{\varepsilon }_{2}}(t)), \\ {{{\dot {R}}}_{{y,z}}} = - 4(J{{R}_{{x,0}}} + {{R}_{{y,z}}}{{\gamma }_{1}} - {{R}_{{z,z}}}{{\Delta }_{1}} + {{R}_{{y,y}}}{{\Delta }_{2}} + {{R}_{{x,z}}}{{\varepsilon }_{1}}(t)), \\ {{{\dot {R}}}_{{z,x}}} = 4(J{{R}_{{0,y}}} - {{R}_{{z,x}}}{{\gamma }_{2}} - {{R}_{{y,x}}}{{\Delta }_{1}} + {{R}_{{z,y}}}{{\varepsilon }_{2}}(t)), \\ {{{\dot {R}}}_{{z,y}}} = - 4(J{{R}_{{0,x}}} + {{R}_{{z,y}}}{{\gamma }_{2}} + {{R}_{{y,y}}}{{\Delta }_{1}} - {{R}_{{z,z}}}{{\Delta }_{2}} + {{R}_{{z,x}}}{{\varepsilon }_{2}}(t)), \\ {{{\dot {R}}}_{{z,z}}} = - 4({{R}_{{y,z}}}{{\Delta }_{1}} + {{R}_{{z,y}}}{{\Delta }_{2}}). \\ \end{gathered} $$

At the initial time at t = 0, the system of two qubits is in the ground state, i.e., the density matrix is constructed on the eigenfunctions of the unperturbed Hamiltonian (7), when A₁ = A₂ = 0 and J = 0 in (4), and is defined by the expression ρ(0) = $\sum\nolimits_i^{} |$ψ_in(0)〉〈ψ_in(0)|. The vector components R_{α, β} are defined as R_{α, β}(0) = Tr(${\boldsymbol{\sigma }}_{\alpha }^{{(1)}} \otimes {\boldsymbol{\sigma }}_{\beta }^{{(2)}}$ρ(0)). The level populations of the qubits (the diagonal elements of the density matrix W_i(t) = ρ_ii) are calculated in terms of the vector components R_{α, β} and have the form

$$\begin{gathered} {{W}_{1}}(t) = \frac{{1 + {{R}_{{z,0}}} + {{R}_{{0,z}}} + {{R}_{{z,z}}}}}{4}, \\ {{W}_{2}}(t) = \frac{{1 + {{R}_{{z,0}}} - {{R}_{{0,z}}} - {{R}_{{z,z}}}}}{4}, \\ {{W}_{3}}(t) = \frac{{1 + {{R}_{{0,z}}} - {{R}_{{z,0}}} - {{R}_{{z,z}}}}}{4}, \\ {{W}_{4}}(t) = \frac{{1 - {{R}_{{z,0}}} - {{R}_{{0,z}}} + {{R}_{{z,z}}}}}{4}. \\ \end{gathered} $$

(B.2)