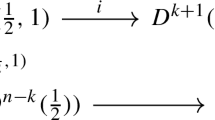Abstract
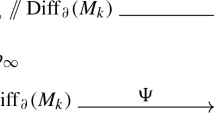
On a Riemannian manifold X, we consider aK+s, where a is a nonnegative constant, K is the sectional curvature and s is the scalar curvature. It is shown that if X admits a metric with aK+s > 0, then so does any manifold obtained from X by surgeries of codimension ≥3. This implies the existence of such metrics on certain compact simply connected manifolds of dimension ≥5 by using the cobordism argument. We also study the corresponding minimal volume problem. As a corollary, we derive that every compact simply connected manifold of dimension ≥5 and every compact complex surface of Kodaira dimension ≤1 whose minimal model is not of Class VII collapse with aK+s bounded below.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
Barth, W., Peters, C. and Van De Ven, A.: Compact Complex Surfaces, Springer-Verlag, Berlin, 1982.
Besse, A.: Einstein Manifolds, Springer-Verlag, Berlin, 1987.
Donaldson, S. K.: Connection, cohomology and the intersection forms of 4-manifolds, J. Differential Geom. 24(1986), 275–341.
Freedman, M.: On the topology of 4-manifolds, J. Differential Geom. 17(1982), 357–454.
Gompf, R. and Stipsicz, A.: 4-Manifolds and Kirby Calculus, Amer. Math. Soc. Providence, 1999.
Gromov, M. and Lawson, H. B.: The classification of simply connected manifolds of positive scalar curvature, Ann. Math. 111(1980), 423–434.
Hitchin, N.: Harmonic spinors, Adv. Math. 14(1974), 1–55.
Joyce, D.: Compact 8-manifolds with Holonomy Spin(7), Invent. Math. 123(1996), 507–552.
Lawson, H. B. and Michelson, M. L.: Spin Geometry, Princeton University Press, Princeton, 1987.
LeBrun, C.: Four-manifolds without Einstein metric, Math. Res. Lett. 3(1996), 133–147.
LeBrun, C.: Kodaira dimension and the Yamabe problem, Comm. Anal. Geom. 7(1999), 133–156.
LeBrun, C.: Ricci curvature, minimal volumes, and Seiberg-Witten theory, Invent. Math. 145(2001), 279–316.
Lichnerowicz, A.: Spineurs harmoniques, C.R. Acad. Aci. Paris Ser. A-B 257(1963), 7–9.
Paternain, G. P. and Petean, J.: Minimal entropy and collapsing with curvature bounded from below, Invent. Math. 151(2003), 415–450.
Petean, J.: Computations of the Yamabe invariant, Math. Res. Lett. 5(1998), 703–709.
Petean, J.: The Yamabe invariant of simply connected manifolds, J. Reine Angew. Math. 523(2000), 225–231.
Petersen, P.: Riemannian Geometry, Springer-Verlag, Berlin, 1998.
Rosenberg, J.: C-algebra, positive scalar curvature, and the Novikov conjecture III, Topology 25(1986), 319–336.
Schoen, R. and Yau, S. T.: On the structure of manifolds with positive scalar curvature, Manuscripta Math. 28(1979), 159–183.
Stolz, S.: Simply connected manifolds of positive scalar curvature, Ann. Math. 136(1992), 511–540.
Rights and permissions
About this article
Cite this article
Sung, C. Surgery, Curvature, and Minimal Volume. Annals of Global Analysis and Geometry 26, 209–229 (2004). https://doi.org/10.1023/B:AGAG.0000042898.55061.ef
Issue Date:
DOI: https://doi.org/10.1023/B:AGAG.0000042898.55061.ef