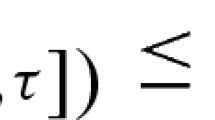Abstract
We investigate the long-time behaviour and the behaviour at singularities of the Yang--Mills heat flow in four dimensions with finite energy initial data. In addition we shall give an upper bound for the total number of singularities.
Similar content being viewed by others
References
Adams, R.: Sobolev Spaces, Academic Press, London, 1978.
Atiyah, M., Hitchin, N. and Singer, I.: Self-duality in four dimensional Riemannian geometry, Proc. Roy. Soc. London Ser. A (1978), 425–461.
Lawson, B.: The Theory of Gauge Fields in Four Dimensions, CBMS Regional Conf. Series 58, Amer. Math. Soc., Providence, 1987.
Bourguignon, J. P. and Lawson, B.: Stability and isolation phenomena for Yang-Mills fields, Comm. Math. Phys. 79 (1981), 189–230.
Donaldson, S. K. and Kronheimer, P.: The Topology of Four-Manifolds, Clarendon Press, Oxford, New York, 1990.
Freed, D. and Uhlenbeck, K.: Instantons and Four-Manifolds, 2nd edition, Springer-Verlag, New York, Berlin, 1991.
Jost, J.: Nonlinear Methods in Riemannian and Kählerian Geometry, DMV Seminar 10, Birkhäuser, Basel, 1991.
Lawson, B. and Michelson, M.-L.: Spin Geometry, Princeton University Press, Princeton, NJ, 1989.
Palais, R.: Foundations of Global Analysis, Springer-Verlag, Berlin, 1969.
Sedlacek, S.: The Yang-Mills functional over four-manifolds, Comm. Math. Phys. 86 (1982), 515–527.
Struwe, M.: The evolution of harmonic maps of Riemannian surfaces, Commentarii Mathematici Helvetici 60 (1985), 558–581.
Struwe, M.: The Yang-Mills flow in four dimensions, Calc. Var. 2 (1994) 123–150.
Uhlenbeck, K.: Connections with Lp — bounds on curvature, Comm. Math. Phys. 83 (1982) 31–42.
Uhlenbeck, K.: Removable singularities in Yang-Mills fields, Comm. Math. Phys. 83 (1982), 11–30.
Uhlenbeck, K.: The Chern classes of Sobolev connections, Comm. Math. Phys. 101 (1985), 449–457.
Author information
Authors and Affiliations
Rights and permissions
About this article
Cite this article
Schlatter, A. Long-Time Behaviour of the Yang--Mills Flow in Four Dimensions. Annals of Global Analysis and Geometry 15, 1–25 (1997). https://doi.org/10.1023/A:1006541305639
Issue Date:
DOI: https://doi.org/10.1023/A:1006541305639