Abstract
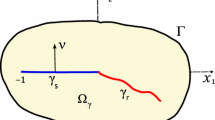
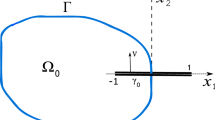
The paper concerns an analysis of equilibrium problems for 2D elastic bodies with a thin Timoshenko inclusion crossing an external boundary at zero angle. The inclusion is assumed to be delaminated, thus forming a crack between the inclusion and the body. We consider elastic inclusions as well as rigid inclusions. To prevent a mutual penetration between the crack faces, inequality type boundary conditions are imposed at the crack faces. Theorems of existence and uniqueness are established. Passages to limits are investigated as a rigidity parameter of the elastic inclusion going to infinity.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
V.A. Kozlov, V.G. Ma’zya, A.B. Movchan, Asymptotic Analysis of Fields in a Multi-Structure, Oxford University Press, New York, 1999.
V.A. Kozlov, V.G. Maz’ya, J. Rossmann, Spectral Problems Associated with Corner Singularities of Solutions to Elliptic Equations, vol. 85, American Mathematical Society, Providence, RI, 2001.
A.M. Khludnev, Elasticity Problems in Non-Smooth Domains, Fizmatlit, Moscow, 2010.
V.A. Kovtunenko, Sensitivity of interfacial cracks to non-linear crack front perturbations, Z. Angev. Math. Mech. 82 (6) (2002) 387–398.
V.A. Kovtunenko, Shape sensitivity of curvilinear cracks on interface to non-linear perturbations, Z. Angew. Math. Phys. 54 (3) (2003) 410–423.
E.M. Rudoi, Differentiation of energy functionals in the problem on a curvilinear crack with possible contact between the shores, Mech. Solids 42 (6) (2007) 935–946.
E.M. Rudoy, Asymptotic behavior of the energy functional for a three-dimensional body with a rigid inclusion and a crack, J. Appl. Mech. Tech. Phys. 52 (2) (2011) 252–263.
A.M. Khludnev, V.A. Kovtunenko, Analysis of Cracks in Solids, WIT Press, Southampton-Boston, 2000.
A. Gaudiello, A.M. Khludnev, Crack on the boundary of two overlapping domain, Z. Angew. Math. Phys. 61 (2) (2010) 341–356.
A.M. Khludnev, G. Leugering, On elastic bodies with thin rigid inclusions and cracks, Math. Methods Appl. Sci. 33 (6) (2010) 1955–1967.
A.M. Khludnev, Thin rigid inclusions with delaminations in elastic plates, Eur. J. Mech. A Solids 32 (1) (2012) 69–75.
A.M. Khludnev, M. Negri, Optimal rigid inclusion shapes in elastic bodies with cracks, Z. Angew. Math. Phys. 64 (1) (2013) 179–191.
N.P. Lazarev, Problem of equilibrium of the Timoshenko plate containing a crack on the boundary of an elastic inclusion with an infinite shear rigidity, J. Appl. Mech. Tech. Phys. 54 (2) (2013) 322–330.
N.P. Lazarev, Shape sensitivity analysis of the energy integrals for the Timoshenko-type plate containing a crack on the boundary of a rigid inclusion, Z. Angew. Math. Phys. 66 (4) (2015) 2025–2040.
N.V. Neustroeva, A rigid inclusion in the contact problem for elastic plates, J. Appl. Ind. Math. 4 (4) (2010) 526–538.
T.A. Rotanova, Unilateral contact problem for two plates with a rigid inclusion in the lower plate, J. Math. Sci. 188 (4) (2013) 452–462.
G. Panasenko, Multi-scale Modelling For Structures and Composites, Springer, New York, 2005.
G. Saccomandi, M.F. Beatty, Universal relations for fiber-reinforced elastic materials, Math. Mech. Solids 7 (1) (2002) 99–110.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Khludnev, A.M., Popova, T.S. Timoshenko inclusions in elastic bodies crossing an external boundary at zero angle. Acta Mech. Solida Sin. 30, 327–333 (2017). https://doi.org/10.1016/j.camss.2017.05.005
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1016/j.camss.2017.05.005