1 Introduction

In current years, fractional calculus (FC) has found to be important in various fields viz fluid dynamics, ecology, financial mathematics [1,2,3,4,5,6]. It is sometimes difficult to obtain the analytical solution of the fractional differential equations (FDEs). Various important research on FC has been deliberated in the past years, and a lot of books have been written by various authors namely Miller and Ross [7], Oldham and Spanier [8], Podlubny [9]. General ideas about FC are introduced in these books which may help the readers to understand the basic concepts of FC. Recently some analytical and numerical techniques have been developed for the solution of physical problems viz. homotopy perturbation method by Wu and He [10], modified homotopy perturbation method by Jena and Chakraverty [11], Adomian decomposition method by Momani and Odibat [12] and Yavuz and Ozdemir [13], and modified decomposition method by Edeki et al. [14].

In the above regard, STM has been found to be a novel method to handle FDEs. The STM was first introduced by Watugala in 1993. This method was implemented to solve various types of engineering control problems by Watugala [15, 16] too. Later, this method was extended to solve two-dimensional engineering problem by Watugala [17]. The significant applications to partial differential equations and inversion formulae were established in two papers by Weerakoon [18, 19] in 1994 and 1998. The Sumudu transform was also first defined by Weerakoon against Deakin’s definition who claimed that there is no difference between the Sumudu and the Laplace and who reminded Weerakoon that the Sumudu transform is really the S-multiplied transform disguised in Deakin [20] and Weerakoon [21]. The solutions of integral equations and discrete dynamical systems of convolution type using STM were later achieved by Asiru [22,23,24]. The Sumudu transform was also used to solve many ordinary differential equations with integer order and although Belgacem’s reasonable advantages for implementing to fractional differential equations commenced in 2008 with various teams of researchers in Katatbeh and Belgacem [25]. It is worth mentioning that novel STM has not been used in solving the Bagley-Torvik equation. So to the best of the present authors’ knowledge, this is the first time that STM has been implemented for solving fractional order Bagley-Torvik equation.

In this paper, the following type of Bagley-Torvik equation is considered

$$ \left\{ {\begin{array}{*{20}l} {m\frac{{d^{2} u\left( x \right)}}{{dx^{2} }} + cD^{\alpha } u\left( x \right) + ku\left( x \right) = f\left( x \right),} \hfill \\ {u\left( 0 \right) = \delta_{0} ,\quad \left. {\frac{du\left( x \right)}{dx}} \right|_{x = 0} = \delta_{1} .} \hfill \\ \end{array} } \right.\quad {\text{where}}\;\;\alpha = \frac{1}{2}\;\;{\text{or}}\;\;\frac{3}{2} $$
(1)

where m, c, k, f(x) and u(x) denote the mass, damping, stiffness coefficients, external force, and displacement function, respectively. \( \frac{{d^{\alpha } u}}{{dx^{\alpha } }} \) is the FD of order \( \alpha \in (0,2) \). Here \( \delta_{0} \) and \( \delta_{1} \) are real constants.

The rest of the manuscript are arranged as follows: some essential definitions related to fractional calculus are included in Sect. 2. Some basic features and theorems of STM are presented in Sect. 3. In Sect. 4, STM is applied to Bagley-Torvik Equation. Finally, a conclusion is illustrated in Sect. 6.

2 Basic features of fractional calculus

Definition 2.1

The operator \( D^{\alpha } \) of order \( \alpha \) in Abel–Riemann (A–R) sense is defined by Podlubny [9] as

$$ D^{\alpha } u(x) = \left\{ {\begin{array}{*{20}l} {\frac{{d^{m} }}{{dx^{m} }}u\left( x \right),} \hfill & {\alpha = m} \hfill \\ {\frac{1}{\varGamma (m - \alpha )}\frac{d}{{dx^{m} }}\int\limits_{0}^{x} {\frac{u(t)}{{(x - t)^{\alpha - m + 1} }}dt} ,} \hfill & {m - 1 < \alpha < m} \hfill \\ \end{array} } \right. $$
(2)

where \( m \in Z^{ + } , \)\( \alpha \in R^{ + } \) and

$$ D^{ - \alpha } u\left( x \right) = \frac{1}{\varGamma \left( \alpha \right)}\int\limits_{0}^{x} {\left( {x - t} \right)^{\alpha - 1} u\left( t \right)dt} ,\quad 0 < \alpha \le 1. $$
(3)

Definition 2.2

The A–R fractional order integration operator \( J^{\alpha } \) is described as

$$ J^{\alpha } u\left( x \right) = \frac{1}{\varGamma \left( \alpha \right)}\int\limits_{0}^{x} {\left( {x - t} \right)^{\alpha - 1} u\left( t \right)dt} ,\quad t > 0,\;\;\alpha > 0 $$
(4)

following Podlubny [9] we may have

$$ J^{\alpha } t^{n} = \frac{{\varGamma \left( {n + 1} \right)}}{{\varGamma \left( {n + \alpha + 1} \right)}}t^{n + \alpha } $$
(5)
$$ D^{\alpha } t^{n} = \frac{{\varGamma \left( {n + 1} \right)}}{{\varGamma \left( {n - \alpha + 1} \right)}}t^{n - \alpha } . $$
(6)

Definition 2.3

The operator \( D^{\alpha } \) of order \( \alpha \) in the Caputo sense is defined in Podlubny [9] and Chakraverty et al. [26] as

$$ {}^{C}D^{\alpha } u(x) = \left\{ {\begin{array}{*{20}l} {\frac{1}{\varGamma (m - \alpha )}\int\limits_{0}^{x} {\frac{{u^{m} (t)}}{{(x - t)^{\alpha - m + 1} }}} dt,} \hfill & {m - 1 < \alpha < m,} \hfill \\ {\frac{{d^{m} }}{{dt^{m} }}u\left( x \right),} \hfill & {\alpha = m} \hfill \\ \end{array} } \right.. $$
(7)

Definition 2.4

Podlubny [9]

  1. (a)
    $$ D_{t}^{\alpha } J_{t}^{\alpha } f\left( t \right) = f\left( t \right) $$
    (8)
  2. (b)
    $$ J_{t}^{\alpha } D_{t}^{\alpha } f\left( t \right) = f\left( t \right) - \sum\limits_{k = 0}^{m} {f^{\left( k \right)} \left( {0^{ + } } \right)} \frac{{t^{k} }}{k!},\;{\text{for}}\;t > 0\;{\text{and}}\;m - 1 < \alpha \le m,\;m \in N. $$

3 Basic properties of STM

Definition 3.1

If \( F\left( u \right) \) is the Sumudu transform (ST) of \( y\left( t \right) \), then the ST of \( y\left( t \right) \) for all real number \( t \ge 0, \) is defined in Weerakoon [18] as

$$ S\left( {y\left( t \right)} \right) = F\left( u \right) = \int\limits_{0}^{\infty } {\frac{1}{u}} \exp \left[ {\frac{t}{u}} \right]y\left( t \right)dt. $$
(9)

Theorem 1

If \( F\left( u \right) \) is the ST of \( y\left( t \right) \), then the ST of \( n^{th} \) order derivative is defined in Belgacem et al. [27] as follows

$$ S\left[ {\frac{{d^{n} }}{{dt^{n} }}y\left( t \right)} \right] = u^{ - n} \left[ {F\left( u \right) - \left. {\sum\limits_{k = 0}^{n - 1} {u^{k} \frac{{d^{k} y\left( t \right)}}{{dt^{k} }}} } \right|_{t = 0} } \right]. $$
(10)

By using Theorem 1, the ST of \( \frac{dy\left( t \right)}{dt} \) and \( \frac{{d^{2} y\left( t \right)}}{{dt^{2} }} \) are given by

$$ S\left[ {\frac{d}{dt}y\left( t \right)} \right] = \frac{1}{u}F\left( u \right) - \frac{1}{u}y\left( 0 \right), $$
(11)
$$ S\left[ {\frac{{d^{2} y\left( t \right)}}{{dt^{2} }}} \right] = \frac{1}{{u^{2} }}\left[ {F\left( u \right) - y\left( 0 \right) - u\left. {\frac{dy\left( t \right)}{dt}} \right|_{t = 0} } \right]. $$
(12)

Theorem 2

The ST of Caputo fractional derivative is well-defined in Chaurasia and Singh [28] as

$$ S\left[ {D_{t}^{\alpha } y\left( t \right)} \right] = u^{ - \alpha } S\left[ {y\left( t \right)} \right] - \sum\limits_{k = 0}^{m - 1} {u^{ - \alpha + k} y^{k} \left( 0 \right),\,\,m - 1 < \alpha \le m} . $$
(13)

4 STM implementation to Bagley-Torvik equations

In this section, STM is implemented to Bagley-Torvik equations of fractional order in the following examples.

Example 1

Let us take following Bagley-Torvik equation given by Pedas and Tamme [29]

$$ \left\{ {\begin{array}{*{20}l} {y^{\left( 2 \right)} \left( t \right) + y^{{\left( {\frac{3}{2}} \right)}} \left( t \right) + y\left( t \right) = \frac{15}{4}\sqrt t + \frac{15}{8}\sqrt \pi t + t^{2} \sqrt t ,} \hfill \\ {y\left( 0 \right) = y^{\left( 1 \right)} \left( 0 \right) = 0.} \hfill \\ \end{array} } \right.\quad t \ge 0. $$
(14)

First, by taking ST of both sides of Eq. (14), we get

$$ S\left[ {y^{\left( 2 \right)} \left( t \right)} \right] + S\left[ {y^{{\left( {\frac{3}{2}} \right)}} \left( t \right)} \right] + S\left[ {y\left( t \right)} \right] = S\left[ {\frac{15}{4}\sqrt t } \right] + S\left[ {\frac{15}{8}\sqrt \pi t} \right] + S\left[ {t^{2} \sqrt t } \right]. $$

which yields

$$ \begin{aligned} & \frac{1}{{u^{2} }}\left[ {F\left( u \right) - y\left( 0 \right) - u\left. {\frac{dy\left( t \right)}{dt}} \right|_{t = 0} } \right] + u^{{\frac{ - 3}{2}}} \left[ {F\left( u \right)} \right] \\ & \quad - \,\sum\limits_{k = 0}^{1} {u^{ - \alpha + k} y^{k} \left( 0 \right) + F\left( u \right) = \frac{15}{8}u^{{\frac{1}{2}}} \sqrt \pi } + \frac{15}{8}\sqrt \pi u + \frac{15}{8}\sqrt \pi u^{{\frac{5}{2}}} . \\ \end{aligned} $$
(15)

Using initial condition to Eq. (15), we get

$$ \begin{aligned} & \frac{1}{{u^{2} }}\left[ {F\left( u \right)} \right] + u^{{\frac{ - 3}{2}}} \left[ {F\left( u \right)} \right] + F\left( u \right) = \frac{15}{8}\sqrt \pi u^{{\frac{1}{2}}} \left( {1 + u^{{\frac{1}{2}}} + u^{2} } \right), \\ &\quad F\left( u \right)\left( {\frac{{1 + u^{2} + u^{{\frac{1}{2}}} }}{{u^{2} }}} \right) = \frac{15}{8}\sqrt \pi u^{{\frac{1}{2}}} \left( {1 + u^{{\frac{1}{2}}} + u^{2} } \right), \\ & \quad \Rightarrow F\left( u \right) = \frac{15}{8}\sqrt \pi u^{{\frac{5}{2}}} . \\ \end{aligned} $$
(16)

Applying inverse ST to Eq. (16) and the table presented by Belgacem and Karaballi in [30], we have

$$ y\left( t \right) = t^{{\frac{5}{2}}} . $$
(17)

This is the analytical solution of Eq. (14).

Example 2

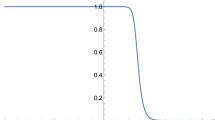
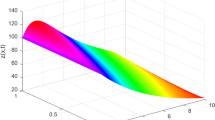
Let us now solve the following Bagley-Torvik equation given in Parisa and Yadollah [31] and (Figs. 1, 2) Mohammadi and Mohyud-Din [32] as

$$ \left\{ {\begin{array}{*{20}l} {y^{{\frac{3}{2}}} \left( t \right) + y\left( t \right) = \frac{{2t^{{\frac{1}{2}}} }}{{\varGamma \left( {\frac{3}{2}} \right)}} + t^{2} - t,} \hfill \\ {y\left( 0 \right) = 0,\quad \left. {\frac{dy\left( t \right)}{dt}} \right|_{t = 0} = - 1,\quad t \ge 0.} \hfill \\ \end{array} } \right. $$
(18)
Fig. 1
figure 1

An inflexible plate of mass m dipped into a Newtonian fluid presented by Gülsu et al. [33]

Full size image
Fig. 2
figure 2

Comparison of the present method with the existing methods a Example 1, b Example 2, c Example 3, d Example 4

Full size image

Applying ST on both sides of Eq. (18) with the initial condition, we get

$$ \begin{aligned} & u^{{\frac{ - 3}{2}}} \left[ {F\left( u \right)} \right] + u^{{\frac{ - 1}{2}}} + F\left( u \right) = 2u^{{\frac{1}{2}}} + 2u^{2} - u, \\ & F\left( u \right)\left( {1 + u^{{\frac{ - 3}{2}}} } \right) = 2u^{{\frac{1}{2}}} + 2u^{2} - u - u^{{\frac{ - 1}{2}}} , \\ & \quad \Rightarrow F\left( u \right) = 2u^{2} - u. \\ \end{aligned} $$
(19)

Taking inverse ST of Eq. (19) and from the table in Belgacem and Karaballi [30], we get

$$ y\left( t \right) = t^{2} - t. $$
(20)

This is the analytical result of Eq. (18).

Example 3

Further, consider the following Bagley-Torvik equation in Ford and Connolly [34]

$$ \left\{ {\begin{array}{*{20}l} {y^{\left( 2 \right)} \left( t \right) + y^{{\left( {\frac{1}{2}} \right)}} \left( t \right) + y\left( t \right) = t^{2} + 2 + \frac{{2.6666666667t^{1.5} }}{{\varGamma \left( {0.5} \right)}},} \hfill \\ {y\left( 0 \right) = \left. {\frac{dy\left( t \right)}{dt}} \right|_{t = 0} = 0,\quad t \ge 0.} \hfill \\ \end{array} } \right. $$
(21)

First, apply STM on both sides of Eq. (21) and initial condition to get

$$ \begin{aligned} & \frac{F\left( u \right)}{{u^{2} }} + u^{{\frac{ - 1}{2}}} F\left( u \right) + F\left( u \right) = 2u^{2} + 2 + \frac{3}{4} \times 2.6666666667 \times u^{{\frac{3}{2}}} , \\ & \quad F\left( u \right)\left( {\frac{{1 + u^{2} + u^{{\frac{3}{2}}} }}{{u^{2} }}} \right) = 2u^{2} + 2 + 2u^{{\frac{3}{2}}} , \\ & \quad \Rightarrow F\left( u \right) = 2u^{2} . \\ \end{aligned} $$
(22)

Applying inverse ST to Eq. (22) and using the table in Belgacem and Karaballi [30], we find the analytical solution of Eq. (22) as follows

$$ y\left( t \right) = t^{2} $$
(23)

Example 4

Finally, let us take the Bagley-Torvik equation in Ford and Connolly [34]

$$ \left\{ {\begin{array}{*{20}l} {y^{\left( 2 \right)} \left( t \right) + y^{{\left( {\frac{1}{2}} \right)}} \left( t \right) + y\left( t \right) = t^{3} + 6t + \frac{{3.2t^{2.5} }}{{\varGamma \left( {0.5} \right)}},} \hfill \\ {y\left( 0 \right) = \left. {\frac{dy\left( t \right)}{dt}} \right|_{t = 0} = 0,\quad t \ge 0.} \hfill \\ \end{array} } \right. $$
(24)

Taking ST on both sides of Eq. (24) and initial condition, the subsequent equation is obtained as

$$ \begin{aligned} & F\left( u \right)\left( {\frac{{1 + u^{2} + u^{{\frac{3}{2}}} }}{{u^{2} }}} \right) = 6\left( {u^{3} + u + u^{{\frac{5}{2}}} } \right), \\ & \quad \Rightarrow F\left( u \right) = 6u^{3} . \\ \end{aligned} $$
(25)

Applying inverse ST to Eq. (25) and from the table in Belgacem and Karaballi [30], we have

$$ y\left( t \right) = t^{3} . $$
(26)

which is the exact solution of Eq. (24).

5 Conclusion

In this paper, STM is successfully applied to solve Bagley-Torvik equations. Four examples are solved by STM which show that it is a very useful and highly effective technique in term of yielding an analytical solution. Due to its properties, it is mostly used for solving a different kind of linear and nonlinear fractional differential equation for finding the exact solution.