Skip to main content
SpringerLink
Log in

Combining the Shooting Method with an Operational Matrix Method to Solve Two Point Boundary Value Problems

  • Original Paper
  • Published:
International Journal of Applied and Computational Mathematics Aims and scope Submit manuscript

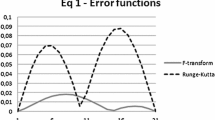
Abstract

An operational matrix method is a well known method to solve an initial value problem (IVP). A recent contribution to the operational matrix method is frame operational matrix (FOM) method. The shooting method is a standard method which converts a boundary value problem (BVP) into an IVP. The BVP is then solved by solving the IVP. In this paper, we combine the shooting method with that of the FOM method to solve two point BVPs. In short, this method is described as the following. First, we convert the BVP into an IVP by shooting method. The IVP is then solved by the FOM method. The approximate solution thus obtained is checked whether it satisfy both the boundary condition. If not, then we take better approximations until they satisfy both the boundary condition. To get a better approximation, we use the Newton’s iteration method.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12

Similar content being viewed by others

References

  1. Ahsan, M., Farrukh, S.: A new type of shooting method for nonlinear boundary value problems. Alex. Eng. J. 52, 801–805 (2013)

    Article  Google Scholar 

  2. Alijani, Z., Baleanu, D., Shiri, B., Wu, G.C.: Spline collocation methods for systems of fuzzy fractional differential equations. Chaos Soliton Fractals 131, 109510 (2020)

    Article  MathSciNet  Google Scholar 

  3. Atkinson, K.E.: An Introductiobn To Numerical Analysis. Wiley, Hoboken (1989)

    Google Scholar 

  4. Aydogan, S.M., Baleanu, D., Mohammadi, H., Rezapour, S.: On the mathematical model of Rabies by using the fractional Caputo–Fabrizio derivative. Adv. Differ. Equ. 382, 1–21 (2020)

    MathSciNet  Google Scholar 

  5. Baleanu, D., Shiri, B.: Collocation methods for fractional differential equations involving non-singular kernel. Chaos Soliton Fractals 116, 136–145 (2018)

    Article  MathSciNet  Google Scholar 

  6. Baleanu, D., Etemad, S., Rezapour, S.: A hybrid Caputo fractional modeling for thermostat with hybrid boundary value conditions. Bound. Value Probl. 64, 1–16 (2020)

    MathSciNet  Google Scholar 

  7. Baleanu, D., Mohammadi, H., Rezapour, S.: A fractional differential equation model for the COVID-19 transmission by using the Caputo–Fabrizio derivative. Adv. Differ. Equ. 299, 1–27 (2020)

    MathSciNet  Google Scholar 

  8. Baleanu, D., Mohammadi, H., Rezapour, S.: A mathematical theoretical study of a particular system of Caputo-Fabrizio fractional differential equations for the Rubella disease model. Adv. Differ. Equ. 184, 1–19 (2020)

    MathSciNet  Google Scholar 

  9. Baleanu, D., Mohammadi, H., Rezapour, S.: Analysis of the model of HIV-1 infection of CD4+ T-cell with a new approach of fractional derivative. Adv Differ Equ 71, 1–17 (2020)

    MathSciNet  Google Scholar 

  10. Bulirsch, R.: Die Mehrzielmethode zur Numerischen Losung von Nichtlinearen Randwertproblemen und Aufgaben der Optimalen Steuerung, Vortrag im Lehrgang “Flugbahnoptimierung” der Carl-Cranz-Gesellschaft, West Germany (1971)

  11. Bulirsch, R., Stoer, J., Deuflhard, P.: Numerical Solution of Nonlinear Two-Point Boundary Value Problems. Numerische Mathematik, L Handbook Series Approximation (in press) (1978)

  12. Chen, C., Hsiao, C.H.: Haar wavelet method for solving lumped and distributed parameter system. IEE Proc. Control Theory Appl. 144, 87–94 (1997)

    Article  Google Scholar 

  13. Christensen, O.: An Introduction to Frames and Riesz Basis. Birkhauser, Berlin (2003)

    Book  Google Scholar 

  14. Chui, C.K.: An Introduction to Wavelets. Academic Press, San Diego (1992)

    MATH  Google Scholar 

  15. Chui, C.K., Wenjie, H.: Compactly supported tight frames associated with refinable functions. Appl. Comput. Harmon. Anal. 8, 293–319 (2000)

    Article  MathSciNet  Google Scholar 

  16. Dadkhah, E., Shiri, B., Ghaffarzadeh, H., Baleanu, D.: Visco-elastic dampers in structural buildings and numerical solution with spline collocation methods. J. Appl. Math. Comput. 63, 29–57 (2019)

    Article  MathSciNet  Google Scholar 

  17. Daubechies, I.: Ten Lectures on Wavelet. SIAM, Philadelphia (1992)

    Book  Google Scholar 

  18. Duffin, R.J., Schaeffer, A.C.: A class of nonharmonic Fourier Series. Trans. Am. Math. Soc. 72, 341–366 (1952)

    Article  MathSciNet  Google Scholar 

  19. EI-Kalla, I.L.: Piecewise continuous solution to a class of nonlinear boundary value problem, Ain Shams Eng. J., 4, 325–331(2013)

  20. Goodman, T.R., Lance, G.N.: The numerical solution of two-point boundary value problems. Math. Tables Other Aids Comput. 10, 82–86 (1956)

    Article  MathSciNet  Google Scholar 

  21. Jena, M.K., Sahu, K.S.: Haar wavelet operational matrix method to solve initial value problems: a short survey. Int. J. Appl. Comput. Math. 3, 3961–3975 (2017)

    Article  MathSciNet  Google Scholar 

  22. Jena, M.K., Sahu, K.S.: Operational matrices from a frame and their applications in solving boundary value problems with mixed boundary conditions. Int. J. Appl. Comput. Math. 4, 127 (2018)

    Article  MathSciNet  Google Scholar 

  23. Keller, H.B.: Numerical methods for two point boundary value problems. Ginn-Blaisdell, Waltham (1968)

    MATH  Google Scholar 

  24. Keller, H.B.: Numerical Solution of Two Point Boundary Value Problems. SIAM, Philadelphia (1976)

    Book  Google Scholar 

  25. Khiabani, E.D., Ghaffarzadeh, H., Shiri, B., Katebi, J.: Spline collocation methods for seismic analysis of multiple degree of freedom systems with visco-elastic dampers using fractional models. J. Vib. Control 26, 1445–1465 (2020)

    Article  MathSciNet  Google Scholar 

  26. Lepik, U.: Numerical solution of differential equations using Haar wavelets. Math. Comput. Simul. 68, 127–143 (2005)

    Article  MathSciNet  Google Scholar 

  27. Lepik, U.: Solving fractional integral equations by the Haar wavelet method. Appl. Math. Comput. 214, 468–478 (2009)

    Article  MathSciNet  Google Scholar 

  28. Lepik, U., Hein, H.: Haar Wavelets with Applications. Springer, Berlin (2014)

    Book  Google Scholar 

  29. Liu, C.: Efficient shooting methods for second order ordinary differential equations. Comput. Model. Eng. Sci. 15, 69–86 (2006)

    MathSciNet  MATH  Google Scholar 

  30. Matinfar, M., Ghasemi, M.: Solving BVPs with shooting method and VIMHP. J. Egypt. Math. Soc. 21, 354–360 (2013)

    Article  MathSciNet  Google Scholar 

  31. Matthij, R.M., Staarink, G.: An efficient algorithm for solving general linear two-point BVP. SIAM J. Sci. Comput. 5, 745–763 (1984)

    Article  MathSciNet  Google Scholar 

  32. Mehra, M.: Wavelet-Galerkin Methods. In: Wavelets Theory and its Applications. Forum for Interdisciplinary Mathematics. Springer, Singapore, pp. 121–133 (2018)

  33. Morrison, D.D., Riley, J.D., Zancanaro, J.F.: Multiple shooting method for two-point boundary value problems. Commun. ACM 5, 613–614 (1962)

    Article  MathSciNet  Google Scholar 

  34. Rezapour, S., Mohammadi, H., Jajarmi, A.: A new mathematical model for Zika virus transmission. Adv Differ. Equ. 589, 1–5 (2020)

    MathSciNet  Google Scholar 

  35. Roberts, S.M., Shipman, J.S.: Continuation in shooting methods for two-point boundary value problems. J. Math. Anal. Appl. 18, 45–58 (1967)

    Article  MathSciNet  Google Scholar 

  36. Sahu, K.S., Jena, M.K.: Solution of initial value problems using an operational matrix. Int. J. Appl. Comput. Math. 6(61), 1–23 (2020)

    MathSciNet  MATH  Google Scholar 

  37. Shiri, B., Baleanu, D.: System of fractional differential algebraic equations with applications. Chaos Soliton Fractals 120, 203–212 (2019)

    Article  MathSciNet  Google Scholar 

  38. Tuan, N.H., Mohammadi, H., Rezapour, S.: A mathematical model for COVID-19 transmission by using the Caputo fractional derivative. Chaos Soliton Fractals 140, 1–11 (2020)

    Article  MathSciNet  Google Scholar 

  39. Xu, J.C., Shann, W.C.: Galerkin-wavelet methods for two-point boundary value problems. Numer. Math. 63, 123–144 (1992)

    Article  MathSciNet  Google Scholar 

  40. You Ma, C., Shiri, B., Wu, G.C., Baleanu, D.: New fractional signal smoothing equations with short memory and variable order. Optik 218, 164507 (2020)

    Article  Google Scholar 

Download references

Acknowledgements

We would like to express our great appreciation to the reviewers for their valuable and constructive suggestions which improved the quality of the article.

Funding

None.

Author information

Authors and Affiliations

  1. Department of Mathematics, Veer Surendra Sai University of Technology, Burla, Sambalpur, Odisha, 768018, India

    Kshama Sagar Sahu & Mahendra Kumar Jena

Authors
  1. Kshama Sagar Sahu
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Mahendra Kumar Jena
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Kshama Sagar Sahu.

Ethics declarations

Conflict of interest

The authors declare that they have no conflict of interest.

Author’s contribution

KSS and MKJ formulated this research to solve boundary value problem. The major part of this research is carried out by KSS.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Supplementary Information

Below is the link to the electronic supplementary material.

Supplementary material 1 (docx 18 KB)

Appendix

Appendix

Frame Matrix for \(J=0\left( M=3\right) \)

$$\begin{aligned} F_0= & {} \left( \begin{array}{ccc} \frac{2}{3} &{} 2 &{} \frac{2}{3} \\ \\ -\frac{1121}{1189} &{} 0 &{} \frac{1121}{1189} \\ \\ -\frac{2}{3} &{} 2 &{} -\frac{2}{3} \end{array}\right) .\\ F_1= & {} \left( \begin{array}{ccc} \frac{1}{18} &{} \frac{1}{2} &{} \frac{17}{18} \\ \\ -\frac{419}{5333} &{} -\frac{1189}{3363} &{} -\frac{419}{5333} \\ \\ -\frac{1}{18} &{} 0 &{} \frac{1}{18} \end{array}\right) . \end{aligned}$$

Operational Matrix for \(J=0\left( M=3\right) \)

$$\begin{aligned} P_1= & {} \left( \begin{array}{ccc} \frac{1}{2} &{} \frac{1121}{2378} &{} -\frac{1}{4} \\ \\ -\frac{1138}{7725} &{} 0 &{} -\frac{1155}{39202} \\ \\ 0 &{} \frac{1155}{19601} &{} 0 \end{array}\right) . \end{aligned}$$

Frame Matrix for \(J=1\left( M=7\right) \)

$$\begin{aligned} F_0= & {} \left( \begin{array}{ccccccc} \frac{2}{7} &{} \frac{6}{7} &{} \frac{10}{7} &{} 2 &{} \frac{10}{7}&{} \frac{6}{7} &{} \frac{2}{7}\\ \\ -\frac{398}{985} &{} -\frac{1194}{985} &{} -\frac{796}{985} &{} 0 &{} \frac{796}{985} &{} \frac{1194}{985} &{} \frac{398}{598} \\ \\ -\frac{796}{985} &{} -\frac{398}{985} &{} \frac{1194}{985} &{} 0 &{} 0 &{} 0 &{} 0 \\ \\ 0 &{} 0 &{} 0 &{} 0 &{} -\frac{1194}{985} &{} \frac{398}{985} &{} \frac{796}{985} \\ \\ -\frac{2}{7} &{} -\frac{6}{7} &{} \frac{2}{7} &{} 2 &{} \frac{2}{7} &{} -\frac{6}{7} &{} -\frac{2}{7} \\ \\ -\frac{4}{7} &{} \frac{8}{7} &{} -\frac{4}{7} &{} 0 &{} 0 &{} 0 &{} 0 \\ \\ 0 &{} 0 &{} 0 &{} 0 &{} -\frac{4}{7} &{} \frac{8}{7} &{} -\frac{4}{7} \end{array}\right) .\\ F_1= & {} \left( \begin{array}{ccccccc} \frac{1}{98} &{} \frac{9}{98} &{} \frac{25}{98} &{} \frac{1}{2} &{} \frac{73}{98} &{} \frac{89}{98} &{} \frac{97}{98} \\ \\ -\frac{226}{15661}&{} -\frac{253}{1948} &{}-\frac{447}{1511} &{} -\frac{1189}{3363}&{} -\frac{447}{1511}&{} -\frac{253}{1948}&{}-\frac{226}{15661} \\ \\ -\frac{253}{4383} &{} -\frac{371}{1094} &{} -\frac{393}{1757} &{} 0 &{} 0 &{} 0 &{} 0 \\ \\ 0 &{} 0 &{} 0 &{} 0 &{} -\frac{393}{1757} &{} -\frac{371}{1094} &{} -\frac{253}{4383} \\ \\ -\frac{1}{98} &{} -\frac{9}{98} &{} -\frac{8}{49} &{} 0 &{} \frac{8}{49}&{} \frac{9}{98} &{}\frac{1}{98} \\ \\ -\frac{2}{49} &{} -\frac{11}{98}&{} \frac{15}{98}&{} 0 &{} 0 &{} 0 &{} 0 \\ \\ 0 &{} 0 &{} 0 &{} 0 &{} -\frac{15}{98} &{} \frac{11}{98}&{} \frac{2}{49} \end{array}\right) . \end{aligned}$$

Operational Matrix for \(J=1\left( M=7\right) \)

$$\begin{aligned} P_1= & {} \left( \begin{array}{ccccccc} \frac{1}{2} &{} \frac{1121}{2378} &{} \frac{66}{19601} &{} \frac{65}{152} &{} -\frac{1}{4} &{} \frac{2}{105} &{} -\frac{44}{105}\\ \\ -\frac{580}{3361} &{} 0 &{} -\frac{17}{420} &{} \frac{17}{420} &{} -\frac{165}{39202} &{} -\frac{33}{19601} &{} -\frac{33}{19601}\\ \\ -\frac{336}{3713} &{} \frac{43}{336} &{} -\frac{13}{420} &{} 0 &{} \frac{336}{3713} &{} -\frac{83}{2293} &{} 0 \\ \\ -\frac{336}{3713} &{} -\frac{43}{336} &{} 0 &{} \frac{13}{420} &{} \frac{336}{3713} &{} 0 &{} -\frac{83}{2293}\\ \\ 0 &{} \frac{529}{4834} &{} -\frac{130}{2413} &{} -\frac{130}{2413} &{} 0 &{} \frac{1}{60} &{} -\frac{1}{60}\\ \\ 0 &{} 0 &{} \frac{195}{2032} &{} 0 &{} 0 &{} -\frac{9}{140} &{} 0\\ \\ 0 &{} 0 &{} 0 &{} \frac{195}{2032} &{} 0 &{} 0 &{} \frac{9}{40} \end{array} \right) . \end{aligned}$$

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Sahu, K.S., Jena, M.K. Combining the Shooting Method with an Operational Matrix Method to Solve Two Point Boundary Value Problems. Int. J. Appl. Comput. Math 7, 29 (2021). https://doi.org/10.1007/s40819-021-00967-x

Download citation

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s40819-021-00967-x

Keywords

Mathematics Subject Classification

Search

Navigation