Skip to main content
SpringerLink
Log in

Stoneley waves in a vicinity of the Wiechert condition

  • Published:
International Journal of Dynamics and Control Aims and scope Submit manuscript

Abstract

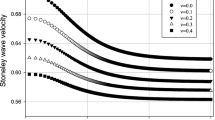
In various areas of the acoustic NDT starting from nano and micro scales to geophysical scales, the high frequency Stoneley waves can give essential information on the physical properties of the adjacent layers along with information on possible interfacial cracks and other defects. The Wiechert condition imposed on the relation between bulk wave velocities of the contacting layers, play an important role in acoustic analyses, especially at analyzing high-frequency Stoneley waves arising and propagating along the interfaces. The present study concerns with a non-propagating condition for Stoneley waves at the vicinity of the Wiechert condition.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2

Similar content being viewed by others

References

  1. Stoneley R (1924) Elastic waves at the surface of separation of two solids. Proc R Soc Lond Ser A Math Phys Sci 106:416–428

    MATH  Google Scholar 

  2. Sezawa K (1938) Formation of boundary waves at the surface of a discontinuity within the Earth's crust. Bull Earthq Res Inst Tokyo Univ 16:504–511

    Google Scholar 

  3. Sezawa K, Kanai K (1939) The range of possible existence of Stoneley waves, and some related problems. Bull Earthq Res Inst Tokyo Univ 17:1–8

    Google Scholar 

  4. Cagniard L (1939) Reflexion et Refraction des Ondes Seismiques Progressive. Gauthier- Villard, Paris

    Google Scholar 

  5. Scholte JG (1942) On the Stoneley wave equation. Proc Koninklijke Nederlandsche Akademie van Weten-schappen 45:159–164

    MathSciNet  MATH  Google Scholar 

  6. Scholte JG (1947) The range of existence of Rayleigh and Stoneley waves. Geophys J Int 5(5):120–126

    Article  MathSciNet  Google Scholar 

  7. Ginzbarg AS, Strick E (1958) Stoneley-wave velocities for a solid-solid interface. Bull Seismol Soc Am 48(1):51–63

    Google Scholar 

  8. Lim TCT & Musgrave MJP (1970) Stoneley waves in anisotropic media. Nature 225:372

    Google Scholar 

  9. Lee DA, Corbly DM (1977) Use of interface waves for nondestructive inspection. IEEE Trans Sonics Ultrason 24:206–212

    Article  Google Scholar 

  10. Murty GS (1975) A theoretical model for the attenuation and dispersion of Stoneley waves at the loosely bonded interface of elastic half spaces. Phys Earth Planet Inter 11:65–79

    Article  Google Scholar 

  11. Murty GS (1975) Wave propagation at an unbounded interface between two elastic half-spaces. J Acoust Soc Am 58:1094–1095

    Article  Google Scholar 

  12. Rokhlin S, Hefet M, Rosen M (1980) An elastic interface wave guided by a thin film between two solids. J Appl Phys 51:3579–3582

    Article  Google Scholar 

  13. Barnett DM, Lothe J, Gavazza SD, Musgrave MJP (1985) Consideration of the existence of interfacial (Stoneley) waves in bonded anisotropic elastic half-spaces. Proc R Soc Lond Ser A Math Phys Sci 412:153–166

    MathSciNet  MATH  Google Scholar 

  14. Stevens JL, Day SM (1986) Shear velocity logging in slow formations using the Stoneley wave. Geophysics 51:137–147

    Article  Google Scholar 

  15. Barnett DM, Gavazza SD, Lothe J (1988) Slip waves along the interface between two anisotropic elastic half-spaces in sliding contact. Proc R Soc Lond Ser A Math Phys Sci 415:389–419

    MATH  Google Scholar 

  16. Norris AN (1989) Stoneley-wave attenuation and dispersion in permeable formations. Geophysics 54(3):330–341

    Article  Google Scholar 

  17. Djeran-Maigre I, Kuznetsov SV (2011) Soliton-like Lamb waves in layered media. In: Vila RP (ed) Waves in fluids and solids. IntechOpen, London

    MATH  Google Scholar 

  18. Chi VP, Ha GPT (2012) Uniqueness of Stoneley waves in pre-stressed incompressible elastic media. Int J Non-Linear Mech 47:128–134

    Article  Google Scholar 

  19. Kuznetsov SV (2019) Abnormal dispersion of flexural Lamb waves in functionally graded plates. Z Angew Math Phys 70(89):1–10

    MathSciNet  MATH  Google Scholar 

  20. Kuznetsov SV (2019) Abnormal dispersion of Lamb waves in stratified media. Z Angew Math Phys 70(175):1–8

    MathSciNet  MATH  Google Scholar 

  21. Kuznetsov SV (2019) Lamb waves in stratified and functionally graded plates: discrepancy, similarity, and convergence. Waves Random Complex Media 30:2–11

    Google Scholar 

  22. Achenbach JD (1987) Wave propagation in elastic solids. North-Holland series in applied mathematics and mechanics, vol 16. North-Holland, Amsterdam

    Google Scholar 

  23. Bailey DH (1993) Automatic translation of Fortran programs to multiprecision. NASA RNR Technical Report, RNR-91-025, pp 1–21

  24. Stoneley R (1934) The transmission of Rayleigh waves in a heterogeneous medium. Mon Not R Astron Soc (Lond) Geophys Suppl 3:222–232

    Article  Google Scholar 

  25. Koppe H (1948) Uber Rayleigh-Wellen an den Oberflache zweier Medien. Z Angew Math Mech 28:355–366

    Article  MathSciNet  Google Scholar 

  26. Eringen AC, Suhibi ES (1971) Elastodynamics: linear theory, vol 2. Academic Press Inc, New York

    Google Scholar 

  27. Takeuchi H, Saito M (1972) Seismic surface waves. In: Bolt BA (ed) Methods of computational physics, vol 11. Academic Press, New York, pp 217–295

    Google Scholar 

  28. Bullen KE, Bolt BA (1985) An introduction to the theory of seismology, 4th edn. Cambridge University Press, Cambridge

    MATH  Google Scholar 

  29. Gaherty JB (2004) A surface wave analysis of seismic anisotropy beneath eastern North America. Geophys J Int 158:1053–1066

    Article  Google Scholar 

  30. Dal Moro G (2014) Surface wave analysis for near surface applications. Elsevier, New York

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Moscow State University of Civil Engineering, Moscow, Russia

    A. V. Ilyashenko

Authors
  1. A. V. Ilyashenko
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to A. V. Ilyashenko.

Additional information

The author’s work was partially supported by the Russian Foundation for Basic Research, Grant No. 20-08-00419.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Ilyashenko, A.V. Stoneley waves in a vicinity of the Wiechert condition. Int. J. Dynam. Control 9, 30–32 (2021). https://doi.org/10.1007/s40435-020-00625-y

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s40435-020-00625-y

Keywords

Search

Navigation