Skip to main content
SpringerLink
Log in

Cross diffusion effects on magnetohydrodynamic slip flow of Carreau liquid over a slendering sheet with non-uniform heat source/sink

  • Technical Paper
  • Published:
Journal of the Brazilian Society of Mechanical Sciences and Engineering Aims and scope Submit manuscript

Abstract

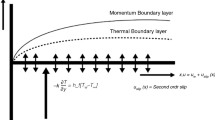
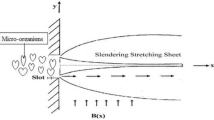
Magnetohydrodynamic flow of Carreau fluid over a slendering sheet (variable thickness) has been numerically studied by considering the multiple slips effect. Thermosolutal boundary layer analysis is also accounted in the presence of cross diffusion and non-uniform heat source/sink. The governing nonlinear coupled partial differential equations are transformed to nonlinear coupled ordinary differential equations before being integrated numerically using Runge–Kutta based Newton’s schemes. The effects of various parameters involved in the present problem were elaborately discussed with help of graphs and tables. The present results in a limiting sense are found to accord with the previous study. The present results indicate that the cross diffusion and slip parameters had a tendency to control the flow. The influence of slip is more evident in Carreau fluid case on contrast with the Newtonian fluid case.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16
Fig. 17
Fig. 18
Fig. 19
Fig. 20
Fig. 21
Fig. 22

Similar content being viewed by others

Abbreviations

u, v :

Velocity components in x and y directions (m/s)

u w :

Stretching sheet velocity

C p :

Specific heat capacity at constant pressure (J/kg K

f :

Dimensionless velocity

A :

Coefficient related to stretching sheet

A*, B*:

Space and temperature dependent heat generation or absorption parameters

m :

Velocity power index parameter

n :

Power law index

B(x):

Magnetic field

U 0 :

Constant.

B 0 :

Constant

C 0 :

Constant

T 0 :

Constant

T :

Temperature of the fluid (K)

k :

Thermal conductivity (W/mK)

D m :

Molecular diffusivity of the species concentration

k T :

Thermal diffusion ratio

C s :

Concentration susceptibility

C :

Concentration of the fluid (Moles/Kg)

T m :

Mean fluid temperature (K)

T ∞ :

Temperature of the fluid in the free

C ∞ :

Concentration of the fluid in the free stream (Mole/Kg)

f 1 :

Maxwell’s reflection coefficient

h *1 :

Dimensional concentration jump parameter

h *2 :

Dimensional concentration jump parameter

h *3 :

Dimensional concentration jump parameter

d :

Concentration accommodation coefficient

a :

Thermal accommodation coefficient

b :

Constant

Pr:

Prandtl number

q′′′:

Space and temperature dependent heat source/sink

We:

Local Weissenberg number

M :

Magnetic parameter

Du:

Dufour number

Sc:

Schmidt number

Sr:

Soret number

h 1 :

Dimensionless velocity slip parameter

h 2 :

Dimensionless temperature jump parameter

h 3 :

Dimensionless concentration jump parameter

C f :

Skin friction coefficient

Nu x :

Local Nusselt number

Sh x :

Local Sherwood number

Re x :

Local Reynolds number

ϕ :

Dimensionless concentration

ζ:

Similarity variable

σ :

Electrical conductivity of the fluid (S/m)

γ :

Ratio of specific heats

θ :

Dimensionless temperature

ρ :

Density of the fluid (Kg/m3)

μ :

Dynamic viscosity (Kg/ms)

ν :

Kinematic viscosity (m2/s)

δ :

Wall thickness parameter

\(\xi_{1} , \xi_{2} , \xi_{3}\) :

Mean free path constants

Γ :

Positive characteristic time

References

  1. Tewfik OE, Eckert ERG, Jurewicz LS (1963) Diffusion-Thermo effects on heat transfer from a cylinder in cross flow. AIAA J 1(7):1537–1543

    Article  Google Scholar 

  2. Ybarra PLG, Velarde MG (1979) The role of Soret and Dufour effects on the stability of a binary gas layer heated from below or above. Geophys Astrophys Fluid Dyn 13:83–94

    Article  Google Scholar 

  3. Hartranft RJ, Sih GC (1980) The influence of the Soret and Dufour effects on the diffusion of heat and moisture in solids. Int J Eng Sci 18:1375–1383

    Article  MATH  Google Scholar 

  4. Vogelsang R, Hoheisel C (1988) The Dufour and Soret coefficients of isotopic mixtures from equilibrium molecular dynamics calculations. J Chem Phys 89:1588–1591

    Article  Google Scholar 

  5. Mohan Hari (1996) The Soret effect on the rotatory thermosolutal convection of the veronis type. Indian J Pure Appl Math 27(6):609–619

    MATH  Google Scholar 

  6. Postelnicu A (2004) Influence of a magnetic field on heat and mass transfer by natural convection from vertical surfaces in porous media considering Soret and Dufour effects. Int J Heat Mass Transf 47:1467–1472

    Article  MATH  Google Scholar 

  7. Postelnicu A (2007) Influence of chemical reaction on heat and mass transfer by natural convection from vertical surfaces in porous media considering Soret and Dufour effects. Heat Mass Transf 43:595–602

    Article  Google Scholar 

  8. Alam MS, Rahman MM (2005) Dufour and Soret effects on MHD free convective heat and mass transfer flow past a vertical porous flat plate embedded in a porous medium. J Naval Archit Mar Eng 1:55–65

    Google Scholar 

  9. Cheng C-Y (2009) Soret and Dufour effects on natural convection heat and mass transfer from a vertical cone in a porous medium. Int Commun Heat Mass Transf 36:1020–1024

    Article  Google Scholar 

  10. Srinivasacharya D, Swamy Reddy G (2013) Soret and Dufour effects on mixed convection from a vertical plate in power-law fluid saturated porous medium. Theoret Appl Mech 40(4):525–542

    Article  MathSciNet  MATH  Google Scholar 

  11. Hayat T, Shehzad SA, Alsaedi A (2012) Soret and Dufour effects on magnetohydrodynamic (MHD) flow of Casson fluid. Appl Math Mech Engl Ed 33(10):1301–1312

    Article  MathSciNet  MATH  Google Scholar 

  12. Hayat T, Abbasi FM, Al-Yami M, Monaquel S (2014) Slip and Joule heating effects in mixed convection peristaltic transport of nanofluid with Soret and Dufour effects. J Mol Liq 194:93–99

    Article  Google Scholar 

  13. Krupa Lakshmi KL, Gireesha BJ, Gorla RSR, Mahanthesh B (2016) Effects of diffusion-thermo and thermo-diffusion on two-phase boundary layer flow past a stretching sheet with fluid-particle suspension and chemical reaction: a numerical study. J Niger Math Soc 35:66–81

    Article  MathSciNet  MATH  Google Scholar 

  14. Khader M, Megahed AM (2003) Numerical solution for boundary layer flow due to a nonlinearly stretching sheet with variable thickness and slip velocity. Eur Phys J Plus 128:100–108

    Article  Google Scholar 

  15. Gireesha BJ, Mahanthesh B, Rashidi MM (2015) MHD boundary layer heat and mass transfer of a chemically reacting Casson fluid over a permeable stretching surface with non-uniform heat source/sink. Int J Ind Math 7:247–260

    Google Scholar 

  16. Fang T, Zhang J, Zhong Y (2012) Boundary layer flow over a stretching sheet with variable thickness. Appl Math Comput 218:7241–7252

    MathSciNet  MATH  Google Scholar 

  17. Anjali Devi SP, Prakash M (2015) Thermal radiation effects on hydromagnetic flow over a slendering stretching sheet. J Braz Soc Mech Sci Eng. https://doi.org/10.1007/s40430-015-0315-7

    MATH  Google Scholar 

  18. Shehzad SA, Abbasi FM, Hayat T, Alsaadi F, Mousa G (2015) Peristalsis in a curved channel with slip condition and radial magnetic field. Int J Heat Mass Transf 91:562–569

    Article  Google Scholar 

  19. Ibrahim W, Shanker B (2013) MHD boundary layer flow and heat transfer of a nanofluid past a permeable stretching sheet with velocity, thermal and solutal slip boundary conditions. Comput Fluids 75:1–10

    Article  MathSciNet  MATH  Google Scholar 

  20. Abelman S, Momoniat E, Hayat T (2009) Couette flow of a third grade fluid with rotating frame and slip condition. Nonlinear Anal Real World Appl 10(6):3329–3334

    Article  MathSciNet  MATH  Google Scholar 

  21. Bég OA, Uddin M, Rashidi MM, Kavyani N (2014) Double-diffusive radiative magnetic mixed convective slip flow with Biot and Richardson number effects. J Eng Thermophys 23(2):79–97

    Article  Google Scholar 

  22. Hayat T, Imtiaz M, Alsaedi A (2015) Partial slip effects in flow over nonlinear stretching surface. Appl Math Mech 36:1513–1526. https://doi.org/10.1007/s10483-015-1999-7

    Article  MathSciNet  MATH  Google Scholar 

  23. Javed M, Hayat T, Mustafa M, Ahmad B (2016) Velocity and thermal slip effects on peristaltic motion of Walters-B fluid. Int J Heat Mass Transf 96:210–217

    Article  Google Scholar 

  24. Khan AA, Sohail A, Rashid S, Rashidi MM, Khan NA (2016) Effects of slip condition variable viscosity and inclined magnetic field on the peristaltic motion of a non-newtonian fluid in an inclined asymmetric channel. J Appl Fluid Mech 9(3):1381–1393

    Article  Google Scholar 

  25. Thammanna GT, Gireesha BJ, Mahanthesh B (2017) Partial slip and joule heating on magnetohydrodynamic radiated flow of nanoliquid with dissipation and convective condition. Results Phys. https://doi.org/10.1016/j.rinp.2017.07.056

    Google Scholar 

  26. Akbar NS, Nadeem S, Haq RU, Ye S (2014) MHD stagnation point flow of Carreau fluid toward a permeable shrinking sheet: dual solutions. Ain Shams Eng J 5:1233–1239. https://doi.org/10.1016/j.asej.2014.05.006

    Article  Google Scholar 

  27. Hayat T, Asad S, Mustafa M, Alsaedi A (2014) Boundary layer flow of Carreau fluid over a convectively heated stretching sheet. Appl Math Comput 246:12–22

    MathSciNet  MATH  Google Scholar 

  28. Khan M (2015) Boundary layer flow and heat transfer to Carreau fluid over a nonlinear stretching sheet. AIP Adv 5:107203. https://doi.org/10.1063/1.4932627

    Article  Google Scholar 

  29. Hayat T, Tanveer A, Alsaedi A (2016) Mixed convective peristaltic flow of Carreau–Yasuda fluid with thermal deposition and chemical reaction. Int J Heat Mass Transf 96:474–481

    Article  Google Scholar 

  30. Abbasi FM, Hayat T, Alsaedi A (2015) Numerical analysis for MHD peristaltic transport of Carreau–Yasuda fluid in a curved channel with Hall effects. J Magn Magn Mater 382:104–110

    Article  Google Scholar 

  31. Ali N, Hayat T (2007) Peristaltic motion of a Carreau fluid in an asymmetric channel. Appl Math Comput 193(2):535–552

    MathSciNet  MATH  Google Scholar 

  32. Hayat T, Saleem N, Ali N (2010) Effect of induced magnetic field on peristaltic transport of a Carreau fluid. Commun Nonlinear Sci Numer Simul 15(9):2407–2423

    Article  MathSciNet  MATH  Google Scholar 

  33. Hayat T, Saleem N, Asghar S, Alhothuali MS, Alhomaidan A (2011) Influence of induced magnetic field and heat transfer on peristaltic transport of a Carreau fluid. Commun Nonlinear Sci Numer Simul 16(9):3559–3577

    Article  MathSciNet  MATH  Google Scholar 

  34. Hayat T, Farooq S, Alsaedi A, Ahmad B (2016) Hall and radial magnetic field effects on radiative peristaltic flow of Carreau-Yasuda fluid in a channel with convective heat and mass transfer. J Magn Magn Mater 412:207–216

    Article  Google Scholar 

  35. Raju CSK, Sandeep N (2016) Falkner Skan flow of a magnetic Carreau fluid past a wedge in the presence of cross diffusion. European Physical Journal Plus 131:267

    Article  Google Scholar 

  36. Gireesha BJ, Kumar PBS, Mahanthesh B, Shehzad SA, Rauf A (2017) Nonlinear 3D flow of Casson-Carreau fluids with homogeneous-heterogeneous reactions: a comparative study. Results in Physics. https://doi.org/10.1016/j.rinp.2017.07.060

    Google Scholar 

  37. Rashidi MM, Laraqi N, Basiri Parsa A (2011) Analytical modeling of heat convection in magnetized micropolar fluid by using modified differential transform method. Heat Transfer-Asian Research 40(3):187–204

    Article  Google Scholar 

  38. Parsa AB, Rashidi MM, Bég OA, Sadri SM (2013) Semi-computational simulation of magneto-hemodynamic flow in a semi-porous channel using optimal homotopy and differential transform methods. Comput Biol Med 43(9):1142–1153

    Article  Google Scholar 

  39. Mabood F, Ibrahim SM, Rashidi MM, Shadloo MS, Lorenzini G (2016) Non-uniform heat source/sink and Soret effects on MHD non-Darcian convective flow past a stretching sheet in a micropolar fluid with radiation. Int J Heat Mass Transf 93:674–682

    Article  Google Scholar 

  40. Bhatti MM, Rashidi MM (2017) Numerical simulation of entropy generation on MHD nanofluid towards a stagnation point flow over a stretching surface. Int J Appl Comput Math 3(3):2275–2289

    Article  MathSciNet  Google Scholar 

  41. Rashad AM, Rashidi MM, Lorenzini G, Sameh EA, Abdelraheem MA (2017) Magnetic field and internal heat generation effects on the free convection in a rectangular cavity filled with a porous medium saturated with Cu–water nanofluid. Int J Heat Mass Transf 104:878–889

    Article  Google Scholar 

  42. Rashidi MM, Yang Z, Awais M, Nawaz M, Hayat T (2017) Generalized magnetic field effects in Burgers’ nanofluid model. PLoS ONE 12(1):e0168923

    Article  Google Scholar 

  43. Khan MI, Hayat T, Khan MI, Alsaedi A (2017) A modified homogeneous-heterogeneous reactions for MHD stagnation flow with viscous dissipation and Joule heating. Int J Heat Mass Transf 113:310–317

    Article  Google Scholar 

  44. Mahanthesh B, Mabood F, Gireesha BJ, Gorla RSR (2017) Effects of chemical reaction and partial slip on the three-dimensional flow of a nanofluid impinging on an exponentially stretching surface. Eur Phys J Plus 132(3):113

    Article  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics, GITAM School of Technology, Bangalore, 562163, India

    C. S. K. Raju

  2. Department of Chemical Engineering, The University of Newcastle, Callaghan, Australia

    M. M. Hoque

  3. Department of Mathematics, Bharatiar University, Coimbatore, India

    P. Priyadharshini

  4. Department of Mathematics, Christ University, Bangalore, 560029, India

    B. Mahanthesh

  5. Department of Studies and Research in Mathematics, Kuvempu University, Shankarghatta, Shimoga, 577451, India

    B. Mahanthesh & B. J. Gireesha

Authors
  1. C. S. K. Raju
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. M. M. Hoque
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. P. Priyadharshini
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. B. Mahanthesh
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. B. J. Gireesha
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to B. J. Gireesha.

Additional information

Technical Editor: Cezar Negrao.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Raju, C.S.K., Hoque, M.M., Priyadharshini, P. et al. Cross diffusion effects on magnetohydrodynamic slip flow of Carreau liquid over a slendering sheet with non-uniform heat source/sink. J Braz. Soc. Mech. Sci. Eng. 40, 222 (2018). https://doi.org/10.1007/s40430-018-1142-4

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s40430-018-1142-4

Keywords

Search

Navigation