Skip to main content
SpringerLink
Log in

Extreme multistability in a fractional-order thin magnetostrictive actuator (TMA)

  • Published:
SeMA Journal Aims and scope Submit manuscript

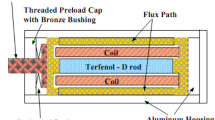
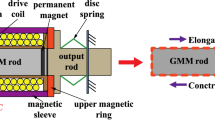
Abstract

In this paper, we consider and investigate the dynamics of a fractional-order thin magnetostrictive actuato with quintic nonlinearity. The energy balance method is used to establish the motion equation of the system. Numerical solutions of the system are studied using the Caputo method and Adams Bashforth Moulton scheme. In order to analyze the dynamical behaviors of the proposed system, some mathematical tools including bifurcation diagrams, Lyapunov exponents, two-parameter bifurcation diagrams, time series plots and phase portraits are exploited. The bifurcation diagrams show that the system under consideration exhibits rich and intriguing behaviors including period-doubling route to chaos, complex phase space trajectory and extreme multistability. One of the most novelty of the proposed system is that its exhibits extreme multistability, which was not yet reported in the existing thin magnetostrictive actuators discussed in the literature to the author’s knowledge. The results carried out in this work may help us in better understanding of the thin magnetostrictive actuators.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14

Similar content being viewed by others

References

  1. Akgul, A.: Chaotic oscillator based on fractional order memcapacitor. J. Circ. Syst. Comput. 28, 1950239 (2019)

    Article  Google Scholar 

  2. Argyris, J., Faust, G., Haase, M.: An Exploration of Chaos, pp. 1–739. North-Holland, Amsterdam (1994)

    MATH  Google Scholar 

  3. Baumann, G.: Fractional calculus. Appl. Results 56(5), 955–966 (2018)

    Google Scholar 

  4. Brzeski, P., Pavlovskaia, E., Kapitaniak, T., Perlikowski, P.: Controlling multistability in coupled systems with soft impacts. Int. J. Mech. Sci. 127, 118–129 (2016)

    Article  Google Scholar 

  5. Cao, Z., Cai, J.: Design of a giant magnetostrictive motor driven by elliptical motion. Sens. Actuators A 118, 332–337 (2005)

    Article  Google Scholar 

  6. Chakraborty, P., Poria, S.: Extreme multistable synchronization in coupled dynamical systems. Pramana J. Phys. 93, 1–13 (2019)

    Article  Google Scholar 

  7. Chengying, L., Jin, Z.: An analysis of a magnetostrictive actuator in nonlinear dynamic. In: Proceeding of the International Conference on Information, Electronic and Computer Science, pp. 1381–1387 (2010)

  8. Garrappa, R.: On linear stability of predictor-corrector algorithms for fractional differential equations. Int. J. Comput. Math. 87, 2281–2290 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  9. Ghodsi, M., Ueno, T., Teshima, H., Hirano, H., Higuchi, T., Summers, E.: “Zero-power” positioning actuator for cryogenic environments by combining magnetostrictive bimetal and HTS. Sens. Actuators A 135, 787–791 (2007)

    Article  Google Scholar 

  10. Grunwald, A., Olabi, A.G.: Design of a magnetostrictive (MS) actuator. Sens. Actuators A 144, 161–175 (2008)

    Article  Google Scholar 

  11. Heaviside, O.: Electromagnetic Theory. Academic Press, New York (1971)

    MATH  Google Scholar 

  12. Hens, C., Dana, S.K., Feudel, U.: Extreme multistability: attractor manipulation and robustness. Interdiscip. J. Nonlinear Sci. 25, 1–8 (2015)

    MathSciNet  MATH  Google Scholar 

  13. Hong, C.: Rapid heating induced vibration of magnetostrictive functionally graded material plates. J. Vib. Acoust. 134, 1–11 (2012)

    Article  Google Scholar 

  14. Hong, C.C.: Application of magnetostrictive actuator. Mater. Des. 46, 617–621 (2013)

    Article  Google Scholar 

  15. Jenner, A.G., Smith, R.J.E., Wilkinson, A.J., Greenough, R.D.: Actuation and transduction by giant magnetostrictive alloys. Mechatronics 10, 457–466 (2000)

    Article  Google Scholar 

  16. Jiaju, Z., Shuying, C., Hongli, W., Wenmei, H.: Hybrid genetic algorithms for parameter identification of a hysteresis model of magnetostrictive actuators. Neurocomputing 70, 749–761 (2007)

    Article  Google Scholar 

  17. Keshtkar, F., Kheiri, H., Erjaee, G.H.: Fixed points classification of nonlinear fractional diferential equations as a dynamical system. J. Fractal Calculus Appl. 5, 59–70 (2014)

    Google Scholar 

  18. Khan, A., Khattar, D., Agrawal, N.: Synchronization of a new fractional order chaotic system. Int. J. Dyn. Control 6, 1585–1591 (2018)

    Article  MathSciNet  Google Scholar 

  19. Krzysztof, C., Szczyglowski, J.: An alternative method to estimate the parameters of Jiles–Atherton model. J. Magn. Magn. Mater. 314, 47–51 (2007)

    Article  Google Scholar 

  20. Lawrence, A.: Vibration analysis and intelligent control of flexible rotor systems using smart materials. Ph. D. Thesis University of Glasgow, pp. 1–246 (2008)

  21. Li, H., Liao, X., Luo, M.: A novel non-equilibrium fractional-order chaotic system and its complete synchronization by circuit implementation. Nonlinear Dyn. 68, 137–149 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  22. Liang, Y.-S.: Progress on estimation of fractal dimensions of fractional calculus of continuous functions. Fractals 27, 1950084 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  23. Liu, Z., Xia, T.: Novel two dimensional fractional-order discrete chaotic map and its application to image encryption. Appl. Comput. Inform. 14, 177–185 (2017)

    Article  Google Scholar 

  24. Ma, C.: Coexistence of multiple attractors for an incommensurate fractional-order chaotic system. Eur. Phys. J. Plus 135(1), 95 (2020)

    Article  Google Scholar 

  25. Nayfeh, A.H., Balachandran, B.: Applied Nonlinear Dynamics: Analytical, Computational and Experimental Method, pp. 1–691. Wiley, New York (1995)

    MATH  Google Scholar 

  26. Ngonghala, C.N., Feudel, U., Showalter, K.: Extreme multistability in a chemical model system. Phys. Rev. E 83–056206, 1–10 (2011)

    Google Scholar 

  27. Nkamgang, G.B., Foagieng, E., Kamdoum, T.V., Talla, P.K., Fomethe, A.: A model for a thin magnetostrictive actuator in nonlinear dynamics. Res. J. Appl. Sci. Eng. Technol. 11, 1245–1256 (2015)

    Article  Google Scholar 

  28. Olabi, A.G., Grunwald, A.: Design and application of magnetostrictive materials. Mater. Des. 29, 469–483 (2008)

    Article  Google Scholar 

  29. Patel, M.S., Patel, U., Sen, A., Sethia, G.C., Hens, C., Dama, S.K., Feudel, U., Showalter, K., Ngonghala, C.N., Amiritkar, R.E.: Experimental observation of extreme multistability in an electronic system of two coupled Rossler oscillators. Phys. Rev. E 89(022918), 1–9 (2014)

    Google Scholar 

  30. Pelap, F.B., Tanekou, G.B., Fogang, C.F., Kengne, R.: Fractional-order stability analysis of earthquake dynamics. J. Geophys. Eng. 15, 1673–1687 (2018)

    Article  Google Scholar 

  31. Pérignon, F.: Vibrations forces des structures minces, élastiques, non linéaires. Ph. D. Thèse Université Aix-Marseille II, pp. 7–37 (2004)

  32. Pham, V.-T., Vaidyanathan, S., Volos, C.K., Azar, A.T., Hoang, T.M., Yem, V.V.: A three-dimensional no-equilibrium chaotic system: analysis, synchronization and its fractional order form. Stud. Comput. Intell. 688, 449–470 (2017)

    Article  MATH  Google Scholar 

  33. Podlubny, I.: Fractional Differential Equation: An Introduction to Fractional Derivatives, Fractional Differential Equations, to Methods of Their Solution and Some of Their Applications, vol. 198, pp. 1–364. Academic Press, San Diego (1999)

    MATH  Google Scholar 

  34. Pretas, I.: Fractional-order Nonlinear Systems, Modeling Analysis and Simulation, pp. 1–235. Springer, New York (2010)

    Google Scholar 

  35. Ram, R., Mohanty, M.N.: Application of fractional calculus in speech enhancement: a novel approach. In: 2nd International Conference on Data Science and Business Analytics (ICDSBA) (2018)

  36. Sridevi, G., Kumar, S.S.: Image enhancement based on fractional calculus and genetic algorithm. In: Proceedings of International Conference on Computational Intelligence and Data Engineering, pp. 197–206 (2019)

  37. Strogatz, S.H.: Nonlinear Dynamics and Chaos, pp. 1–505. Addison-Wesley, Reading (1994)

    Google Scholar 

  38. Sun, H.G., Yong, Z., Baleanu, D., Wen, C., Chen, Y.Q.: A new collection of real world applications of fractional calculus in science and engineering. Commun Nonlinear Sci Numer Simulat 64, 213–231 (2018)

    Article  MATH  Google Scholar 

  39. Tanekou, G.B., Fogang, C.F., Kengne, R., Pelap, F.B.: Lubrication pressure and fractional viscous damping effects on the spring-block model of earthquakes. Eur. Phys. J. Plus 133, 1–13 (2018)

    Article  Google Scholar 

  40. Tirandaz, H., Karami-Mollaee, A.: On active synchronization of fractional-order bloch chaotic system and its practical application in secure image transmission. Int. J. Intell. Comput. Cybern. 11, 1–19 (2018)

    Article  Google Scholar 

  41. Venkataraman, I. R.: Modeling and Adaptive Control of Magnetostrictive Actuators. Ph. D. Thesis University of Maryland, pp. 1–243 (1999)

  42. Venkataraman, I.R., Krishnaprasad, P.S.: On a low-dimensional model for ferromagnetism. Nonlinear Anal. 61, 1447–1482 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  43. Venkataraman, I. R., Rameau, J., Krishnaprasad, P. S.: Characterisation of an extrema MP 50/6 magnetostrictive actuator. Technical Report of the Institude for systems research, University of Maryland at College Park, pp. 1–11 (1998)

  44. Vyawahare, V., Nataraj, P.S.V.: Fractional-order Modeling of Neutron Transport in a Nuclear Reactor. Appl. Math. Modelling 37, 9747–9767 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  45. Wang, T.Z., Zhou, Y.H.: A nonlinear transient constitutive model with eddy current effects for giant magnetostrictive materials. J. Appl. Phys. 108–123905, 1–10 (2010)

    Google Scholar 

  46. Yuan, F., Wang, G., Wang, X.: Extreme multistability in a memristor-based multi-scroll-chaotic system. Interdiscip. J. Nonlinear Sci. 26, 073107 (2016)

    Article  MathSciNet  Google Scholar 

  47. Zhang, X., Li, Z.: Hidden extreme multistability in a novel 4D fractional-order chaotic system. Int. J. Non-Linear Mech. 111, 14–27 (2019)

    Article  Google Scholar 

  48. Zhang, Y., Wang, X., Liu, L., Liu, J.: Fractional order spatiotemporal chaos with delay in spatial nonlinear coupling. Int. J. Bifurc. Chaos 28, 1850020 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  49. Zhang, S., Zeng, Y.: Hidden extreme multistability, antimonotonicity and offset boosting control in a novel fractional-order hyperchaotic system without equilibrium. Int. J. Bifurc. Chaos 13, 1–18 (2018)

    MATH  Google Scholar 

  50. Zhou, C., Li, Z., Xie, F.: Coexisting attractors, crisis route to chaos in a novel 4d fractional-order system and variable-order circuit implementation. Eur. Phys. J. Plus 134, 1–6 (2019)

    Article  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. UR de Mécanique Et de Modélisation Des Systèmes Physiques (UR-2MSP), UFR/DSST, Université de Dschang, BP 67, Dschang, Cameroun

    Zango Nkeutia Sylvain, Nkamgang Gilbert Bruno & Talla Pierre Kisito

  2. Department of Telecommunication and Network Engineering, Fotso Victor University Institute of Technology, University of Dschang, P.O. Box 134, Bandjoun, Cameroon

    Kamdoum Tamba Victor

  3. UR de Matière Condensée, Electronique Et Traitement du Signal (UR-MACETS), UFR/DSST, Université de Dschang, BP 67, Dschang, Cameroun

    Kamdoum Tamba Victor

Authors
  1. Zango Nkeutia Sylvain
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Kamdoum Tamba Victor
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Nkamgang Gilbert Bruno
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Talla Pierre Kisito
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Kamdoum Tamba Victor.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Sylvain, Z.N., Victor, K.T., Bruno, N.G. et al. Extreme multistability in a fractional-order thin magnetostrictive actuator (TMA). SeMA 78, 347–365 (2021). https://doi.org/10.1007/s40324-020-00238-7

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s40324-020-00238-7

Keywords

Mathematics subject classification

Search

Navigation