Abstract
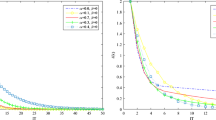
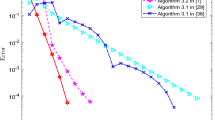
In this paper, we propose a successive mth (\(m\ge 2\)) approximation method for the nonlinear eigenvalue problem (NEP) and analyze its local convergence. Applying the partially orthogonal projection method to the successive mth approximation problem, we present the partially orthogonal projection method with the successive mth approximation for solving the NEP. Numerical experiments are reported to illustrate the effectiveness of the proposed methods.
Similar content being viewed by others
References
Atkinson KE (1989) An Introduction to Numerical Analysis, 2nd edn. Wiley, New York
Bao L, Lin Y, Wei Y (2009) Restarted generalized Krylov subspace methods for solving large-scale polynomial eigenvalue problems. Numer Algorithm 50:17–32
Betcke T, Voss H (2004) A Jacobi–Davidson-type projection method for nonlinear eigenvalue problems. Future Gener Comput Syst 20:363–372
Bramely JS, Dannis SCR (1982) The calculation of eigenvalues for the stationary perturbation of Poiseuille flow. J Comput Phys 47:179–198
Dai H, Bai Z-Z (2010) On smooth LU decompositions with applications to solutions of nonlinear eigenvalue problems. J Comput Math 28:745–766
Guo J-S, Lin W-W, Wang C-S (1995) Nonequivalence deflation for the solution of matrix talent value problems. Linear Algebra Appl 231:15–45
Hoffnung L, Li R-C, Ye Q (2006) Krylov type subspace methods for matrix polynomials. Linear Algebra Appl 415:52–81
Hu HY, Dowell EH, Virgin LN (1998) Stability estimation of high dimensional vibrating systems under state delay feedback control. J Sound Vib 214:497–511
Jarlebring E (2012) Convergence factors of Newton methods for nonlinear eigenvalue problems. Linear Algebra Appl 436:3943–3953
Kim CW, Bennighof JK (2007) Fast frequency response analysis of large-scale structures with nonproportional damping. Int J Numer Methods Eng 69:978–992
Kressner D (2009) A block Newton method for nonlinear eigenvalue problems. Numer Math 114:355–372
Kublanovskaya VN (1970) On an approach to the solution of the generalized latent value problem for lambda-matrices. SIAM J Numer Anal 7:532–537
Lancaster P, Markus AS, Zhou F (2003) Perturbation theory for analytic matrix functions: the semisimple case. SIAM J Matrix Anal Appl 25:606–626
Li R-C (1989) QR decomposition and nonlinear eigenvalue problems (in Chinese). Math Numer Sin 11:374–385
Mehrmann V, Voss H (2004) Nonlinear eigenvalue problems: a challenge for modern eigenvalue methods. GAMM Mitt 27:121–152
Ruhe A (1973) Algorithms for the nonlinear eigenvalue problems. SIAM J Numer Anal 10:674–689
Sun W, Liu K-M (2001) Iterative algorithms for nonlinear ordinary differential eigenvalue problems. Appl Numer Math 38:361–376
Voss H (2003) Numerical methods for sparse nonlinear eigenproblems. In: Marek I (ed) Proceedings of the XVth Summer School on Software and Algorithms of Numerical Mathematics, Hejnice
Voss H (2004) An Arnoldi method for nonlinear eigenvalue problems. BIT 44:387–401
Voss H (2007) A Jacobi–Davidson method for nonlinear and nonsymmetric eigenproblems. Comput Struct 85:1284–1292
Voss H (2009) A minmax principle for nonlinear eigenproblems depending continuously on the eigenparameter. Numer Linear Algebra Appl 16:899–913
Wei W, Dai H (2015) Partially orthogonal projection method and its variations for solving polynomial eigenvalue problem (in Chinese). Numer Math A J Chin Univ (to appear)
Acknowledgments
The authors would like to express their heartfelt thanks to Professor Jinyun Yuan and anonymous referees for their useful comments and suggestions which helped to improve the presentation of this paper. The work is supported by the National Natural Science Foundation of China under Grant No. 11571171 and No. 11401305, the Natural Science Foundation of Jiangsu Province of China under grant BK20141408, and the Fundamental Research Funds for the Central Universities No. NZ2014101. We would like to thank Dr. Jianhua Zhang for his helpful suggestions.
Author information
Authors and Affiliations
Corresponding author
Additional information
Communicated by Jinyun Yuan.
Rights and permissions
About this article
Cite this article
Chen, X., Dai, H. & Wei, W. Successive mth approximation method for the nonlinear eigenvalue problem. Comp. Appl. Math. 36, 1009–1021 (2017). https://doi.org/10.1007/s40314-015-0277-5
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s40314-015-0277-5
Keywords
- Nonlinear eigenvalue problem
- Successive mth approximation method
- Partially orthogonal projection method